User:Tohline/H BookTiledMenu

Tiled Menu

Context

Global Energy Considerations

Principal Governing Equations (PGEs)		Continuity		Euler		1st Law of Thermodynamics		Poisson

 

Equation of State (EOS)		Total Pressure

Spherically Symmetric Configurations

Spherically Symmetric Configurations

 

		Structural Form Factors		Free-Energy of Spherical Systems

One-Dimensional PGEs

Equilibrium Structures

1D STRUCTURE

 

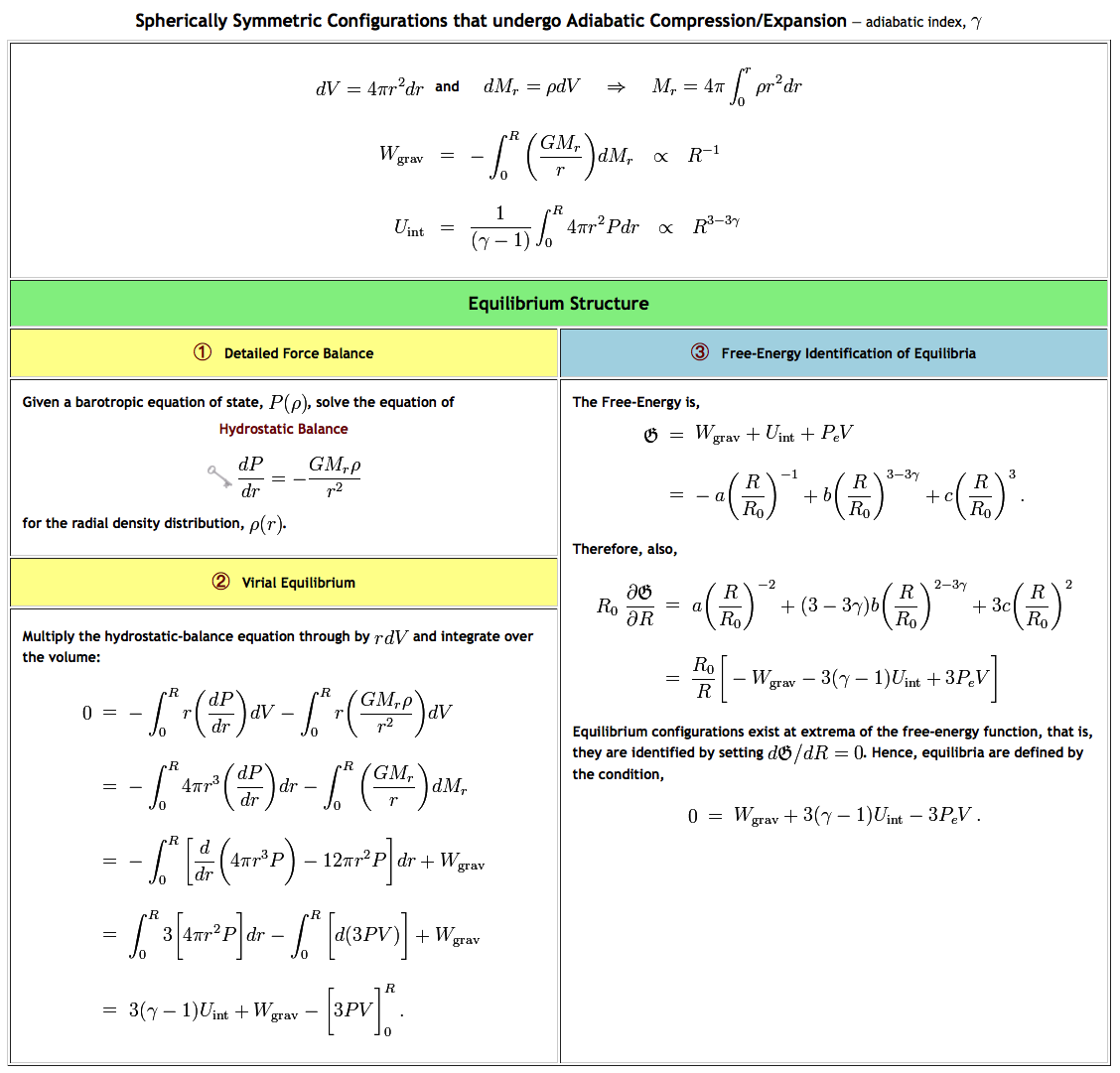
		Scalar Virial Theorem

Hydrostatic Balance Equation		<math>~\frac{dP}{dr} = - \frac{GM_r \rho}{r^2}</math>		Solution Strategies

 

Isothermal Sphere		<math>~\frac{1}{\xi^2} \frac{d}{d\xi}\biggl( \xi^2 \frac{d\psi}{d\xi} \biggr) = e^{-\psi}</math>		via Direct Numerical Integration

 

Isolated Polytropes		<math>~\frac{1}{\xi^2} \frac{d}{d\xi}\biggl( \xi^2 \frac{d\Theta_H}{d\xi} \biggr) = - \Theta_H^n</math>		Known Analytic Solutions		via Direct Numerical Integration		via Self-Consistent Field (SCF) Technique

 

Zero-Temperature White Dwarf		Chandrasekhar Limiting Mass (1935)

 

Pressure-Truncated Configurations		Bonnor-Ebert (Isothermal) Spheres (1955 - 56)		Polytropes		Equilibrium Sequence Turning-Points				Turning-Points (Broader Context)

 

Composite Polytropes (Bipolytropes)		Schönberg- Chandrasekhar Mass (1942)		Analytic <math>~(n_c, n_e)</math> = <math>~(5,1)</math>		Analytic <math>~(n_c, n_e)</math> = <math>~(1,5)</math>

 

Stability Analysis

1D STABILITY

 

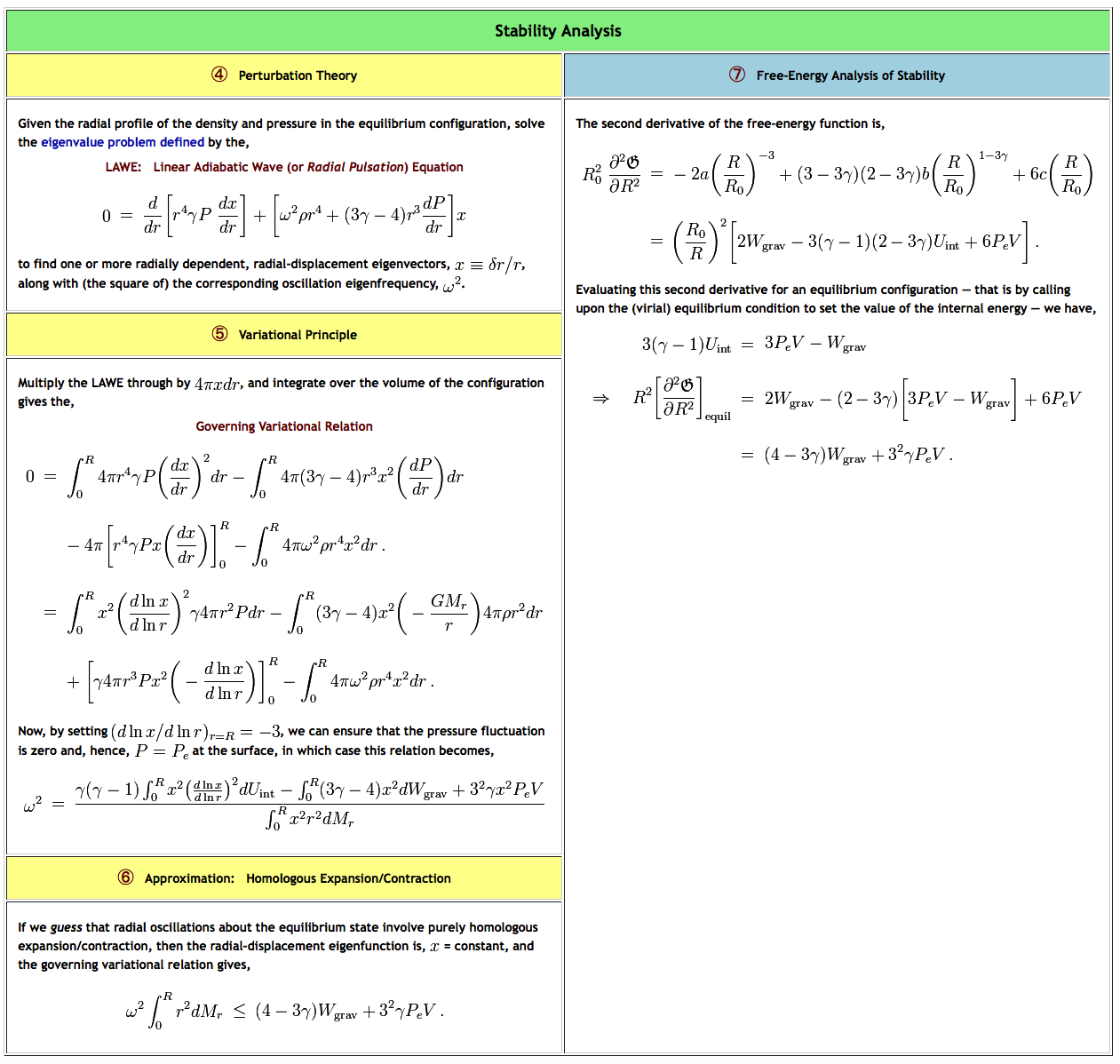
		Variational Principle

Radial Pulsation Equation		Example Derivations & Statement of Eigenvalue Problem		Jeans (1928) or Bonnor (1957)		Ledoux & Walraven (1958)		Rosseland (1969)		Relationship to Sound Waves

 

Uniform-Density Configurations		Sterne's Analytic Sol'n of Eigenvalue Problem (1937)

 

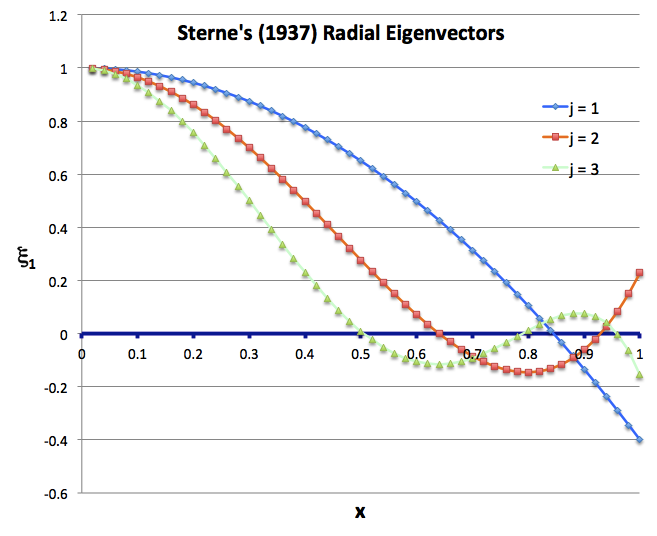
Pressure-Truncated Isothermal Spheres		<math>~0 = \frac{d^2x}{d\xi^2} + \biggl[4 - \xi \biggl( \frac{d\psi}{d\xi} \biggr) \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ \biggl( \frac{\sigma_c^2}{6\gamma_\mathrm{g}}\biggr)\xi^2 - \alpha \xi \biggl( \frac{d\psi}{d\xi} \biggr) \biggr] \frac{x}{\xi^2} </math> where:    <math>~\sigma_c^2 \equiv \frac{3\omega^2}{2\pi G\rho_c}</math>     and,     <math>~\alpha \equiv \biggl(3 - \frac{4}{\gamma_\mathrm{g}}\biggr)</math>		via Direct Numerical Integration

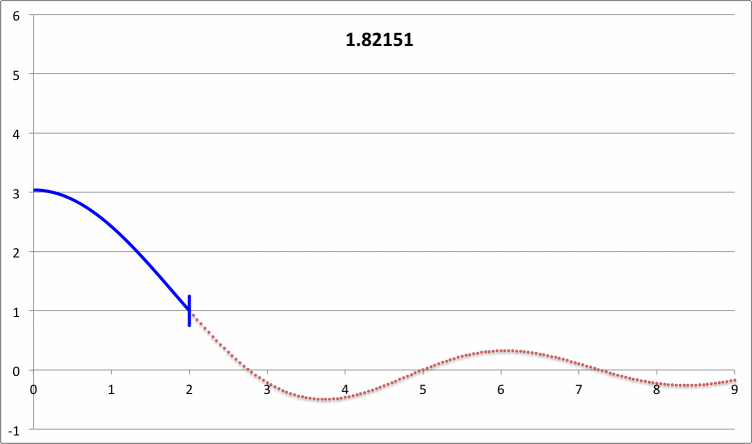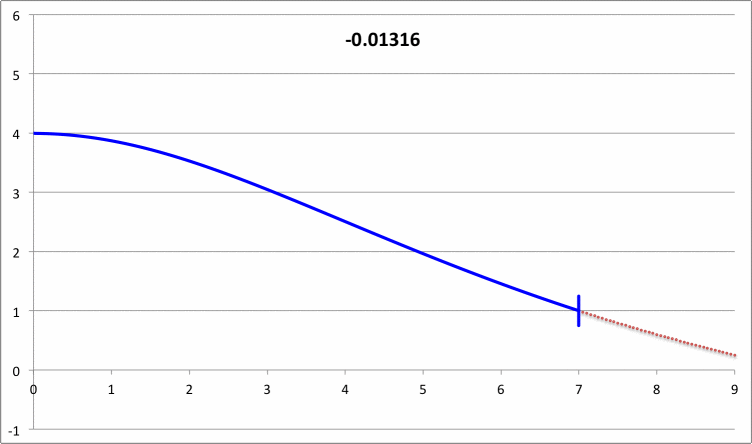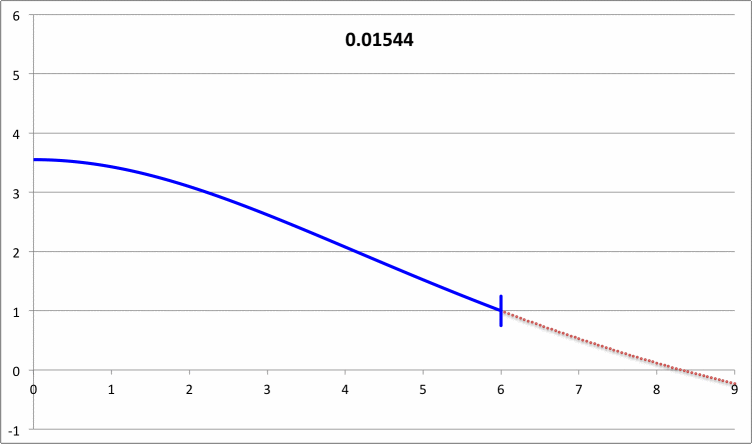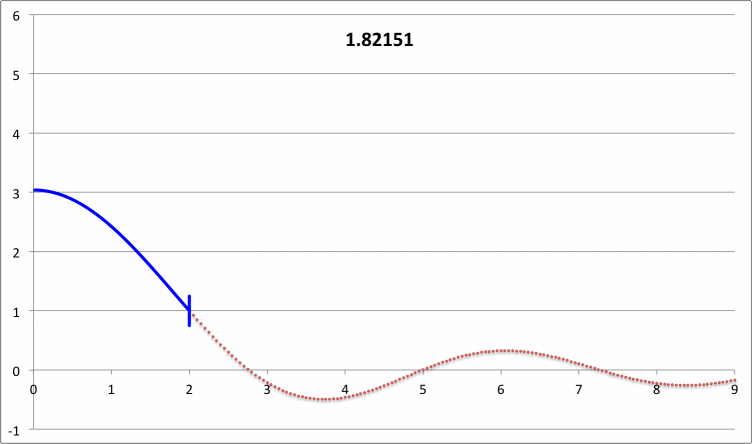
Yabushita's Analytic Sol'n for Marginally Unstable Configurations (1974)		<math>~\sigma_c^2 = 0 \, , ~~~~\gamma_\mathrm{g} = 1</math>  and   <math>~x = 1 - \biggl( \frac{1}{\xi e^{-\psi}}\biggr) \frac{d\psi}{d\xi} </math>

 

Polytropes		<math>~0 = \frac{d^2x}{d\xi^2} + \biggl[ 4 - (n+1) Q \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + (n+1) \biggl[ \biggl( \frac{\sigma_c^2}{6\gamma_g } \biggr) \frac{\xi^2}{\theta} - \alpha Q\biggr] \frac{x}{\xi^2} </math> where:    <math>~Q(\xi) \equiv - \frac{d\ln\theta}{d\ln\xi} \, ,</math>    <math>~\sigma_c^2 \equiv \frac{3\omega^2}{2\pi G\rho_c} \, ,</math>     and,     <math>~\alpha \equiv \biggl(3 - \frac{4}{\gamma_\mathrm{g}}\biggr)</math>		Isolated n = 3 Polytrope				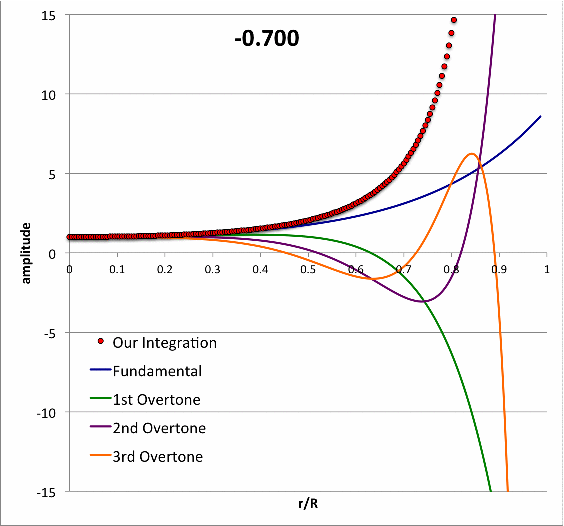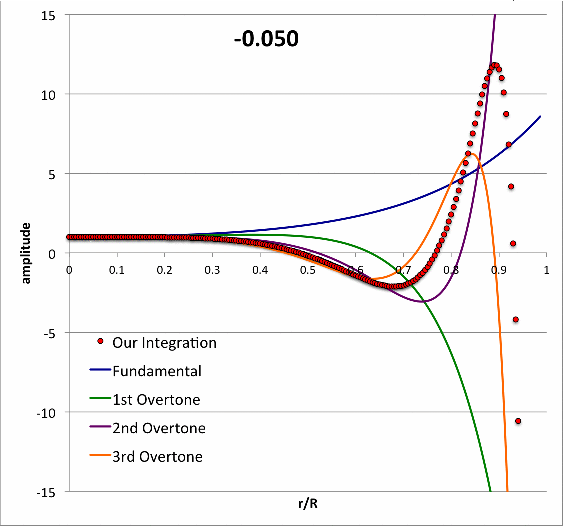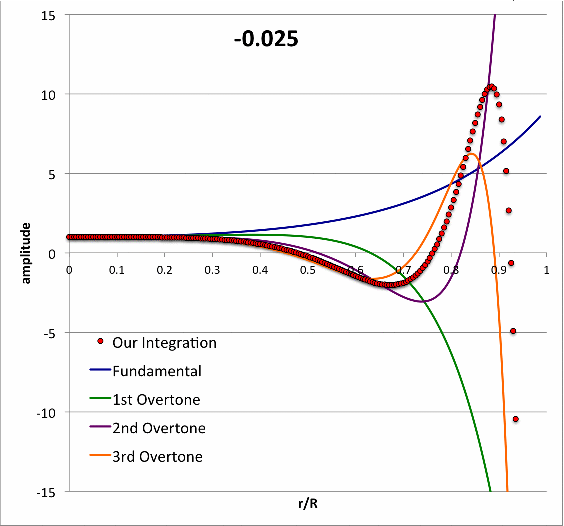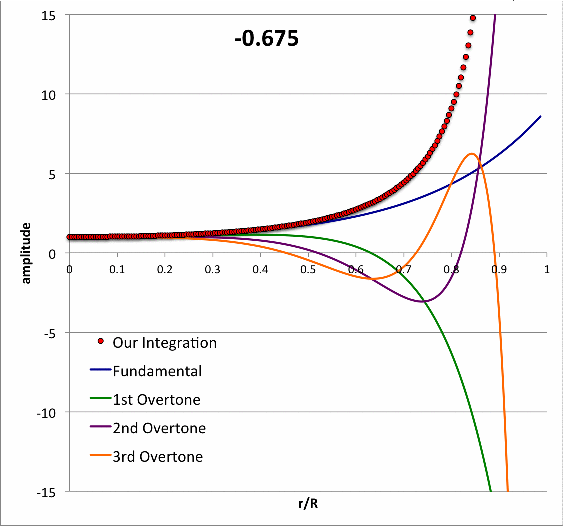Pressure-Truncated Configurations

Exact Demonstration of B-KB74 Conjecture		Exact Demonstration of Variational Principle		Pressure-Truncated n = 5 Polytropes

Our Analytic Sol'n for Marginally Unstable Configurations (2017)		<math>~\sigma_c^2 = 0 \, , ~~~~\gamma_\mathrm{g} = (n+1)/n</math>  and   <math>~x = \frac{3(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{1}{\xi \theta^{n}}\biggr) \frac{d\theta}{d\xi}\biggr] </math>

 

Nonlinear Dynamical Evolution

1D DYNAMICS

 

Free-Fall Collapse

 

Collapse of Isothermal Spheres		via Direct Numerical Integration		Similarity Solution

 

Collapse of an Isolated n = 3 Polytrope

 

Two-Dimensional Configurations (Axisymmetric)

Axisymmetric Configurations

 

PGEs for Axisymmetric Systems

Axisymmetric Equilibrium Structures

2D STRUCTURE

 

Constructing Axisymmetric Equilibrium Configurations		Axisymmetric Instabilities to Avoid		Simple Rotation Profiles		Hachisu Self-Consistent-Field [HSCF] Technique		Solving the Poisson Equation

 

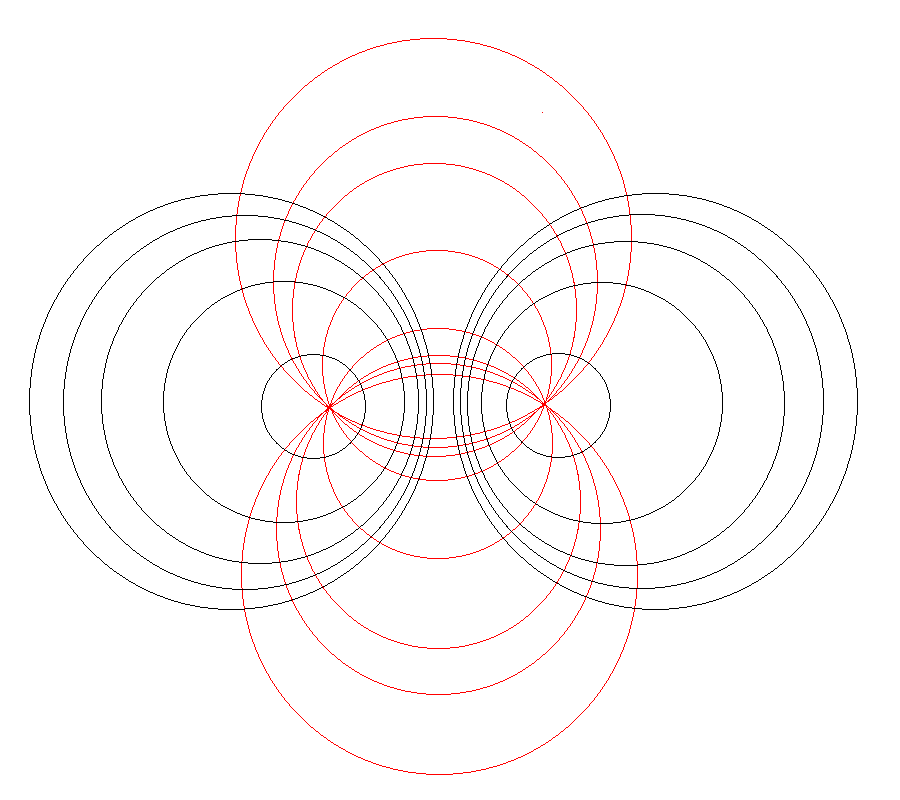
Using Toroidal Coordinates to Determine the Gravitational Potential				Attempt at Simplification		Wong's Analytic Potential (1973)

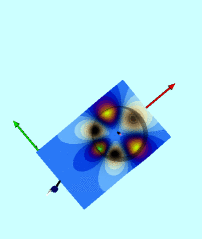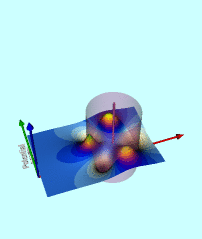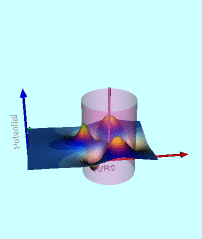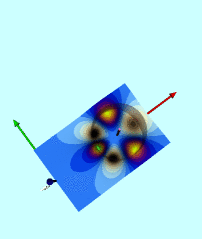
Spheroidal & Spheroidal-Like

Uniform-Density (Maclaurin) Spheroids		Maclaurin's Original Text & Analysis (1742)

 

Rotationally Flattened Isothermal Configurations		Hayashi, Narita & Miyama's Analytic Sol'n (1982)		Review of Stahler's (1983) Technique

Toroidal & Toroidal-Like

Definition: anchor ring

Massless Polytropic Configurations		Papaloizou-Pringle Tori (1984)

 

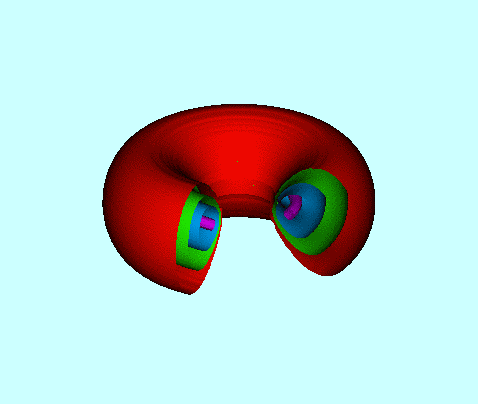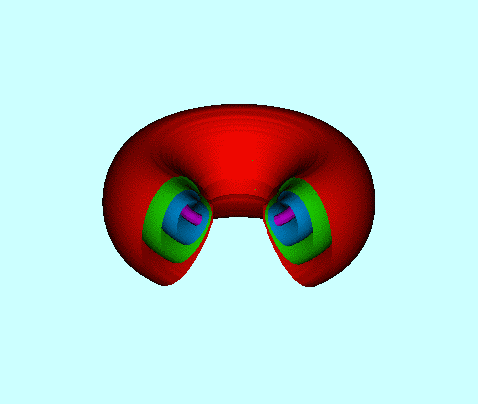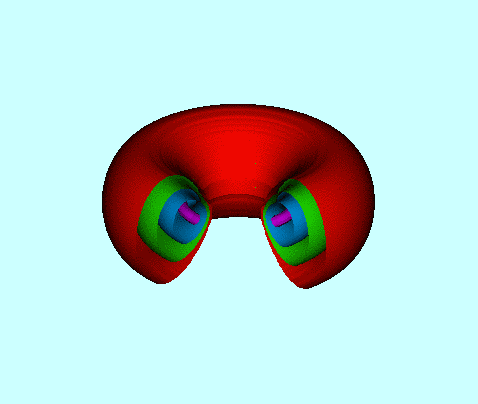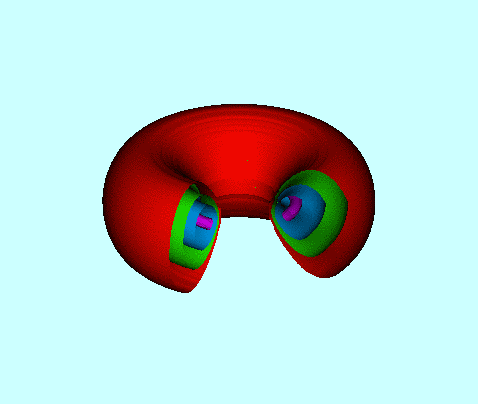
Self-Gravitating Incompressible Configurations		Dyson (1893)		Dyson-Wong Tori

 

Self-Gravitating Compressible Configurations		Ostriker (1964)

 

Stability Analysis

2D STABILITY

 

Toroidal & Toroidal-Like

Defining the Eigenvalue Problem

 

(Massless) Papaloizou-Pringle Tori		Analytic Analysis by Blaes (1985)

 

Two-Dimensional Configurations (Nonaxisymmetric Disks)

Infinitesimally Thin, Nonaxisymmetric Disks

 

2D STRUCTURE

 

Constructing Infinitesimally Thin Nonaxisymmetric Disks

 

Three-Dimensional Configurations

Three-Dimensional Configurations

 

Equilibrium Structures

3D STRUCTURE

 

Ellipsoidal & Ellipsoidal-Like

Constructing Ellipsoidal & Ellipsoidal-Like Configurations

 

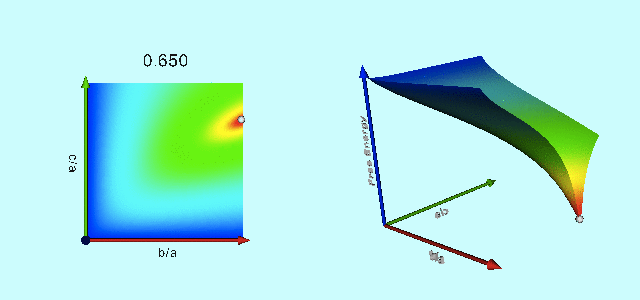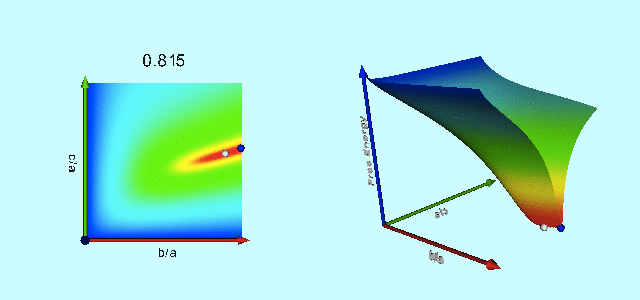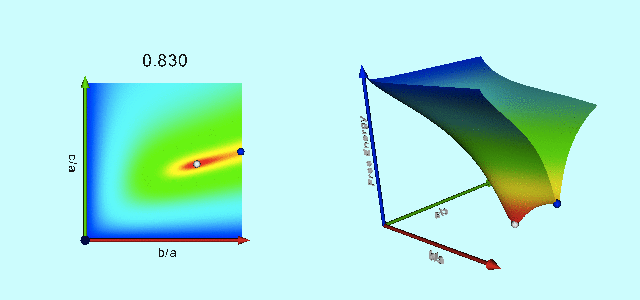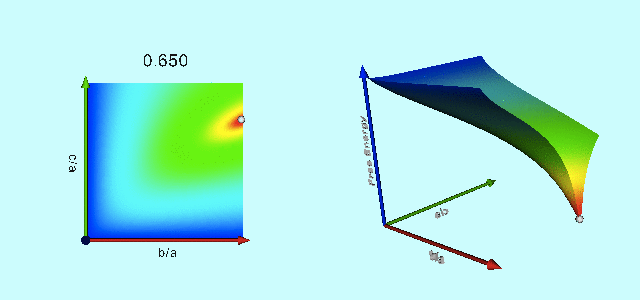
		Free-Energy Evolution from the Maclaurin to the Jacobi Sequence

Jacobi Ellipsoids

 

See Also

© 2014 - 2021 by Joel E. Tohline |   H_Book Home   |   YouTube   | Appendices: | Equations | Variables | References | Ramblings | Images | myphys.lsu | ADS | Recommended citation:   Tohline, Joel E. (2021), The Structure, Stability, & Dynamics of Self-Gravitating Fluids, a (MediaWiki-based) Vistrails.org publication, https://www.vistrails.org/index.php/User:Tohline/citation