Difference between revisions of "User:Tohline/H BookTiledMenu"
m (1 revision: Joel edits) |
|||
| (227 intermediate revisions by the same user not shown) | |||
| Line 5: | Line 5: | ||
{{LSU_HBook_header}} | {{LSU_HBook_header}} | ||
<font size="+1"> | |||
[Preamble by Joel E. Tohline — ''Circa'' 2020] In an effort to serve ongoing research activities of the astrophysics community, this media-wiki-based hypertext book (H_Book) reviews what is presently understood about the structure, stability, and dynamical evolution of (Newtonian) self-gravitating fluids. In the context of this review, the "dynamical evolution" categorization generally will refer to studies that begin with an equilibrium configuration that has been identified — via a stability analysis — as being dynamically (or, perhaps, secularly) unstable then, using numerical hydrodynamic technique, follow the development to nonlinear amplitude of deformations that spontaneously develop as a result of the identified instability. | |||
As is reflected in our choice of the overarching set of ''Principle Governing Equations,'' our focus is on studies of ''compressible'' fluid systems — those that obey a barotropic equation of state. But, in addition, chapters are included that review what is known about the structure and stability of self-gravitating ''incompressible'' (uniform-density) fluid systems because: (a) in this special case, the set of governing relations is often amenable to a closed-form, analytic solution; and (b) most modern computationally assisted studies of compressible fluid systems — both in terms of their design and in the manner in which results have been interpreted — have been heavily influenced by these, more classical, studies of incompressible fluid systems. | |||
As the layout of the following ''tiled menu'' reflects, this review is broken into three major topical areas based primarily on ''geometrical'' considerations: | |||
<ul> | |||
<li>Studies of configurations that are — at least initially — spherically symmetric.</li> | |||
<li>Two-dimensional configurations — such as rotationally flattened spheroidal-like or toroidal-like structures; or infinitesimally thin, but nonaxisymmetric, disk-like structures.</li> | |||
<li>Configurations that require a full three-dimensional treatment — most notably, "spinning" ellipsoidal-like structures; or binary systems.</li> | |||
</ul> | |||
This is very much a living review. The chosen theme encompasses an enormous field of research that, because of its relevance to the astrophysics community, over time is continuing to expand at a healthy pace. As a consequence the review is incomplete now, and it will always be incomplete, so please bear with me. On any given day/week/month I will turn my attention to a topic that seems particularly interesting to me and I will begin writing a new chapter or I will edit/expand the contents of an existing tiled_menu chapter. This necessarily means that all chapters are incomplete while, in practice, some are much more polished than others. Hopefully steady ''forward'' progress is being made and the review will indeed be viewed as providing a service to the community. | |||
</font> | |||
==Context== | ==Context== | ||
{| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: | {| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | ||
|- | |||
! style="height: 150px; width: 150px; background-color:#9390DB;"|[[User:Tohline/VE#Global_Energy_Considerations|Global Energy<br />Considerations]] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color: black;" | |||
<!-- |+ style="text-align:left; height:40px;" | <font size="+2">'''CONTEXT'''</font> --> | <!-- |+ style="text-align:left; height:40px;" | <font size="+2">'''CONTEXT'''</font> --> | ||
|- | |- | ||
| Line 17: | Line 36: | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/PGE/Euler#Euler_Equation|Euler]] | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/PGE/Euler#Euler_Equation|Euler]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |1<sup>st</sup> Law of<br />Thermodynamics | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/PGE/FirstLawOfThermodynamics|1<sup>st</sup> Law of<br />Thermodynamics]] | ||
| | | | ||
! style="height: 150px; width: 150px;"|Poisson | ! style="height: 150px; width: 150px;"|[[User:Tohline/SR/PoissonOrigin#Origin_of_the_Poisson_Equation|Poisson]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SR#Supplemental_Relations|<b>Equation<br />of State</b>]]<br />(EOS) | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SR#Supplemental_Relations|<b>Equation<br />of State</b>]]<br />(EOS) | ||
| | | | ||
! style="height: 150px; width: 150px; " |[[User:Tohline/SR/PressureCombinations#Total_Pressure|Total<br /> | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SR/IdealGas#Ideal_Gas_Equation_of_State|Ideal Gas]] | ||
| | |||
! style="height: 150px; width: 150px; " |[[User:Tohline/SR/PressureCombinations#Total_Pressure|Total Pressure]] <br /> <hr /><br />[[User:Tohline/Apps/SMS#Rotating.2C_Supermassive_Stars|Bond, Arnett, & Carr<br />(1984)]] | |||
|} | |} | ||
==Spherically Symmetric Configurations== | ==Spherically Symmetric Configurations== | ||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:navy;" | |||
|- | |||
! style="height: 50px; width: 800px; background-color:lightgrey;"|<font color="navy" size="+2">(Initially) Spherically Symmetric Configurations</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: | {| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | ||
|- | |||
! style="height: 150px; width: 150px;"|[[File:FreeNRGpressureRadiusIsothermal.png|150px|link=User:Tohline/SSC/Virial/PolytropesEmbeddedOutline#Virial_Equilibrium_of_Embedded_Polytropic_Spheres|Whitworth's (1981) Isothermal Free-Energy Surface]] | |||
| | |||
! style="height: 150px; width: 150px; border-left:2px dashed black;"|[[User:Tohline/SSC/Virial/FormFactors#PTtable|Structural<br />Form<br />Factors]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#9390DB; border-left:2px solid black;"|[[User:Tohline/SphericallySymmetricConfigurations/Virial#Free_Energy_Expression|Free-Energy<br />of<br />Spherical<br />Systems]] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:black;" | |||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:lightgreen;" |[[User:Tohline/SphericallySymmetricConfigurations/PGE#Spherically_Symmetric_Configurations_.28Part_I.29|One-Dimensional<br /> PGEs]] | ! style="height: 150px; width: 150px; background-color:lightgreen;border-right:2px; " |[[User:Tohline/SphericallySymmetricConfigurations/PGE#Spherically_Symmetric_Configurations_.28Part_I.29|One-Dimensional<br /> PGEs]] | ||
|} | |} | ||
===Equilibrium Structures=== | ===Equilibrium Structures=== | ||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: | |- | ||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">1D STRUCTURE</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | |||
|- | |||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[File:SSC_SynopsisImage1.png|150px|link=User:Tohline/SSC/Synopsis_StyleSheet#Structure|Spherical Structures Synopsis]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#9390DB;"|[[User:Tohline/VE#Scalar_Virial_Theorem|Scalar<br />Virial<br />Theorem]] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black;" | |||
<!-- |+ style="text-align:left; height:40px;" | <font size="+2">'''CONTEXT'''</font> --> | <!-- |+ style="text-align:left; height:40px;" | <font size="+2">'''CONTEXT'''</font> --> | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:lightgreen; border-right:2px solid black;" |[[User:Tohline/SphericallySymmetricConfigurations/ | ! style="height: 150px; width: 150px; background-color:lightgreen; border-right:2px solid black;" |[[User:Tohline/SphericallySymmetricConfigurations/IntroductorySummary#Applications|<b>Hydrostatic<br />Balance<br />Equation</b>]] | ||
| | | | ||
! style="height: 150px; width: 310px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_SShydrostaticBalance01 }}</div> | ! style="height: 150px; width: 310px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_SShydrostaticBalance01 }}</div> | ||
| Line 50: | Line 93: | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; " |[[User:Tohline/SSC/Structure/UniformDensity#Isolated_Uniform-Density_Sphere|<b>Uniform-Density<br />Sphere</b>]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Structure/IsothermalSphere#Isothermal_Sphere|<b>Isothermal<br />Sphere</b>]] | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Structure/IsothermalSphere#Isothermal_Sphere|<b>Isothermal<br />Sphere</b>]] | ||
| Line 56: | Line 104: | ||
! style="height: 150px; width: 310px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_SSLaneEmden02 }}</div> | ! style="height: 150px; width: 310px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_SSLaneEmden02 }}</div> | ||
| | | | ||
! style="height: 150px; width: 150px;" |[[User:Tohline/ | ! style="height: 150px; width: 150px;" |[[User:Tohline/SSC/Structure/IsothermalSphere#Our_Numerical_Integration|via<br />Direct<br />Numerical<br />Integration]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Structure/Polytropes#Polytropic_Spheres|<b>Isolated<br />Polytropes</b>]] | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Structure/Polytropes#Polytropic_Spheres|<b>Isolated<br />Polytropes</b>]] | ||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/Lane1870#Lane.27s_1870_Work|<b>Lane<br />(1870)</b>]] | |||
| | | | ||
! style="height: 150px; width: 310px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_SSLaneEmden01 }}</div> | ! style="height: 150px; width: 310px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_SSLaneEmden01 }}</div> | ||
| Line 67: | Line 117: | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/Polytropes#Known_Analytic_Solutions|Known<br />Analytic<br />Solutions]] | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/Polytropes#Known_Analytic_Solutions|Known<br />Analytic<br />Solutions]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/ | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/Polytropes#Straight_Numerical_Integration|via<br />Direct<br />Numerical<br />Integration]] | ||
| | | | ||
! style="height: 150px; width: 150px; " |[[User:Tohline/ | ! style="height: 150px; width: 150px; " |[[User:Tohline/SSC/Structure/Polytropes#HSCF_Technique|via<br />Self-Consistent<br />Field (SCF)<br />Technique]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |[[User:Tohline/SSC/Structure/WhiteDwarfs#White_Dwarfs|<b>Zero-Temperature<br />White Dwarf</b>]] | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |[[User:Tohline/SSC/Structure/WhiteDwarfs#White_Dwarfs|<b>Zero-Temperature<br />White Dwarf</b>]] | ||
| | | | ||
! style="height: 150px; width: 150px; background-color:#ffeeee;" |[[User:Tohline/SSC/Structure/WhiteDwarfs#Chandrasekhar_mass|Chandrasekhar<br />Limiting<br />Mass]] | ! style="height: 150px; width: 150px; background-color:#ffeeee;" |[[User:Tohline/SSC/Structure/WhiteDwarfs#Chandrasekhar_mass|Chandrasekhar<br />Limiting<br />Mass<br />(1935)]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | ||
|- | |||
! style="height: 150px; width: 150px; "|[[User:Tohline/SSC/Structure/Polytropes/VirialSummary|Virial Equilibrium<br />of<br />Pressure-Truncated<br />Polytropes]] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |<b>Pressure-Truncated<br />Configurations</b> | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |<b>Pressure-Truncated<br />Configurations</b> | ||
| | | | ||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/BonnorEbert#Pressure-Bounded_Isothermal_Sphere|Bonnor-Ebert<br />(Isothermal)<br />Spheres]] | ! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/BonnorEbert#Pressure-Bounded_Isothermal_Sphere|Bonnor-Ebert<br />(Isothermal)<br />Spheres<br />(1955 - 56)]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/PolytropesEmbedded#Embedded_Polytropic_Spheres|Polytropes]] | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/PolytropesEmbedded#Embedded_Polytropic_Spheres|Polytropes]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/ | ! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/SSC/Stability/InstabilityOnsetOverview#Marginally_Unstable_Pressure-Truncated_Gas_Clouds|Equilibrium<br />Sequence<br />Turning-Points<br /><br /><font color="green" size="+2">♥</font>]] | ||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black; " |[[File:MassVsRadiusCombined02.png|130px|link=User:Tohline/SSC/Stability/InstabilityOnsetOverview#Turning_Points_along_Sequences_of_Pressure-Truncated_Polytropes|Equilibrium sequences of Pressure-Truncated Polytropes]] | |||
| | | | ||
! style="height: 150px; width: 150px; " |[[ | ! style="height: 150px; width: 150px; " |[[User:Tohline/Appendix/Ramblings/Turning_Points#Turning_Points|Turning-Points<br />(Broader Context)]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | ||
|- | |||
! style="height: 150px; width: 150px; "|[[User:Tohline/SSC/Structure/BiPolytropes/FreeEnergy5_1#Free_Energy_of_BiPolytrope_with|Free Energy<br />of<br />Bipolytropes]]<br /><br />(n<sub>c</sub>, n<sub>e</sub>) = (5, 1) | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Structure/BiPolytropes#BiPolytropes|<b>Composite<br />Polytropes</b>]]<br />(Bipolytropes) | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Structure/BiPolytropes#BiPolytropes|<b>Composite<br />Polytropes</b>]]<br />(Bipolytropes) | ||
| | | | ||
! style="height: 150px; width: 150px | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/BiPolytropes/Analytic1.5_3#BiPolytrope_with__and_ne_.3D_3|Milne<br />(1930)]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/ | ! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/LimitingMasses#Sch.C3.B6nberg-Chandrasekhar_Mass|Schönberg-<br />Chandrasekhar<br />Mass<br />(1942)]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/BiPolytropes/ | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#BiPolytrope_with_nc_.3D_5_and_ne_.3D_1|Analytic]]<br /><br />(n<sub>c</sub>, n<sub>e</sub>) = (5, 1) | ||
| | | | ||
! style="height: 150px; width: 150px; " |[[User:Tohline/SSC/ | ! style="height: 150px; width: 150px; " |[[User:Tohline/SSC/Structure/BiPolytropes/Analytic1_5#BiPolytrope_with_nc_.3D_1_and_ne_.3D_5|Analytic]]<br /><br />(n<sub>c</sub>, n<sub>e</sub>) = (1, 5) | ||
|} | |} | ||
<p> </p> | |||
===Stability Analysis=== | ===Stability Analysis=== | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | ||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">1D STABILITY</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | |||
|- | |||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[File:SSC_SynopsisImage2.png|150px|link=User:Tohline/SSC/Synopsis_StyleSheet#Stability|Synopsis: Stability of Spherical Structures]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#9390DB;"|[[User:Tohline/SSC/VariationalPrinciple#Ledoux.27s_Variational_Principle|Variational<br />Principle]] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:lightgreen; border-right:2px solid black;" |<b>Radial<br />Pulsation<br />Equation</b> | ! style="height: 150px; width: 150px; background-color:lightgreen; border-right:2px solid black;" |<b>Radial<br />Pulsation<br />Equation</b> | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Perturbations#Spherically_Symmetric_Configurations_.28Stability_.E2.80.94_Part_II.29|Example<br />Derivations<br />&<br />Statement of<br />Eigenvalue<br />Problem]] | ||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/SSC/Perspective_Reconciliation#Reconciling_Eulerian_versus_Lagrangian_Perspectives|(poor attempt at)<br />Reconciliation]] | |||
| | | | ||
! style="height: 150px; width: 150px;" |[[User:Tohline/SSC/SoundWaves#Sound_Waves|Relationship<br />to<br />Sound Waves]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 230px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[File:ImageOfDerivations06GoodJeansBonnor.png|120px|thumb|center|Jeans (1928) or Bonnor (1957)]] | ! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[File:ImageOfDerivations06GoodJeansBonnor.png|120px|thumb|center|Jeans (1928) or Bonnor (1957)]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[File:ImageOfDerivations07GoodLedouxWalraven.png|120px|thumb|center|Ledoux & Walraven (1958)]] | ! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[File:ImageOfDerivations07GoodLedouxWalraven.png|120px|thumb|center|Ledoux & Walraven (1958)]] | ||
| | | | ||
! style="height: 150px; width: 150px | ! style="height: 150px; width: 150px; " |[[File:ImageOfDerivations08GoodRosseland.png|120px|thumb|center|Rosseland (1969)]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Uniform-Density<br />Configurations</b> | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Uniform-Density<br />Configurations</b> | ||
| | | | ||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black; " |[[User:Tohline/SSC/UniformDensity#The_Stability_of_Uniform-Density_Spheres|Sterne's<br />Analytic Sol'n<br />of<br />Eigenvalue<br />Problem]] | ! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black; " |[[User:Tohline/SSC/UniformDensity#The_Stability_of_Uniform-Density_Spheres|Sterne's<br />Analytic Sol'n<br />of<br />Eigenvalue<br />Problem<br />(1937)]] | ||
| | | | ||
! style="height: 150px; width: 150px; " |[[File:Sterne1937SolutionPlot1.png|150px| | ! style="height: 150px; width: 150px; " |[[File:Sterne1937SolutionPlot1.png|150px|link=User:Tohline/SSC/UniformDensity#Properties_of_Eigenfunction_Solutions|Sterne's (1937) Solution to the Eigenvalue Problem for Uniform-Density Spheres]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/ | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Stability/Isothermal|<b>Pressure-Truncated<br />Isothermal<br />Spheres</b>]] | ||
| | | | ||
! style="height: 150px; width: 620px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_RadialPulsation03 }}</div> | ! style="height: 150px; width: 620px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_RadialPulsation03 }}</div> | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/SSC/Stability/Isothermal# | ! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/SSC/Stability/Isothermal#Our_Numerical_Integration|via<br />Direct<br />Numerical<br />Integration]] | ||
| | | | ||
! style="height: 150px; width: 310px | ! style="height: 150px; width: 310px;" |[[File:TaffVanHorn1974Fundamental.gif|240px|link=User:Tohline/SSC/Stability/Isothermal#CompositeDisplay2|Fundamental-Mode Eigenvectors]] | ||
|} | |||
<br /> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 230px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black; " |[[User:Tohline/SSC/Stability/InstabilityOnsetOverview#Yabushita.27s_Insight_Regarding_Stability|Yabushita's<br />Analytic Sol'n<br />for<br />Marginally Unstable<br />Configurations<br />(1974)]] | |||
| | | | ||
! style="height: 150px; width: | ! style="height: 150px; width: 310px;"|<table border="0" cellpadding="2" align="center"> | ||
<tr> | |||
<td align="center"> | |||
<math>~\sigma_c^2 = 0 \, , ~~~~\gamma_\mathrm{g} = 1</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
and | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~x = 1 - \biggl( \frac{1}{\xi e^{-\psi}}\biggr) \frac{d\psi}{d\xi} </math> | |||
</td> | |||
</tr> | |||
</table> | |||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/ | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/Stability/Polytropes#Radial_Oscillations_of_Polytropic_Spheres|<b>Polytropes</b>]] | ||
| | | | ||
! style="height: 150px; width: 620px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_RadialPulsation02 }}</div> | ! style="height: 150px; width: 620px; text-align:center; border-right:2px dashed black;" |<div align="center">{{ User:Tohline/Math/EQ_RadialPulsation02 }}</div> | ||
| Line 153: | Line 250: | ||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/SSC/Stability/n3PolytropeLAWE#Radial_Oscillations_of_n_.3D_3_Polytropic_Spheres|Isolated<br />n = 3<br />Polytrope]] | ! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/SSC/Stability/n3PolytropeLAWE#Radial_Oscillations_of_n_.3D_3_Polytropic_Spheres|Isolated<br />n = 3<br />Polytrope]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[File:Schwarzschild1941movie.gif|140px|Schwarzschild's Modal Analysis]] | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[File:Schwarzschild1941movie.gif|140px|link=User:Tohline/SSC/Stability/n3PolytropeLAWE#SchwarzschildMovie|Schwarzschild's Modal Analysis]] | ||
| | | | ||
! style="height: 150px; width: 150px | ! style="height: 150px; width: 150px;" |[[User:Tohline/SSC/Stability/n5PolytropeLAWE#Radial_Oscillations_of_n_.3D_5_Polytropic_Spheres|Pressure-Truncated<br />n = 5<br />Configurations]] | ||
|} | |||
<br /> | |||
{| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | |||
|- | |||
! style="height: 150px; width: 150px;"|[[User:Tohline/Appendix/Ramblings/Nonlinar_Oscillation#Radial_Oscillations_in_Pressure-Truncated_n_.3D_5_Polytropes|''Exact''<br />Demonstration<br />of<br />B-KB74<br />Conjecture]] | |||
| | | | ||
! style="height: 150px; width: 150px; background-color:#ffeeee;" |[[User:Tohline/SSC/Stability/InstabilityOnsetOverview#Analyses_of_Radial_Oscillations|Our | ! style="height: 150px; width: 150px; border-left:2px dashed black;"|[[User:Tohline/SSC/VariationalPrinciple#Directly_to_n_.3D_5_Polytropic_Configurations|''Exact''<br />Demonstration<br />of<br />Variational<br />Principle]] | ||
| | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-left:2px solid black;"|Pressure-Truncated<br />n = 5<br />Polytropes | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 230px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black; " |[[User:Tohline/SSC/Stability/InstabilityOnsetOverview#Analyses_of_Radial_Oscillations|Our Analytic Sol'n<br />for<br />Marginally Unstable<br />Configurations<br />(2017)<br /><font color="green" size="+2">♥</font>]] | |||
| | |||
! style="height: 150px; width: 465px;"|<table border="0" cellpadding="2" align="center"> | |||
<tr> | |||
<td align="center"> | |||
<math>~\sigma_c^2 = 0 \, , ~~~~\gamma_\mathrm{g} = (n+1)/n</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
and | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~x = \frac{3(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{1}{\xi \theta^{n}}\biggr) \frac{d\theta}{d\xi}\biggr] </math> | |||
</td> | |||
</tr> | |||
</table> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>BiPolytropes</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black; " |[[User:Tohline/SSC/Stability/MurphyFiedler85|Murphy & Fiedler<br />(1985b)]]<br /><br />(n<sub>c</sub>, n<sub>e</sub>) = (1,5) | |||
| | |||
! style="height: 150px; width: 150px; " |[[User:Tohline/SSC/Stability/BiPolytropes#Marginally_Unstable_Bipolytropes|Our<br />Broader<br />Analysis]] | |||
|} | |} | ||
<p> </p> | <p> </p> | ||
===Nonlinear Dynamical Evolution=== | ===Nonlinear Dynamical Evolution=== | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | ||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">1D DYNAMICS</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99;;" |[[User:Tohline/SSC/FreeFall#Free- | ! style="height: 150px; width: 150px; background-color:#ffff99;;" |[[User:Tohline/SSC/FreeFall#Free-Fall|<b>Free-Fall<br />Collapse</b>]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |<b>Collapse of<br />Isothermal<br />Spheres</b> | ! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black;" |[[User:Tohline/SSC/IsothermalCollapse#Collapse_of_Isothermal_Spheres|<b>Collapse of<br />Isothermal<br />Spheres</b>]] | ||
| | | | ||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[http://adsabs.harvard.edu/abs/1993ApJ...416..303F via<br/>Direct<br />Numerical<br />Integration] | ! style="height: 150px; width: 150px; border-right:2px dashed black;" |[http://adsabs.harvard.edu/abs/1993ApJ...416..303F via<br/>Direct<br />Numerical<br />Integration] | ||
| | | | ||
! style="height: 150px; width: 150px;" | | ! style="height: 150px; width: 150px;" |[[User:Tohline/SSC/IsothermalSimilaritySolution#Similarity_Solution|Similarity<br />Solution]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px border-color: black" | {| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | ||
|- | |- | ||
! style="height: 150px; width: 150px; background-color:#ffff99;" |[[User:Tohline/Apps/GoldreichWeber80#Homologously_Collapsing_Stellar_Cores|<b>Collapse of<br />an Isolated<br />n = 3<br />Polytrope</b>]] | ! style="height: 150px; width: 150px; background-color:#ffff99;" |[[User:Tohline/Apps/GoldreichWeber80#Homologously_Collapsing_Stellar_Cores|<b>Collapse of<br />an Isolated<br />n = 3<br />Polytrope</b>]] | ||
|} | |} | ||
<p> </p> | <p> </p> | ||
==Two-Dimensional Configurations (Axisymmetric)== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:navy;" | |||
|- | |||
! style="height: 50px; width: 800px; background-color:lightgrey;"|<font color="navy" size="+2">(Initially) Axisymmetric Configurations</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color: black;" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:black;" |[[User:Tohline/AxisymmetricConfigurations/Storyline|<font color="white">Storyline</font>]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color: black;" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:lightgreen;" |[[User:Tohline/AxisymmetricConfigurations/PGE#Axisymmetric_Configurations_.28Part_I.29|PGEs<br />for<br />Axisymmetric<br />Systems]] | |||
|} | |||
===Axisymmetric Equilibrium Structures=== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">2D STRUCTURE</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black;" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:lightgreen; border-right:2px solid black;" |[[User:Tohline/AxisymmetricConfigurations/Equilibria|<b>Constructing<br />Steady-State<br />Axisymmetric<br />Configurations</b>]] | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/2DStructure/AxisymmetricInstabilities|Axisymmetric<br />Instabilities<br />to Avoid]] | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/AxisymmetricConfigurations/SolutionStrategies#Simple_Rotation_Profile_and_Centrifugal_Potential|''Simple''<br />Rotation<br />Profiles]] | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/AxisymmetricConfigurations/HSCF|Hachisu Self-Consistent-Field<br />[HSCF]<br />Technique]] | |||
| | |||
! style="height: 150px; width: 150px; " |[[User:Tohline/AxisymmetricConfigurations/SolvingPE#Common_Theme:_Determining_the_Gravitational_Potential_for_Axisymmetric_Mass_Distributions|Solving the<br />Poisson Equation]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 230px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/2DStructure/UsingTC#Common_Theme:_Determining_the_Gravitational_Potential_for_Axisymmetric_Mass_Distributions|Using<br />Toroidal Coordinates<br />to Determine the<br />Gravitational<br /> Potential]] | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[File:Apollonian_myway4.png|150px|link=User:Tohline/2DStructure/ToroidalCoordinateIntegrationLimits#Mapping_from_Cylindrical_to_Toroidal_Coordinates|Apollonian Circles]] | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/2DStructure/TCsimplification#Common_Theme:_Determining_the_Gravitational_Potential_for_Axisymmetric_Mass_Distributions|Attempt at<br />Simplification<br /><br /><font color="green" size="+2">♥</font>]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black;" |[[User:Tohline/Apps/WongAP#Common_Theme:_Determining_the_Gravitational_Potential_for_Axisymmetric_Mass_Distributions|Wong's<br />Analytic Potential<br />(1973)]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#D0FFFF;" |[[File:MovieWongN4.gif|130px|link=User:Tohline/Apps/DysonWongTori#The_Coulomb_Potential|n = 3 contribution to potential]] | |||
|} | |||
====Spheroidal & Spheroidal-Like==== | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |[[User:Tohline/Apps/MaclaurinSpheroids#Maclaurin_Spheroids_.28axisymmetric_structure.29|<b>Uniform-Density<br />(Maclaurin)<br />Spheroids</b>]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black;" |[[User:Tohline/Apps/MaclaurinSpheroids/GoogleBooks#Excerpts_from_A_Treatise_of_Fluxions|Maclaurin's<br />Original Text<br />&<br />Analysis<br />(1742)]] | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[File:Maclaurin01.gif|150px|center|link=User:Tohline/Apps/MaclaurinSpheroids/GoogleBooks#Prolate_Spheroid|Our Construction of Maclaurin's Figure 291Pt2]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:maroon;" |[[User:Tohline/Apps/MaclaurinSpheroidSequence|<font color="white">Maclaurin<br />Spheroid<br />Sequence</font>]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Rotationally<br />Flattened<br />Isothermal<br />Configurations</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black;" |[[User:Tohline/Apps/HayashiNaritaMiyama82#Rotationally_Flattened_Isothermal_Structures|Hayashi, Narita<br /> & Miyama's<br />Analytic Sol'n<br />(1982)]] | |||
| | |||
! style="height: 150px; width: 150px;" |[[User:Tohline/Apps/ReviewStahler83|Review of<br /> Stahler's (1983)<br />Technique]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Rotationally<br />Flattened<br />Polytropes</b> | |||
| | |||
! style="height: 150px; width: 150px;" |[[User:Tohline/Apps/RotatingPolytropes|Example<br />Equilibria]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Rotationally<br />Flattened<br />White Dwarfs</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black;" |[[User:Tohline/Apps/OstrikerBodenheimerLyndenBell66|Ostriker<br />Bodenheimer<br />& Lynden-Bell<br />(1966)]] | |||
| | |||
! style="height: 150px; width: 150px;" |[[User:Tohline/Apps/RotatingWhiteDwarfs|Example<br />Equilibria]] | |||
|} | |||
====Toroidal & Toroidal-Like==== | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px;" | |||
|- | |||
! style="height: 25px;" |Definition: [http://www.mathematicsdictionary.com/english/vmd/full/t/torusanchorring.htm anchor ring] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Massless<br />Polytropic<br />Configurations</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black;" |[[User:Tohline/Apps/PapaloizouPringleTori#Massless_Polytropic_Tori|Papaloizou-Pringle<br />Tori<br />(1984)]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#D0FFFF;" |[[File:TorusMovie1.gif|150px|link=User:Tohline/Apps/PapaloizouPringleTori#Boundary_Conditions|Pivoting PP Torus]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Self-Gravitating<br />Incompressible<br />Configurations</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dashed black;" |[[User:Tohline/Apps/DysonPotential|Dyson<br />(1893)]] | |||
| | |||
! style="height: 150px; width: 150px; " |[[User:Tohline/Apps/DWT#Common_Theme:_Determining_the_Gravitational_Potential_for_Axisymmetric_Mass_Distributions|Dyson-Wong<br />Tori]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Self-Gravitating<br />Compressible<br />Configurations</b> | |||
| | |||
! style="height: 150px; width: 150px;" |[[User:Tohline/Apps/Ostriker64|Ostriker<br />(1964)]] | |||
|} | |||
<p> </p> | |||
===Stability Analysis=== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">2D STABILITY</font> | |||
|} | |||
<p> </p> | |||
====Sheroidal & Spheroidal-Like==== | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Linear<br />Analysis<br /> of<br />Bar-Mode<br />Instability</b> | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dashed black;" |[[User:Tohline/Apps/RotatingPolytropes/BarmodeIncompressible|Bifurcation<br />from<br />Maclaurin<br />Sequence]] | |||
| | |||
! style="height: 150px; width: 150px;" |[[User:Tohline/Apps/RotatingPolytropes/BarmodeEigenvector|Traditional<br />Analyses]] | |||
|} | |||
<p> </p> | |||
* [https://ui.adsabs.harvard.edu/abs/1949ApJ...109..149C/abstract T. G. Cowling & R. A. Newing (1949)], ApJ, 109, 149: ''The Oscillations of a Rotating Star'' | |||
* [https://ui.adsabs.harvard.edu/abs/1965ApJ...141..210C/abstract M. J. Clement (1965)], ApJ, 141, 210: ''The Radial and Non-Radial Oscillations of Slowly Rotating Gaseous Masses'' | |||
* [https://ui.adsabs.harvard.edu/abs/1963ApJ...137..777R/abstract P. H. Roberts & K. Stewartson (1963)], ApJ, 137, 777: ''On the Stability of a Maclaurin spheroid of small viscosity'' | |||
* [https://ui.adsabs.harvard.edu/abs/1967ApJ...148..825R/abstract C. E. Rosenkilde (1967)], ApJ, 148, 825: ''The tensor virial-theorem including viscous stress and the oscillations of a Maclaurin spheroid'' | |||
* [https://ui.adsabs.harvard.edu/abs/1968ApJ...152..267C/abstract S. Chandrasekhar & N. R. Lebovitz (1968)], ApJ, 152, 267: ''The Pulsations and the Dynamical Stability of Gaseous Masses in Uniform Rotation'' | |||
* [https://ui.adsabs.harvard.edu/abs/1977ApJ...213..497H/abstract C. Hunter (1977)], ApJ, 213, 497: ''On Secular Stability, Secular Instability, and Points of Bifurcation of Rotating Gaseous Masses'' | |||
* [https://ui.adsabs.harvard.edu/abs/1985ApJ...294..474I/abstract J. N. Imamura, J. L. Friedman & R. H. Durisen (1985)], ApJ, 294, 474: ''Secular stability limits for rotating polytropic stars'' | |||
<table border="0" align="center" width="100%" cellpadding="1"><tr> | |||
<td align="center" width="5%"> </td><td align="left"> | |||
<font color="green">The equilibrium models are calculated using the polytrope version (Bodenheimer & Ostriker 1973) of the Ostriker and Mark (1968) self-consistent field (SCF) code … the equilibrium models rotate on cylinders and are completely specified by <math>~n</math>, the total angular momentum, and the specific angular momentum distribution <math>~j(m_\varpi)</math>. Here <math>~m_\varpi</math> is the mass contained within a cylinder of radius <math>~\varpi</math> centered on the rotation axis. The angular momentum distribution is prescribed in several ways: (1) imposing strict uniform rotation; (2) using the same <math>~j(m_\varpi)</math> as that of a uniformly rotating spherical polybrope of index <math>~n^'</math> (see Bodenheimer and Ostriker 1973); and (3) using <math>~j(m_\varpi) \propto m_\varpi</math>, which we refer to as <math>~n^' = L</math>, <math>~L</math> for "linear."</font> | |||
</td></tr></table> | |||
* [https://ui.adsabs.harvard.edu/abs/1990ApJ...355..226I/abstract J. R. Ipser & L. Lindblom (1990)], ApJ, 355, 226: ''The Oscillations of Rapidly Rotating Newtonian Stellar Models'' | |||
* [https://ui.adsabs.harvard.edu/abs/1991ApJ...373..213I/abstract J. R. Ipser & L. Lindblom (1991)], ApJ, 373, 213: ''The Oscillations of Rapidly Rotating Newtonian Stellar Models. II. Dissipative Effects'' | |||
* [https://ui.adsabs.harvard.edu/abs/2000ApJ...528..946I/abstract J. N. Imamura, J. L. Friedman & R. H. Durisen (2000)], ApJ, 528, 946: ''Nonaxisymmetric Dynamic Instabilities of Rotating Polytropes. II. Torques, Bars, and Mode Saturation with Applications to Protostars and Fizzlers'' | |||
* [https://ui.adsabs.harvard.edu/abs/2003MNRAS.343..619S/abstract M. Shibata, S. Karino, & Y. Eriguchi (2003)], MNRAS, 343, 619 - 626: ''Dynamical bar-mode instability of differentially rotating stars: effects of equations of state and velocity profiles'' | |||
* [https://ui.adsabs.harvard.edu/abs/2019ApJ...877....9H/abstract G. P. Horedt (2019)], ApJ, 877, 9: ''On the Instability of Polytropic Maclaurin and Roche ellipsoids'' | |||
<p> </p> | |||
====Toroidal & Toroidal-Like==== | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black;" | |||
<!-- |+ style="text-align:left; height:40px;" | <font size="+2">'''CONTEXT'''</font> --> | |||
|- | |||
! style="height: 150px; width: 150px; background-color:lightgreen; " |[[User:Tohline/Apps/PapaloizouPringle84#Nonaxisymmetric_Instability_in_Papaloizou-Pringle_Tori|<b>Defining the<br />Eigenvalue Problem</b>]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>(Massless)<br />Papaloizou-Pringle<br />Tori</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black;" |[[User:Tohline/Apps/ImamuraHadleyCollaboration#Characteristics_of_Unstable_Eigenvectors_in_Self-Gravitating_Tori|Analytic Analysis<br />by<br />Blaes<br />(1985)]] | |||
| | |||
! style="height: 150px; width: 150px; " |[[File:N1.5j2_Combinedsmall.png|450px|center|link=User:Tohline/Apps/ImamuraHadleyCollaboration#Plots_of_a_Few_Example_Eigenvectors|j2 Eigenfunction from Blaes85|]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Self-Gravitating<br />Polytropic<br />Rings</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right:2px dotted black;" |[https://ui.adsabs.harvard.edu/abs/1990ApJ...361..394T/abstract Numerical Analysis<br />by<br />Tohline & Hachisu<br />(1990)] | |||
| | |||
! style="height: 150px; width: 150px; background-color:black; border-right:2px dashed black; " |[[File:Minitorus.animated.gif|150px|center|PP torus instability]] | |||
| | |||
! style="height: 150px; width: 150px;" |[[User:Tohline/Apps/WoodwardTohlineHachisu94#Online_Movies|Thick<br />Accretion<br />Disks]]<br />(WTH94) | |||
|} | |||
<p> </p> | |||
===Nonlinear Dynamical Evolution=== | |||
====Sheroidal & Spheroidal-Like==== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">2D DYNAMICS</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99;;" |[[User:Tohline/Aps/MaclaurinSpheroidFreeFall|<b>Free-Fall<br />Collapse<br />of an<br />Homogeneous<br />Spheroid</b>]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right:2px solid black; " |<b>Nonlinear<br />Development of<br />Bar-Mode</b> | |||
| | |||
! style="height: 150px; width: 150px; border-right:2px dotted black;" |[[User:Tohline/Apps/RotatingPolytropes/BarmodeLinearTimeDependent|Initially<br />Axisymmetric<br /> & Differentially<br />Rotating<br />Polytropes]] | |||
| | |||
! style="height: 150px; width: 150px; background-color: black;" |[[File:Dissertation.fig3.jpg|112px|link=User:Tohline/Apps/RotatingPolytropes/BarmodeLinearTimeDependent|Cazes Model A Simulation]] | |||
|} | |||
<p> </p> | |||
==Two-Dimensional Configurations (Nonaxisymmetric Disks)== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:navy;" | |||
|- | |||
! style="height: 50px; width: 800px; background-color:lightgrey;"|<font color="navy" size="+2">Infinitesimally Thin, Nonaxisymmetric Disks</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">2D STRUCTURE</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black;" | |||
<!-- |+ style="text-align:left; height:40px;" | <font size="+2">'''CONTEXT'''</font> --> | |||
|- | |||
! style="height: 150px; width: 150px; background-color:lightgreen; " |[[User:Tohline/Apps/Korycansky_Papaloizou_1996#Korycansky_and_Papaloizou_.281996.29|<b>Constructing<br />Infinitesimally Thin<br />Nonaxisymmetric<br />Disks</b>]] | |||
|} | |||
<p> </p> | |||
==Three-Dimensional Configurations== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:navy;" | |||
|- | |||
! style="height: 50px; width: 800px; background-color:lightgrey;"|<font color="navy" size="+2">(Initially) Three-Dimensional Configurations</font> | |||
|} | |||
<p> </p> | |||
===Equilibrium Structures=== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">3D STRUCTURE</font> | |||
|} | |||
<!-- | |||
<table border="0" cellpadding="3" align="center" width="60%"> | |||
<tr><td align="left"> | |||
<font color="darkgreen">"One interesting aspect of our models … is the pulsation characteristic of the final central triaxial figure … our interest in the pulsations stems from a general concern about the equilibrium structure of self-gravitating, triaxial objects. In the past, attempts to construct hydrostatic models of any equilibrium, triaxial structure having both a high <math>~T/|W|</math> value and a compressible equation of state have met with very limited success … they have been thwarted by a lack of understanding of how to represent complex internal motions in a physically realistic way… We suggest … that a ''natural'' attribute of [such] configurations may be pulsation and that, as a result, a search for simple circulation hydrostatic analogs of such systems may prove to a fruitless endeavor.</font> | |||
</td></tr> | |||
<tr><td align="right"> | |||
— Drawn from §IVa of [https://ui.adsabs.harvard.edu/abs/1988ApJ...334..449W/abstract Williams & Tohline (1988)], ApJ, 334, 449 | |||
</td></tr></table> | |||
--> | |||
<table border="0" cellpadding="3" align="center" width="60%"> | |||
<tr><td align="left"> | |||
Special numerical techniques must be developed <font color="darkgreen">"to build three-dimensional compressible equilibrium models with complicated flows."</font> To date … <font color="darkgreen">"techniques have only been developed to build compressible equilibrium models of nonaxisymmetric configurations for a few systems with simplified rotational profiles, e.g., rigidly rotating systems ([https://ui.adsabs.harvard.edu/abs/1984PASJ...36..239H/abstract Hachisu & Eriguchi 1984]; [https://ui.adsabs.harvard.edu/abs/1986ApJS...62..461H/abstract Hachisu 1986)], irrotational systems ([https://ui.adsabs.harvard.edu/abs/1998ApJS..118..563U/abstract Uryū & Eriguchi 1998]), and configurations that are stationary in the inertial frame ([https://ui.adsabs.harvard.edu/abs/1996MNRAS.282..653U/abstract Uryū & Eriguchi 1996])."</font> | |||
</td></tr> | |||
<tr><td align="right"> | |||
— Drawn from §1 of [https://ui.adsabs.harvard.edu/abs/2006ApJ...639..549O/abstract Ou (2006)], ApJ, 639, 549 | |||
</td></tr></table> | |||
====Ellipsoidal & Ellipsoidal-Like==== | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black;" | |||
<!-- |+ style="text-align:left; height:40px;" | <font size="+2">'''CONTEXT'''</font> --> | |||
|- | |||
! style="height: 150px; width: 150px; background-color:lightgreen; " |[https://ui.adsabs.harvard.edu/abs/2006ApJ...639..549O/abstract <b>Constructing<br />Ellipsoidal<br /> & Ellipsoidal-Like<br />Configurations</b>] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#9390DB;"|[[User:Tohline/VE/RiemannEllipsoids|Steady-State<br />2<sup>nd</sup>-Order<br />Tensor Virial<br />Equations]] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right: 2px solid black; " |<b>Uniform-Density<br />Incompressible<br />Ellipsoids</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:white; border-right: 2px dashed black; " |[[User:Tohline/ThreeDimensionalConfigurations/HomogeneousEllipsoids|<b>The<br />Gravitational<br />Potential</b>]]<p></p>(A<sub>i</sub> coefficients) | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right: 2px dashed black; " |[[User:Tohline/ThreeDimensionalConfigurations/JacobiEllipsoids#Jacobi_Ellipsoids|<b>Jacobi<br />Ellipsoids</b>]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right: 2px dashed black;" |[[User:Tohline/ThreeDimensionalConfigurations/RiemannStype#Riemann_S-type_Ellipsoids|<b>Riemann<br />S-Type<br />Ellipsoids</b>]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#ffeeee; border-right: 2px dashed black;" |[[User:Tohline/ThreeDimensionalConfigurations/RiemannTypeI|<b>Type I<br />Riemann<br />Ellipsoids</b>]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:white;" |[[User:Tohline/ThreeDimensionalConfigurations/MeetsCOLLADAandOculusRiftS|<b>Riemann<br />meets<br />COLLADA<br />&<br /> Oculus Rift S</b>]] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 550px; border-style: solid; border-width: 3px; border-color: black;" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:white; border-right: 2px dashed black; " |[https://www.aimspress.com/article/10.3934/math.2019.2.215/fulltext.html <b>A Gauge Theory<br />of<br />Riemann Ellipsoids</b>] | |||
| | |||
! style="height: 150px; width: 150px; background-color:white; " |[https://ui.adsabs.harvard.edu/abs/2020PhRvL.124e2501S/abstract <b>Nuclear<br />Wobbling Motion</b>] | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right: 2px solid black; " |<b>Compressible<br />Analogs of<br />Riemann Ellipsoids</b> | |||
| | |||
! style="height: 150px; width: 150px; background-color:pink; border-right: 2px dashed black;" |[[User:Tohline/ThreeDimensionalConfigurations/FerrersPotential|<b>Ferrers<br />Potential<br />(1877)</b>]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:white;" |[[User:Tohline/ThreeDimensionalConfigurations/CAREs|<b>Thoughts<br />&<br />Challenges</b>]] | |||
|} | |||
<p> </p> | |||
* [https://ui.adsabs.harvard.edu/abs/1985Ap.....23..654K/abstract B. P. Kondrat'ev (1985)], Astrophysics, 23, 654: ''Irrotational and zero angular momentum ellipsoids in the Dirichlet problem'' | |||
* [https://ui.adsabs.harvard.edu/abs/1993ApJS...88..205L/abstract D. Lai, F. A. Rasio & S. L. Shapiro (1993)], ApJS, 88, 205: ''Ellipsoidal Figures of Equilibrium: Compressible models'' | |||
====Binary Systems==== | |||
* [https://ui.adsabs.harvard.edu/abs/1933MNRAS..93..539C/abstract S. Chandrasekhar (1933)], MNRAS, 93, 539: ''The equilibrium of distorted polytropes. IV. the rotational and the tidal distortions as functions of the density distribution'' | |||
* [https://ui.adsabs.harvard.edu/abs/1963ApJ...138.1182C/abstract S. Chandrasekhar (1963)], ApJ, 138, 1182: ''The Equilibrium and the Stability of the Roche Ellipsoids'' | |||
<table border="0" align="center" width="100%" cellpadding="1"><tr> | |||
<td align="center" width="5%"> </td><td align="left"> | |||
<font color="green">Roche's problem is concerned with the equilibrium and the stability of rotating homogeneous masses which are, further, distorted by the constant tidal action of an attendant rigid spherical mass.</font> | |||
</td></tr></table> | |||
<p> </p> | |||
===Stability Analysis=== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">3D STABILITY</font> | |||
|} | |||
====Ellipsoidal & Ellipsoidal-Like==== | |||
<p> </p> | |||
====Binary Systems==== | |||
* [https://ui.adsabs.harvard.edu/abs/1963ApJ...138.1182C/abstract S. Chandrasekhar (1963)], ApJ, 138, 1182: ''The Equilibrium and the Stability of the Roche Ellipsoids'' | |||
* [https://ui.adsabs.harvard.edu/abs/2019ApJ...877....9H/abstract G. P. Horedt (2019)], ApJ, 877, 9: ''On the Instability of Polytropic Maclaurin and Roche Ellipsoids'' | |||
===Nonlinear Evolution=== | |||
{| class="wikitable" width=100% style="margin-right: auto; margin-left: 0px; border-style: solid; border-width: 3px; border-color:darkgrey;" | |||
|- | |||
! style="height: 30px; width: 800px; background-color:lightgrey;"|<font color="white" size="+1">3D DYNAMICS</font> | |||
|} | |||
<p> </p> | |||
{| class="wikitable" style="float:right; margin-left: 150px; border-style: solid; border-width: 3px; border-color:black;" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#D0FFFF;"|[[File:JacobiMaclaurin2.gif|300px|link=User:Tohline/ThreeDimensionalConfigurations/EFE_Energies#Animation|Animation related to Fig. 3 from Christodoulou1995]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:#9390DB; border-left:2px solid black;"|[[User:Tohline/ThreeDimensionalConfigurations/EFE_Energies#Properties_of_Homogeneous_Ellipsoids_.282.29|Free-Energy<br />Evolution<br />from the Maclaurin<br />to the Jacobi<br />Sequence]] | |||
|} | |||
{| class="wikitable" style="margin-right: auto; margin-left: 75px; border-style: solid; border-width: 3px; border-color: black;" | |||
|- | |||
! style="height: 150px; width: 150px; background-color:#ffff99; border-right: 2px solid black; " |[[User:Tohline/ThreeDimensionalConfigurations/BinaryFission#Fission_Hypothesis_of_Binary_Star_Formation|<b>Fission<br />Hypothesis</b>]] | |||
| | |||
! style="height: 150px; width: 150px; background-color:white;" |[http://www.phys.lsu.edu/~tohline/fission.movies.html <b>"Fission"<br />Simulations<br />at LSU</b>] | |||
|} | |||
<p> </p> | |||
====Secular==== | |||
* [https://ui.adsabs.harvard.edu/abs/1971ApJ...170..143F/abstract M. Fujimoto (1971)], ApJ, 170, 143: ''Nonlinear Motions of Rotating Gaseous Ellipsoids'' | |||
* [https://ui.adsabs.harvard.edu/abs/1973ApJ...181..513P/abstract W. H. Press & S. A. Teukolsky (1973)], ApJ, 181, 513: ''On the Evolution of the Secularly Unstable, Viscous Maclaurin Spheroids'' | |||
* [https://ui.adsabs.harvard.edu/abs/1977ApJ...213..193D/abstract S. L. Detweiler & L. Lindblom (1977)], ApJ, 213, 193: ''On the evolution of the homogeneous ellipsoidal figures.'' | |||
====Dynamical==== | |||
=See Also= | =See Also= | ||
Latest revision as of 17:27, 28 May 2021
Tiled Menu

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
[Preamble by Joel E. Tohline — Circa 2020] In an effort to serve ongoing research activities of the astrophysics community, this media-wiki-based hypertext book (H_Book) reviews what is presently understood about the structure, stability, and dynamical evolution of (Newtonian) self-gravitating fluids. In the context of this review, the "dynamical evolution" categorization generally will refer to studies that begin with an equilibrium configuration that has been identified — via a stability analysis — as being dynamically (or, perhaps, secularly) unstable then, using numerical hydrodynamic technique, follow the development to nonlinear amplitude of deformations that spontaneously develop as a result of the identified instability.
As is reflected in our choice of the overarching set of Principle Governing Equations, our focus is on studies of compressible fluid systems — those that obey a barotropic equation of state. But, in addition, chapters are included that review what is known about the structure and stability of self-gravitating incompressible (uniform-density) fluid systems because: (a) in this special case, the set of governing relations is often amenable to a closed-form, analytic solution; and (b) most modern computationally assisted studies of compressible fluid systems — both in terms of their design and in the manner in which results have been interpreted — have been heavily influenced by these, more classical, studies of incompressible fluid systems.
As the layout of the following tiled menu reflects, this review is broken into three major topical areas based primarily on geometrical considerations:
- Studies of configurations that are — at least initially — spherically symmetric.
- Two-dimensional configurations — such as rotationally flattened spheroidal-like or toroidal-like structures; or infinitesimally thin, but nonaxisymmetric, disk-like structures.
- Configurations that require a full three-dimensional treatment — most notably, "spinning" ellipsoidal-like structures; or binary systems.
This is very much a living review. The chosen theme encompasses an enormous field of research that, because of its relevance to the astrophysics community, over time is continuing to expand at a healthy pace. As a consequence the review is incomplete now, and it will always be incomplete, so please bear with me. On any given day/week/month I will turn my attention to a topic that seems particularly interesting to me and I will begin writing a new chapter or I will edit/expand the contents of an existing tiled_menu chapter. This necessarily means that all chapters are incomplete while, in practice, some are much more polished than others. Hopefully steady forward progress is being made and the review will indeed be viewed as providing a service to the community.
Context
| Global Energy Considerations |
|---|
| Principal Governing Equations (PGEs) |
Continuity | Euler | 1st Law of Thermodynamics |
Poisson |
|---|
| Equation of State (EOS) |
Ideal Gas | Total Pressure Bond, Arnett, & Carr (1984) |
|---|
Spherically Symmetric Configurations
| (Initially) Spherically Symmetric Configurations |
|---|

|
Structural Form Factors |
Free-Energy of Spherical Systems |
|---|
| One-Dimensional PGEs |
|---|
Equilibrium Structures
| 1D STRUCTURE |
|---|
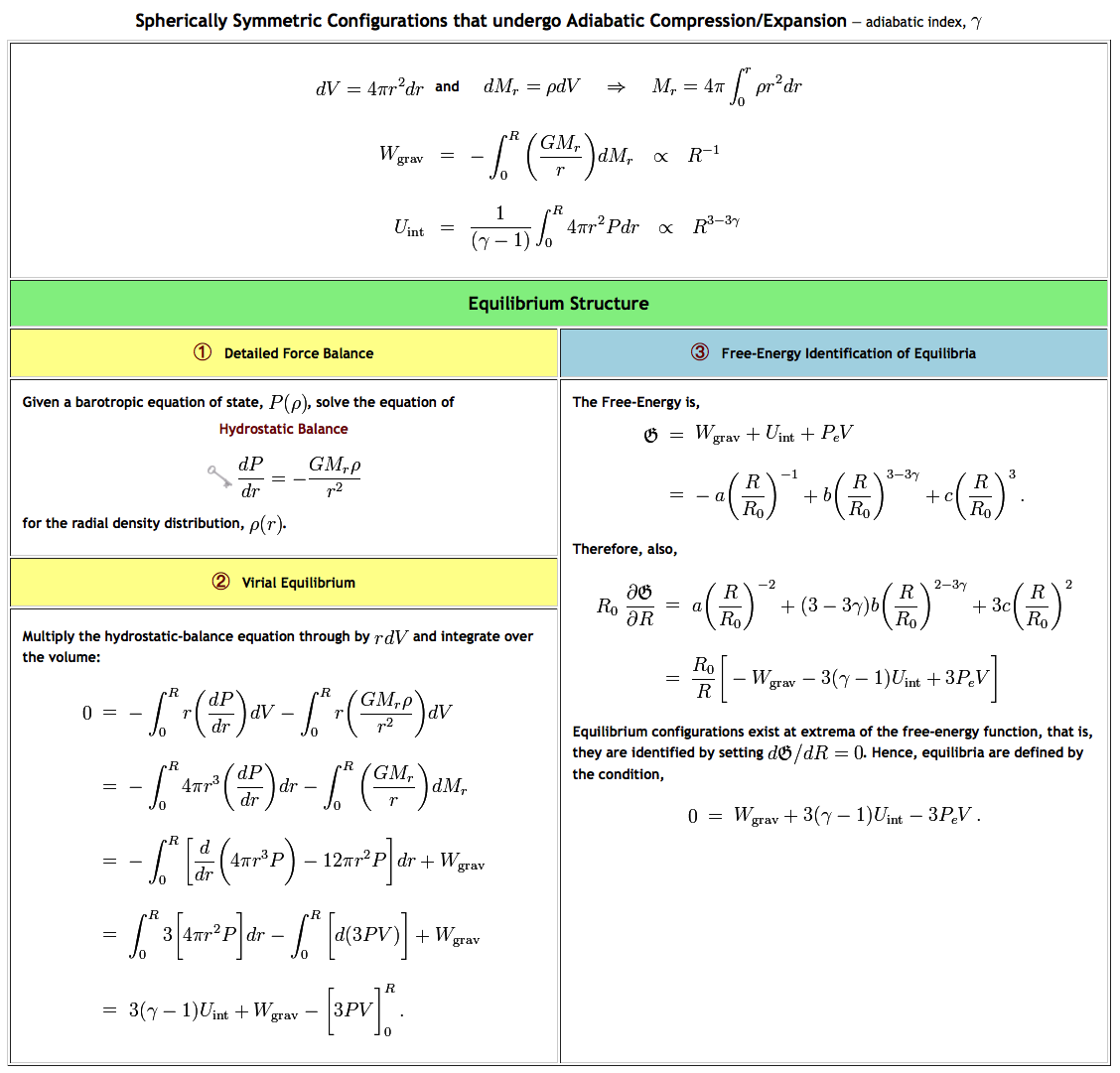
|
Scalar Virial Theorem |
|---|
| Hydrostatic Balance Equation |
Solution Strategies |
|---|
| Uniform-Density Sphere |
|---|
| Isothermal Sphere |
via Direct Numerical Integration |
|---|
| Isolated Polytropes |
Lane (1870) |
Known Analytic Solutions |
via Direct Numerical Integration |
via Self-Consistent Field (SCF) Technique |
|---|
| Zero-Temperature White Dwarf |
Chandrasekhar Limiting Mass (1935) |
|---|
| Virial Equilibrium of Pressure-Truncated Polytropes |
|---|
| Pressure-Truncated Configurations |
Bonnor-Ebert (Isothermal) Spheres (1955 - 56) |
Polytropes | Equilibrium Sequence Turning-Points ♥ |

|
Turning-Points (Broader Context) |
|---|
| Free Energy of Bipolytropes (nc, ne) = (5, 1) |
|---|
| Composite Polytropes (Bipolytropes) |
Milne (1930) |
Schönberg- Chandrasekhar Mass (1942) |
Analytic (nc, ne) = (5, 1) |
Analytic (nc, ne) = (1, 5) |
|---|
Stability Analysis
| 1D STABILITY |
|---|
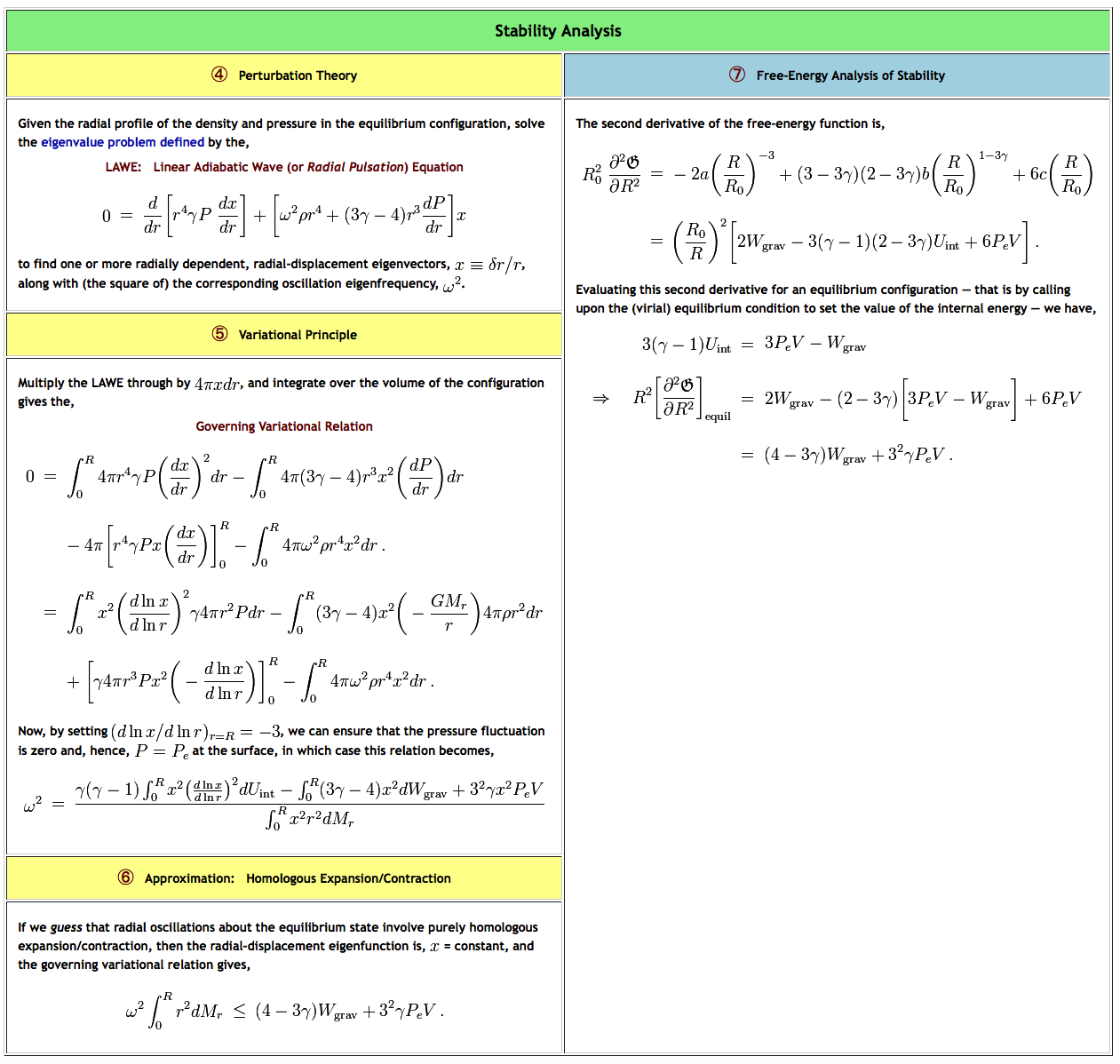
|
Variational Principle |
|---|
| Radial Pulsation Equation |
Example Derivations & Statement of Eigenvalue Problem |
(poor attempt at) Reconciliation |
Relationship to Sound Waves |
|---|
| Uniform-Density Configurations |
Sterne's Analytic Sol'n of Eigenvalue Problem (1937) |
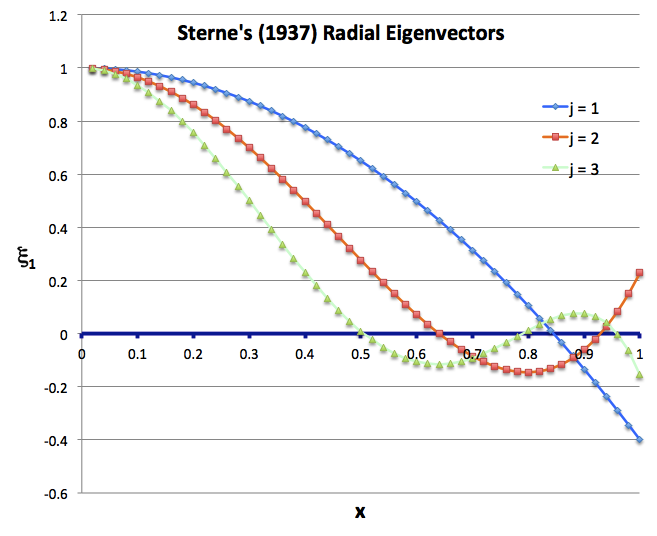
|
|---|
| Pressure-Truncated Isothermal Spheres |
|
via Direct Numerical Integration |
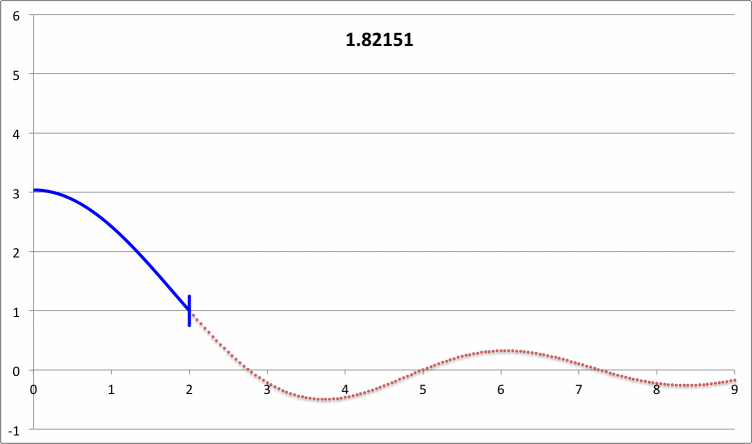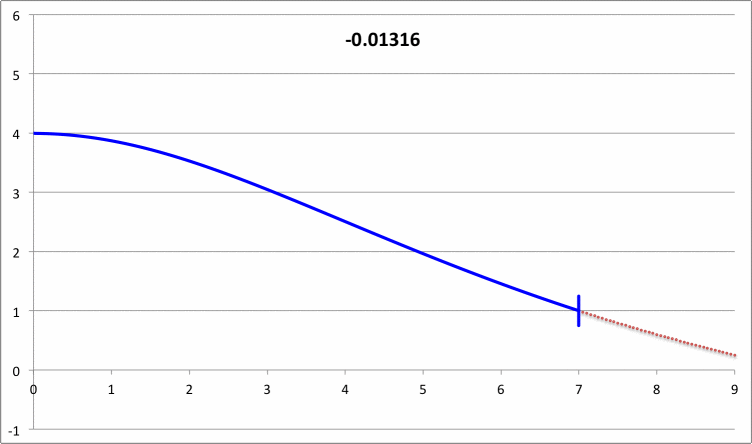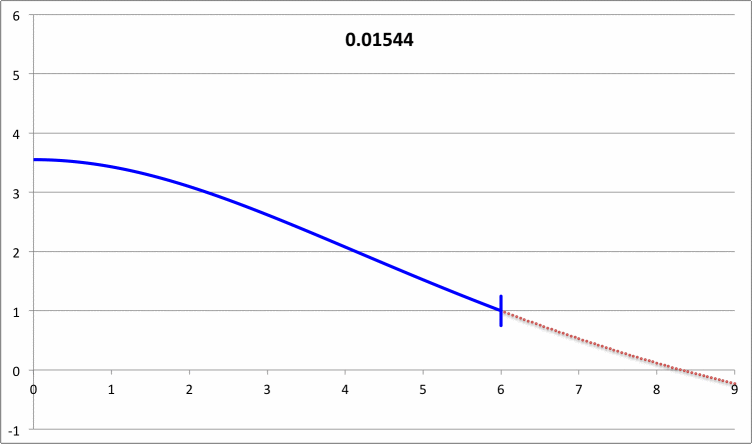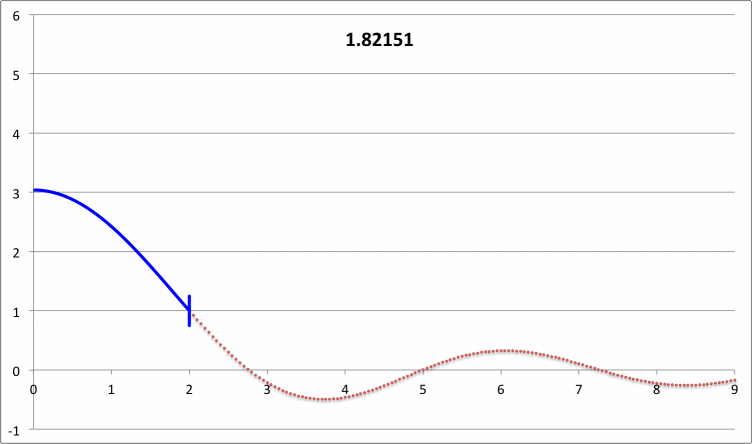
| |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Yabushita's Analytic Sol'n for Marginally Unstable Configurations (1974) |
|
|---|
| Polytropes |
|
Isolated n = 3 Polytrope |
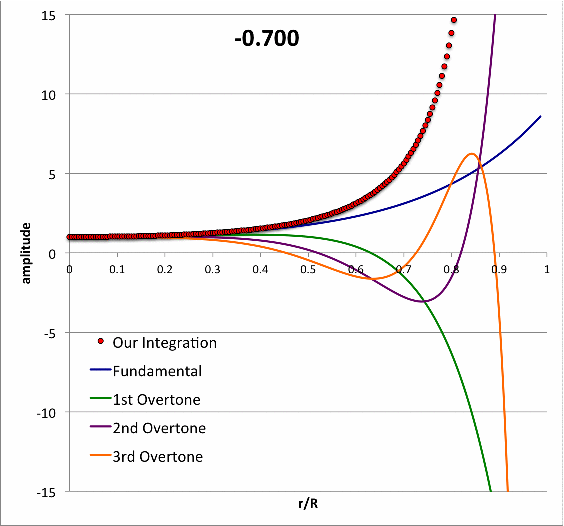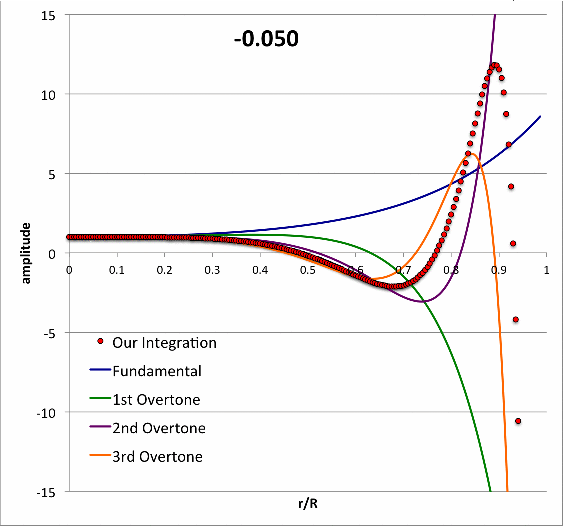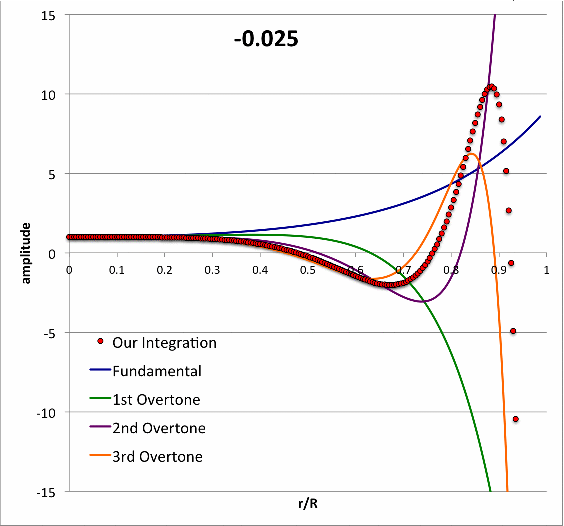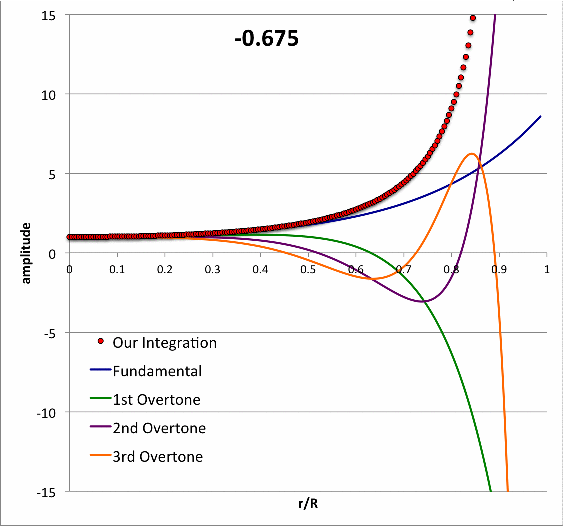
|
Pressure-Truncated n = 5 Configurations | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Exact Demonstration of B-KB74 Conjecture |
Exact Demonstration of Variational Principle |
Pressure-Truncated n = 5 Polytropes |
|---|
| Our Analytic Sol'n for Marginally Unstable Configurations (2017) ♥ |
|
|---|
| BiPolytropes | Murphy & Fiedler (1985b) (nc, ne) = (1,5) |
Our Broader Analysis |
|---|
Nonlinear Dynamical Evolution
| 1D DYNAMICS |
|---|
| Free-Fall Collapse |
|---|
| Collapse of Isothermal Spheres |
via Direct Numerical Integration |
Similarity Solution |
|---|
| Collapse of an Isolated n = 3 Polytrope |
|---|
Two-Dimensional Configurations (Axisymmetric)
| (Initially) Axisymmetric Configurations |
|---|
| Storyline |
|---|
| PGEs for Axisymmetric Systems |
|---|
Axisymmetric Equilibrium Structures
| 2D STRUCTURE |
|---|
| Constructing Steady-State Axisymmetric Configurations |
Axisymmetric Instabilities to Avoid |
Simple Rotation Profiles |
Hachisu Self-Consistent-Field [HSCF] Technique |
Solving the Poisson Equation |
|---|
| Using Toroidal Coordinates to Determine the Gravitational Potential |
|
Attempt at Simplification ♥ |
Wong's Analytic Potential (1973) |
|
|---|
Spheroidal & Spheroidal-Like
| Uniform-Density (Maclaurin) Spheroids |
Maclaurin's Original Text & Analysis (1742) |
Maclaurin Spheroid Sequence |
|---|
| Rotationally Flattened Isothermal Configurations |
Hayashi, Narita & Miyama's Analytic Sol'n (1982) |
Review of Stahler's (1983) Technique |
|---|
| Rotationally Flattened Polytropes |
Example Equilibria |
|---|
| Rotationally Flattened White Dwarfs |
Ostriker Bodenheimer & Lynden-Bell (1966) |
Example Equilibria |
|---|
Toroidal & Toroidal-Like
| Definition: anchor ring |
|---|
| Massless Polytropic Configurations |
Papaloizou-Pringle Tori (1984) |
|
|---|
| Self-Gravitating Incompressible Configurations |
Dyson (1893) |
Dyson-Wong Tori |
|---|
| Self-Gravitating Compressible Configurations |
Ostriker (1964) |
|---|
Stability Analysis
| 2D STABILITY |
|---|
Sheroidal & Spheroidal-Like
| Linear Analysis of Bar-Mode Instability |
Bifurcation from Maclaurin Sequence |
Traditional Analyses |
|---|
- T. G. Cowling & R. A. Newing (1949), ApJ, 109, 149: The Oscillations of a Rotating Star
- M. J. Clement (1965), ApJ, 141, 210: The Radial and Non-Radial Oscillations of Slowly Rotating Gaseous Masses
- P. H. Roberts & K. Stewartson (1963), ApJ, 137, 777: On the Stability of a Maclaurin spheroid of small viscosity
- C. E. Rosenkilde (1967), ApJ, 148, 825: The tensor virial-theorem including viscous stress and the oscillations of a Maclaurin spheroid
- S. Chandrasekhar & N. R. Lebovitz (1968), ApJ, 152, 267: The Pulsations and the Dynamical Stability of Gaseous Masses in Uniform Rotation
- C. Hunter (1977), ApJ, 213, 497: On Secular Stability, Secular Instability, and Points of Bifurcation of Rotating Gaseous Masses
- J. N. Imamura, J. L. Friedman & R. H. Durisen (1985), ApJ, 294, 474: Secular stability limits for rotating polytropic stars
|
The equilibrium models are calculated using the polytrope version (Bodenheimer & Ostriker 1973) of the Ostriker and Mark (1968) self-consistent field (SCF) code … the equilibrium models rotate on cylinders and are completely specified by <math>~n</math>, the total angular momentum, and the specific angular momentum distribution <math>~j(m_\varpi)</math>. Here <math>~m_\varpi</math> is the mass contained within a cylinder of radius <math>~\varpi</math> centered on the rotation axis. The angular momentum distribution is prescribed in several ways: (1) imposing strict uniform rotation; (2) using the same <math>~j(m_\varpi)</math> as that of a uniformly rotating spherical polybrope of index <math>~n^'</math> (see Bodenheimer and Ostriker 1973); and (3) using <math>~j(m_\varpi) \propto m_\varpi</math>, which we refer to as <math>~n^' = L</math>, <math>~L</math> for "linear." |
- J. R. Ipser & L. Lindblom (1990), ApJ, 355, 226: The Oscillations of Rapidly Rotating Newtonian Stellar Models
- J. R. Ipser & L. Lindblom (1991), ApJ, 373, 213: The Oscillations of Rapidly Rotating Newtonian Stellar Models. II. Dissipative Effects
- J. N. Imamura, J. L. Friedman & R. H. Durisen (2000), ApJ, 528, 946: Nonaxisymmetric Dynamic Instabilities of Rotating Polytropes. II. Torques, Bars, and Mode Saturation with Applications to Protostars and Fizzlers
- M. Shibata, S. Karino, & Y. Eriguchi (2003), MNRAS, 343, 619 - 626: Dynamical bar-mode instability of differentially rotating stars: effects of equations of state and velocity profiles
- G. P. Horedt (2019), ApJ, 877, 9: On the Instability of Polytropic Maclaurin and Roche ellipsoids
Toroidal & Toroidal-Like
| Defining the Eigenvalue Problem |
|---|
| (Massless) Papaloizou-Pringle Tori |
Analytic Analysis by Blaes (1985) |
|---|
| Self-Gravitating Polytropic Rings |
Numerical Analysis by Tohline & Hachisu (1990) |
Thick Accretion Disks (WTH94) |
|---|
Nonlinear Dynamical Evolution
Sheroidal & Spheroidal-Like
| 2D DYNAMICS |
|---|
| Free-Fall Collapse of an Homogeneous Spheroid |
|---|
| Nonlinear Development of Bar-Mode |
Initially Axisymmetric & Differentially Rotating Polytropes |
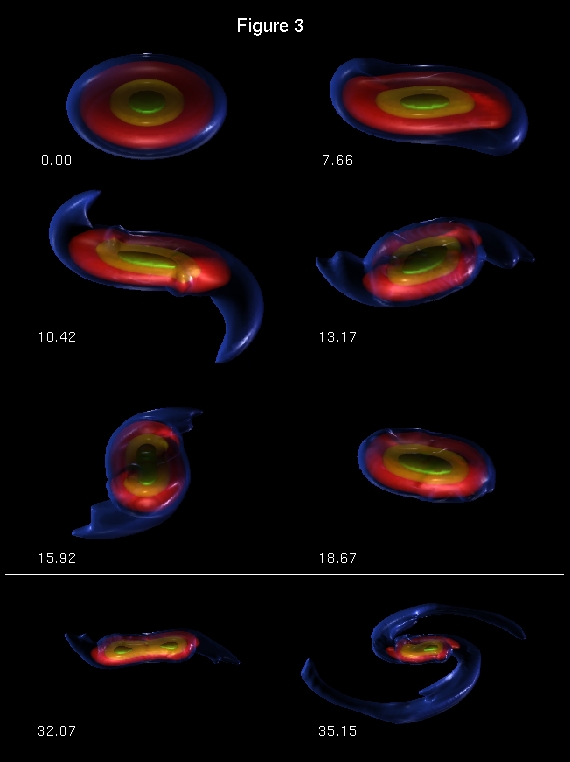
|
|---|
Two-Dimensional Configurations (Nonaxisymmetric Disks)
| Infinitesimally Thin, Nonaxisymmetric Disks |
|---|
| 2D STRUCTURE |
|---|
| Constructing Infinitesimally Thin Nonaxisymmetric Disks |
|---|
Three-Dimensional Configurations
| (Initially) Three-Dimensional Configurations |
|---|
Equilibrium Structures
| 3D STRUCTURE |
|---|
|
Special numerical techniques must be developed "to build three-dimensional compressible equilibrium models with complicated flows." To date … "techniques have only been developed to build compressible equilibrium models of nonaxisymmetric configurations for a few systems with simplified rotational profiles, e.g., rigidly rotating systems (Hachisu & Eriguchi 1984; Hachisu 1986), irrotational systems (Uryū & Eriguchi 1998), and configurations that are stationary in the inertial frame (Uryū & Eriguchi 1996)." |
|
— Drawn from §1 of Ou (2006), ApJ, 639, 549 |
Ellipsoidal & Ellipsoidal-Like
| Constructing Ellipsoidal & Ellipsoidal-Like Configurations |
|---|
| Steady-State 2nd-Order Tensor Virial Equations |
|---|
| Uniform-Density Incompressible Ellipsoids |
The Gravitational Potential(Ai coefficients) |
Jacobi Ellipsoids |
Riemann S-Type Ellipsoids |
Type I Riemann Ellipsoids |
Riemann meets COLLADA & Oculus Rift S |
|---|
| A Gauge Theory of Riemann Ellipsoids |
Nuclear Wobbling Motion |
|---|
| Compressible Analogs of Riemann Ellipsoids |
Ferrers Potential (1877) |
Thoughts & Challenges |
|---|
- B. P. Kondrat'ev (1985), Astrophysics, 23, 654: Irrotational and zero angular momentum ellipsoids in the Dirichlet problem
- D. Lai, F. A. Rasio & S. L. Shapiro (1993), ApJS, 88, 205: Ellipsoidal Figures of Equilibrium: Compressible models
Binary Systems
- S. Chandrasekhar (1933), MNRAS, 93, 539: The equilibrium of distorted polytropes. IV. the rotational and the tidal distortions as functions of the density distribution
- S. Chandrasekhar (1963), ApJ, 138, 1182: The Equilibrium and the Stability of the Roche Ellipsoids
|
Roche's problem is concerned with the equilibrium and the stability of rotating homogeneous masses which are, further, distorted by the constant tidal action of an attendant rigid spherical mass. |
Stability Analysis
| 3D STABILITY |
|---|
Ellipsoidal & Ellipsoidal-Like
Binary Systems
- S. Chandrasekhar (1963), ApJ, 138, 1182: The Equilibrium and the Stability of the Roche Ellipsoids
- G. P. Horedt (2019), ApJ, 877, 9: On the Instability of Polytropic Maclaurin and Roche Ellipsoids
Nonlinear Evolution
| 3D DYNAMICS |
|---|
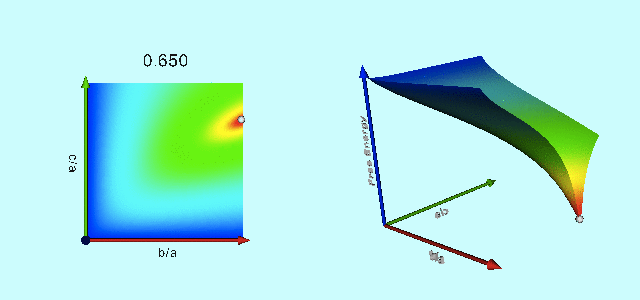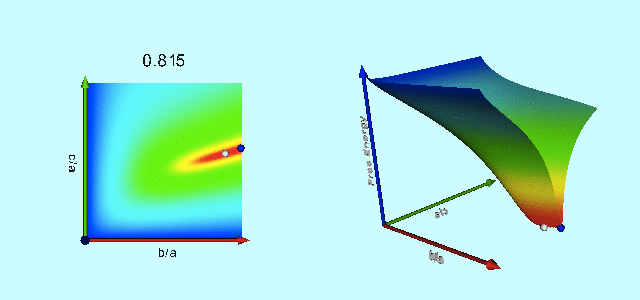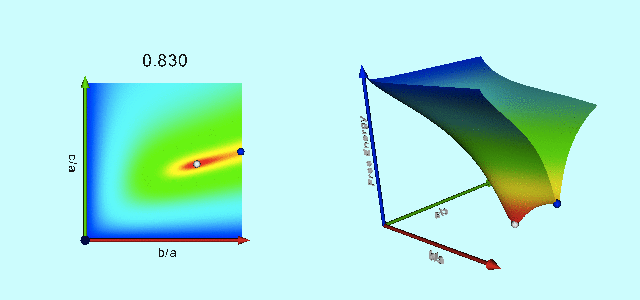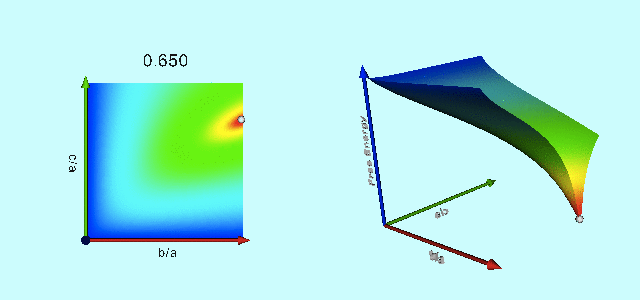
|
Free-Energy Evolution from the Maclaurin to the Jacobi Sequence |
|---|
| Fission Hypothesis |
"Fission" Simulations at LSU |
|---|
Secular
- M. Fujimoto (1971), ApJ, 170, 143: Nonlinear Motions of Rotating Gaseous Ellipsoids
- W. H. Press & S. A. Teukolsky (1973), ApJ, 181, 513: On the Evolution of the Secularly Unstable, Viscous Maclaurin Spheroids
- S. L. Detweiler & L. Lindblom (1977), ApJ, 213, 193: On the evolution of the homogeneous ellipsoidal figures.
Dynamical
See Also

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |