Difference between revisions of "User:Tohline/2DStructure/ToroidalCoordinateIntegrationLimits"
(→Preamble: More preamble material, including general volume integral and general integral for the gravitational potential) |
|||
| Line 43: | Line 43: | ||
<tr> | <tr> | ||
<th align="center" colspan="1"><font size="+1">Zone I</font><p></p> | <th align="center" colspan="1"><font size="+1">Zone I</font><p></p> | ||
<math>~Z_0 > r_t</math> | <math>~Z_0 > r_t</math><p></p>for any <math>~a</math></th> | ||
<th align="center" colspan="1"><font size="+1">Zone II</font><p></p> | <th align="center" colspan="1"><font size="+1">Zone II</font><p></p> | ||
<math>~r_t > Z_0 > 0</math> | <math>~r_t > Z_0 > 0</math><p></p>and<p></p><math>~a < (\varpi_t-r_t)</math></th> | ||
<th align="center" colspan="1"><font size="+1">Zone | <th align="center" colspan="1"><font size="+1">Zone III</font><p></p> | ||
<math>~r_t > Z_0 > 0</math> | <math>~r_t > Z_0 > 0</math><p></p>and<p></p><math>~\varpi_t - \sqrt{r_t^2 - Z_0^2} < a < \varpi+ + \sqrt{r_t^2 - Z_0^2}</math></th> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
| Line 61: | Line 61: | ||
</tr> | </tr> | ||
</table> | </table> | ||
<div align="center"> | |||
<table border="1" cellpadding="8"> | |||
<tr><td align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{V_i}{V_\mathrm{torus}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{a^3}{2\pi \varpi_t r_t^2} \int\limits_{\xi_1 = \lambda_i}^{\xi_1 = \Lambda_i} d\xi_1 | |||
\biggl\{ | |||
\frac{(1-\xi_2^2)^{1/2} [ 4\xi_1^2 - 3\xi_1 \xi_2 - 1]}{(\xi_1^2-1)^2 (\xi_1 - \xi_2)^2} | |||
+ \biggl[ \frac{(2\xi_1^2 + 1)}{(\xi_1^2-1)^{5/2}}\biggr] \cos^{-1}\biggl[ \frac{(\xi_1\xi_2 - 1 )}{(\xi_1- \xi_2)} \biggr] | |||
\biggr\}_{\xi_2 = \gamma_i}^{\xi_2 = \Gamma_i} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<div align="center"> | |||
<table border="1" cellpadding="8" align="center"> | |||
<tr><td align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Phi_i(a,Z_0)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{2^{5/2} G \rho_0 a^{2}}{3} | |||
\int\limits_{\xi_1 = \lambda_i}^{\xi_1 = \Lambda_i} \frac{(\xi_1+1)^{1/2}K(\mu) d\xi_1}{(\xi_1^2 - 1)^2 [ (\xi_1^2 - 1)^{1/2}+\xi_1 ]^{1/2} } | |||
\biggr[ \frac{\sin \theta(5\xi_1^2 - 4\xi_1 \cos \theta - 1)}{(\xi_1+1)^{1/2} (\xi_1 - \cos \theta)^{3/2}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ - 4\xi_1 E\biggl( \frac{\pi-\theta}{2} \, , \sqrt{\frac{2}{\xi_1 + 1}} \biggr) | |||
+ (\xi_1-1) F\biggl( \frac{\pi-\theta}{2} \, , \sqrt{\frac{2}{\xi_1 + 1}} \biggr) \biggr]_{\theta = \cos^{-1}(\gamma_i)}^{\theta = \cos^{-1}(\Gamma_i)} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td></tr> | |||
</table> | |||
</div> | |||
=See Also= | =See Also= | ||
Revision as of 02:41, 10 November 2015
Toroidal-Coordinate Integration Limits
In support of our accompanying discussion of the gravitational potential of a uniform-density circular torus, here we explain in detail what limits of integration must be specified in order to accurately determine the volume — and, hence also the total mass — of such a torus using toroidal coordinates.

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Preamble
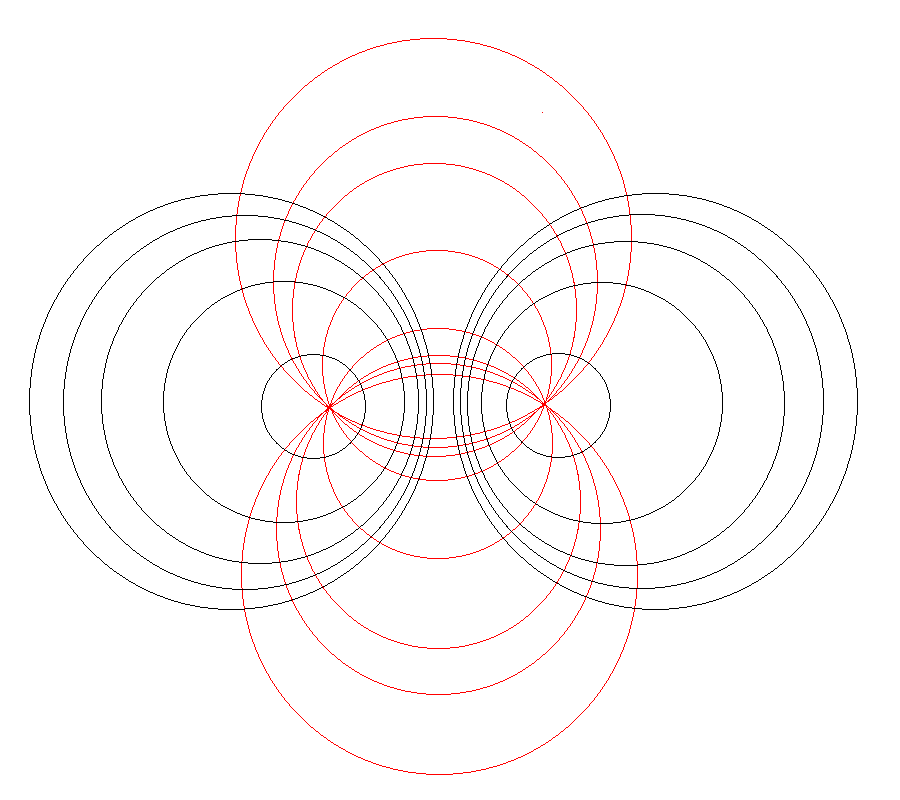
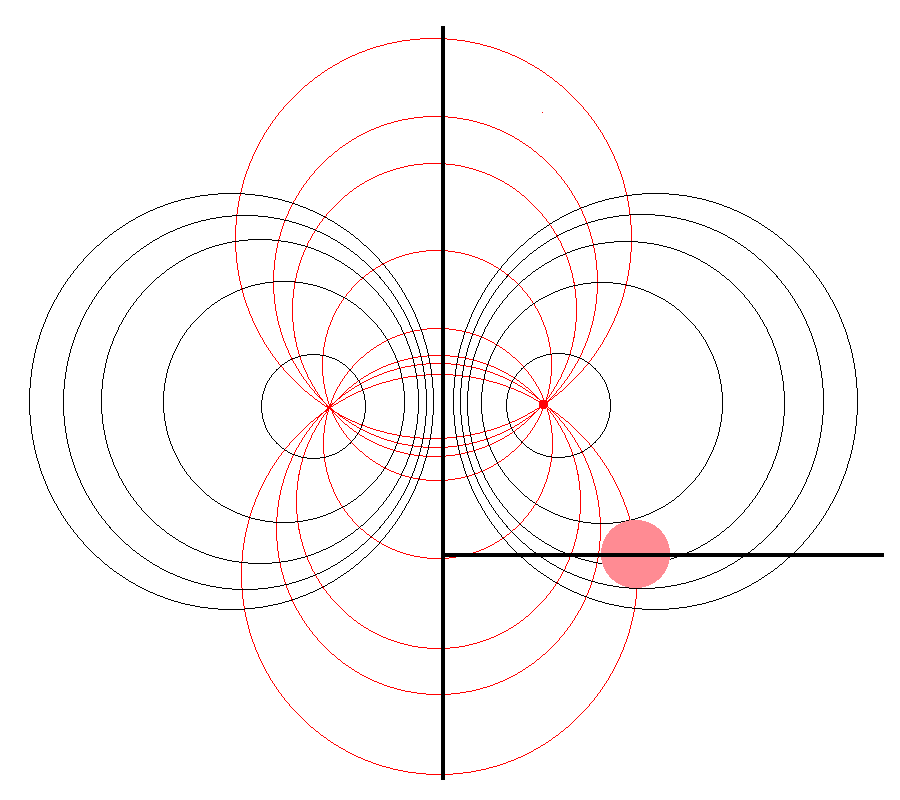
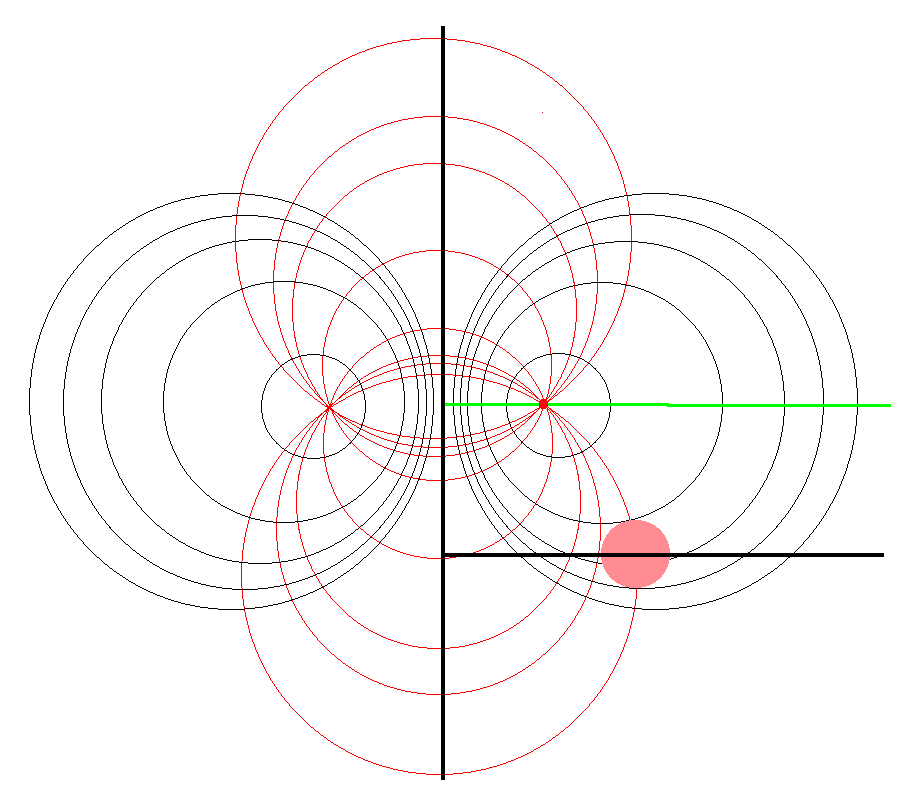
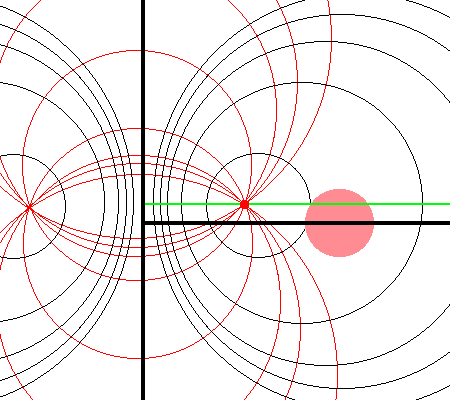
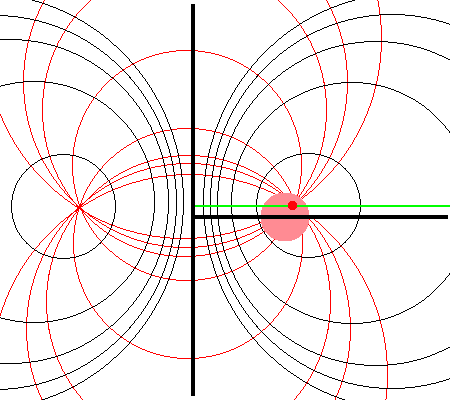
| Apollonian Circles (schematic) (see also Wikipedia's Apollonian Circles) | |
|---|---|
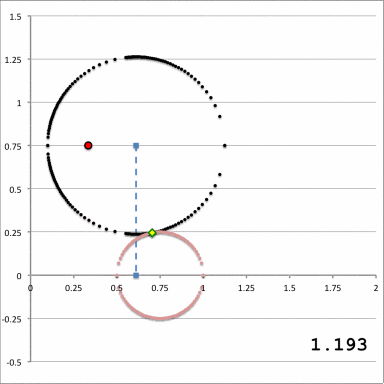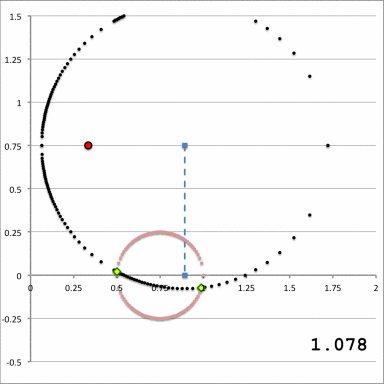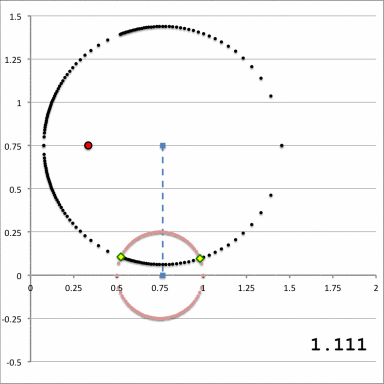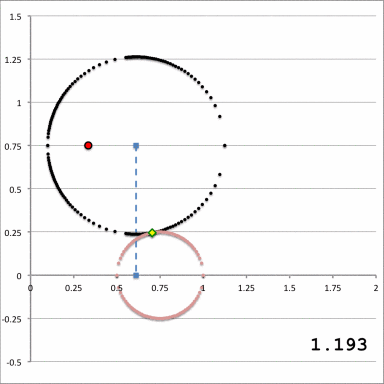
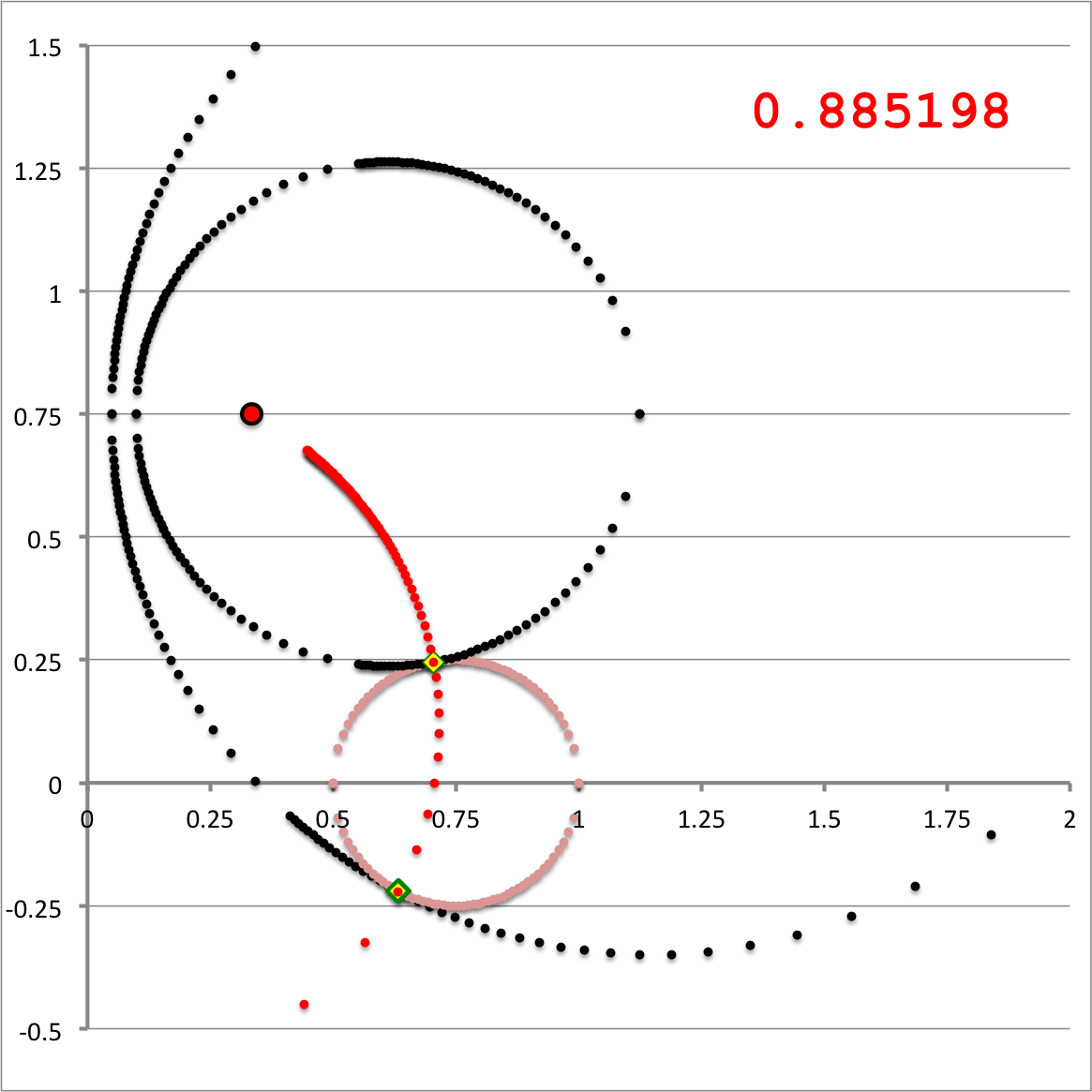
| Quantitative Illustration of Employed Toroidal Coordinate System | |
| Schematic Zones | ||
|---|---|---|
| Zone I <math>~Z_0 > r_t</math>for any <math>~a</math> | Zone II <math>~r_t > Z_0 > 0</math>and<math>~a < (\varpi_t-r_t)</math> | Zone III <math>~r_t > Z_0 > 0</math>and<math>~\varpi_t - \sqrt{r_t^2 - Z_0^2} < a < \varpi+ + \sqrt{r_t^2 - Z_0^2}</math> |
|
|
See Also

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |