User:Tohline/SSC/Structure/PolytropesEmbedded
Embedded Polytropic Spheres
In a separate discussion we showed how to determine the structure of isolated polytropic spheres. These are rather idealized stellar structures in which the pressure and density both drop to zero at the surface of the star. Here we consider how the equilibrium radius of a polytropic configuration of a given <math>~M</math> and <math>~K_\mathrm{n}</math> is modified when it is embedded in an external medium of pressure <math>~P_e</math>. We will begin by reviewing the general properties of embedded (and truncated) polytropes for a wide range of polytropic indexes, principally summarizing the published descriptions provided by Horedt (1970), by Whitworth (1981) and by Stahler (1983). Then we will focus in more detail on polytropes of index <math>~n</math> = 1 and <math>~n</math> = 5 because their structures can be described by analytic mathematical expressions.

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
General Properties
Horedt's Presentation
It appears as though Horedt (1970) was the first to draw an analogy between the mass limit that is associated with bounded isothermal spheres — the so-called Bonnor-Ebert spheres — and the limiting mass that can be found in association with equilibrium sequences of embedded polytropes that have polytropic indexes <math>~n > 3 </math>. Using a tilde to denote values of parameters at the (truncated) edge of a pressure-bounded polytropic sphere, Horedt (see the bottom of his p. 83) derives the following set of parametric equations relating the configuration's dimensionless radius, <math>~r_a</math>, to a specified dimensionless bounding pressure, <math>~p_a</math>:
|
<math> ~r_a \equiv \frac{R_\mathrm{eq}}{R_\mathrm{Horedt}} </math> |
<math>~=~</math> |
<math> \tilde\xi ( -\tilde\xi^2 \tilde\theta' )^{(1-n)/(n-3)} \, , </math> |
|
<math> ~p_a \equiv \frac{P_\mathrm{e}}{P_\mathrm{Horedt}} </math> |
<math>~=~</math> |
<math> \tilde\theta^{n+1}( -\tilde\xi^2 \tilde\theta' )^{2(n+1)/(n-3)} \, , </math> |
where,
|
<math> \tilde\theta' </math> |
<math>~\equiv~</math> |
<math> \frac{d\theta_n}{d\xi} ~~~\mathrm{evaluated}~\mathrm{at}~\tilde\xi \, , </math> |
|
<math> ~R_\mathrm{Horedt} </math> |
<math>~\equiv~</math> |
<math> \alpha_r \biggl( \frac{\alpha_M}{M} \biggr)^{(1-n)/(n-3)} = \biggl[ \frac{4\pi}{(n+1)^n}\biggl( \frac{G}{K} \biggr)^n M^{n-1} \biggr]^{1/(n-3)} \, , </math> |
|
<math> ~P_\mathrm{Horedt} </math> |
<math>~\equiv~</math> |
<math> K \biggl( \frac{\alpha_M}{M} \biggr)^{2(n+1)/(n-3)} = K^{4n/(n-3)}\biggl[ \frac{(n+1)^3}{4\pi G^3 M^2} \biggr]^{2(n+1)/(n-3)} \, . </math> |
Notice that, via these normalizations, Horedt chose to express <math>~R_\mathrm{eq}</math> and <math>~P_\mathrm{e}</math> in terms of the system's polytropic constant, <math>~K</math>, and total mass, <math>~M</math>.
Whitworth's Presentation
In §5 of his paper, Whitworth (1981) also presents the set of parametric equations that define what the equilibrium radius, <math>~R_\mathrm{eq}</math>, is of an embedded polytrope for a certain imposed external pressure, <math>~P_\mathrm{ex}</math>, namely,
|
<math> ~R_\mathrm{eq} </math> |
<math>~=~</math> |
<math> ~R_\mathrm{rf} \biggl\{ \frac{4\eta}{5|\eta-1|} \biggl(\frac{\xi}{3} \biggr)^\eta \biggl|\frac{d\theta_n}{d\xi} \biggr|^{(2-\eta)} \biggr\}_{\xi_e}^{1/(3\eta - 4)} </math> |
|
<math> \Rightarrow ~~~~~ \biggl( \frac{R_\mathrm{eq}}{R_\mathrm{rf}} \biggr)^{(3-n)} </math> |
<math>~=~</math> |
<math> \biggl[ \frac{4(n+1)}{5} \biggr]^{n} \biggl(\frac{\xi_e}{3} \biggr)^{(n+1)} \biggl|\frac{d\theta_n}{d\xi} \biggr|^{(n-1)}_{\xi_e} \, , </math> |
|
<math> ~P_\mathrm{ex} </math> |
<math>~=~</math> |
<math> ~P_\mathrm{rf} \biggl\{ 2^{-8/\eta} \biggl(\frac{5|\eta-1|}{\eta} \biggr)^3 \biggl(\frac{3}{\xi} \biggr)^4 \biggl|\frac{d\theta_n}{d\xi} \biggr|^{-2} \biggr\}_{\xi_e}^{\eta/(3\eta - 4)} \theta_n^{\eta/(\eta-1)} </math> |
|
<math> \Rightarrow ~~~~~ \biggl( \frac{P_\mathrm{ex}}{P_\mathrm{rf}} \biggr)^{(3-n)} </math> |
<math>~=~</math> |
<math> 2^{-8n}\biggl\{ \biggl(\frac{5}{n+1} \biggr)^3 \biggl(\frac{3}{\xi} \biggr)^4 \theta_n^{(3-n)} \biggl|\frac{d\theta_n}{d\xi} \biggr|^{-2} \biggr\}_{\xi_e}^{(n+1)} \, , </math> |
where, in order to obtain the second line of the two relations we have used the substitution, <math>~\eta \rightarrow (1+1/n)</math>, and, as is detailed in an accompanying ASIDE, Whitworth "referenced" <math>~P_\mathrm{ex}</math> and <math>~R_\mathrm{eq}</math> to, respectively,
|
<math> P_\mathrm{rf}^{(4-3\eta)} </math> |
<math>~=~</math> |
<math> 2^{-2(4+\eta)} \biggl( \frac{3^4 \cdot 5^3}{\pi} \biggr)^\eta \biggl[ \frac{K^4}{G^{3\eta} M^{2\eta} } \biggr] </math> |
|
<math> \Rightarrow ~~~~~ P_\mathrm{rf}^{(n-3)} </math> |
<math>~=~</math> |
<math> 2^{-2(5n+1)} \biggl( \frac{3^4 \cdot 5^3}{\pi} \biggr)^{(n+1)} \biggl[ \frac{K^{4n}}{G^{3(n+1)} M^{2(n+1)} } \biggr] \, , </math> |
|
<math> ~R_\mathrm{rf}^\eta </math> |
<math>~=~</math> |
<math> \frac{2^2}{K} \biggl(\frac{GM}{3\cdot 5}\biggr)^\eta P_\mathrm{rf}^{(1-\eta)} </math> |
|
<math> \Rightarrow ~~~~~ R_\mathrm{rf}^{(n+1)} </math> |
<math>~=~</math> |
<math> \biggl( \frac{2^2}{K} \biggr)^{n} \biggl(\frac{GM}{3\cdot 5}\biggr)^{(n+1)} P_\mathrm{rf}^{-1} </math> |
|
<math> \Rightarrow ~~~~~ R_\mathrm{rf}^{(3-n)} </math> |
<math>~=~</math> |
<math> \biggl( \frac{2^2}{K} \biggr)^{n(3-n)/(n+1)} \biggl(\frac{GM}{3\cdot 5}\biggr)^{(3-n)} P_\mathrm{rf}^{(n-3)/(n+1)} </math> |
|
|
<math>~=~</math> |
<math> \biggl( \frac{2^2}{K} \biggr)^{n(3-n)/(n+1)} \biggl(\frac{GM}{3\cdot 5}\biggr)^{(3-n)} \biggl\{2^{-2(5n+1)} \biggl( \frac{3^4 \cdot 5^3}{\pi} \biggr)^{(n+1)} \biggl[ \frac{K^{4n}}{G^{3(n+1)} M^{2(n+1)} } \biggr] \biggr\}^{1/(n+1)} </math> |
|
|
<math>~=~</math> |
<math>K^{n} ( 2^2 )^{-(n+1)} \biggl(\frac{GM}{3\cdot 5}\biggr)^{(3-n)} \biggl( \frac{3^4 \cdot 5^3}{\pi} \biggr) \biggl[ \frac{1}{G^{3} M^{2} } \biggr] </math> |
|
|
<math>~=~</math> |
<math> 2^{-2(n+1)} \pi^{-1} 3^{n+1} \cdot 5^{n} K^n G^{-n} M^{1-n} </math> |
Via these normalizations, Whitworth — as did Hoerdt (1970) — chose to express <math>~R_\mathrm{eq}</math> and <math>~P_\mathrm{ex}</math> in terms of the system's polytropic constant, <math>~K</math>, and total mass, <math>~M</math>.
To convert from Whitworth's expressions, which use one set of normalization parameters <math>~(R_\mathrm{rf},P_\mathrm{rf})</math>, to Horedt's expressions, which use a somewhat different set of normalization parameters — identified here as <math>~(R_\mathrm{Horedt},P_\mathrm{Horedt})</math> — one simply needs to make use of the relations,
|
<math> \biggl( \frac{R_\mathrm{rf}}{R_\mathrm{Horedt}} \biggr)^{(3-n)} </math> |
<math>~=~</math> |
<math> 3^{(n+1)} \biggl[ \frac{5}{2^2 (n+1)} \biggr]^{n} \, . </math> |
|
<math> \biggl( \frac{P_\mathrm{rf}}{P_\mathrm{Horedt}} \biggr)^{(3-n)} </math> |
<math>~=~</math> |
<math> 2^{8n} \biggl[ \frac{(n+1)^3}{3^4 \cdot 5^3} \biggr]^{(n+1)} \, , </math> |
Stahler's Presentation
Similarly, in Appendix B of his work, Stahler (1983) states that the mass, <math>~M_0</math>, associated with the equilibrium radius, <math>~R_0</math>, of embedded polytropic spheres is,
|
<math> ~M_0 </math> |
<math>~=~</math> |
<math> M_\mathrm{norm} \biggl( \frac{n^3}{4\pi} \biggr)^{1/2} \biggl\{ \theta^{(n-3)/2} \xi^2 \biggl| \frac{d\theta}{d\xi} \biggr| \biggr\}_{\xi_e} </math> |
|
<math> ~R_0 </math> |
<math>~=~</math> |
<math> R_\mathrm{norm} \biggl( \frac{n}{4\pi} \biggr)^{1/2} \biggl\{ \xi \theta^{(n-1)/2} \biggr\}_{\xi_e} </math> |
where, from his equations (7) and (B3) we deduce,
<math>M_\mathrm{norm} = \biggl( \frac{n+1}{nG} \biggr)^{3/2} K^{2n/(n+1)} P_\mathrm{ex}^{(3-n)/[2(n+1)]} \, ,</math>
<math> R_\mathrm{norm} = \biggl( \frac{n+1}{nG} \biggr)^{1/2} K^{n/(n+1)} P_\mathrm{ex}^{(1-n)/[2(n+1)]} \, . </math>
Notice that, via these two normalizations, Stahler chose to express <math>~R_\mathrm{0}</math> and <math>~M_\mathrm{0}</math> in terms of the system's polytropic constant, <math>~K</math>, and the applied external pressure, <math>~P_\mathrm{ex}</math>.
Reconciliation
Here we demonstrate that Whitworth's and Stahler's presentations are equivalent to one another. We begin by plugging Stahler's definition of <math>~M_\mathrm{norm}</math> into his expression for <math>~M_0</math>, then inverting it to obtain an expression for <math>~P_\mathrm{ex}</math> in terms of <math>~M_0</math> and <math>~K</math>.
|
<math> ~M_0 </math> |
<math>~=~</math> |
<math> \biggl[ \frac{(n+1)^3}{4\pi G^3} \biggr]^{1/2} K^{2n/(n+1)} P_\mathrm{ex}^{(3-n)/[2(n+1)]} \biggl\{ \theta^{(n-3)/2} \xi^2 \biggl| \frac{d\theta}{d\xi} \biggr| \biggr\}_{\xi_e} </math> |
|
<math> \Rightarrow ~~~~~ P_\mathrm{ex}^{(3-n)} </math> |
<math>~=~</math> |
<math> \biggl[ \frac{4\pi G^3}{(n+1)^3} \biggr]^{(n+1)} K^{-4n} M_0^{2(n+1)} \biggl\{ \theta^{(n-3)/2} \xi^2 \biggl| \frac{d\theta}{d\xi} \biggr| \biggr\}^{-2(n+1)}_{\xi_e} </math> |
|
|
<math>~=~</math> |
<math> \biggl[ \frac{4\pi G^3 M_0^2}{(n+1)^3} \biggr]^{(n+1)} K^{-4n} \biggl\{ \theta^{(3-n)} \xi^{-4} \biggl| \frac{d\theta}{d\xi} \biggr|^{-2} \biggr\}^{(n+1)}_{\xi_e} </math> |
Alternatively, plugging Whitworth's definition of <math>~P_\mathrm{rf}</math> into his expression for <math>~P_\mathrm{ex}</math> gives,
|
<math> ~P_\mathrm{ex}^{(3-n)} </math> |
<math>~=~</math> |
<math> 2^{2(5n+1)} \biggl( \frac{\pi}{3^4 \cdot 5^3} \biggr)^{(n+1)} 2^{-8n} \cdot 3^{4(n+1)} \biggl(\frac{5}{n+1} \biggr)^{3(n+1)} [ G^{3} M^{2} ]^{(n+1)} K^{-4n} \biggl\{ \theta_n^{(3-n)}\xi^{-4} \biggl|\frac{d\theta_n}{d\xi} \biggr|^{-2} \biggr\}_{\xi_e}^{(n+1)} </math> |
|
|
<math>~=~</math> |
<math> 2^{2(n+1)} \biggl[ \frac{\pi}{(n+1)^3} \biggr]^{(n+1)} [ G^{3} M^{2} ]^{(n+1)} K^{-4n} \biggl\{ \theta_n^{(3-n)}\xi^{-4} \biggl|\frac{d\theta_n}{d\xi} \biggr|^{-2} \biggr\}_{\xi_e}^{(n+1)} \, . </math> |
So Whitworth's and Stahler's relations for <math>~P_\mathrm{ex}(M_\mathrm{tot},K)</math> are, indeed, identical. Similarly examining Stahler's expression for the equilibrium radius, we find,
|
<math> ~R_0 </math> |
<math>~=~</math> |
<math> \biggl( \frac{n+1}{4\pi G} \biggr)^{1/2} K^{n/(n+1)} \biggl[ \xi \theta^{(n-1)/2} \biggr]_{\xi_e} \biggl\{ P_\mathrm{ex}^{1/(n+1)} \biggr\}^{(1-n)/2} </math> |
|
|
<math>~=~</math> |
<math> \biggl( \frac{n+1}{4\pi G} \biggr)^{1/2} K^{n/(n+1)} \biggl[ \xi \theta^{(n-1)/2} \biggr]_{\xi_e} \biggl\{ \biggl[ \frac{4\pi G^3 M_0^2}{(n+1)^3} \biggr] K^{-4n/(n+1)} \biggl[ \theta^{(3-n)} \xi^{-4} \biggl| \frac{d\theta}{d\xi} \biggr|^{-2} \biggr]_{\xi_e} \biggr\}^{(1-n)/[2(3-n)]} </math> |
|
<math> \Rightarrow ~~~~~ R_0^{(3-n)} </math> |
<math>~=~</math> |
<math> \biggl( \frac{n+1}{4\pi G} \biggr)^{(3-n)/2} K^{n(3-n)/(n+1)} \xi_e^{3-n} \biggl\{ \biggl[ \frac{4\pi G^3 M_0^2}{(n+1)^3} \biggr]^{1/2} K^{-2n/(n+1)} \biggl[ \xi^{-2} \biggl| \frac{d\theta}{d\xi} \biggr|^{-1} \biggr]_{\xi_e} \biggr\}^{(1-n)} </math> |
|
|
<math>~=~</math> |
<math> (n+1)^{[(3-n)-3(1-n)]/2} (4\pi)^{[(n-3) +(1-n)]/2} G^{[(n-3)+3(1-n)]/2} [K^{(3-n)+2(n-1)}]^{n/(n+1)} \xi_e^{(3-n)+2(n-1)} M_0^{(1-n)} \biggl| \frac{d\theta}{d\xi} \biggr|^{(n-1)}_{\xi_e} </math> |
|
|
<math>~=~</math> |
<math> (n+1)^{n} (4\pi)^{-1} G^{-n} K^n M_0^{(1-n)} \biggl[ \xi^{(n+1)} \biggl| \frac{d\theta}{d\xi} \biggr|^{(n-1)}\biggr]_{\xi_e} \, . </math> |
And Whitworth's expression becomes,
|
<math> ~R_\mathrm{eq}^{(3-n)} </math> |
<math>~=~</math> |
<math> 2^{-2(n+1)} \pi^{-1} 3^{n+1} \cdot 5^{n} K^n G^{-n} M^{1-n} \biggl[ \frac{4(n+1)}{5} \biggr]^{n} \biggl(\frac{\xi_e}{3} \biggr)^{(n+1)} \biggl|\frac{d\theta_n}{d\xi} \biggr|^{(n-1)}_{\xi_e} </math> |
|
|
<math>~=~</math> |
<math> (n+1)^n (4\pi)^{-1} K^n G^{-n} M^{1-n} \xi_e^{(n+1)} \biggl|\frac{d\theta_n}{d\xi} \biggr|^{(n-1)}_{\xi_e} \, . </math> |
Hence, Stahler's equilibrium radius, <math>~R_0</math>, exactly matches Whitworth's <math>~R_\mathrm{eq}</math>.
Summary
|
Once the function, <math>~\theta_n(\xi)</math>, and its first derivative with respect to the dimensionless radial coordinate, <math>~d\theta_n/d\xi</math>, are obtained via a solution of the Lane-Emden equation, the equilibrium radius, <math>~R_\mathrm{eq}</math>, and total mass, <math>~M_\mathrm{tot}</math>, of a pressure-bounded polytrope can be expressed in terms of Stahler's normalizations as follows:
where,
Then, the external pressure, expressed in terms of Whitworth's normalization, is,
and the conversion from Stahler's normalization to Whitworth's normalization of the radius is achieved via the expression,
|
<math>~n</math> = 1 Polytrope
Drawing from the earlier discussion of isolated polytropes, we will reference various radial locations within the spherical configuration by the dimensionless radius,
<math> \xi \equiv \frac{r}{a_\mathrm{n=1}} , </math>
where,
<math> a_\mathrm{n=1} \equiv \biggl[\frac{1}{4\pi G}~ \biggl( \frac{H_c}{\rho_c} \biggr)_{n=1}\biggr]^{1/2} = \biggl[\frac{K}{2\pi G} \biggr]^{1/2} \, . </math>
Review
Again, from the earlier discussion, we can describe the properties of an isolated, spherical <math>~n</math> = 1 polytrope as follows:
- Mass:
- In terms of the central density, <math>\rho_c</math>, and <math>~K_\mathrm{n}</math>, the total mass is,
<math>M = \frac{4}{\pi} \rho_c (\pi a_{n=1})^3 = 4\pi^2 \rho_c \biggl[\frac{K}{2\pi G} \biggr]^{3/2} = \rho_c \biggl[\frac{2\pi K^3}{G^3} \biggr]^{1/2}</math> ;
- and, expressed as a function of <math>M</math>, the mass that lies interior to the dimensionless radius <math>\xi</math> is,
<math>\frac{M_\xi}{M} = \frac{1}{\pi} \biggl[ \sin\xi - \xi\cos\xi \biggr] \, ,~~~~~~\mathrm{for}~\pi \ge \xi \ge 0 \, .</math>
- Hence,
<math>M_\xi = \rho_c \biggl[\frac{2K^3}{\pi G^3} \biggr]^{1/2} \biggl[ \sin\xi - \xi\cos\xi \biggr] \, .</math>
- Pressure:
- The central pressure of the configuration is,
<math>P_c = \biggl[ \frac{G^3}{2\pi} \rho_c^4 M^2 \biggr]^{1/3} = \biggl[ \frac{G^3}{2\pi} \rho_c^6 \biggl(\frac{2\pi K^3}{G^3} \biggr) \biggr]^{1/3} = K\rho_c^2</math> ;
- and, expressed in terms of the central pressure <math>P_c</math>, the variation with radius of the pressure is,
<math>P_\xi= P_c \biggl[ \frac{\sin\xi}{\xi} \biggr]^2</math> .
- Hence,
<math>P_\xi= K\rho_c^2 \biggl[ \frac{\sin\xi}{\xi} \biggr]^2</math> .
Extension to Bounded Sphere
Eliminating <math>\rho_c</math> between the last expression for <math>M_\xi</math> and the last expression for <math>P_\xi</math> gives,
<math>P_\xi= \biggl[\frac{\pi}{2} \cdot \frac{G^3 M_\xi^2}{K^2} \biggr] \biggl[ \frac{\sin\xi}{\xi(\sin\xi - \xi \cos\xi )} \biggr]^2</math> .
Now, if we rip off an outer layer of the star down to some dimensionless radius <math>\xi_e < \pi</math>, the interior of the configuration that remains — containing mass <math>M_{\xi_e}</math> — should remain in equilibrium if we impose the appropriate amount of externally applied pressure <math>P_e = P_{\xi_e} </math> at that radius. (This will work only for spherically symmetric configurations, as the gravitation acceleration at any location only depends on the mass contained inside that radius.) If we rescale our solution such that the mass enclosed within <math>\xi_e</math> is the original total mass <math>M</math>, then the pressure that must be imposed by the external medium in which the configuration is embedded is,
<math>P_e= \biggl[\frac{\pi}{2} \cdot \frac{G^3 M^2}{K^2} \biggr] \biggl[ \frac{\sin\xi_e}{\xi_e(\sin\xi_e - \xi_e \cos\xi_e )} \biggr]^2</math> .
The associated equilibrium radius of this pressure-confined configuration is,
<math> R_\mathrm{eq} = \xi_e a_\mathrm{n=1} = \biggl[ \frac{K}{2\pi G} \biggr]^{1/2} \xi_e </math>
Overlap with Whitworth's Presentation
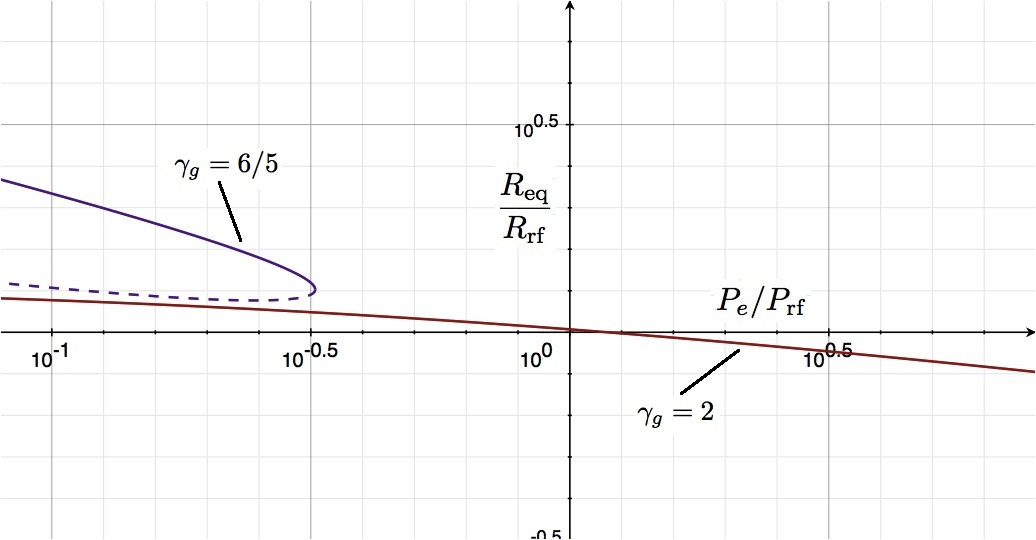
The solid red curve in the top portion of Figure 1 shows how <math>R_\mathrm{eq}</math> varies with the applied external pressure <math>P_e</math>. Following the lead of Whitworth (1981, MNRAS, 195, 967) — for clarification, read the accompanying ASIDE — these two quantities have been respectively normalized (or, "referenced") to,
<math> R_\mathrm{rf}\biggr|_\mathrm{n=1} \equiv \biggl( \frac{3^2 \cdot 5}{2^4 \pi} \biggr)^{1/2} \biggl(\frac{K}{G}\biggr)^{1/2} ~~~\Rightarrow ~~~ \frac{R_\mathrm{eq}}{R_\mathrm{rf}} = \biggl( \frac{2^3}{3^2 \cdot 5} \biggr)^{1/2} \xi_e \, ; </math>
and,
<math> P_\mathrm{rf}\biggr|_\mathrm{n=1} \equiv \frac{2^6 \pi}{3^4 \cdot 5^3} \biggl(\frac{G^3 M^2}{K^2}\biggr) ~~~\Rightarrow ~~~ \frac{P_e}{P_\mathrm{rf}} = \biggl( \frac{3^4 \cdot 5^3}{2^7} \biggr) \biggl[ \frac{\sin\xi_e}{\xi_e(\sin\xi_e - \xi_e \cos\xi_e )} \biggr]^2 \, . </math>
|
Figure 1: Equilibrium R-P Diagram |
|
|
Top: The solid red curve shows how the equilibrium radius <math>(R_\mathrm{eq})</math> of an <math>~n</math> = 1 <math>(\gamma_g = 2)</math> polytrope varies with applied external pressure, <math>P_e</math>; similarly, the purple curve shows the <math>R_\mathrm{eq}(P_e)</math> behavior for an <math>~n</math> = 5 <math>(\gamma_g=6/5)</math> polytrope. Logarithmic units are used along both axes; <math>P_e</math> is normalized to <math>P_\mathrm{rf}</math> and <math>R_\mathrm{eq}</math> is normalized to <math>R_\mathrm{rf}</math>, as defined in the text and in the accompanying ASIDE.
|
|
Overlap with Stahler's Presentation
We can invert the above expression for <math>~P_e(K,M)</math> to obtain the following expression for <math>~M(K,P_e)</math>:
<math>~M= K \biggl[\frac{2}{\pi} \cdot \frac{P_e}{G^3} \biggr]^{1/2} \biggl[ \frac{\xi_e(\sin\xi_e - \xi_e \cos\xi_e )}{\sin\xi_e} \biggr]</math> .
If, following Stahler's lead, we normalize this expression by <math>~M_\mathrm{norm}</math> (evaluated for <math>~n=1</math>) and we normalize the above expression for <math>~R_\mathrm{eq}</math> by <math>~R_\mathrm{norm}</math> (evaluated for <math>~n=1</math>), we obtain,
|
<math> \frac{M}{M_\mathrm{norm}} </math> |
<math>~=~</math> |
<math> K \biggl[\frac{2}{\pi} \cdot \frac{P_e}{G^3} \biggr]^{1/2} \biggl[ \frac{\xi_e(\sin\xi_e - \xi_e \cos\xi_e )}{\sin\xi_e} \biggr] \biggl[ \biggl( \frac{G}{2} \biggr)^{3/2} K^{-1} P_\mathrm{ex}^{-1/2} \biggr] </math> |
|
|
<math>~=~</math> |
<math> (4\pi)^{-1/2} \biggl[ \frac{\xi_e(\sin\xi_e - \xi_e \cos\xi_e )}{\sin\xi_e} \biggr] \, , </math> |
|
<math> \frac{R_\mathrm{eq}}{R_\mathrm{norm}} </math> |
<math>~=~</math> |
<math> \biggl[ \frac{K}{2\pi G} \biggr]^{1/2} \xi_e \biggl[ \frac{G}{2K} \biggr]^{1/2} = (4\pi)^{-1/2} \xi_e \, . </math> |
<math>~n</math> = 5 Polytrope
Drawing from the earlier discussion of isolated polytropes, we will reference various radial locations within a spherical <math>~n</math> = 5 polytrope by the dimensionless radius,
<math> \xi \equiv \frac{r}{a_\mathrm{n=5}} , </math>
where,
<math> a_{n=5} = \biggr[ \frac{(n+1)K}{4\pi G} \rho_c^{(1/n - 1)} \biggr]^{1/2}_{n=5} = \biggr[ \frac{3K}{2\pi G} \biggr]^{1/2} \rho_c^{-2/5} \, . </math>
Review
Again, from the earlier discussion, we can describe the properties of an isolated, spherical <math>~n</math> = 5 polytrope as follows:
- Mass:
- In terms of the central density, <math>\rho_c</math>, and <math>~K_\mathrm{n}</math>, the total mass is,
<math>M = \biggr[ \frac{2\cdot 3^4 K^3}{\pi G^3} \biggr]^{1/2} \rho_c^{-1/5} </math> ;
- and, expressed as a function of <math>M</math>, the mass that lies interior to the dimensionless radius <math>\xi</math> is,
<math> \frac{M_\xi}{M} = \xi^3 (3 + \xi^2)^{-3/2} \, . </math>
- Hence,
<math> M_\xi = \biggr[ \frac{2\cdot 3^4 K^3}{\pi G^3} \biggr]^{1/2} \rho_c^{-1/5} \biggl[ \xi^3 (3 + \xi^2)^{-3/2} \biggr] \, . </math>
- Pressure:
- The central pressure of the configuration is,
<math> P_c = \biggr[ \frac{\pi M^2 G^3}{2\cdot 3^4} \biggr]^{1/3} \rho_c^{4/3} = \biggr[ \frac{\pi G^3}{2\cdot 3^4} \biggr( \frac{2\cdot 3^4 K^3}{\pi G^3} \biggr) \rho_c^{-2/5}\biggr]^{1/3} \rho_c^{4/3} = K\rho_c^{6/5} </math> ;
- and, expressed in terms of the central pressure <math>P_c</math>, the variation with radius of the pressure is,
<math>P_\xi= P_c \biggl[ 1 + \frac{1}{3}\xi^2 \biggr]^{-3}</math> .
- Hence,
<math> P_\xi = K \rho_c^{6/5} \biggl[ 1 + \frac{1}{3}\xi^2 \biggr]^{-3} = 3^3K \rho_c^{6/5} [ 3 + \xi^2 ]^{-3} </math> .
Extension to Bounded Sphere
Eliminating <math>\rho_c</math> between the last expression for <math>M_\xi</math> and the last expression for <math>P_\xi</math> gives,
| <math>P_\xi</math> | <math>=</math> |
<math> 3^3K [ 3 + \xi^2 ]^{-3} \biggr[ \frac{2\cdot 3^4 K^3}{\pi G^3} \biggr]^{3} M_\xi^{-6} \biggl[ \xi^3 (3 + \xi^2)^{-3/2} \biggr]^6 </math> |
| <math>=</math> |
<math> \biggl( \frac{2^3\cdot 3^{15} K^{10}}{\pi^3 M_\xi^{6} G^9} \biggr) \frac{\xi^{18}}{(3 + \xi^2)^{12}} \, . </math> |
Now, if we rip off an outer layer of the star down to some dimensionless radius <math>\xi_e < \infty</math>, the interior of the configuration that remains — containing mass <math>M_{\xi_e}</math> — should remain in equilibrium if we impose the appropriate amount of externally applied pressure <math>P_e = P_{\xi_e} </math> at that radius. (This will work only for spherically symmetric configurations, as the gravitation acceleration at any location only depends on the mass contained inside that radius.) If we rescale our solution such that the mass enclosed within <math>\xi_e</math> is the original total mass <math>M</math>, then the pressure that must be imposed by the external medium in which the configuration is embedded is,
<math>P_e= \biggr( \frac{2^3\cdot 3^{15} K^{10}}{\pi^3 M^{6} G^9} \biggr) \frac{\xi_e^{18}}{(3 + \xi_e^2)^{12}} </math> .
The associated equilibrium radius of this pressure-confined configuration is,
<math> R_\mathrm{eq} = \xi_e a_\mathrm{n=5} = \biggl[ \frac{3K}{2\pi G} \biggr]^{1/2} \rho_c^{-2/5} \xi_e = \biggl[ \frac{\pi M^4 G^5}{2^3 \cdot 3^7 K^5} \biggr]^{1/2} \frac{(3+\xi_e^2)^3}{\xi_e^5} \, . </math>
Overlap with Whitworth's Presentation
The purple curve in the top portion of Figure 1 shows how <math>R_\mathrm{eq}</math> varies with the applied external pressure <math>P_e</math>. Following the lead of Whitworth (1981, MNRAS, 195, 967) — for clarification, read the accompanying ASIDE — these two quantities have been respectively normalized (or, "referenced") to,
<math> R_\mathrm{rf}\biggr|_\mathrm{n=5} \equiv \frac{2^6}{3^3} \biggl( \frac{\pi}{5^5} \biggr)^{1/2} \biggl[ \frac{G^5 M^4}{K^5} \biggr]^{1/2} ~~~\Rightarrow ~~~ \frac{R_\mathrm{eq}}{R_\mathrm{rf}} = \biggl( \frac{5^5}{2^{15}\cdot 3} \biggr)^{1/2} \frac{(3+\xi_e^2)^3}{\xi_e^5} \, ; </math>
and,
<math> P_\mathrm{rf}\biggr|_\mathrm{n=5} \equiv \frac{3^{12} 5^9}{2^{26} \pi^3} \biggl( \frac{K^{10}}{G^9 M^6} \biggr) ~~~\Rightarrow ~~~ \frac{P_e}{P_\mathrm{rf}} = \biggl( \frac{2^{29}\cdot 3^{3} }{5^9} \biggr) \frac{\xi_e^{18}}{(3 + \xi_e^2)^{12}} \, . </math>
We see that this (purple) curve bends back on itself. That is to say, for this polytropic index there is an externally applied pressure above which no equilibrium configuration exists. This limiting pressure arises along the curve where,
<math>\frac{dP_e}{dR_\mathrm{eq}} = \biggl( \frac{dP_e}{d\xi_e} \biggr) \biggl( \frac{dR_\mathrm{eq}}{d\xi_e} \biggr)^{-1} = 0 \, .</math>
Evaluation of this expression shows that the limiting pressure occurs precisely at <math>\xi_e = 3</math>, that is,
<math> \biggl( \frac{P_e}{P_\mathrm{rf}} \biggr)_\mathrm{max} = \biggl( \frac{2^{29}\cdot 3^{3} }{5^9} \biggr) \frac{3^{18}}{12^{12}} = \frac{2^5 \cdot 3^9}{5^9} \, , </math>
and the radius of this limiting configuration is,
<math> \biggl( \frac{R_\mathrm{eq}}{R_\mathrm{rf}} \biggr) = \biggl( \frac{5^5}{2^{15}\cdot 3} \biggr)^{1/2} \frac{12^3}{3^5} = \biggl( \frac{5^5}{2^3 \cdot 3^5} \biggr)^{1/2} \, . </math>
On the above log-log plot, the location of this special point is <math>[ \log(P_e/P_\mathrm{rf}) , \log(R_\mathrm{eq}/R_\mathrm{rf}) ] \approx [ -0.49149, +0.10308 ] \, .</math>
Overlap with Stahler's Presentation
We can invert the above expression for <math>~P_e(K,M)</math> to obtain the following expression for <math>~M(K,P_e)</math>:
<math>M^{6}= \biggr( \frac{2^3\cdot 3^{15} K^{10}}{\pi^3 P_e G^9} \biggr) \frac{\xi_e^{18}}{(3 + \xi_e^2)^{12}} </math> .
If, following Stahler's lead, we normalize this expression by <math>~M_\mathrm{norm}</math> (evaluated for <math>~n=5</math>) and we normalize the above expression for <math>~R_\mathrm{eq}</math> by <math>~R_\mathrm{norm}</math> (evaluated for <math>~n=5</math>), we obtain,
|
<math> \frac{M}{M_\mathrm{norm}} </math> |
<math>~=~</math> |
<math> \biggr( \frac{2^3\cdot 3^{15} K^{10}}{\pi^3 P_e G^9} \biggr)^{1/6} \frac{\xi_e^{3}}{(3 + \xi_e^2)^{2}} \biggl[ \biggl( \frac{2\cdot 3}{5G} \biggr)^{3/2} K^{5/3} P_\mathrm{ex}^{-1/6} \biggr]^{-1} </math> |
|
|
<math>~=~</math> |
<math> \biggr( \frac{3^{2} \cdot 5^3 }{4\pi } \biggr)^{1/2} \frac{\xi_e^{3}}{(3 + \xi_e^2)^{2}} \, , </math> |
|
<math> \frac{R_\mathrm{eq}}{R_\mathrm{norm}} </math> |
<math>~=~</math> |
<math> \biggl[ \frac{\pi M^4 G^5}{2^3 \cdot 3^7 K^5} \biggr]^{1/2} \frac{(3+\xi_e^2)^3}{\xi_e^5} \biggl[ \biggl( \frac{2\cdot 3}{5G} \biggr)^{1/2} K^{5/6} P_\mathrm{ex}^{-1/3} \biggr]^{-1} \, . </math> |
Related Discussions
- Constructing BiPolytropes
- Analytic description of BiPolytrope with <math>(n_c, n_e) = (5,1)</math>
- Bonnor-Ebert spheres
- Bonnor-Ebert Mass according to Wikipedia
- A MATLAB script to determine the Bonnor-Ebert Mass coefficient developed by Che-Yu Chen as a graduate student in the University of Maryland Department of Astronomy
- Schönberg-Chandrasekhar limiting mass
- Relationship between Bonnor-Ebert and Schönberg-Chandrasekhar limiting masses
- Wikipedia introduction to the Lane-Emden equation
- Wikipedia introduction to Polytropes

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |