User:Tohline/SSC/VariationalPrinciple
Ledoux's Variational Principle

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Review by Ledoux and Walraven
P. Ledoux & Th. Walraven (1958) discuss linearization of the principal governing equations and stellar pulsation primarily from an Eulerian perspective. Focusing on §57 (pp. 455 - 458) of their Handbuch der Physik article — which falls under the major heading, "Radial oscillations of a gaseous sphere under its own gravitation" — we note, first that they use <math>~\delta r</math> to denote the radial displacement and use primes to identify all Eulerian perturbations. Then, in separating out the spatial and time dependences, they use the notation (see their equation 57.14),
Ledoux and Pekeris (1941)
Historically, by the 1940s, the expression just derived was a relatively familiar one to astrophysicists. For example, the opening paragraph of a 1941 paper by Ledoux & Pekeris (1941, ApJ, 94, 124), reads:
|
Paragraph extracted from P. Ledoux & C. L. Pekeris (1941)
"Radial Pulsations of Stars"
ApJ, vol. 94, pp. 124-135 © American Astronomical Society |
If we divide their equation (1) through by <math>~Xr = \Gamma_1 P r</math> and recognize that,
<math> \frac{dX}{dr} = \frac{dX}{dm}\frac{dm}{dr} = - \Gamma_1 g_0 \rho \, , </math>
we obtain,
<math> \frac{d^2\xi}{dr^2} + \biggl[ \frac{4}{r} - \frac{g_0 \rho}{P} \biggr] \frac{d\xi}{dr} +\frac{\rho}{\Gamma_1 P} \biggl[ \sigma^2 + (4 - 3\Gamma_1) \frac{g_0}{r} \biggr] \xi = 0 \, . </math>
This is clearly the same <math>2^\mathrm{nd}</math>-order, ordinary differential equation as the one we have derived, but with a more general definition of the adiabatic exponent that allows consideration of a situation where the total pressure is a sum of both gas and radiation pressure.
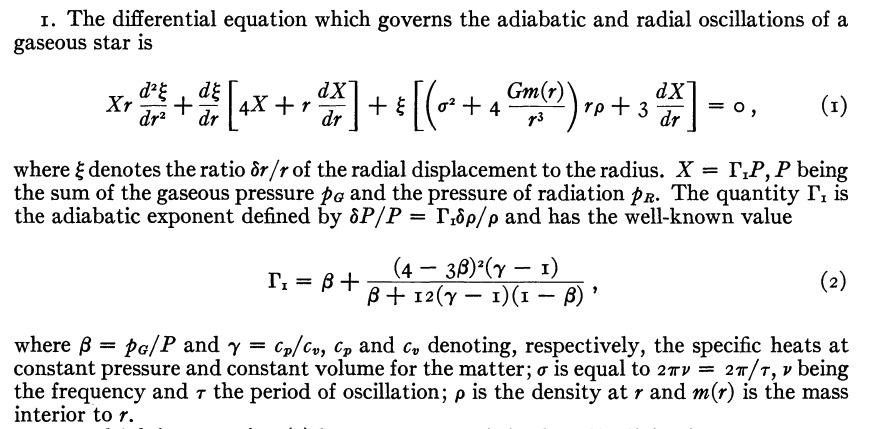
Chandrasekhar (1964)
S. Chandrasekhar (1964, ApJ, 139, 664)
See Also

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |