Numerically Determined Eigenvectors of a Zero-Zero Bipolytrope

Here we build on the analytic foundation summarized in an accompanying chapter and attempt to numerically construct a variety of eigenvectors that describe radial oscillations of bipolytropes for which, <math>~(n_c, n_e) = (0,0)</math>.

Setup

We'll begin with the linear-adiabatic wave equations that describe oscillations of the core and envelope, separately. We also will immediately restrict our investigation to configurations for which,

<math>~g^2 = \mathcal{B} </math> <math>~\Rightarrow</math> <math>~g^2 = \frac{1+8q^3}{ (1+2q^3)^2 } \, ,</math> and, <math>~q^3 = \mathcal{D} = \biggl[ \frac{\rho_e/\rho_c}{2(1-\rho_e/\rho_c)} \biggr] </math> <math>~\Rightarrow</math> <math>~\frac{\rho_e}{\rho_c} = \frac{2q^3}{1+2q^3} \, .</math>

For the core we have,

<math>~ (1 - \eta^2)\frac{d^2x}{d\eta^2} + ( 4 - 6\eta^2 ) \frac{1}{\eta} \cdot \frac{dx}{d\eta} + \mathfrak{F}_\mathrm{core} x \, , </math>

where,

<math>~\eta \equiv \frac{\xi}{g} \, ,</math> and <math>~\mathfrak{F}_\mathrm{core} \equiv \frac{3\omega_\mathrm{core}^2}{2\pi G\gamma_c \rho_c} - 2\alpha_c\, .</math>

And, for the envelope we have,

<math>~ ( 1 - q^3 \xi^3 ) \frac{d^2x}{d\xi^2} + ( 3 - 6q^3 \xi^3 ) \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]\frac{x}{\xi^2} \, , </math>

where,

<math>~\mathfrak{F}_\mathrm{env}</math>

<math>~\equiv</math>

<math>~\frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} - 2\alpha_e \, . </math>

Initial Focus

Properties of 21Analytic Solution

Same as here, but renormalized such that the eigenfunction amplitude is unity at the center of the configuration.

Evidently, one analytic solution with quantum numbers, <math>~(\ell,j) = (2,1)</math>, shown again here on the right, is available for a zero-zero bipolytrope that has the following properties:

<math>~q</math>	<math>~\approx</math>	<math>~0.6840119</math>
<math>~\frac{\rho_e}{\rho_c} = \frac{2q^3}{1+2q^3}</math>	<math>~\approx</math>	<math>~0.3902664</math>
<math>~\gamma_e = \frac{4}{3+0.35}</math>	<math>~\approx</math>	<math>~1.1940299</math>
<math>~\gamma_c </math>	<math>~\approx</math>	<math>~1.845579</math>
<math>~\sigma_c^2 \equiv \frac{3\omega^2}{2\pi G \rho_c} = 20\gamma_c - 8 </math>	<math>~\approx</math>	<math>~28.91158 \, .</math>

This means, as well, that,

<math>~c_0 \equiv \sqrt{1+\alpha_e} - 1</math>	<math>~=</math>	<math>~\sqrt{0.65}-1 \approx - 0.1937742</math>
<math>~g^2 \equiv \frac{1+8q^3}{(1+2q^3)^2}</math>	<math>~\approx</math>	<math>~1.3236092</math>
<math>~\mathfrak{F}_\mathrm{core} \equiv \frac{\sigma_c^2 + 8}{\gamma_c} - 6</math>	<math>~=</math>	<math>~14</math>
<math>~\mathfrak{F}_\mathrm{env} \equiv \frac{1}{\gamma_e} \biggl[ \sigma_c^2 \biggl(\frac{\rho_c}{\rho_e} \biggr) + 8\biggr]- 6</math>	<math>~=</math>	<math>~(c_0^2 + 17c_0 +66) = 62.743385</math>

In the envelope, the analytically defined eigenfunction is given by the expression,

<math>~x_{\ell=2} |_\mathrm{env}</math>

<math>~ \xi^{c_0}\biggl[ \frac{ 1 + q^3 A_{21} \xi^{3} + q^6 A_{21}B_{21}\xi^{6} }{ 1 + q^3 A_{21} + q^6 A_{21}B_{21}}\biggr] \, , </math>

where,

<math>~A_{21}</math>	<math>~\equiv</math>	<math>~-\biggl( \frac{ 4c_0 + 22}{2c_0 + 5}\biggr) \approx -4.6016533 \, ,</math>
<math>~B_{21}</math>	<math>~\equiv</math>	<math>~-\biggl( \frac{c_0 + 7 }{2c_0+8}\biggr) \approx -0.8940912 \, ; </math>

and in the core, it is,

<math>~x_{j=1} |_\mathrm{core}</math>

More succinctly we have,

<math>~x_\mathrm{core}</math>

and,

<math>~a \cdot x_\mathrm{env}</math>

<math>~ \xi^{- 0.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] \, , </math>

where,

<math>~a \equiv -[ 1 + q^3 A_{21} + q^6 A_{21}B_{21}] \approx - 0.05128445 \, .</math>

Demonstrate Core Solution

This means that,

<math>~\frac{dx_\mathrm{core}}{d\xi}</math>

and,

<math>~\frac{d^2x_\mathrm{core}}{d\xi^2}</math>

Therefore, the LAWE for the core becomes,

<math>~[\mathrm{LAWE}]_\mathrm{core}</math>	<math>~=</math>	<math>~ (1 - \eta^2)\frac{d^2x_\mathrm{core}}{d\eta^2} + ( 4 - 6\eta^2 ) \frac{1}{\eta} \cdot \frac{dx_\mathrm{core}}{d\eta} + \mathfrak{F}_\mathrm{core} x_\mathrm{core} </math>
	<math>~=</math>	<math>~ (g^2 - \xi^2)\frac{d^2x_\mathrm{core}}{d\xi^2} + ( 4g^2 - 6\xi^2 ) \frac{1}{\xi} \cdot \frac{dx_\mathrm{core}}{d\xi} + \mathfrak{F}_\mathrm{core} x_\mathrm{core} </math>
	<math>~=</math>	<math>~ 36.65364(1.3236092 - \xi^2) + 36.65364( 5.2944368 - 6\xi^2 ) + 14( -17.326820 + 18.326820~\xi^2) </math>
	<math>~=</math>	<math>~ 36.65364(1.3236092 ) + 36.65364( 5.2944368 ) + 14( -17.326820 ) + [36.65364(-1) + 36.65364( - 6 ) + 14( 18.326820)]\xi^2 </math>
	<math>~=</math>	<math>~ 36.65364(6.618046 ) - 14( 17.326820 ) + 36.65364 [-1 - 6 + 7]\xi^2 </math>
	<math>~=</math>	<math>~ 0 \, . </math>

Q.E.D.

Demonstrate Envelope Solution

Given that,

<math>~a\cdot x_\mathrm{env}</math>

<math>~ \xi^{- 0.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] \, , </math>

we deduce that,

<math>~a\cdot \frac{dx_\mathrm{env}}{d\xi}</math>

<math>~ -0.1937742~ \xi^{- 1.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] + \xi^{- 0.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] \, , </math>

and,

<math>~a \cdot \frac{d^2x_\mathrm{env}}{d\xi^2}</math>	<math>~=</math>	<math>~ 0.2313226~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] - 2 \times 0.1937742~ \xi^{- 1.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] </math>
		<math>~ + \xi^{- 0.1937742}\biggl[ -8.8360086~ \xi + 12.641508~\xi^{4} \biggr] \, . </math>

Therefore, the LAWE for the envelope becomes,

<math>~a\cdot [\mathrm{LAWE}]_\mathrm{env}</math>	<math>~=</math>	<math>~ a( 1 - q^3 \xi^3 ) \frac{d^2x}{d\xi^2} + a( 3 - 6q^3 \xi^3 ) \frac{1}{\xi} \cdot \frac{dx}{d\xi} + a\biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]\frac{x}{\xi^2} \, , </math>
	<math>~=</math>	<math>~ a\biggl\{ \frac{d^2x}{d\xi^2} + \frac{3}{\xi} \cdot \frac{dx}{d\xi} -\alpha_e \biggl(\frac{x}{\xi^2} \biggr) \biggr\} - a q^3 \xi^3 \biggl\{ \frac{d^2x}{d\xi^2} + \frac{6}{\xi} \cdot \frac{dx}{d\xi} - \mathfrak{F}_\mathrm{env} \biggl(\frac{x}{\xi^2} \biggr)\biggr\} \, . </math>

Now, the first of these sub-expressions gives,

<math>~ a\biggl\{ \frac{d^2x}{d\xi^2} + \frac{3}{\xi} \cdot \frac{dx}{d\xi} -\alpha_e \biggl(\frac{x}{\xi^2} \biggr) \biggr\} </math>	<math>~=</math>	<math>~ 0.2313226~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] - 2 \times 0.1937742~ \xi^{- 1.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] </math>
		<math>~ + \xi^{- 0.1937742}\biggl[ -8.8360086~ \xi + 12.641508~\xi^{4} \biggr] </math>
		<math>~ -0.5813226~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] + 3\xi^{- 1.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] </math>
		<math>~+0.35 \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] </math>
	<math>~=</math>	<math>~ (0.2313226 -0.5813226 + 0.35) ~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] </math>
		<math>~ + (3 - 2 \times 0.1937742)~ \xi^{- 1.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] + \xi^{- 0.1937742}\biggl[ -8.8360086~ \xi + 12.641508~\xi^{4} \biggr] </math>
	<math>~=</math>	<math>~ (3 - 2 \times 0.1937742)~ \xi^{- 2.1937742}\biggl[ - 4.4180043~ \xi^{3} + 2.5283016~\xi^{6} \biggr] + \xi^{- 2.1937742}\biggl[ -8.8360086~ \xi^3 + 12.641508~\xi^{6} \biggr] </math>
	<math>~=</math>	<math>~ \xi^{- 2.1937742}\biggl[ -20.37783~ \xi^3 + 19.24657~\xi^{6} \biggr] </math>

And the sub-expression inside the second set of curly braces gives,

<math>~ a\biggl\{ \frac{d^2x}{d\xi^2} + \frac{6}{\xi} \cdot \frac{dx}{d\xi} -\mathfrak{F}_\mathrm{env} \biggl(\frac{x}{\xi^2} \biggr) \biggr\} </math>	<math>~=</math>	<math>~ 0.2313226~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] - 2 \times 0.1937742~ \xi^{- 1.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] </math>
		<math>~ + \xi^{- 0.1937742}\biggl[ -8.8360086~ \xi + 12.641508~\xi^{4} \biggr] </math>
		<math>~ -1.1626452~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] + 6\xi^{- 1.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] </math>
		<math>~- 62.74339 \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] </math>
	<math>~=</math>	<math>~ (0.2313226 -1.1626452 - 62.74339)~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] </math>
		<math>~ + (6- 2 \times 0.1937742)~ \xi^{- 1.1937742}\biggl[ - 4.4180043~ \xi^{2} + 2.5283016~\xi^{5} \biggr] + \xi^{- 0.1937742}\biggl[ -8.8360086~ \xi + 12.641508~\xi^{4} \biggr] </math>
	<math>~=</math>	<math>~ -63.67471~ \xi^{- 2.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] </math>
		<math>~ + 5.612452~ \xi^{- 2.1937742}\biggl[ - 4.4180043~ \xi^{3} + 2.5283016~\xi^{6} \biggr] + \xi^{- 2.1937742}\biggl[ -8.8360086~ \xi^3 + 12.641508~\xi^{6} \biggr] </math>
	<math>~=</math>	<math>~ \xi^{- 2.1937742}\biggl[ -63.67471 + 93.77171~ \xi^{3} -26.83148~\xi^{6} \biggr] </math>
		<math>~ + ~ \xi^{- 2.1937742}\biggl[ - 24.79584~ \xi^{3} + 14.18997~\xi^{6} \biggr] + \xi^{- 2.1937742}\biggl[ -8.8360086~ \xi^3 + 12.641508~\xi^{6} \biggr] </math>
	<math>~=</math>	<math>~ \xi^{- 2.1937742}\biggl[ -63.67471 + (93.77171-24.79584- 8.8360086) ~ \xi^{3} + (-26.83148+14.18997 + 12.641508)~\xi^{6} \biggr] </math>
	<math>~=</math>	<math>~ \xi^{- 2.1937742}\biggl[ -63.67471 + 60.13986 ~ \xi^{3} \biggr] </math>
<math>~\Rightarrow~~~ a(q^3\xi^3) \biggl\{ \frac{d^2x}{d\xi^2} + \frac{6}{\xi} \cdot \frac{dx}{d\xi} -\mathfrak{F}_\mathrm{env} \biggl(\frac{x}{\xi^2} \biggr) \biggr\} </math>	<math>~=</math>	<math>~ \xi^{- 2.1937742}\biggl[ -20.37783 +19.24657 ~ \xi^{6} \biggr] \, . </math>

But these two sub-expressions cancel precisely, which means that our eigenfunction satisfies the LAWE! Q.E.D.

Boundary Conditions

Notice that for this particular eigenfunction solution, the value and first radial derivative at the center <math>~(\xi=0)</math> of the configuration is,

<math>~x_\mathrm{core}</math>

<math>~-17.326820 + 18.326820~\cancelto{0}{\xi^2} = -17.326820 \, ;</math>

and,

<math>~\frac{dx_\mathrm{core}}{d\xi}</math>

<math>~36.65364~\cancelto{0}{\xi} = 0 \, .</math>

And, at the surface <math>~(\xi = q^{-1}) </math> the value and first radial derivative are,

<math>~a \cdot x_\mathrm{env}</math>	<math>~=</math>	<math>~ \biggl\{\xi^{- 0.1937742}\biggl[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} \biggr] \biggr\}_{\xi=1/q} </math>
	<math>~\approx</math>	<math>~ 0.47627246\, , </math>

where,

<math>~a \approx - 0.05128445 \, ;</math>

and,

<math>~\frac{d\ln x_\mathrm{env}}{d\ln \xi} </math>	<math>~=</math>	<math>~ \frac{\xi}{a\cdot x_\mathrm{env}} \biggl[ a\cdot \frac{dx_\mathrm{env}}{d\xi} \biggr]</math>
	<math>~=</math>	<math>~ \frac{ -0.1937742~\xi^{- 0.1937742}[ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} ] + \xi^{- 0.1937742}[ - 4.4180043~ \xi^{3} + 2.5283016~\xi^{6} ] }{\xi^{- 0.1937742} [ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} ] } </math>
	<math>~=</math>	<math>~ -0.1937742 + \frac{~ [ - 4.4180043~ \xi^{3} + 2.5283016~\xi^{6} ] }{ [ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} ] } </math>
<math>~\Rightarrow ~~~ \biggl\{ \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr\}_{\xi=1/q} </math>	<math>~=</math>	<math>~ -0.1937742 + \biggl\{ \frac{[ - 4.4180043~ \xi^{3} + 2.5283016~\xi^{6} ] }{ [ 1 - 1.4726681~ \xi^{3} + 0.4213836~\xi^{6} ] } \biggr\}_{\xi=1/q} </math>
	<math>~=</math>	<math>~ -0.1937742 + 21.22492 = 21.03115 \, . </math>

Finite-Difference Representation

General Approach

Working with the Taylor series expansion, we can write,

<math>~\approx</math>

<math>~ x(a) + (\xi - a) x_a' + \tfrac{1}{2} (\xi-a)^2 x_a \, , </math>

and letting <math>~\xi_\pm = a \pm \Delta </math>, we have,

<math>~\approx</math>

<math>~ x(a) + \Delta \cdot x_a' + \tfrac{1}{2} \Delta^2 x_a \, , </math>

and,

<math>~\approx</math>

<math>~ x(a) - \Delta \cdot x_a' + \tfrac{1}{2} \Delta^2 x_a \, . </math>

Subtracting the second of these two expressions from the first gives,

<math>~x_+ - x_-</math>	<math>~\approx</math>	<math>~ 2 \Delta \cdot x_a' </math>
<math>~\Rightarrow ~~~ x_a'</math>	<math>~\approx</math>	<math>~ \frac{x_+ - x_-}{2 \Delta} \, ; </math>

while, adding the two expressions together gives,

<math>~\frac{x_+ - 2x_a + x_-}{\Delta^2}</math>

<math>~\approx</math>

Integrating Outward Through the Core

From the LAWE for the core, we have,

<math>~ - ( 4g^2 - 6a^2 ) x_a' - a \mathfrak{F}_\mathrm{core} x_a \, . </math>

So, putting these last three expressions together gives an approximate relation between <math>~x_+</math> and the previous two values of the function, <math>~x_-</math> and <math>~x_a</math>, namely,

<math>~a (g^2 - a^2) \biggl[ \frac{x_+ - 2x_a + x_-}{\Delta^2} \biggr]</math>	<math>~\approx</math>	<math>~ - ( 4g^2 - 6a^2 ) \biggl[\frac{x_+ - x_-}{2 \Delta} \biggr] - a \mathfrak{F}_\mathrm{core} x_a </math>
<math>~\Rightarrow~~~ a (g^2 - a^2) \biggl[ \frac{x_+ }{\Delta^2} \biggr] + ( 4g^2 - 6a^2 ) \biggl[\frac{x_+ }{2 \Delta} \biggr] </math>	<math>~\approx</math>	<math>~a (g^2 - a^2) \biggl[ \frac{2x_a - x_-}{\Delta^2} \biggr] + ( 4g^2 - 6a^2 ) \biggl[\frac{x_-}{2 \Delta} \biggr] - a \mathfrak{F}_\mathrm{core} x_a </math>
<math>~ \Rightarrow~~~x_+[2a (g^2 - a^2) + \Delta( 4g^2 - 6a^2 ) ] </math>	<math>~\approx</math>	<math>~2a (g^2 - a^2) [ 2x_a - x_- ] + ( 4g^2 - 6a^2 ) [\Delta x_- ] - 2\Delta^2 a \mathfrak{F}_\mathrm{core} x_a </math>
	<math>~\approx</math>	<math>~[4a (g^2 - a^2) - 2\Delta^2 a \mathfrak{F}_\mathrm{core} ]x_a + [ \Delta( 4g^2 - 6a^2 ) - 2a (g^2 - a^2)] x_- \, . </math>

Now, at the very center of the configuration, <math>~(a = 0)</math>, we expect the function, <math>~x(\xi)</math>, to be symmetric; that is, we expect <math>~x_- = x_+</math>. So for this case alone, we have,

<math>~ x_+[2a (g^2 - a^2) + \Delta( 4g^2 - 6a^2 ) - \Delta( 4g^2 - 6a^2 ) + 2a (g^2 - a^2)] </math>	<math>~=</math>	<math>~[4a (g^2 - a^2) - 2\Delta^2 a \mathfrak{F}_\mathrm{core} ]x_a </math>
<math>~\Rightarrow~~~ x_+[2(g^2 - \cancelto{0}{a^2}) + 2(g^2 - \cancelto{0}{a^2})] </math>	<math>~=</math>	<math>~[4 (g^2 - \cancelto{0}{a^2}) - 2\Delta^2 \mathfrak{F}_\mathrm{core} ]x_a </math>
<math>~\Rightarrow~~~ x_+ </math>	<math>~=</math>	<math>~\biggl[ 1 - \frac{\Delta^2 \mathfrak{F}_\mathrm{core}}{2g^2} \biggr]x_a \, . </math>

For all other coordinate locations, <math>~a = \xi</math>, in the range, <math>~0 < \xi < 1</math>, we will use the general expression, namely,

<math>~ \Rightarrow~~~x_+ </math>

<math>~\approx</math>

<math>~\frac{[4a (g^2 - a^2) - 2\Delta^2 a \mathfrak{F}_\mathrm{core} ]x_a + [ \Delta( 4g^2 - 6a^2 ) - 2a (g^2 - a^2)] x_- }{[2a (g^2 - a^2) + \Delta( 4g^2 - 6a^2 ) ] } \, . </math>

Keep in mind that, when we move across the interface at <math>~a = 1</math>, we want both the value of the function, <math>~x_q</math>, and its first derivative, <math>~x_q'</math>, to be the same as viewed from both the envelope and the core. In a numerical integration algorithm, it will be very straightforward to set the value of the eigenfunction at the interface. In order to properly handle the first derivative, I propose that we extend the core solution and evaluate the eigenfunction at one zone beyond the interface, and identify the values of the eigenfunction that straddles the interface as,

<math>~(x_-)_q</math> and <math>~(x_+)_q</math>.

Then define the slope of the eigenfunction at the interface by the expression,

Slope at the Interface

<math>~\equiv</math>

<math>~\frac{(x_+)_q - (x_-)_q}{2\Delta} \, .</math>

Integrating Outward Through the Envelope

From the LAWE for the envelope, we have,

<math>~ - ( 3 - 6q^3 a^3 ) a x_a' - [ q^3 \mathfrak{F}_\mathrm{env} a^3 -\alpha_e ]x_a \, . </math>

Inserting the same finite-difference expressions for the first and second derivatives, we therefore have,

<math>~a^2( 1 - q^3 a^3 ) \biggl[ \frac{x_+ - 2x_a + x_-}{\Delta^2} \biggr] </math>	<math>~=</math>	<math>~ - ( 3 - 6q^3 a^3 ) a \biggl[ \frac{x_+ - x_-}{2 \Delta} \biggr] - [ q^3 \mathfrak{F}_\mathrm{env} a^3 -\alpha_e ]x_a </math>
<math>~\Rightarrow ~~~ a^2( 1 - q^3 a^3 ) \biggl[ \frac{x_+ }{\Delta^2} \biggr] + ( 3 - 6q^3 a^3 ) a \biggl[ \frac{x_+ }{2 \Delta} \biggr] </math>	<math>~=</math>	<math>~ ( 3 - 6q^3 a^3 ) a \biggl[ \frac{x_-}{2 \Delta} \biggr] - a^2( 1 - q^3 a^3 ) \biggl[ \frac{x_- - 2x_a }{\Delta^2} \biggr] - [ q^3 \mathfrak{F}_\mathrm{env} a^3 -\alpha_e ]x_a </math>
<math>~\Rightarrow ~~~ x_+ [2a^2( 1 - q^3 a^3 ) + \Delta ( 3 - 6q^3 a^3 ) a ] </math>	<math>~=</math>	<math>~ [ \Delta ( 3 - 6q^3 a^3 ) a - 2a^2( 1 - q^3 a^3 ) ] x_- + [4a^2( 1 - q^3 a^3 ) - 2\Delta^2 ( q^3 \mathfrak{F}_\mathrm{env} a^3 -\alpha_e ) ] x_a </math>

Now, at the interface (only), we need to relate <math>~x_-</math> to <math>~x_+</math> in such a way that the slope gives the proper value at the interface. Specifically, we need to set,

<math>~x_+ - 2\Delta (x_q') \, ,</math>

where, <math>~x_q'</math> takes the value that was determined for the core. Hence, at the interface <math>~(a = 1)</math>, the first step into the envelope is special and demands that,

<math>~ x_+ [2a^2( 1 - q^3 a^3 ) + \Delta ( 3 - 6q^3 a^3 ) a ] </math>	<math>~=</math>	<math>~ [ \Delta ( 3 - 6q^3 a^3 ) a - 2a^2( 1 - q^3 a^3 ) ] [x_+ - 2\Delta (x_q')] + [4a^2( 1 - q^3 a^3 ) - 2\Delta^2 ( q^3 \mathfrak{F}_\mathrm{env} a^3 -\alpha_e ) ] x_a </math>
<math>~\Rightarrow ~~~ x_+ [2a^2( 1 - q^3 a^3 ) + \Delta ( 3 - 6q^3 a^3 ) a -\Delta ( 3 - 6q^3 a^3 ) a + 2a^2( 1 - q^3 a^3 ) ] </math>	<math>~=</math>	<math>~ [ \Delta ( 3 - 6q^3 a^3 ) a - 2a^2( 1 - q^3 a^3 ) ] [- 2\Delta (x_q')] + [4a^2( 1 - q^3 a^3 ) - 2\Delta^2 ( q^3 \mathfrak{F}_\mathrm{env} a^3 -\alpha_e ) ] x_a </math>
<math>~\Rightarrow ~~~ x_+ [4a^2( 1 - q^3 a^3 ) ] </math>	<math>~=</math>	<math>~ -2\Delta [ \Delta ( 3 - 6q^3 a^3 ) a - 2a^2( 1 - q^3 a^3 ) ] x_q' + [4a^2( 1 - q^3 a^3 ) - 2\Delta^2 ( q^3 \mathfrak{F}_\mathrm{env} a^3 -\alpha_e ) ] x_a </math>
and, setting, <math>~a = 1 ~~~~\Rightarrow ~~~ x_+ </math>	<math>~=</math>	<math>~ \frac{ 2\Delta [2( 1 - q^3 ) - \Delta ( 3 - 6q^3 ) ] x_q' + [4( 1 - q^3 ) - 2\Delta^2 ( q^3 \mathfrak{F}_\mathrm{env} -\alpha_e ) ] x_a }{ 4( 1 - q^3 ) } \, . </math>

Varying the Oscillation Frequency

Approach

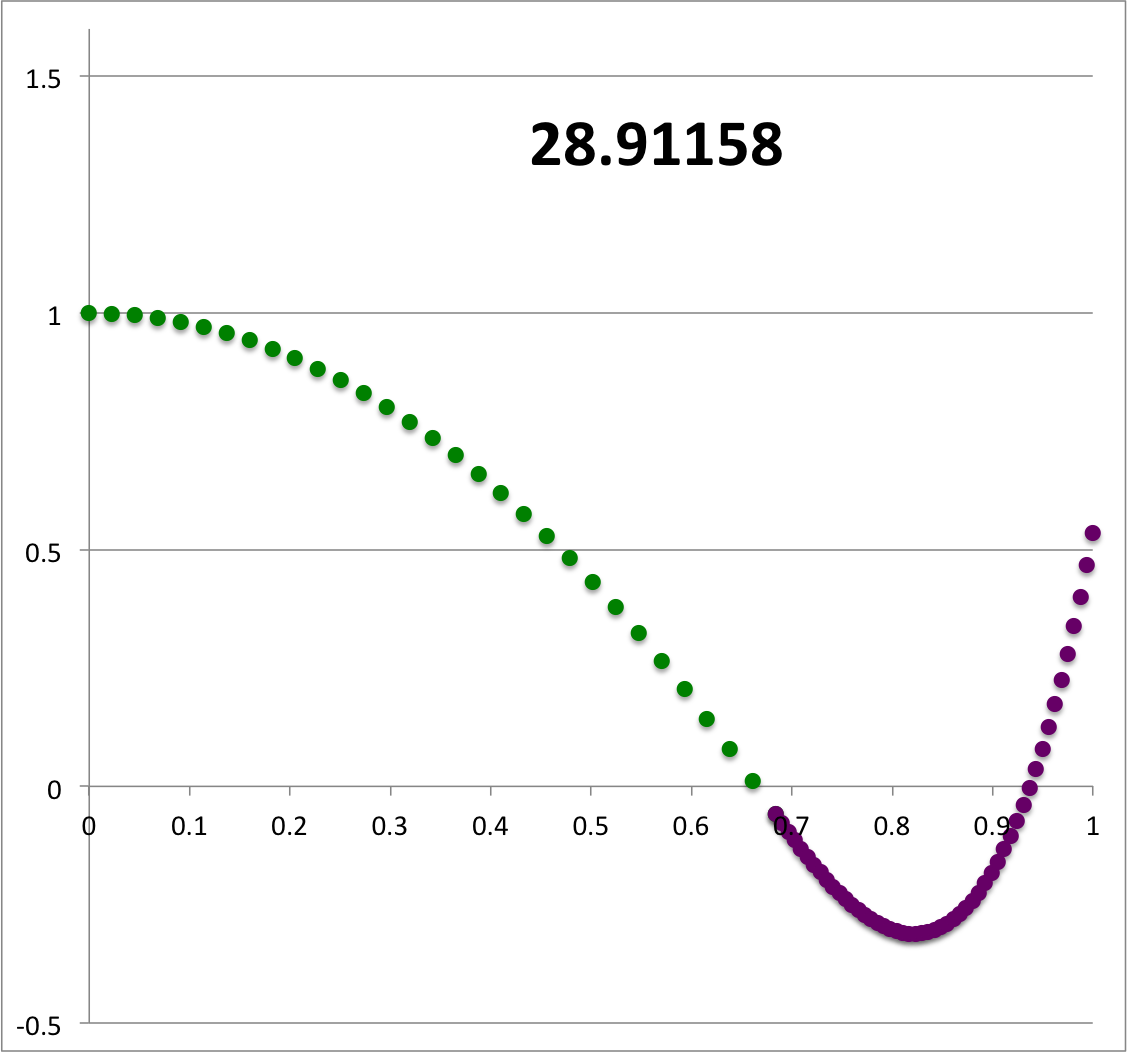
First, we fix <math>~q</math>, <math>~\gamma_e</math>, and <math>~\gamma_c</math>; in the example, here (as above) we choose: <math>~(q,\gamma_e,\gamma_c) = ( 0.6840119, 1.1940299, 1.845579)</math>. For this example, we will also retain the constraint, <math>~g^2 = \mathcal{B}</math>, in which case,

<math>~\frac{\rho_e}{\rho_c} = \frac{2q^3}{1+2q^3}</math>	<math>~\approx</math>	<math>~0.3902664</math>
<math>~\sigma_c^2 \equiv \frac{3\omega^2}{2\pi G \rho_c} = 20\gamma_c - 8 </math>	<math>~\approx</math>	<math>~28.91158 \, .</math>

Next, we pick various values of the (square of the) dimensionless oscillation frequency, <math>~\sigma_c^2</math>, and from each value we set,

<math>~\mathfrak{F}_\mathrm{core} </math>	<math>~=</math>	<math>~\frac{\sigma_c^2 + 8}{\gamma_c} - 6 \, ,</math>
<math>~\mathfrak{F}_\mathrm{env} </math>	<math>~=</math>	<math>~\frac{1}{\gamma_e} \biggl[ \sigma_c^2 \biggl(\frac{\rho_e}{\rho_c} \biggr)^{-1} + 8\biggr]- 6 \, .</math>

For the finite-difference algorithm, we divide the core — radial coordinate range, <math>~0 \le \xi \le 1</math> — into N_core zones, and the envelope — radial coordinate range, <math>~1\le \xi \le 1/q</math> — into N_env zones. This means that the spacing between successive radial zones in the core and envelope is, respectively,

<math>~\Delta_c \equiv \frac{1}{\mathrm{N}_\mathrm{core}}</math>

and

<math>~\Delta_e \equiv \frac{q^{-1} - 1}{\mathrm{N}_\mathrm{env}} \, .</math>

Starting at the center of the configuration <math>~(\xi = 0)</math>, where we arbitrarily set the value of the eigenfuntion to <math>~x_0 = 1</math>, the value of the eigenfunction at the first grid point away from the center <math>~(\xi = \Delta_c)</math> is,

<math>~\biggl[ 1 - \frac{\Delta_c^2 \mathfrak{F}_\mathrm{core}}{2g^2} \biggr]x_0 \, . </math>

Thereafter — moving out toward and just beyond the interface location <math>~(\xi = 1/q)</math>, the radial coordinate of each successive grid point is <math>~\xi_k = k\Delta_c</math>, and the numerically determined value of the eigenfunction at each successive grid point <math>~(k = 1 \rightarrow \mathrm{N}_\mathrm{core})</math> is,

<math>~\approx</math>

<math>~\frac{[4\xi_k (g^2 - \xi_k^2) - 2\Delta_c^2 \xi_k \mathfrak{F}_\mathrm{core} ]x_k + [ \Delta_c( 4g^2 - 6\xi_k^2 ) - 2\xi_k (g^2 - \xi_k^2)] x_{k-1} }{[2a (g^2 - \xi_k^2) + \Delta_c( 4g^2 - 6\xi_k^2 ) ] } \, . </math>

Then, at the interface, which is associated with <math>~k = \mathrm{N}_\mathrm{core}</math>, we define the reference slope as,

<math>~\frac{x_{k+1} - x_{k-1}}{2\Delta_c} \, .</math>

Next, we move outward into the envelope, using the integer index, <math>~n = 1 \rightarrow \mathrm{N}_\mathrm{env}</math>, to label successive radial grid locations <math>~(\xi_n = 1 + n\Delta_e)</math>. Letting the value of the eigenfunction at the interface be represented by <math>~x_q</math>, at the first grid location outside the interface <math>~(\xi = 1 + \Delta_e)</math>, the value of the eigenfunction is,

<math>~ \frac{ 2\Delta_e [2( 1 - q^3 ) - \Delta_e ( 3 - 6q^3 ) ] x_q' + [4( 1 - q^3 ) - 2\Delta_e^2 ( q^3 \mathfrak{F}_\mathrm{env} -\alpha_e ) ] x_q }{ 4( 1 - q^3 ) } \, . </math>

Thereafter, moving outward through the envelope to the surface, the value of the eigenfunction at each successive grid location is,

<math>~ \frac{[ \Delta_e ( 3 - 6q^3 \xi_n^3 ) \xi_n - 2\xi_n^2( 1 - q^3 \xi_n^3 ) ] x_{n-1} + [4\xi_n^2( 1 - q^3 \xi_n^3 ) - 2\Delta_e^2 ( q^3 \mathfrak{F}_\mathrm{env} \xi_n^3 -\alpha_e ) ] x_{n} }{ [2\xi_n^2( 1 - q^3 \xi_n^3 ) + \Delta_e ( 3 - 6q^3 \xi_n^3 ) \xi_n ] } \, . </math>

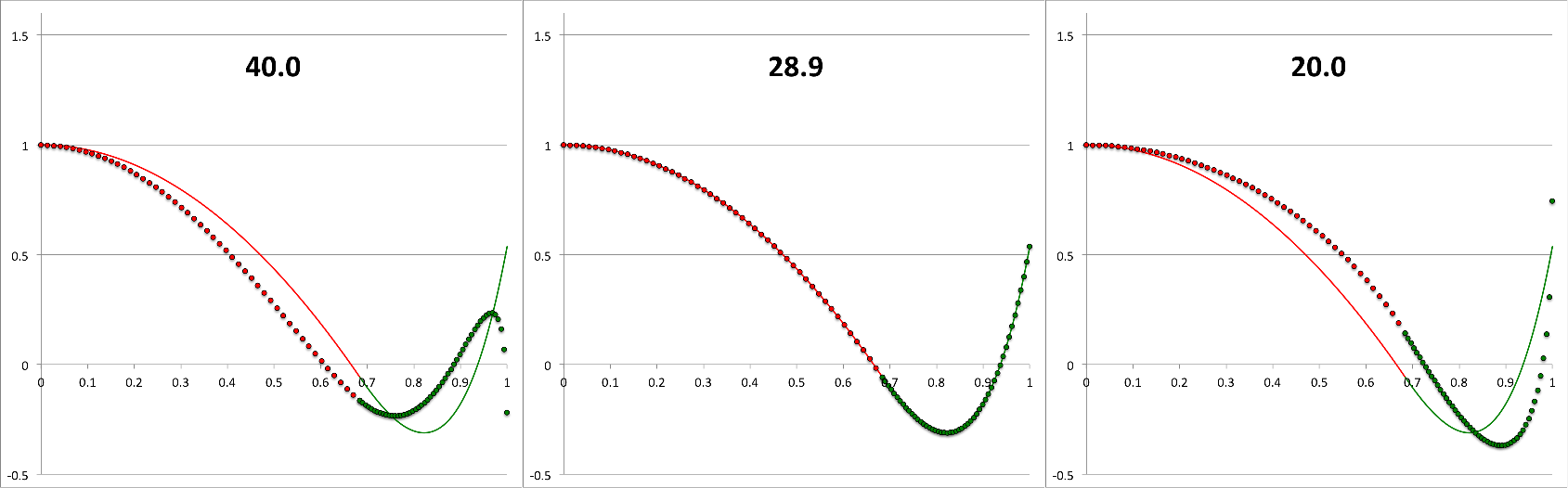
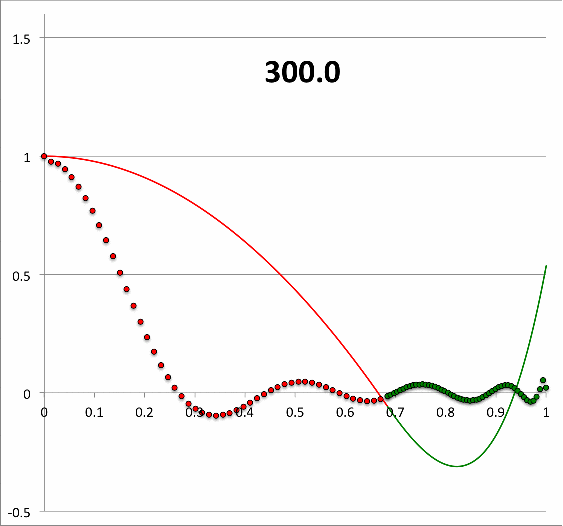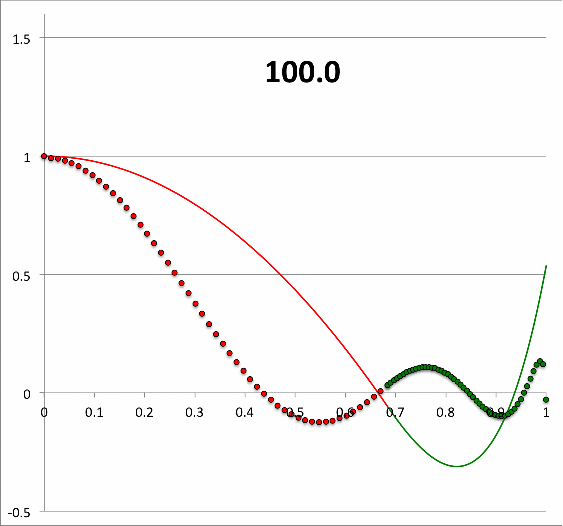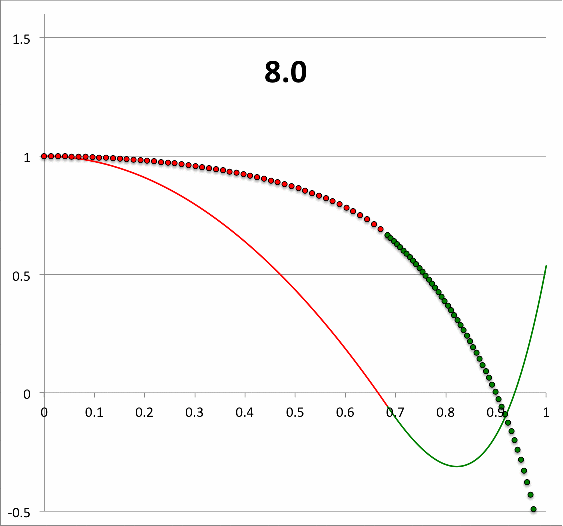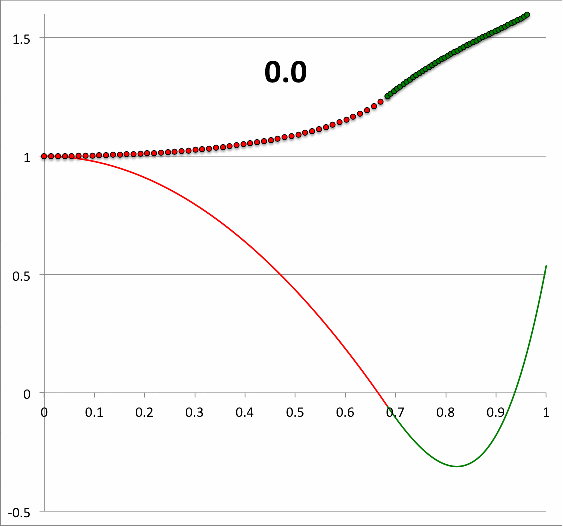
Results

User:Tohline/Appendix/Ramblings/NumericallyDeterminedEigenvectors

Contents

Numerically Determined Eigenvectors of a Zero-Zero Bipolytrope

Setup

Initial Focus

Properties of 21Analytic Solution

Demonstrate Core Solution

Demonstrate Envelope Solution

Boundary Conditions

Finite-Difference Representation

General Approach

Integrating Outward Through the Core

Integrating Outward Through the Envelope

Varying the Oscillation Frequency

Approach

Results

Navigation menu

Search