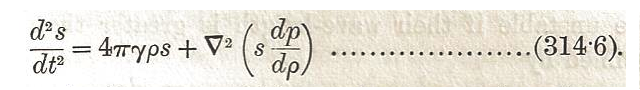User:Tohline/SSC/Stability Eulerian Perspective
Stability of Spherically Symmetric Configurations (Eulerian Perspective)

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
A standard technique that is used throughout astrophysics to test the stability of self-gravitating fluids involves perturbing physical variables away from their initial (usually equilibrium) values then linearizing each of the principal governing equations before seeking solutions describing the time-dependent behavior of the variables that simultaneously satisfy all of the equations. When the effects of the fluid's self gravity are ignored and this analysis technique is applied to an initially homogeneous medium, the combined set of linearized governing equations generates a wave equation that governs the propagation of sound waves. Here we build on our separate, introductory discussion of sound waves and apply standard perturbation & linearization techniques to spherically symmetric, inhomogeneous and self-gravitating fluids. We will assume that the reader has read this separate introductory discussion and, in particular, understands how the linear wave equation that governs the propagation of sound waves is derived from the set of nonlinear, principal governing equations.
Assembling the Key Relations
Governing Equations and Supplemental Relations
We begin with the set of principal governing equations that provides the foundation for all of our discussions in this H_Book, namely, the
Eulerian Representation
of the Continuity Equation,
<math>~\frac{\partial\rho}{\partial t} + \nabla \cdot (\rho \vec{v}) = 0</math>
Eulerian Representation
of the Euler Equation,
<math>~\frac{\partial\vec{v}}{\partial t} + (\vec{v}\cdot \nabla) \vec{v}= - \frac{1}{\rho} \nabla P - \nabla \Phi</math>
Adiabatic Form of the
First Law of Thermodynamics
<math>~\frac{d\epsilon}{dt} + P \frac{d}{dt} \biggl(\frac{1}{\rho}\biggr) = 0</math> .
Poisson Equation
|
<math>\nabla^2 \Phi = 4\pi G \rho</math> |
As was done in our separate, introductory discussion of sound waves, we will assume that we are dealing with an ideal gas and supplement this set of equations with a barotropic (polytropic) equation of state,
<math>~P = K\rho^{\gamma_\mathrm{g}}</math> … with … <math>\gamma_\mathrm{g} \equiv \frac{d\ln P_0}{d\ln \rho_0} = \frac{\rho_0}{P_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \, ,</math>
which will ensure that the adiabatic form of the first law of thermodynamics is satisfied. When we develop the linearized Euler equation, below, it will be useful to recognize that, assuming <math>~\gamma_\mathrm{g}</math> is uniform throughout the fluid, we can rewrite this last expression as,
|
<math>~\frac{\nabla P_0}{P_0}</math> |
<math>~=</math> |
<math>~\biggl[\frac{\rho_0}{P_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] \frac{\nabla \rho_0}{\rho_0}</math> |
|
<math>~\Rightarrow ~~~ \nabla P_0</math> |
<math>~=</math> |
<math>~\biggl( \frac{dP}{d\rho} \biggr)_0 \nabla \rho_0 \, .</math> |
Perturbation then Linearization of Equations
In this Eulerian analysis, we are investigating how conditions vary with time at a fixed point in space, <math>~\vec{r}</math>. By analogy with our separate introductory analysis of sound waves, we will write the four primary variables in the form,
|
<math>~\rho</math> |
<math>~=</math> |
<math>~\rho_0(\vec{r}) + \rho_1(\vec{r},t) \, ,</math> |
|
<math>~\vec{v}</math> |
<math>~=</math> |
<math>~\cancelto{0}{\vec{v}_0} + \vec{v}_1(\vec{r},t) = \vec{v}(\vec{r},t) \, ,</math> |
|
<math>~P</math> |
<math>~=</math> |
<math>~P_0(\vec{r}) + P_1(\vec{r},t) \, ,</math> |
|
<math>~\Phi</math> |
<math>~=</math> |
<math>~\Phi_0(\vec{r}) + \Phi_1(\vec{r},t) \, ,</math> |
where quantities with subscript "0" are initial values — independent of time, but not necessarily spatially uniform, and usually specified via some choice of an initial equilibrium configuration — and quantities with subscript "1" denote variations away from the initial state, which are assumed to be small in amplitude — for example, <math>~|\rho_1/\rho_0 | \ll 1</math> and <math>~| P_1/P_0 | \ll 1</math>. As indicated, we will assume that the fluid configuration is initially stationary <math>~(\mathrm{i.e.,}~\vec{v}_0 = 0)</math> and, for simplicity, will not append the subscript "1" to the velocity perturbation. It is to be understood, however, that the velocity, <math>~\vec{v}</math>, is small also where, ultimately, this will mean <math>~|\vec{v}| \ll c_s</math>. In what follows, by definition, <math>~P_1</math>, <math>~\rho_1</math>, <math>~\Phi_1</math>, and <math>~\vec{v}</math> are considered to be of first order in smallness, while products of these quantities are of second (or even higher) order in smallness.
Continuity Equation
Substituting the expression for <math>~\rho</math> into the lefthand side of the continuity equation and neglecting small quantities of the second order, we have,
|
<math>~~\frac{\partial}{\partial t} (\rho_0 + \rho_1) + \nabla\cdot [(\rho_0 + \rho_1)\vec{v}]</math> |
<math>~=</math> |
<math>~ \cancelto{0}{\frac{\partial \rho_0}{\partial t}} + \frac{\partial \rho_1}{\partial t} + \nabla\cdot (\rho_0 \vec{v}) + \nabla\cdot\cancelto{\mathrm{small}}{(\rho_1\vec{v} )} </math> |
|
|
<math>~\approx</math> |
<math>~ \frac{\partial \rho_1}{\partial t} + \rho_0\nabla\cdot \vec{v} + \vec{v}\cdot \nabla\rho_0 \, , </math> |
where, in the first line, the first term on the righthand side has been set to zero because <math>~\rho_0</math> is independent of time. Hence, we have the desired,
Linearized Continuity Equation
|
<math>~ \frac{\partial \rho_1}{\partial t} + \rho_0\nabla\cdot \vec{v} + \vec{v}\cdot \nabla\rho_0 </math> |
<math>~=</math> |
<math>~0 \, .</math> |
Euler Equation
Next, we turn to the Euler equation and note that the term,
|
<math>(\vec{v} \cdot \nabla)\vec{v} \, ,</math> |
may be altogether neglected because it is of second order in smallness. Substituting the expressions for <math>~\rho</math>, <math>~P</math>, and <math>~\Phi</math> into the righthand side of the Euler equation and neglecting small quantities of the second order, we have,
|
<math>~- \frac{1}{(\rho_0 + \rho_1)} \nabla (P_0 + P_1) - \nabla(\Phi_0 + \Phi_1)</math> |
<math>~=</math> |
<math>~- \frac{1}{\rho_0} \biggl( 1 + \frac{\rho_1}{\rho_0} \biggr)^{-1} \biggl[ \nabla P_0 + \nabla P_1\biggr] - \nabla(\Phi_0 + \Phi_1) </math> |
|
|
<math>~=</math> |
<math>~- \frac{1}{\rho_0} \biggl[ 1 - \frac{\rho_1}{\rho_0} + \cancelto{\mathrm{small}}{\biggl(\frac{\rho_1}{\rho_0} \biggr)^2} + \cdots \biggr] \biggl[ \nabla P_0 + \nabla P_1\biggr] - \nabla(\Phi_0 + \Phi_1) </math> |
|
|
<math>~=</math> |
<math>~ - \cancelto{0}{\biggl[ \frac{1}{\rho_0} \nabla P_0 + \nabla \Phi_0 \biggr]} -\frac{1}{\rho_0} \nabla P_1 - \nabla\Phi_1 + \frac{\rho_1}{\rho_0^2} \nabla P_0 + \frac{1}{\rho_0^2} \cancelto{\mathrm{small}}{(\rho_1 \nabla P_1)} \, , </math> |
where, the binomial theorem has been used to obtain the expression on the righthand side of the second line and, in the last line, the sum of the first pair of terms has been set to zero because the initial configuration is assumed to be in equilibrium. Combining these simplification steps, we have the,
Linearized Euler Equation
|
<math>~ \frac{\partial \vec{v}}{\partial t} </math> |
<math>~=</math> |
<math>~ - \frac{1}{\rho_0} \nabla P_1 + \frac{\rho_1}{\rho_0^2} \nabla P_0 - \nabla\Phi_1 \, . </math> |
First Law of Thermodynamics
In a similar fashion, perturbing the variables in the barotropic equation of state leads to,
|
<math>~ P_0 + P_1 </math> |
<math>~=</math> |
<math>~ K (\rho_0 + \rho_1)^{\gamma_\mathrm{g}} </math> |
|
|
<math>~=</math> |
<math>~ K\rho_0^{\gamma_\mathrm{g}} \biggl(1 + \frac{\rho_1}{\rho_0} \biggr)^{\gamma_\mathrm{g}} </math> |
|
<math>~\Rightarrow~~~ P_1</math> |
<math>~=</math> |
<math>~ K\rho_0^{\gamma_\mathrm{g}} \biggl(1 + \frac{\rho_1}{\rho_0} \biggr)^{\gamma_\mathrm{g}} - K\rho_0^{\gamma_\mathrm{g}} </math> |
|
|
<math>~=</math> |
<math>~ K\rho_0^{\gamma_\mathrm{g}} \biggl[1 + \gamma_\mathrm{g}\biggl(\frac{\rho_1}{\rho_0} \biggr) + \frac{\gamma_\mathrm{g}(\gamma_\mathrm{g}-1)}{2} \cancelto{\mathrm{small}}{\biggl(\frac{\rho_1}{\rho_0} \biggr)^2} + \cdots \biggr] - K\rho_0^{\gamma_\mathrm{g}} </math> |
|
|
<math>~\approx</math> |
<math>~ \gamma_\mathrm{g} \biggl( \frac{P_0}{\rho_0} \biggr) \rho_1 \, . </math> |
Hence, as in our separate introductory discussion of sound waves, we have the,
Linearized Equation of State
|
<math>~P_1</math> |
<math>~=</math> |
<math>~ \biggl( \frac{dP}{d\rho} \biggr)_0 \rho_1\, . </math> |
Poisson Equation
Finally, plugging the "perturbed" expressions for <math>~\Phi</math> and <math>~\rho</math> into the Poisson equation — which, by its very nature, is a linear equation — we have,
|
<math>~\nabla^2 (\Phi_0 + \Phi_1)</math> |
<math>~=</math> |
<math>~ 4\pi G (\rho_0 + \rho_1) </math> |
|
<math>~\Rightarrow ~~~\biggl[ \cancelto{0}{\nabla^2 \Phi_0 - 4\pi G \rho_0} \biggr]</math> |
<math>~=</math> |
<math>~ \nabla^2 \Phi_1 - 4\pi G \rho_1 \, , </math> |
where, in the second line, the sum of the pair of terms on the lefthand side has been set to zero because it is a self-contained representation of the Poisson equation for the initial unperturbed medium. This gives us the desired,
Linearized Poisson Equation
|
<math>~\nabla^2 \Phi_1</math> |
<math>~=</math> |
<math>~ 4\pi G \rho_1 \, . </math> |
Summary and Combinations
In summary, the following four linearized equations govern the time-dependent physical relationship between the four perturbation amplitudes <math>~P_1(\vec{r},t)</math>, <math>~\rho_1(\vec{r},t)</math>, <math>~\Phi_1(\vec{r},t)</math> and <math>~\vec{v}(\vec{r},t)</math> in self-gravitating fluids:
|
Linearized Linearized Linearized <math> P_1 = \biggl( \frac{dP}{d\rho} \biggr)_0 \rho_1\, , </math> Linearized <math> \nabla^2 \Phi_1 = 4\pi G \rho_1\, . </math> |
This set of linearized governing equations is more general than the set of equations that has traditionally been used to describe the propagation of sound waves. The equations governing sound-wave propagation can be retrieved by choosing an initially homogeneous medium — in which case, <math>~\nabla\rho_0 = 0</math> and <math>~\nabla P_0 = 0</math> — and by ignoring the fluid's self gravity, that is, by setting <math>~\Phi_1 = 0</math>.
As is explicitly demonstrated in our discussion of Bonnor's (1957) research, below, the linearized Euler equation can be combined with the linearized adiabatic form of the first law of thermodynamics to give,
|
<math>~\frac{\partial \vec{v}}{\partial t} </math> |
<math>~=</math> |
<math>~- \nabla\Phi_1 - \nabla\biggl[\frac{\rho_1}{\rho_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] \, . </math> |
Taking the divergence of this relation, we have,
|
<math>~\frac{\partial }{\partial t} \nabla\cdot \vec{v}</math> |
<math>~=</math> |
<math>~- \nabla^2 \Phi_1 - \nabla^2\biggl[\frac{\rho_1}{\rho_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] </math> |
|
|
<math>~=</math> |
<math>~- 4\pi G \rho_1 - \nabla^2\biggl[\frac{\rho_1}{\rho_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] \, , </math> |
where, in order to obtain this last expression, we have used the linearized Poisson equation to replace <math>~\Phi_1</math> in favor of <math>~\rho_1</math>. Alternatively, taking the time derivative of the linearized continuity equation gives,
|
<math>~\frac{\partial^2 \rho_1}{\partial t^2} + \rho_0 \frac{\partial}{\partial t}\nabla\cdot \vec{v} + \nabla\rho_0 \cdot \frac{\partial \vec{v}}{\partial t} </math> |
<math>~=</math> |
<math>~0 </math> |
|
<math>~\Rightarrow ~~~ \frac{\partial}{\partial t}\nabla\cdot \vec{v} </math> |
<math>~=</math> |
<math>~ - \frac{\partial^2 }{\partial t^2}\biggl(\frac{\rho_1}{\rho_0}\biggr) - \frac{\nabla\rho_0}{\rho_0} \cdot \frac{\partial \vec{v}}{\partial t} \, . </math> |
Combining these last two expressions, then, gives us a
| ||||||
that describes the time-variation at any point in space of the fractional density fluctuation,
<math>s \equiv \frac{\rho_1}{\rho_0} \, ,</math>
in a self-gravitating, barotropic fluid. For purposes of comparison with the wave equation that governs the behavior of sound waves, it is worth rewriting this expression in the form,
|
<math>~ \frac{\partial^2 s }{\partial t^2} - c_s^2 \nabla^2 s </math> |
<math>~=</math> |
<math>4\pi G \rho_0 s - \frac{\nabla\rho_0}{\rho_0} \cdot \frac{\partial \vec{v}}{\partial t} \, ,</math> |
where, as before,
<math> c_s \equiv \sqrt{\biggl( \frac{dP}{d\rho} \biggr)_0 } \, . </math>
Comparison with Classic Research Publications
James Jeans (1902 & 1928)
James H. Jeans (1902, Philosophical Transactions of the royal Society of London. Series A, 199, 1) used precisely this type of perturbation and linearization analysis when he first derived what is now commonly referred to as the Jeans Instability. For example, if our discussion is restricted only to fluctuations in the radial coordinate direction of a spherically symmetric configuration — in which case <math>~\nabla \rightarrow \partial/\partial r</math> and <math>\vec{v} \rightarrow \hat\mathbf{e}_r \cdot \vec{v} = v_r</math> — our expression for the linearized Euler equation exactly matches equation (12) from Jeans (1902), which, for purposes of illustration, is displayed in the following framed image.
|
Linearized Euler Equation as Derived and Presented by Jeans (1902) |
||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
The correspondence between the righthand-sides of equation (12) from Jeans (1902) and our derived expression for the linearized Euler equation is clear after accepting the following variable mappings:
The lefthand side of equation (12) from Jeans (1902) also matches the lefthand side of our linearized Euler equation, although this may not be immediately apparent. In the paper by Jeans, <math>~u</math> is not a component of the velocity vector but is, rather, the radial displacement of a fluid element. Hence,
|
A broader discussion of gravitational instability in the context of the formation of "great nebulae" (i.e., galaxies) and stars appears in Chapter XIII — specifically, pp. 337-342 — of the book by Jeans (1928) titled, "Astronomy and Cosmogony." The governing wave equation for self-gravitating fluids that we have derived, above, appears as equation (314.6) in this published discussion by Jeans (1928), although the term on the lefthand side involving <math>~\nabla\rho_0</math> does not appear, presumably because Jeans assumed that the initial, unperturbed medium was homogeneous. In an effort to facilitate comparison with our derived expression, equation (314.6) from Jeans (1928) has been reprinted here as a framed image.
|
Wave Equation for Self-Gravitating Fluids as Derived and Presented by Jeans (1928) |
|---|
|
Note that Jeans (1928) uses <math>~\rho</math>, without the subscript "0", to represent the initial density, and <math>~\gamma</math>, rather than "G", for the Newtonian gravitational constant. |
W. B. Bonnor (1957)
Above, in our opening layout of the governing equations and supplemental relations, we pointed out that,
|
<math>~\nabla P_0</math> |
<math>~=</math> |
<math>~\biggl( \frac{dP}{d\rho} \biggr)_0 \nabla \rho_0 \, .</math> |
If we make this substitution in our linearized Euler equation, and also use the linearized first law of thermodynamics to replace <math>~P_1</math> in favor of <math>~\rho_1</math>, we obtain,
|
<math>~\frac{\partial \vec{v}}{\partial t} </math> |
<math>~=</math> |
<math>~- \nabla\Phi_1 - \frac{1}{\rho_0} \nabla\biggl[ \biggl( \frac{dP}{d\rho} \biggr)_0 \rho_1\biggr] + \frac{\rho_1}{\rho_0^2} \biggl( \frac{dP}{d\rho} \biggr)_0 \nabla \rho_0 </math> |
|
|
<math>~=</math> |
<math>~- \nabla\Phi_1 - \frac{1}{\rho_0} \nabla\biggl[ \biggl( \frac{dP}{d\rho} \biggr)_0 \rho_1\biggr] - \rho_1 \biggl( \frac{dP}{d\rho} \biggr)_0 \nabla \biggl(\frac{1}{\rho_0} \biggr) </math> |
|
|
<math>~=</math> |
<math>~- \nabla\Phi_1 - \nabla\biggl[\frac{\rho_1}{\rho_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] \, . </math> |
This is the version of the linearized Euler equation that was derived by Bonnor (1957, MNRAS, 117, 104) in the section of his paper that addresses the growth of Newtonian, self-gravitating fluctuations on a static (cosmological) background. The two equation images reproduced in the following outlined box document Bonnor's (1957) initial expression (his equation 2.1) for the nonlinear Euler equation and his derived expression (equation 2.7) for the linearized Euler equation. After allowing for the identified variable mappings, Bonnor's two expressions precisely match, respectively, the form of the nonlinear Euler equation that is included among our set of principal governing equations, and this last derived form of our linearized Euler equation.
|
Bonnor's (1957, MNRAS, 117, 104) Derivation |
|||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
|
Original nonlinear Euler Equation |
<math>~\rightarrow</math> |
Linearized Euler Equation |
|||||||||
|
The correspondence between these two equations from Bonnor (1957) and our derived expressions is clear after accepting the following variable mappings:
|
|||||||||||
Bonnor then proceeded to combine the full set of linearized governing equations, in the manner we have detailed above, into a wave equation that is appropriately modified to handle self-gravitating fluids. In an effort to facilitate comparison with our derived expression, Bonnor's (1957) modified wave equation (2.10) has been reprinted here as a framed image.
|
Wave Equation for Self-Gravitating Fluids as Derived and Presented by Bonnor (1957) |
|---|
See Also
- Part II of Spherically Symmetric Configurations: Stability
- Wave Equation
- Sound Waves and Gravitational Instability — class notes provided online by David H. Weinberg (The Ohio State University)
Scratch Work
Material that appears after this point in our presentation is under development and therefore
may contain incorrect mathematical equations and/or physical misinterpretations.
| Go Home |
Part 1
|
<math>~\frac{\partial\vec{v}}{\partial t} + \cancelto{\mathrm{small}}{(\vec{v}\cdot \nabla) \vec{v} }</math> |
<math>~=</math> |
<math>~- \frac{1}{\rho} \nabla P - \nabla \Phi</math> |
|
<math>~g \equiv \frac{d\Phi}{dr}</math> |
<math>~=</math> |
<math>~\frac{GM_r}{r^2}</math> |
|
<math>~\frac{1}{\rho_0}\frac{dP_0}{dr_0}</math> |
<math>~=</math> |
<math>~-g_0</math> |
|
<math>~\frac{dP}{dt}</math> |
<math>~=</math> |
<math>~\gamma_g P \nabla \cdot \vec{v}</math> |
|
<math>~\frac{d\ln P}{d\ln\rho}</math> |
<math>~=</math> |
<math>~\gamma_g</math> |
|
Linearized
<math> P^' = \biggl( \frac{\gamma_g P}{\rho} \biggr)_0 \rho^' </math>
<math>
\nabla^2 \Phi^' = 4\pi G \rho^'
</math>
|
Replace <math>~P^'</math> in favor of <math>~\rho^'</math> in Euler equation.
<math> ~\frac{\partial \vec{v}}{\partial t} = - \nabla\Phi^' - \nabla \biggl[ \frac{\rho^'}{\rho_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] </math>
Take the divergence of this entire expression, then use linearized Poisson equation to replace <math>~\Phi^'</math> in favor of <math>~\rho^'</math>.
<math> ~\frac{\partial }{\partial t}\nabla\cdot \vec{v} = - 4\pi G \rho^' - \nabla^2 \biggl[ \frac{\rho^'}{\rho_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] </math>
Rearrange terms in the linearized equation of continuity, then take the partial time-derivative of the entire expression.
<math>~\frac{\partial }{\partial t}\nabla\cdot \vec{v} = - \frac{\partial^2}{\partial t^2}\biggl(\frac{\rho^'}{\rho_0} \biggr) - \vec{v}\cdot \frac{\nabla\rho_0}{\rho_0} </math>
Subtract the step #2 expression from the step #3 expression.
<math>~\frac{\partial^2}{\partial t^2}\biggl(\frac{\rho^'}{\rho_0} \biggr) + \vec{v}\cdot \frac{\nabla\rho_0}{\rho_0} ~= 4\pi G \rho^' + \nabla^2 \biggl[ \frac{\rho^'}{\rho_0} \biggl( \frac{dP}{d\rho} \biggr)_0 \biggr] ~~~~\Downarrow ~~~~~\Leftarrow </math>
<math>~\frac{d\ln P}{dt} = \gamma_g \frac{d\ln\rho}{dt} ~~~~~\Rightarrow ~~~~~\frac{dP}{dt} = \biggl(\frac{\gamma_g P}{\rho}\biggr) \frac{d\rho}{dt}</math>
If homentropic as well, then,
<math>~\frac{d\ln P}{dr} = \gamma_g \frac{d\ln\rho}{dr} ~~~~~\Leftrightarrow ~~~~~\nabla P = \biggl(\frac{\gamma_g P}{\rho}\biggr) \nabla\rho</math>
<math>~ - \frac{1}{\rho_0} \nabla_r P^' + \frac{1}{\rho_0^2} \biggl[ \rho^' \biggr] \biggl[\nabla_r P_0\biggr] = - \frac{1}{\rho_0} \nabla_r P^' + \frac{1}{\rho_0^2} \biggl[ \biggl( \frac{\rho}{\gamma_g P}\biggr)_0 P^' \biggr] \biggl[\biggl( \frac{\gamma_g P}{\rho} \biggr)_0 \nabla_r \rho_0\biggr] = - \nabla_r \biggl( \frac{P^'}{\rho_0}\biggr) </math>
<math>~= \hat{e}_r f_\sigma \biggl\{ - \nabla_r\Phi^' - \nabla_r \biggl[\frac{\rho^'}{\rho_0} \biggl( \frac{\gamma_g P}{\rho}\biggr)_0 \biggr] \biggr\}</math>
<math>~ \frac{\partial}{\partial t} \nabla \cdot [\hat{e}_r(f_\sigma v_r^')] = - f_\sigma \biggl\{ 4\pi G \rho^' + \nabla^2 \biggl[\frac{\rho^'}{\rho_0} \biggl( \frac{\gamma_g P}{\rho}\biggr)_0 \biggr] \biggr\} </math>
<math>~ \frac{\partial}{\partial t} \nabla \cdot [\hat{e}_r(f_\sigma v_r^')] = - \frac{\partial^2 }{\partial t^2} \biggl(\frac{f_\sigma \rho^'}{\rho_0}\biggr) - \biggl[\frac{\nabla_r \rho_0}{\rho_0} \biggr] \frac{\partial (f_\sigma v_r^') }{\partial t} </math>
<math>~ \frac{\partial^2 }{\partial t^2} \biggl(\frac{f_\sigma \rho^'}{\rho_0}\biggr) + \biggl[\frac{\nabla_r \rho_0}{\rho_0} \biggr] \frac{\partial (f_\sigma v_r^') }{\partial t} = f_\sigma \biggl\{ 4\pi G \rho^' + \nabla^2 \biggl[\frac{\rho^'}{\rho_0} \biggl( \frac{\gamma_g P}{\rho}\biggr)_0 \biggr] \biggr\} </math>
<math>~ \frac{\partial^2 s}{\partial t^2} + \biggl[\frac{\nabla_r \rho_0}{\rho_0} \biggr] \frac{\partial (f_\sigma v_r^') }{\partial t} = 4\pi G \rho_0 s + \nabla^2 \biggl[s \biggl( \frac{\gamma_g P}{\rho}\biggr)_0 \biggr] </math>
where: <math>~s \equiv \biggl(\frac{f_\sigma \rho^'}{\rho_0}\biggr)</math>
Part 2
① ② ③ ④ ⑤ ⑥ ⑦ ⑧ ⑨ ⑩
|
Linearized
<math> P^' = \biggl( \frac{\gamma_g P}{\rho} \biggr)_0 \rho^' </math>
<math>
\rho^' = - \frac{1}{r^2}\frac{\partial}{\partial r} \biggl( r^2 \rho_0 \delta r \biggr)
</math> Euler Equation <math>
~- \sigma^2 \rho_0 \delta r = - \rho_0 \frac{\partial \Phi^'}{\partial r} - \frac{\partial P^'}{\partial r} + \frac{\rho^'}{\rho_0} \frac{\partial P_0}{\partial r}
</math>
<math>
\nabla^2 \Phi^' = 4\pi G \rho^'
</math> |
|
<math>~\rho_0 \frac{\partial \Phi^'}{\partial r}</math> |
<math>~=</math> |
<math>~- (\rho_0 \delta r) 4\pi G \rho_0</math> |
|
|
<math>~=</math> |
<math>~- (\rho_0 \delta r) \biggl[\frac{1}{r^2} \frac{\partial}{\partial r} \biggl(r^2 \frac{\partial\Phi_0}{\partial r}\biggr) \biggr]</math> |
|
|
<math>~=</math> |
<math>~+ (\rho_0 \delta r) \biggl[\frac{1}{r^2} \frac{\partial}{\partial r} \biggl(\frac{r^2}{\rho_0} \frac{\partial P_0}{\partial r}\biggr) \biggr]</math> |
|
|
<math>~=</math> |
<math>~\frac{1}{r^2} \frac{\partial}{\partial r} \biggl[ (r^2 \delta r) \frac{\partial P_0}{\partial r} \biggr] - \frac{1}{\rho_0} \frac{\partial P_0}{\partial r} \frac{\partial (\rho_0 \delta r)}{\partial r}</math> |
|
|
<math>~=</math> |
<math>~\frac{\partial}{\partial r} \biggl[ (\delta r) \frac{\partial P_0}{\partial r} \biggr] + \frac{1}{\rho_0}\frac{\partial P_0}{\partial r} \biggl( \frac{2\rho_0 \delta r}{r} \biggr) - \frac{1}{\rho_0} \frac{\partial P_0}{\partial r} \frac{\partial (\rho_0 \delta r)}{\partial r} \, ;</math> |
|
<math>~- \frac{1}{\rho_0} \frac{\partial P_0}{\partial r} \biggl[ \rho^' \biggr]</math> |
<math>~=</math> |
<math>~+ \frac{1}{\rho_0} \frac{\partial P_0}{\partial r} \biggl[ \frac{1}{r^2} \frac{\partial}{\partial r} \biggl(r^2 \rho_0 \delta r\biggr) \biggr]</math> |
|
|
<math>~=</math> |
<math>~+ \frac{1}{\rho_0} \frac{\partial P_0}{\partial r} \biggl[ \frac{\partial (\rho_0 \delta r )}{\partial r} + \frac{2\rho_0 \delta r}{r} \biggr] \, ;</math> |
|
<math>~\frac{\partial P^'}{\partial r}</math> |
<math>~=</math> |
<math>~ \frac{\partial}{\partial r} \biggl\{ -\gamma_g P_0 \biggl[\frac{1}{r^2} \frac{\partial}{\partial r}(r^2 \delta r) \biggr] \biggr\} - \frac{\partial}{\partial r}\biggl[ (\delta r) \frac{\partial P_0}{\partial r} \biggr] \, .</math> |
<math>~\frac{\partial P}{\partial t} + \vec{v}\cdot \nabla P = \biggl( \frac{\gamma_g P}{\rho}\biggr) \biggl[\frac{\partial \rho}{\partial t} + \vec{v}\cdot \nabla \rho \biggr]</math>
<math>~\frac{\partial (f_\sigma P^')}{\partial t} + f_\sigma v_r^'\nabla_r P_0 = \biggl( \frac{\gamma_g P}{\rho}\biggr)_0 \biggl[\frac{\partial (f_\sigma\rho^')}{\partial t} + f_\sigma v_r^'\nabla_r \rho_0 \biggr]</math>

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |