User:Tohline/Appendix/Ramblings/Dyson1893Part1
Dyson (1893a) Part I: Some Details
This chapter provides some derivation details relevant to our accompanying discussion of Dyson's analysis of the gravitational potential exterior to an anchor ring.

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Overview
In his pioneering work, F. W. Dyson (1893a, Philosophical Transactions of the Royal Society of London. A., 184, 43 - 95) and (1893b, Philosophical Transactions of the Royal Society of London. A., 184, 1041 - 1106) used analytic techniques to determine the approximate equilibrium structure of axisymmetric, uniformly rotating, incompressible tori. C.-Y. Wong (1974, ApJ, 190, 675 - 694) extended Dyson's work, using numerical techniques to obtain more accurate — but still approximate — equilibrium structures for incompressible tori having solid body rotation. Since then, Y. Eriguchi & D. Sugimoto (1981, Progress of Theoretical Physics, 65, 1870 - 1875) and I. Hachisu, J. E. Tohline & Y. Eriguchi (1987, ApJ, 323, 592 - 613) have mapped out the full sequence of Dyson-Wong tori, beginning from a bifurcation point on the Maclaurin spheroid sequence.
External Potential in Terms of Angle ψ
Step 1
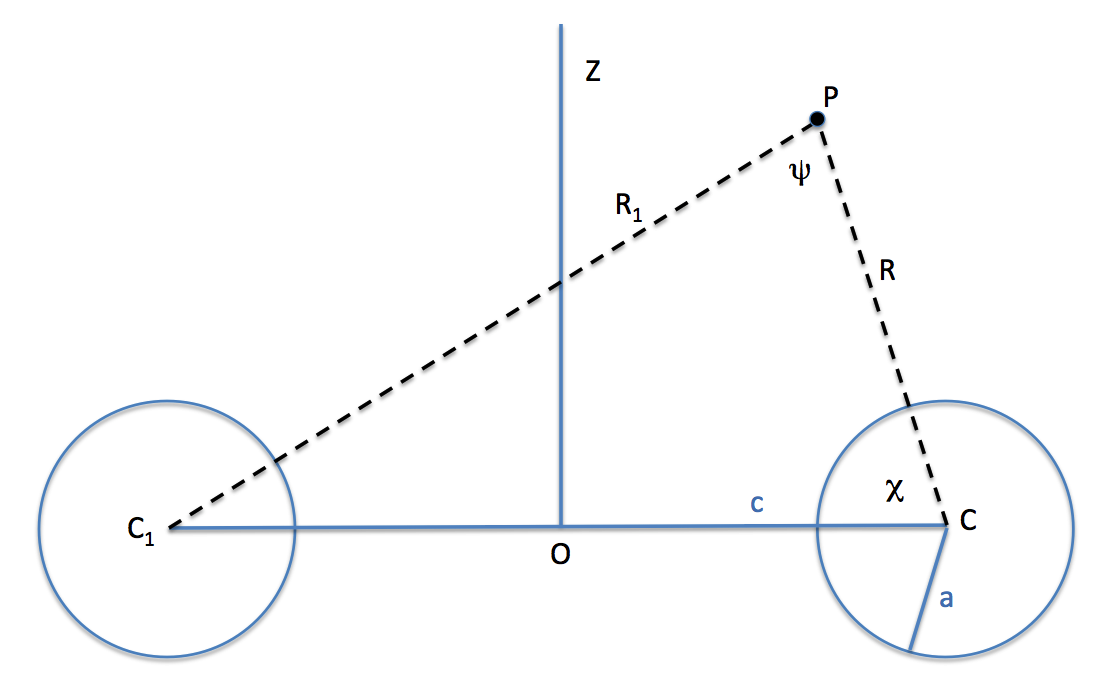
On p. 59, at the end of §6 of Dyson (1893a), we find the following expression for the potential at point "P", anywhere exterior to an anchor ring:
|
<math>~\frac{\pi V(r,\theta)}{M}</math> |
<math>~=</math> |
<math>~ \mathfrak{I}(r,\theta,c) ~+~ \frac{a^2}{2^3} ~\frac{1}{c} \cdot \frac{d}{dc} \biggl[ \mathfrak{I}(r,\theta,c)\biggr] ~-~ \frac{a^4}{2^6\cdot 3} ~\frac{1}{c} \cdot \frac{d}{dc} \biggl\{ \frac{1}{c} \cdot \frac{d}{dc} \biggl[ \mathfrak{I}(r,\theta,c)\biggr]\biggr\} ~+~\cdots </math> |
|
|
|
<math>~ ~+~(-1)^{n+1} \frac{2a^{2n}}{2n+2} \biggl[ \frac{1\cdot 3\cdot 5 \cdots (2n-3)}{2^2\cdot 4^2\cdot 6^2\cdots(2n)^2} \biggr] \biggl( \frac{1}{c}\cdot \frac{d}{dc}\biggr)^n \biggl[ \mathfrak{I}(r,\theta,c)\biggr] ~+~ \cdots </math> |
where (see beginning of §8 on p. 61),
|
|
<math>~\mathfrak{I}(r,\theta,c)</math> |
<math>~\equiv</math> |
<math>~ \int_0^\pi d\phi \biggl[r^2 - 2cr\sin\theta \cos\phi +c^2\biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ 2\int_0^{\pi/2} d\phi \biggl[ R_1^2 - (R_1^2-R^2)\sin^2\phi \biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ \frac{2}{R_1}\int_0^{\pi/2} d\phi \biggl[ 1 - \biggl( \frac{R_1^2-R^2}{R_1^2}\biggr) \sin^2\phi \biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ \frac{2K(k)}{R_1} \, , </math> |
and, where furthermore,
|
<math>~K(k)</math> |
<math>~=</math> |
<math>~ \int_0^{\pi/2} d\phi \biggl[1 - k^2\sin^2\phi \bigg]^{-1 / 2} </math> |
and |
<math>~k</math> |
<math>~\equiv</math> |
<math>~ \biggl[ \frac{R_1^2-R^2}{R_1^2} \biggr]^{1 / 2} \, . </math> |
Step 2
Taking a queue from our accompanying discussion of toroidal coordinates, if we adopt the variable notation,
<math>~\eta \equiv \ln\biggl(\frac{R_1}{R}\biggr) \, ,</math>
then we can write,
|
<math>~\cosh\eta = \frac{1}{2}\biggl[e^\eta + e^{-\eta}\biggr]</math> |
<math>~=</math> |
<math>~\frac{R^2 + R_1^2}{2RR_1} \, ,</math> |
which implies that,
|
<math>~\biggl[ \frac{2}{\coth\eta +1} \biggr]^{1 / 2} = [1 - e^{-2\eta}]^{1 / 2}</math> |
<math>~=</math> |
<math>~\biggl[ 1 - \biggl(\frac{R}{R_1}\biggr)^2 \biggr]^{1 / 2} = k \, .</math> |
Now, if we employ the Descending Landen Transformation for the complete elliptic integral of the first kind, we can make the substitution,
|
<math>~K(k)</math> |
<math>~=</math> |
<math>~ (1 + \mu)K(\mu) \, , </math> |
where, |
<math>~\mu</math> |
<math>~\equiv</math> |
<math>~ \frac{1-\sqrt{1-k^2}}{1+\sqrt{1-k^2}} \, . </math> |
But notice that, <math>~\sqrt{1-k^2} = e^{-\eta}</math>, in which case,
|
<math>~\mu </math> |
<math>~=</math> |
<math>~ \frac{1-e^{-\eta}}{1+e^{-\eta}} </math> |
<math>~=</math> |
<math>~ \frac{1-R/R_1}{1+R/R_1} </math> |
<math>~=</math> |
<math>~ \frac{R_1-R}{R_1+R} \, . </math> |
Hence, we can write,
|
<math>~\mathfrak{I}(r,\theta,c) = \frac{2K(k)}{R_1}</math> |
<math>~=</math> |
<math>~ \frac{2}{R_1} \biggl[(1+\mu)K(\mu) \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{2K(\mu)}{R_1} \biggl[1+\frac{R_1-R}{R_1+R} \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{4K(\mu)}{R_1+R} \, .</math> |
This is the expression for <math>~\mathfrak{I}(r,\theta,c) </math> that was adopted by Dyson at the beginning of his §8.
Step 3
Subsequently, Dyson was able to obtain analytic expressions for successive derivatives of the function, <math>~\mathfrak{I}(r,\theta,c) </math>, by first demonstrating that
|
<math>~\frac{dR}{dc}</math> |
<math>~=</math> |
<math>~\frac{4c^2 + R^2 - R_1^2}{4cR} \, ,</math> |
|
<math>~\frac{dR_1}{dc}</math> |
<math>~=</math> |
<math>~\frac{4c^2 + R_1^2 - R^2}{4cR_1} \, ,</math> and, |
|
<math>~\frac{d\mu}{dc}</math> |
<math>~=</math> |
<math>~\frac{\mu}{c} \cos\psi \, ,</math> |
where — as shown above in the Anchor ring schematic — <math>~\psi</math> is the angle between <math>~R</math> and <math>~R_1</math> for which (according to the law of cosines),
|
<math>~\cos\psi</math> |
<math>~=</math> |
<math>~\frac{R^2 + R_1^2 - 4c^2}{2RR_1} \, .</math> |
It will be useful for us to note that,
|
<math>~\frac{d(\cos\psi)}{dc}</math> |
<math>~=</math> |
<math>~ \frac{d}{dc}\biggl[ \frac{R^2 + R_1^2 - 4c^2}{2RR_1} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{2RR_1}\frac{d}{dc}\biggl[ R^2 + R_1^2 - 4c^2 \biggr] ~+~(R^2 + R_1^2 - 4c^2) \frac{d}{dc}\biggl[ \frac{1}{2RR_1} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{2RR_1}\biggl[2R\frac{dR}{dc} + 2R_1\frac{dR_1}{dc} - 8c \biggr] ~+~(R^2 + R_1^2 - 4c^2)\biggl[ - \frac{1}{2R^2R_1} \frac{dR}{dc} - \frac{1}{2RR_1^2}\frac{dR_1}{dc} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{4cRR_1}\biggl[ 4c^2 + R^2 - R_1^2 + 4c^2 + R_1^2 - R^2 - 2^4c^2 \biggr] ~-~(R^2 + R_1^2 - 4c^2)\biggl\{ \biggl[ \frac{4c^2 + R^2 - R_1^2}{8cR^3R_1}\biggr] + \biggl[ \frac{4c^2 + R_1^2 - R^2}{8cR_1^3 R} \biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ -~\frac{2c}{RR_1} ~-~\frac{\cos\psi}{4cR^2 R_1^2} \biggl[4c^2(R_1^2 + R^2) + 2R_1^2 R^2 - R_1^4 - R^4 \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{\cos\psi}{4cR^2 R_1^2} \biggl[ R_1^4 + R^4- 4c^2(R_1^2 + R^2) - 2R_1^2 R^2 \biggr] -~\frac{2c}{RR_1} </math> |
|
|
<math>~=</math> |
<math>~\frac{1}{4cR^2 R_1^2}\biggl\{ \cos\psi [R_1^4 + R^4 - 2R_1^2 R^2 - 4c^2(R_1^2 + R^2) ] -~8c^2R R_1 \biggr\} </math> |
But,
|
<math>~[R_1^4 + R^4 - 2R_1^2 R^2 - 4c^2(R_1^2 + R^2)]</math> |
<math>~=</math> |
<math>~ (R_1^2 - R^2)^2 - 4c^2(R_1^2 + R^2) </math> |
|
|
<math>~=</math> |
<math>~ [(R_1+R)(R_1-R)]^2 - 4c^2(R_1^2 + R^2) </math> |
|
|
<math>~=</math> |
<math>~ (R_1^2 + 2R_1 R +R^2)(R_1-R)^2 - 4c^2(R_1^2 + R^2) </math> |
|
|
<math>~=</math> |
<math>~ 2R_1 R(R_1-R)^2 +(R_1^2 + R^2)(R_1-R)^2 - 4c^2(R_1^2 + R^2) </math> |
|
|
<math>~=</math> |
<math>~ 2R_1 R(R_1-R)^2 +(R_1^2 + R^2)[R_1^2 -2R_1 R + R^2 - 4c^2] </math> |
|
|
<math>~=</math> |
<math>~ 2R_1 R[ (R_1-R)^2 +(R_1^2 + R^2)(\cos\psi - 1)] </math> |
|
|
<math>~=</math> |
<math>~ 2R_1 R[ (R_1^2 + R^2)\cos\psi - 2R_1 R] </math> |
|
|
<math>~=</math> |
<math>~ (2R_1 R)^2 \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr] </math> |
Hence,
|
<math>~ \frac{d(\cos\psi)}{dc} </math> |
<math>~=</math> |
<math>~\frac{(2R_1 R)^2 }{4cR^2 R_1^2}\biggl\{ \cos\psi \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr] -~\frac{8c^2R R_1}{(2R_1 R)^2 } \biggr\} </math> |
|
|
<math>~=</math> |
<math>~\frac{1}{c}\biggl\{ \cos\psi \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr] -~\frac{2c^2}{R_1 R } \biggr\} </math> |
|
<math>~ \Rightarrow ~~~ 2c\cdot \frac{d(\cos\psi)}{dc} </math> |
<math>~=</math> |
<math>~ 2\cos\psi \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr] -~\frac{4c^2}{R_1 R } </math> |
Step 4
Then, drawing upon known expressions for the derivatives of elliptic integrals, as are now tidily catalogued online in NIST's Digital Library of Mathematical Functions, Dyson showed that,
|
LaTeX mathematical expressions cut-and-pasted directly from
|
|||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
According to §19.4 of NIST's Digital Library of Mathematical Functions,
where, <math>~k^{\prime} \equiv \sqrt{1 - k^2} \, .</math> |
|
<math>~\frac{d\mathfrak{I}(r,\theta,c)}{dc} = \frac{d}{dc}\biggl[\frac{4K(\mu)}{R_1+R}\biggr]</math> |
<math>~=</math> |
<math>~ 4K(\mu) \frac{d}{dc}\biggl[ R_1 + R \biggr]^{-1} + \frac{4}{(R_1+R)} \biggl[ \frac{E\left(\mu\right)-{\mu^{\prime}}^{2}K\left(\mu\right)}{\mu{\mu^{\prime}}^{2}} \biggr]\frac{d\mu}{dc} </math> |
|
|
<math>~=</math> |
<math>~ -~\frac{4K(\mu)}{(R_1+R)^2} \biggl[ \frac{dR_1}{dc} + \frac{dR}{dc} \biggr] ~-~ \frac{4}{(R_1+R)} \biggl[ \frac{K\left(\mu\right)}{\mu} \biggr]\frac{\mu}{c}\cos\psi + \frac{4}{(R_1+R)} \biggl[ \frac{E\left(\mu\right)}{\mu{\mu^{\prime}}^{2}} \biggr]\frac{\mu}{c}\cos\psi </math> |
|
|
<math>~=</math> |
<math>~ -~\frac{4K(\mu)}{(R_1+R)^2} \biggl[ \frac{4c^2 + R_1^2 - R^2}{4cR_1} + \frac{4c^2 + R^2 - R_1^2}{4cR} \biggr] ~-~ \biggl[ \frac{4K\left(\mu\right)}{c(R_1+R)} \biggr] \cos\psi + \frac{4E\left(\mu\right)}{c(R_1+R)} \biggl[ \frac{(R_1+R)^2}{4RR_1} \biggr]\cos\psi </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{cRR_1}\biggr] \cos\psi ~-~ \biggl[ \frac{4K\left(\mu\right)}{c(R_1+R)}\biggr] \cos\psi ~-~ \frac{4K(\mu)}{4cR_1 R (R_1+R)^2} \biggl[ R(4c^2 + R_1^2 - R^2) + R_1(4c^2 + R^2 - R_1^2) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{cRR_1}\biggr] \cos\psi ~-~ \biggl[ \frac{4K\left(\mu\right)}{c(R_1+R)}\biggr] \cos\psi ~-~ \frac{K(\mu)}{cR_1 R (R_1+R)^2}\biggl[(4c^2 + R_1R)(R_1+R) - (R_1^3 + R^3) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{cRR_1}\biggr] \cos\psi ~-~ \biggl[ \frac{4K\left(\mu\right)}{c(R_1+R)}\biggr] \cos\psi ~-~ \frac{K(\mu)}{cR_1 R (R_1+R)}\biggl[(4c^2 + R_1R) - (R_1^2 + R^2 - R_1R) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{cRR_1}\biggr] \cos\psi ~-~ \biggl[ \frac{4K\left(\mu\right)}{c(R_1+R)}\biggr] \cos\psi ~-~ \frac{K(\mu)}{cR_1 R (R_1+R)}\biggl[4c^2 - (R_1 - R)^2 \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{cRR_1}\biggr] \cos\psi ~-~ \biggl[ \frac{4K\left(\mu\right)}{c(R_1+R)}\biggr] \cos\psi ~-~ \frac{K(\mu)}{c (R_1+R)}\biggl[2(1-\cos\psi) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{cRR_1}\biggr] \cos\psi~-~\frac{2K(\mu)}{c (R_1+R)}(1+\cos\psi) </math> |
|
<math>~\Rightarrow ~~~\frac{1}{c}\cdot \frac{d\mathfrak{I}(r,\theta,c)}{dc} </math> |
<math>~=</math> |
<math>~ \frac{1}{c^2}\biggl\{\biggl[ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] \cos\psi ~-~\biggl[ \frac{4K(\mu)}{R+R_1} \biggr] \cos^2\frac{\psi}{2} \biggr\} \, . </math> |
This expression appears at the top of Dyson's p. 62.
Step 5
Differentiating a second time gives,
|
<math>~\frac{d}{dc}\biggl[\frac{1}{c}\cdot \frac{d\mathfrak{I}(r,\theta,c)}{dc}\biggr] </math> |
<math>~=</math> |
<math>~ \frac{d}{dc}\biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{c^2RR_1}\biggr] \cos\psi~-~\frac{2K(\mu)}{c^2 (R_1+R)}(1+\cos\psi) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~\frac{2}{c^3}\biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \cos\psi~-~\frac{2K(\mu)}{ (R_1+R)}(1+\cos\psi) \biggr\} </math> |
|
|
|
<math>~ ~+~\frac{1}{c^2}\cdot \frac{d}{dc}\biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \cos\psi~-~\frac{2K(\mu)}{ (R_1+R)}(1+\cos\psi) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~\frac{2}{c^3}\biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \cos\psi~-~\frac{2K(\mu)}{ (R_1+R)}(1+\cos\psi) \biggr\} </math> |
|
|
|
<math>~ ~+~\frac{1}{c^2}\cdot \frac{d}{dc}\biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}~-~\frac{2K(\mu)}{ (R_1+R)}\biggr] \cos\psi\biggr\} ~-~\frac{1}{c^2}\cdot \frac{d}{dc}\biggl[ \frac{2K(\mu)}{ (R_1+R)} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ ~-~\frac{2}{c^3}\biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \cos\psi~-~\frac{2K(\mu)}{ (R_1+R)}(1+\cos\psi) \biggr\} ~+~\frac{1}{c^2}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}~-~\frac{2K(\mu)}{ (R_1+R)}\biggr] \frac{d(\cos\psi)}{dc} </math> |
|
|
|
<math>~ ~+~\frac{\cos\psi}{c^2}\cdot \frac{d}{dc}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] ~-~\frac{(1+\cos\psi)}{2c^2}\cdot \frac{d}{dc}\biggl[\frac{4K(\mu)}{ (R_1+R)}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{c^3}\biggl[ \frac{4K(\mu)}{ (R_1+R)}\biggr]\biggl[(1+\cos\psi) - \biggl(\frac{c}{2}\biggr) \frac{d(\cos\psi)}{dc}\biggr] ~-~\frac{2}{c^3}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[ \cos\psi - \biggl(\frac{c}{2}\biggr) \frac{d(\cos\psi)}{dc}\biggr] </math> |
|
|
|
<math>~ ~+~\frac{\cos\psi}{c^2}\cdot \frac{d}{dc}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] ~-~\frac{(1+\cos\psi)}{2c^3} \biggl\{\biggl[ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] \cos\psi ~-~\biggl[ \frac{4K(\mu)}{R+R_1} \biggr] \cos^2\frac{\psi}{2} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{c^3}\biggl[ \frac{4K(\mu)}{ (R_1+R)}\biggr]\biggl[\frac{(1+\cos\psi)^2}{2^2} + (1+\cos\psi) - \biggl(\frac{c}{2}\biggr) \frac{d(\cos\psi)}{dc}\biggr] </math> |
|
|
|
<math>~ ~-~\frac{2}{c^3}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[\frac{(\cos\psi+\cos^2\psi)}{4} ~+~ \cos\psi ~-~ \biggl(\frac{c}{2}\biggr) \frac{d(\cos\psi)}{dc}\biggr] ~+~\frac{\cos\psi}{c^2}\cdot \frac{d}{dc}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{4c^3}\biggl[ \frac{4K(\mu)}{ (R_1+R)}\biggr]\biggl[5+6\cos\psi + \cos^2\psi - 2c \cdot \frac{d(\cos\psi)}{dc}\biggr] </math> |
|
|
|
<math>~ ~-~\frac{1}{2c^3}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[5\cos\psi+\cos^2\psi ~-~ 2c \cdot \frac{d(\cos\psi)}{dc}\biggr] ~+~\frac{\cos\psi}{c^2}\cdot \frac{d}{dc}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \, . </math> |
Now,
|
<math>~ \frac{d}{dc}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)}{RR_1}\biggr]\frac{dE\left(\mu\right)}{d\mu} \cdot \frac{d\mu}{dc} ~+~ E\left(\mu\right)\frac{d}{dc}\biggl[ \frac{(R_1+R)}{RR_1}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)}{RR_1}\biggr]\biggl[\frac{E(\mu) - K(\mu)}{c} \biggr] \cos\psi ~+~ E\left(\mu\right)\biggl\{ \frac{1}{RR_1}\biggl[\frac{dR_1}{dc} + \frac{dR}{dc}\biggr] ~-~ (R_1+R)\biggl[\frac{1}{R R_1^2} \frac{dR_1}{dc} + \frac{1}{R^2 R_1} \frac{dR}{dc}\biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{(R_1+R)}{RR_1}\biggr]\biggl[\frac{E(\mu) - K(\mu)}{c} \biggr] \cos\psi ~-~ E\left(\mu\right)\biggl\{ \biggl[\frac{1}{R^2} \frac{dR}{dc}\biggr] ~+~ \biggl[\frac{1}{R_1^2} \frac{dR_1}{dc} \biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~K(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi +E(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi ~-~ E\left(\mu\right)\biggl\{ \biggl[\frac{4c^2 + R^2-R_1^2}{4cR^3}\biggr] ~+~ \biggl[ \frac{4c^2+R_1^2-R^2}{4cR_1^3} \biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~K(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi +E(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi ~+~ E\left(\mu\right)\biggl\{ \biggl[\frac{R_1^2 + R^2-4c^2 }{4cR^3}\biggr] ~-~\biggl[\frac{R^2 }{2cR^3}\biggr] ~+~ \biggl[ \frac{R_1^2 + R^2-4c^2}{4cR_1^3} \biggr] ~-~ \biggl[ \frac{R_1^2}{2cR_1^3} \biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~K(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi +E(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi ~+~ \frac{E\left(\mu\right)}{2cR_1^2R^2} \biggl\{ R_1^3 \cos\psi ~-~R R_1^2 ~+~ R^3\cos\psi ~-~ R_1 R^2 \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~K(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi +E(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi ~+~ \frac{E\left(\mu\right)}{2cR_1^2R^2} \biggl\{ (R_1^3 + R^3) \cos\psi ~-~R R_1(R_1 + R) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~K(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi +E(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi ~+~ \frac{E\left(\mu\right)(R_1+R)}{2cR_1^2R^2} \biggl\{ (R_1^2 + R^2 - 4c^2) \cos\psi + 4c^2\cos\psi~-~R R_1(1+\cos\psi) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~K(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi +E(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi ~+~ \frac{E\left(\mu\right)(R_1+R)}{cR_1 R} \biggl\{ \cos^2\psi ~+~ \frac{2c^2\cos\psi }{R_1R} ~-~\frac{1}{2}(1+\cos\psi) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ ~-~K(\mu)\biggl[ \frac{(R_1+R)}{cRR_1}\biggr] \cos\psi ~+~ \frac{E\left(\mu\right)(R_1+R)}{cR_1 R} \biggl[ \cos^2\psi ~+~\frac{1}{2}(\cos\psi - 1) ~+~ \frac{2c^2\cos\psi }{R_1R} \biggr] \, . </math> |
We therefore have,
|
<math>~\frac{1}{c} \cdot \frac{d}{dc}\biggl[\frac{1}{c}\cdot \frac{d\mathfrak{I}(r,\theta,c)}{dc}\biggr] </math> |
<math>~=</math> |
<math>~ \frac{1}{4c^4}\biggl[ \frac{4K(\mu)}{ (R_1+R)}\biggr]\biggl\{ 5+6\cos\psi + \cos^2\psi ~-~2\cos\psi \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr]~+~\frac{4c^2}{R_1 R } \biggr\} </math> |
|
|
|
<math>~ ~-~\frac{1}{2c^4}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl\{ 5\cos\psi+\cos^2\psi ~-~2\cos\psi \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr]~+~\frac{4c^2}{R_1 R } \biggr\} </math> |
|
|
|
<math>~ ~+~\frac{\cos\psi}{c^4} \biggl\{ \frac{E\left(\mu\right)(R_1+R)}{R_1 R} \biggl[ \cos^2\psi ~+~\frac{1}{2}(\cos\psi - 1) ~+~ \frac{2c^2\cos\psi }{R_1R} \biggr] ~-~K(\mu)\biggl[ \frac{(R_1+R)}{RR_1}\biggr] \cos\psi \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{c^4}\biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl\{ 5+6\cos\psi + \cos^2\psi ~-~2\cos\psi \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr]~+~\frac{4c^2}{R_1 R } \biggr\} </math> |
|
|
|
<math>~ ~-~\frac{1}{2c^4}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl\{ 5\cos\psi+\cos^2\psi ~-~2\cos\psi \biggl[ \cos^2\psi + \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos\psi - 1 \biggr]~+~\frac{4c^2}{R_1 R } \biggr\} </math> |
|
|
|
<math>~ ~-~\frac{1}{2c^4} \biggl[ \frac{E\left(\mu\right)(R_1+R)}{R_1 R}\biggr] \biggl[ ~-~2\cos^3\psi ~-~\cos^2\psi ~+~ \cos\psi ~-~ \frac{4c^2\cos^2\psi }{R_1R} \biggr] </math> |
|
|
|
<math>~ ~+~\frac{1}{c^4}\biggl[\frac{K(\mu)}{(R_1 + R)}\biggr] \biggl[~-~ \frac{(R_1+R)^2\cos^2\psi}{RR_1}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{c^4}\biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl\{ 5 ~+~ 8\cos\psi ~+~ \cos^2\psi ~-~2\cos^3\psi ~+~\frac{4c^2}{R_1 R } ~-~ \biggl[ \frac{R_1^2 + R^2 - 4c^2 }{R_1 R} \biggr]\cos^2\psi ~-~ \biggl[ \frac{2R_1R }{R_1 R} \biggr]\cos^2\psi ~-~ \biggl[ \frac{8c^2 }{R_1 R} \biggr]\cos^2\psi \biggr\} </math> |
|
|
|
<math>~ ~-~\frac{1}{2c^4}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl\{ 8\cos\psi ~-~4\cos^3\psi ~+~ \biggl(\frac{4c^2 }{R_1 R}\biggr)(1-2\cos^2\psi) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{c^4}\biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl\{ 5 ~+~ 8\cos\psi ~-~ \cos^2\psi ~-~4\cos^3\psi ~+~\frac{4c^2}{R_1 R }(1-2\cos^2\psi) \biggr\} </math> |
|
|
|
<math>~ ~-~\frac{1}{c^4}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl\{ 4\cos\psi ~-~2\cos^3\psi ~+~ \biggl(\frac{2c^2 }{R_1 R}\biggr)(1-2\cos^2\psi) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{c^4}\biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl\{ 5 ~+~ 8\cos\psi ~-~ \cos^2\psi ~-~4\cos^3\psi ~-~\biggl( \frac{4c^2}{R_1 R } \biggr) \cos 2\psi \biggr\} </math> |
|
|
|
<math>~ ~+~\frac{1}{c^4}\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl\{ ~-~4\cos\psi ~+~2\cos^3\psi ~+~ \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos 2\psi \biggr\} \, , </math> |
which exactly matches the second equation from the top of p. 62 in Dyson (1893a).
Step 6 (Summary)
In summary, then,
|
<math>~\frac{\pi V(r,\theta)}{M}</math> |
<math>~=</math> |
<math>~ \mathfrak{I}(r,\theta,c) ~+~ \frac{a^2}{2^3} ~\frac{1}{c} \cdot \frac{d}{dc} \biggl[ \mathfrak{I}(r,\theta,c)\biggr] ~-~ \frac{a^4}{2^6\cdot 3} ~\frac{1}{c} \cdot \frac{d}{dc} \biggl\{ \frac{1}{c} \cdot \frac{d}{dc} \biggl[ \mathfrak{I}(r,\theta,c)\biggr]\biggr\} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) </math> |
|
|
<math>~=</math> |
<math>~ \frac{4K(\mu)}{R_1+R} ~+~ \frac{1}{2^3} ~\frac{a^2}{c^2}\biggl\{\biggl[ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] \cos\psi ~-~\biggl[ \frac{4K(\mu)}{R+R_1} \biggr] \cos^2\frac{\psi}{2} \biggr\} </math> |
|
|
|
<math>~ ~-~ \frac{1}{2^6\cdot 3} \frac{a^4}{c^4} \biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[ ~-~4\cos\psi ~+~2\cos^3\psi ~+~ \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos 2\psi \biggr] </math> |
|
|
|
<math>~ ~+~ \biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl[ 5 ~+~ 8\cos\psi ~-~ \cos^2\psi ~-~4\cos^3\psi ~-~\biggl( \frac{4c^2}{R_1 R } \biggr) \cos 2\psi \biggr] \biggr\} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \, . </math> |
In preparation for a comparison between Dyson's expression and the expression derived by Wong (1973), let's regroup terms according to the various trigonometric functions. Keep in mind that,
|
<math>~\cos^2 \frac{\psi}{2}</math> |
<math>~=</math> |
<math>~\frac{1}{2}(1 + \cos\psi) \, ;</math> |
|
<math>~\cos^2 \psi</math> |
<math>~=</math> |
<math>~\frac{1}{2}(1 + \cos2\psi) \, ;</math> |
|
<math>~\cos^3 \psi</math> |
<math>~=</math> |
<math>~\frac{1}{4}(3\cos\psi + \cos3\psi) \, .</math> |
Hence, we have,
|
<math>~\frac{\pi V(r,\theta)}{M}</math> |
<math>~=</math> |
<math>~ \frac{4K(\mu)}{R_1+R} ~+~ \frac{1}{2^3} ~\frac{a^2}{c^2}\biggl\{\biggl[ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] \cos\psi ~-~\biggl[ \frac{2K(\mu)}{R+R_1} \biggr] (1+\cos\psi) \biggr\} </math> |
|
|
|
<math>~ ~-~ \frac{1}{2^6\cdot 3} \frac{a^4}{c^4} \biggl\{ \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[ ~-~4\cos\psi ~+~\frac{1}{2}(3\cos\psi + \cos3\psi) ~+~ \biggl(\frac{2c^2 }{R_1 R}\biggr)\cos 2\psi \biggr] </math> |
|
|
|
<math>~ ~+~ \biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl[ 5 ~+~ 8\cos\psi ~-~ \frac{1}{2}(1 + \cos2\psi) ~-~(3\cos\psi + \cos3\psi) ~-~\biggl( \frac{4c^2}{R_1 R } \biggr) \cos 2\psi \biggr] \biggr\} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) </math> |
|
|
<math>~=</math> |
<math>~ \frac{4K(\mu)}{R_1+R} ~-~\frac{1}{2^3} ~\frac{a^2}{c^2}\biggl[ \frac{2K(\mu)}{R+R_1} \biggr] ~+~ \frac{1}{2^3} ~\frac{a^2}{c^2}\biggl[ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] \cos\psi ~-~\frac{1}{2^3} ~\frac{a^2}{c^2}\biggl[ \frac{2K(\mu)}{R+R_1} \biggr] \cos\psi </math> |
|
|
|
<math>~ ~-~ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[ ~-~5\cos\psi ~+~ \biggl(\frac{4c^2 }{R_1 R}\biggr)\cos 2\psi ~+~ \cos3\psi \biggr] </math> |
|
|
|
<math>~ ~-~ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl[ 9 ~+~ 10\cos\psi ~-~ \cos2\psi ~-~\biggl( \frac{8c^2}{R_1 R } \biggr) \cos 2\psi ~-~2 \cos3\psi \biggr] ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) </math> |
|
|
<math>~=</math> |
<math>~ \frac{2K(\mu)}{R_1+R} \biggl[ 2 ~-~\frac{1}{2^3} ~\frac{a^2}{c^2} ~-~ \frac{3}{2^8} \frac{a^4}{c^4}~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr] </math> |
|
|
|
<math>~ ~+~\biggl\{ ~-~ \frac{5}{2^6\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr] ~+~\frac{1}{2^3} ~\frac{a^2}{c^2}\biggl[ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] ~-~\frac{1}{2^3} ~\frac{a^2}{c^2}\biggl[ \frac{2K(\mu)}{R+R_1} \biggr] ~+~ \frac{5}{2^7\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggr\}\cos\psi </math> |
|
|
|
<math>~ ~+~ \biggl\{ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr]\biggl[ 1 ~+~\biggl( \frac{8c^2}{R_1 R } \biggr) \biggr] ~-~ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl(\frac{4c^2 }{R_1 R}\biggr) \biggr\} \cos 2\psi </math> |
|
|
|
<math>~ ~+~\biggl\{ \frac{1}{2^6\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{K(\mu)}{ (R_1+R)}\biggr] ~-~ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} \biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggr\}\cos3\psi ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) </math> |
|
|
<math>~=</math> |
<math>~ \frac{2K(\mu)}{R_1+R} \biggl[ 2 ~-~\frac{1}{2^3} ~\frac{a^2}{c^2} ~-~ \frac{3}{2^8} \frac{a^4}{c^4}~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr] </math> |
|
|
|
<math>~ ~-~\biggl[ \frac{2K(\mu)}{R+R_1} ~-~ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] \biggl[ \frac{1}{2^3} ~\frac{a^2}{c^2} ~+~ \frac{5}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos\psi </math> |
|
|
|
<math>~ ~+~ \biggl\{ \biggl[ \frac{ 2K(\mu)}{ (R_1+R)}\biggr]\biggl[ \frac{1}{2} ~+~\biggl( \frac{4c^2}{R_1 R } \biggr) \biggr] ~-~\biggl[ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl(\frac{4c^2 }{R_1 R}\biggr) \biggr\} \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos 2\psi </math> |
|
|
|
<math>~ ~+~ \biggl[ \frac{2K(\mu)}{ (R_1+R)} ~-~ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr)\biggr] \cos3\psi </math> |
|
|
<math>~=</math> |
<math>~ \frac{2K(\mu)}{R_1+R} \biggl[ 2 ~-~\frac{1}{2^3} ~\frac{a^2}{c^2} ~-~ \frac{3}{2^8} \frac{a^4}{c^4}~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr] </math> |
|
|
|
<math>~ ~-~\biggl[ \frac{2K(\mu)}{R+R_1} ~-~ \frac{E(\mu)(R+R_1)}{RR_1} \biggr] \biggl[ \frac{1}{2^3} ~\frac{a^2}{c^2} ~+~ \frac{5}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos\psi </math> |
|
|
|
<math>~ ~+~ \biggl[ \frac{ 2K(\mu)}{ (R_1+R)}~-~\frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl(\frac{4c^2 }{R_1 R}\biggr) \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos 2\psi ~+~ \biggl[ \frac{ 2K(\mu)}{ (R_1+R)}\biggr] \biggl[ \frac{1}{2^8\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos 2\psi </math> |
|
|
|
<math>~ ~+~\biggl[ \frac{2K(\mu)}{ (R_1+R)} ~-~ \frac{(R_1+R)E\left(\mu\right)}{RR_1}\biggr] \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr)\biggr] \cos3\psi </math> |
Now, drawing from our accompanying discussion of relationships between complete elliptic integrals and relationships between their parameter arguments and, in particular, associating Dyson's parameter, <math>~\mu = (R_1-R)/(R_1+R)</math>, with <math>~k_1</math>, we understand that,
|
<math>~\frac{2E(\mu)}{1+\mu} - (1-\mu)K(\mu)</math> |
<math>~=</math> |
<math>~E(k) \, ,</math> |
where the alternate parameter,
<math>~k = \frac{2\sqrt{\mu}}{1+\mu} = \biggl[1 - \biggr(\frac{R}{R_1}\biggr)^2\biggr]^{1 / 2} = \biggl[ \frac{2}{\coth\eta+1}\biggr]^{1 / 2} = \sqrt{1-e^{-2\eta}} \, .</math>
Hence, we appreciate that,
|
<math>~E(k)</math> |
<math>~=</math> |
<math>~ 2E(\mu) \biggl[ 1 + \frac{R_1-R}{R_1+R} \biggr]^{-1} - \biggl[ 1 - \frac{R_1-R}{R_1+R} \biggr]K(\mu) </math> |
|
|
<math>~=</math> |
<math>~ 2E(\mu) \biggl[ \frac{2R_1}{R_1+R} \biggr]^{-1} - \biggl[ \frac{2R}{R_1+R} \biggr]K(\mu) </math> |
|
|
<math>~=</math> |
<math>~ R \biggl[ \frac{E(\mu)(R_1+R)}{RR_1} - \frac{2K(\mu)}{R_1+R} \biggr] </math> |
As a result, we can rewrite Dyson's expression for the external potential as,
|
<math>~\frac{\pi V(r,\theta)}{M}</math> |
<math>~=</math> |
<math>~ \frac{2K(\mu)}{R_1+R} \biggl[ 2 ~-~\frac{1}{2^3} ~\frac{a^2}{c^2} ~-~ \frac{3}{2^8} \frac{a^4}{c^4}~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr] ~+~\frac{E(k)}{R} \biggl[ \frac{1}{2^3} ~\frac{a^2}{c^2} ~+~ \frac{5}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos\psi </math> |
|
|
|
<math>~ ~-~ \frac{E(k)}{R}\biggl(\frac{4c^2 }{R_1 R}\biggr) \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos 2\psi ~+~ \biggl[ \frac{ 2K(\mu)}{ (R_1+R)}\biggr] \biggl[ \frac{1}{2^8\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos 2\psi </math> |
|
|
|
<math>~ ~-~\frac{E(k)}{R} \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr)\biggr] \cos3\psi </math> |
|
|
<math>~=</math> |
<math>~ \frac{2K(\mu)}{R_1+R} \biggl\{\biggl[ 2 ~-~\frac{1}{2^3} ~\frac{a^2}{c^2} ~-~ \frac{3}{2^8} \frac{a^4}{c^4}~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr] ~+~\biggl[ \frac{1}{2^8\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos 2\psi \biggr\} </math> |
|
|
|
<math>~ ~+~\frac{E(k)}{R} \biggl\{ \biggl[ \frac{1}{2^3} ~\frac{a^2}{c^2} ~+~ \frac{5}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos\psi ~-~ \biggl(\frac{4c^2 }{R_1 R}\biggr) \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \biggr]\cos 2\psi ~-~ \biggl[ \frac{1}{2^7\cdot 3} \frac{a^4}{c^4} ~+~\mathcal{O}\biggl(\frac{a^5}{c^5}\biggr)\biggr] \cos3\psi \biggr\} \, . </math> |
External Potential in Terms of Angle χ
|
Is it relatively straightforward to develop a similar expression for the external potential that is written in terms of the angle, <math>~\chi</math>, instead of (as above) in terms of the angle, <math>~\psi</math> ? This would make the transition to Dyson's Paper II smoother. Initially I have in mind making the transformation via the law of sines, whereby,
|
<math>~\frac{R_1}{\sin\chi}</math> |
<math>~=</math> |
<math>~\frac{2c}{\sin\psi}</math> |
<math>~=</math> |
<math>~\frac{R}{\sin(\pi - \psi - \chi)} \, .</math> |
This means that the following associations may be used as well:
|
<math>~\sin\psi</math> |
<math>~=</math> |
<math>~ \biggl(\frac{2c}{R_1} \biggr)\sin\chi \, ; </math> |
|
<math>~\cos^2\psi = 1 - \sin^2\psi</math> |
<math>~=</math> |
<math>~ 1 - \biggl[\biggl(\frac{2c}{R_1} \biggr)\sin\chi \biggr]^2\, . </math> |
From an accompanying discussion that builds upon the law of cosines, we also may write,
|
<math>~2\biggl(\frac{R_1}{c}\biggr)^{-1} </math> |
<math>~=</math> |
<math>~1 + \frac{1}{2}\biggl(\frac{a}{c}\biggr)\cos\chi + \frac{1}{2^3}\biggl(\frac{a}{c}\biggr)^2 \biggl[ 3\cos^2\chi - 1 \biggr] + \frac{1}{2^4}\biggl(\frac{a}{c}\biggr)^3\biggl[ 5\cos^3\chi ~-~ 3\cos\chi \biggr] </math> |
|
|
|
<math>~ ~+~ \frac{1}{2^7} \biggl( \frac{a}{c}\biggr)^4 \biggl[ 3 ~-~ 30 \cos^2\chi ~+~ 35 \cos^4\chi \biggr] ~+~ \mathcal{O}\biggl(\frac{a^5}{c^5}\biggr) \, . </math> |
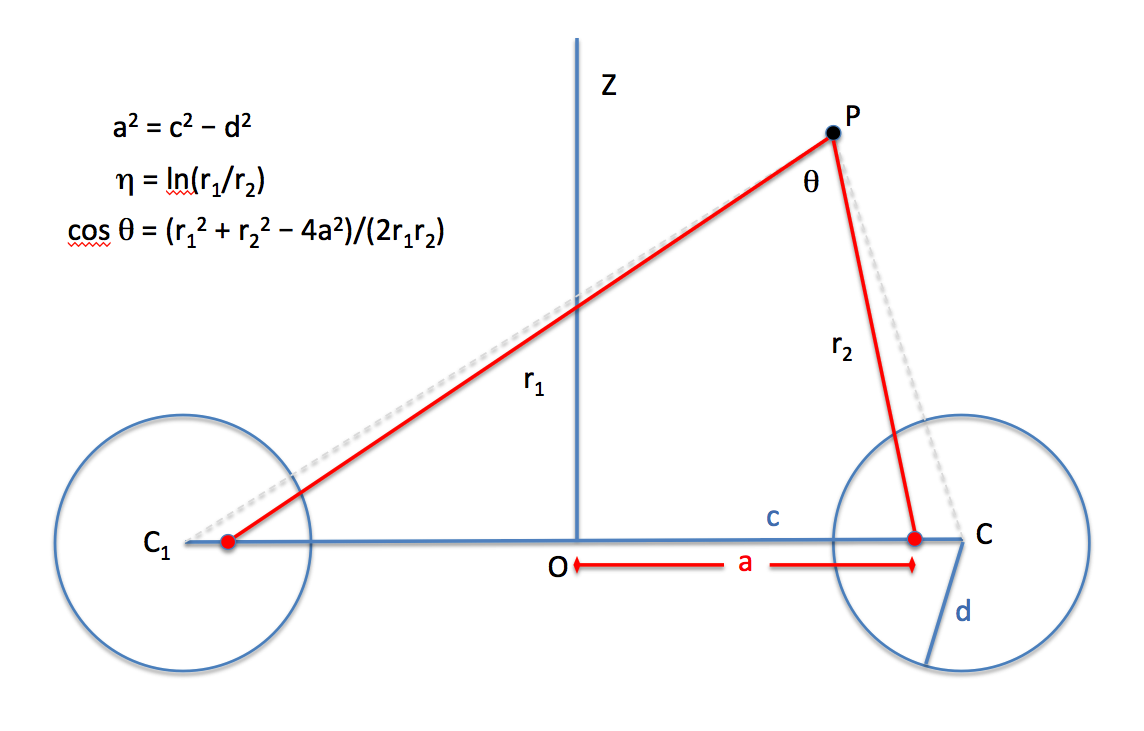
Compare Notations Used by Dyson and Wong
|
Dyson's Notation (slightly modified) |
Wong's Notation (slightly modified) |
Key relationship, with understanding that, <math>~\epsilon \equiv d/c</math>:
|
<math>~a^2</math> |
<math>~=</math> |
<math>~c^2 (1 - \epsilon^2)</math> |
<math>~\Rightarrow</math> |
<math>~\frac{a}{c}</math> |
<math>~=</math> |
<math>~(1 - \epsilon^2)^{1 / 2} \, .</math> |
Relationship Between Lengths
Subtraction
In both cases, lengths are referenced to the same "external" point whose meridional-plane coordinates are, <math>~(\varpi,z)</math>. Hence,
|
<math>~R^2</math> |
<math>~=</math> |
<math>~z^2 + (c-\varpi)^2 </math> |
|
<math>~r_2^2</math> |
<math>~=</math> |
<math>~z^2 + (a-\varpi)^2</math> |
|
<math>~\Rightarrow~R^2 - r_2^2</math> |
<math>~=</math> |
<math>~(c-\varpi)^2 - (a-\varpi)^2</math> |
|
|
<math>~=</math> |
<math>~(c^2 - 2c\varpi + \varpi^2) - (a^2 -2a\varpi +\varpi^2)</math> |
|
|
<math>~=</math> |
<math>~c^2 - a^2 - 2(c-a)\varpi</math> |
|
<math>~\Rightarrow~\varpi </math> |
<math>~=</math> |
<math>~\frac{c^2 - a^2 + r_2^2 - R^2}{2(c-a)} \, .</math> |
and,
|
<math>~R_1^2</math> |
<math>~=</math> |
<math>~z^2 + (c+\varpi)^2</math> |
|
<math>~r_1^2</math> |
<math>~=</math> |
<math>~z^2 + (a+\varpi)^2</math> |
|
<math>~\Rightarrow ~~~ R_1^2 - r_1^2</math> |
<math>~=</math> |
<math>~(c+\varpi)^2 - (a+\varpi)^2</math> |
|
|
<math>~=</math> |
<math>~(c^2 + 2c\varpi + \varpi^2) - (a^2 + 2a\varpi +\varpi^2)</math> |
|
|
<math>~=</math> |
<math>~c^2 - a^2 + 2(c - a)\varpi </math> |
|
<math>~\Rightarrow ~~~\varpi </math> |
<math>~=</math> |
<math>~ \frac{R_1^2 - r_1^2 - (c^2 - a^2)}{2(c-a)} \, .</math> |
Put together, then,
|
<math>~\frac{c^2 - a^2 + r_2^2 - R^2}{2(c-a)}</math> |
<math>~=</math> |
<math>~\frac{R_1^2 - r_1^2 - (c^2 - a^2)}{2(c-a)}</math> |
|
<math>~\Rightarrow ~~~(R^2 + R_1^2) - (r_1^2 + r_2^2) </math> |
<math>~=</math> |
<math>~2(c^2 - a^2)</math> |
|
|
<math>~=</math> |
<math>~2c^2 - 2c^2(1-\epsilon^2)</math> |
|
|
<math>~=</math> |
<math>~2c^2\epsilon^2</math> |
Multiplication
|
<math>~R^2R_1^2</math> |
<math>~=</math> |
<math>~[z^2 + (c-\varpi)^2][z^2 + (c+\varpi)^2]</math> |
|
<math>~\Rightarrow ~~~ 0</math> |
<math>~=</math> |
<math>~z^4 + z^2[(c-\varpi)^2 + (c+\varpi)^2] + [(c-\varpi)^2(c+\varpi)^2 - R^2R_1^2]</math> |
|
<math>~\Rightarrow~~~ 2z^2</math> |
<math>~=</math> |
<math>~ \pm \biggl[ [(c-\varpi)^2 + (c+\varpi)^2]^2 - 4[(c-\varpi)^2(c+\varpi)^2-R^2R_1^2] \biggr]^{1 / 2} ~-~ [(c-\varpi)^2 + (c+\varpi)^2] </math> |
|
<math>~r_1^2r_2^2</math> |
<math>~=</math> |
<math>~[z^2 + (a-\varpi)^2][z^2 + (a+\varpi)^2]</math> |
|
<math>~\Rightarrow ~~~ 0</math> |
<math>~=</math> |
<math>~z^4 + z^2[(a-\varpi)^2 + (a+\varpi)^2 ] + [(a-\varpi)^2(a+\varpi)^2 - r_1^2r_2^2]</math> |
|
<math>~\Rightarrow~~~ 2z^2</math> |
<math>~=</math> |
<math>~ \pm \biggl[ [(a-\varpi)^2 + (a+\varpi)^2 ]^2 ~-~ 4[(a-\varpi)^2(a+\varpi)^2 - r_1^2r_2^2] \biggr]^{1 / 2} ~-~ [(a-\varpi)^2 + (a+\varpi)^2 ] </math> |
Note: In order to be physically relevant, the quantity, <math>~2z^2</math>, must be positive. Given that the second term on the RHS is always negative, we conclude that only a solution with the superior (plus) sign is physically relevant.
Hence,
|
<math>~ \biggl[ [(c-\varpi)^2 + (c+\varpi)^2]^2 - 4[(c-\varpi)^2(c+\varpi)^2-R^2R_1^2] \biggr]^{1 / 2} ~-~ [(c-\varpi)^2 + (c+\varpi)^2] </math> |
<math>~=</math> |
<math>~ \biggl[ [(a-\varpi)^2 + (a+\varpi)^2 ]^2 ~-~ 4[(a-\varpi)^2(a+\varpi)^2 - r_1^2r_2^2] \biggr]^{1 / 2} ~-~ [(a-\varpi)^2 + (a+\varpi)^2 ] </math> |
Ratios
Let's work with the following (dimensionless) length ratios:
|
<math>~\lambda_2^2 \equiv \frac{R^2}{r_2^2}</math> |
<math>~=</math> |
<math>~\frac{z^2 + (c-\varpi)^2}{z^2 + (a-\varpi)^2} \, ,</math> |
|
<math>~\lambda_1^2 \equiv \frac{R_1^2}{r_1^2}</math> |
<math>~=</math> |
<math>~\frac{z^2 + (c+\varpi)^2}{z^2 + (a+\varpi)^2} \, .</math> |
Solving for <math>~z^2</math> in the first case, we find,
|
<math>~\lambda_2^2 [z^2 + (a-\varpi)^2]</math> |
<math>~=</math> |
<math>~z^2 + (c-\varpi)^2</math> |
|
<math>~\Rightarrow ~~~z^2( \lambda_2^2 - 1) </math> |
<math>~=</math> |
<math>~(c-\varpi)^2 - \lambda_2^2(a-\varpi)^2</math> |
|
<math>~\Rightarrow ~~~z^2 </math> |
<math>~=</math> |
<math>~\frac{(c-\varpi)^2 - \lambda_2^2(a-\varpi)^2}{( \lambda_2^2 - 1)} \, ;</math> |
and in the second case, we find,
|
<math>~\lambda_1^2 [z^2 + (a+\varpi)^2]</math> |
<math>~=</math> |
<math>~z^2 + (c+\varpi)^2</math> |
|
<math>~\Rightarrow ~~~ z^2 (\lambda_1^2 - 1)</math> |
<math>~=</math> |
<math>~(c+\varpi)^2 - \lambda_1^2 (a+\varpi)^2</math> |
|
<math>~\Rightarrow ~~~ z^2 </math> |
<math>~=</math> |
<math>~\frac{(c+\varpi)^2 - \lambda_1^2(a+\varpi)^2}{(\lambda_1^2 - 1)} \, .</math> |
Combined, this gives,
|
<math>~\frac{(c-\varpi)^2 - \lambda_2^2(a-\varpi)^2}{( \lambda_2^2 - 1)} </math> |
<math>~=</math> |
<math>~\frac{(c+\varpi)^2 - \lambda_1^2(a+\varpi)^2}{(\lambda_1^2 - 1)} </math> |
|
<math>~\Rightarrow ~~~ (\lambda_1^2 - 1)[(c-\varpi)^2 - \lambda_2^2(a-\varpi)^2] </math> |
<math>~=</math> |
<math>~( \lambda_2^2 - 1) [ (c+\varpi)^2 - \lambda_1^2(a+\varpi)^2 ]</math> |
|
<math>~\Rightarrow ~~~ (\lambda_1^2 - 1)[(c^2 - 2c\varpi + \varpi^2) - \lambda_2^2(a^2 - 2a\varpi +\varpi^2 )] </math> |
<math>~=</math> |
<math>~( \lambda_2^2 - 1) [ (c^2 + 2c\varpi + \varpi^2) - \lambda_1^2(a^2+ 2a \varpi + \varpi^2) ]</math> |
|
<math>~\Rightarrow ~~~ (\lambda_1^2 - 1)[c^2 - 2c\varpi + \varpi^2 - a^2\lambda_2^2 + 2a\varpi\lambda_2^2 - \varpi^2\lambda_2^2 ] </math> |
<math>~=</math> |
<math>~( \lambda_2^2 - 1) [ c^2 + 2c\varpi + \varpi^2 -a^2\lambda_1^2 - 2a \varpi\lambda_1^2 - \varpi^2\lambda_1^2 ]</math> |
|
<math>~\Rightarrow ~~~ (\lambda_1^2 - 1)[c^2 - 2c\varpi + \varpi^2 - a^2\lambda_2^2 + 2a\varpi\lambda_2^2 - \varpi^2\lambda_2^2 ] </math> |
<math>~=</math> |
<math>~( \lambda_2^2 - 1) [ c^2 + 2c\varpi + \varpi^2 -a^2\lambda_1^2 - 2a \varpi\lambda_1^2 - \varpi^2\lambda_1^2 ]</math> |
Relationship Between Key Angles
Law of Cosines:
|
<math>~(2c)^2</math> |
<math>~=</math> |
<math>~ R_1^2 + R^2 - 2RR_1\cos\psi </math> |
|
<math>~(2a)^2</math> |
<math>~=</math> |
<math>~ r_1^2 + r_2^2 - 2r_1 r_2 \cos\theta </math> |

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |