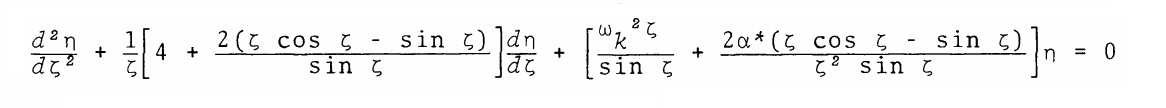Radial Oscillations of n = 1 Polytropic Spheres

Groundwork

In an accompanying discussion, we derived the so-called,

Adiabatic Wave (or Radial Pulsation) Equation

<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math>

whose solution gives eigenfunctions that describe various radial modes of oscillation in spherically symmetric, self-gravitating fluid configurations. Because this widely used form of the radial pulsation equation is not dimensionless but, rather, has units of inverse length-squared, we have found it useful to also recast it in the following dimensionless form:

<math> \frac{d^2x}{d\chi_0^2} + \biggl[\frac{4}{\chi_0} - \biggl(\frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{P_0}{P_c}\biggr)^{-1} \biggl(\frac{g_0}{g_\mathrm{SSC}}\biggr) \biggr] \frac{dx}{d\chi_0} + \biggl(\frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{P_0}{P_c}\biggr)^{-1} \biggl(\frac{1}{\gamma_\mathrm{g}} \biggr)\biggl[\tau_\mathrm{SSC}^2 \omega^2 + (4 - 3\gamma_\mathrm{g})\biggl(\frac{g_0}{g_\mathrm{SSC}}\biggr) \frac{1}{\chi_0} \biggr] x = 0 , </math>

where,

<math>~g_\mathrm{SSC} \equiv \frac{P_c}{R\rho_c} \, ,</math> and <math>~\tau_\mathrm{SSC} \equiv \biggl[\frac{R^2 \rho_c}{P_c}\biggr]^{1/2} \, .</math>

In a separate discussion, we showed that specifically for isolated, polytropic configurations, this linear adiabatic wave equation (LAWE) can be rewritten as,

<math>~0 </math>	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[\frac{4 - (n+1)V(\xi)}{\xi} \biggr] \frac{dx}{d\xi} + \biggl[\frac{\omega^2}{\gamma_g \theta} \biggl(\frac{n+1 }{4\pi G \rho_c} \biggr) - \biggl(3-\frac{4}{\gamma_g}\biggr) \cdot \frac{(n+1)V(x)}{\xi^2} \biggr] x </math>
	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[\frac{4}{\xi} - \frac{(n+1)}{\theta} \biggl(- \frac{d\theta}{d\xi}\biggr)\biggr] \frac{dx}{d\xi} + \frac{(n+1)}{\theta}\biggl[\frac{\sigma_c^2}{6\gamma_g } - \frac{\alpha}{\xi} \biggl(- \frac{d\theta}{d\xi}\biggr)\biggr] x \, ,</math>

where we have adopted the dimensionless frequency notation,

<math>~\sigma_c^2</math>

<math>~\equiv</math>

<math>~\frac{3\omega^2}{2\pi G \rho_c} \, .</math>

Here we focus on an analysis of the specific case of isolated, <math>~n=1</math> polytropic configurations, whose unperturbed equilibrium structure can be prescribed in terms of analytic functions. Our hope — as yet unfulfilled — is that we can discover an analytically prescribed eigenvector solution to the governing LAWE.

Search for Analytic Solutions to the LAWE

Setup

From our derived structure of an n = 1 polytrope, in terms of the configuration's radius <math>R</math> and mass <math>M</math>, the central pressure and density are, respectively,

<math>P_c = \frac{\pi G}{8}\biggl( \frac{M^2}{R^4} \biggr) </math> ,

and

<math>\rho_c = \frac{\pi M}{4 R^3} </math> .

Hence the characteristic time and acceleration are, respectively,

<math> \tau_\mathrm{SSC} = \biggl[ \frac{R^2 \rho_c}{P_c} \biggr]^{1/2} = \biggl[ \frac{2R^3 }{GM} \biggr]^{1/2} = \biggl[ \frac{\pi}{2 G\rho_c} \biggr]^{1/2}, </math>

and,

<math> g_\mathrm{SSC} = \frac{P_c}{R \rho_c} = \biggl( \frac{GM}{2R^2} \biggr) . </math>

The required functions are,

Density:

<math>\frac{\rho_0(\chi_0)}{\rho_c} = \frac{\sin(\pi\chi_0)}{\pi\chi_0} </math> ;

Pressure:

<math>\frac{P_0(\chi_0)}{P_c} = \biggl[ \frac{\sin(\pi\chi_0)}{\pi\chi_0} \biggr]^2 </math> ;

Gravitational acceleration:

<math> \frac{g_0(r_0)}{g_\mathrm{SSC}} = \frac{2}{\chi_0^2} \biggl[ \frac{M_r(\chi_0)}{M}\biggr] = \frac{2}{\pi \chi_0^2} \biggl[ \sin (\pi\chi_0 ) - \pi\chi_0 \cos (\pi\chi_0 ) \biggr]. </math>

So our desired Eigenvalues and Eigenvectors will be solutions to the following ODE:

<math> \frac{d^2x}{d\chi_0^2} + \frac{2}{\chi_0} \biggl[ 1 + \pi\chi_0 \cot (\pi\chi_0 ) \biggr] \frac{dx}{d\chi_0} + \frac{1}{\gamma_\mathrm{g}} \biggl\{ \frac{\pi \chi_0}{\sin(\pi\chi_0)} \biggl[ \frac{\pi \omega^2}{2G\rho_c} \biggr] + \frac{2}{\chi_0^2 } (4 - 3\gamma_\mathrm{g}) \biggl[ 1 - \pi\chi_0 \cot (\pi\chi_0 ) \biggr] \biggr\} x = 0 , </math>

or, replacing <math>\chi_0</math> with <math>\xi \equiv \pi\chi_0</math> and dividing the entire expression by <math>\pi^2</math>, we have,

<math> \frac{d^2x}{d\xi^2} + \frac{2}{\xi} \biggl[ 1 + \xi \cot \xi \biggr] \frac{dx}{d\xi} + \frac{1}{\gamma_\mathrm{g}} \biggl\{ \frac{\xi}{\sin \xi} \biggl[ \frac{\omega^2}{2\pi G\rho_c} \biggr] + \frac{2}{\xi^2 } (4 - 3\gamma_\mathrm{g}) \biggl[ 1 - \xi \cot \xi \biggr] \biggr\} x = 0 . </math>

This is identical to the formulation of the wave equation that is relevant to the (n = 1) core of the composite polytrope studied by J. O. Murphy & R. Fiedler (1985b); for comparison, their expression is displayed, here, in the following boxed-in image.

n = 1 Polytropic Formulation of Wave Equation as Presented by Murphy & Fiedler (1985b)

Material that appears after this point in our presentation is under development and therefore
may contain incorrect mathematical equations and/or physical misinterpretations.
| Go Home |

Attempt at Deriving an Analytic Eigenvector Solution

Multiplying the last expression through by <math>~\xi^2\sin\xi</math> gives,

<math> (\xi^2\sin\xi ) \frac{d^2x}{d\xi^2} + 2 \biggl[ \xi \sin\xi + \xi^2 \cos \xi \biggr] \frac{dx}{d\xi} + \biggl[ \sigma^2 \xi^3 - 2\alpha ( \sin\xi - \xi \cos \xi ) \biggr] x = 0 \, , </math>

where,

<math>~\sigma^2</math>	<math>~\equiv</math>	<math> ~\frac{\omega^2}{2\pi G\rho_c \gamma_g} \, , </math>
<math>~\alpha</math>	<math>~\equiv</math>	<math> ~3-\frac{4}{\gamma_g} \, . </math>

The first two terms can be folded together to give,

<math>~ \frac{1}{\xi^2 \sin^2\xi} \cdot \frac{d}{d\xi}\biggl[ \xi^2 \sin^2\xi \frac{dx}{d\xi} \biggr] </math>	<math>~=</math>	<math> ~\frac{1}{\xi^2 \sin\xi} \biggl[ 2\alpha ( \sin\xi - \xi \cos \xi ) - \sigma^2 \xi^3 \biggr] x </math>
	<math>~=</math>	<math> ~- \biggl[ \frac{2\alpha}{\xi^2} \biggl( \frac{\xi \cos \xi}{\sin\xi} -1\biggr) + \sigma^2 \biggl( \frac{\xi}{\sin\xi}\biggr) \biggr] x </math>
	<math>~=</math>	<math> ~- \biggl[ \frac{2\alpha}{\xi^2} \frac{\xi^2}{\sin\xi} \cdot \frac{d}{d\xi} \biggl( \frac{\sin\xi}{\xi} \biggr) + \sigma^2 \biggl( \frac{\xi}{\sin\xi}\biggr) \biggr] x </math>
	<math>~=</math>	<math> ~- \biggl[ \frac{2\alpha}{\xi} \frac{d}{d\xi}\biggl( \frac{\sin\xi}{\xi} \biggr) + \sigma^2 \biggr] \biggl( \frac{\xi}{\sin\xi}\biggr) x \, , </math>

where, in order to make this next-to-last step, we have recognized that,

<math>~ \frac{d}{d\xi} \biggl( \frac{\sin\xi}{\xi} \biggr) </math>

<math> ~\frac{\sin\xi}{\xi^2} \biggl[ \frac{\xi \cos\xi}{\sin\xi} - 1 \biggr] \, . </math>

It would seem that the eigenfunction, <math>~x(\xi)</math>, should be expressible in terms of trigonometric functions and powers of <math>~\xi</math>; indeed, it appears as though the expression governing this eigenfunction would simplify considerably if <math>~x \propto \sin\xi/\xi</math>. With this in mind, we have made some attempts to guess the exact form of the eigenfunction. Here is one such attempt.

First Guess (n1)

Let's try,

which means,

<math>~x^' \equiv \frac{dx}{d\xi}</math>

<math> ~\frac{\sin\xi}{\xi^2} \biggl[ \frac{\xi \cos\xi}{\sin\xi} - 1 \biggr] \, . </math>

Does this satisfy the governing expression? Let's see. The right-and-side (RHS) gives:

RHS

<math> ~- \biggl[ \frac{2\alpha}{\xi} \frac{d}{d\xi}\biggl( \frac{\sin\xi}{\xi} \biggr) + \sigma^2 \biggr] \biggl( \frac{\xi}{\sin\xi}\biggr) x = - \biggl[ \frac{2\alpha x^'}{\xi} + \sigma^2 \biggr] \, . </math>

At the same time, the left-hand-side (LHS) may, quite generically, be written as:

LHS	<math>~=</math>	<math>~ \frac{x^'}{\xi} \biggl\{ \frac{\xi}{(\xi^2 \sin^2\xi)x^'} \cdot \frac{d[ (\xi^2 \sin^2\xi)x^']}{d\xi} \biggr\} </math>
	<math>~=</math>	<math>~ \frac{x^'}{\xi} \biggl[\frac{d\ln[ (\xi^2 \sin^2\xi)x^']}{d\ln\xi} \biggr] \, . </math>

Putting the two sides together therefore gives,

<math>~ \frac{x^'}{\xi} \biggl[\frac{d\ln[ (\xi^2 \sin^2\xi)x^']}{d\ln\xi} +2\alpha \biggr] </math>	<math>~=</math>	<math> ~-\sigma^2 </math>
<math>~ \Rightarrow ~~~~~ \biggl[\frac{d\ln[ (\xi^2 \sin^2\xi)x^']^{1/(2\alpha)}}{d\ln\xi} +1 \biggr] </math>	<math>~=</math>	<math> ~- \frac{\sigma^2}{2\alpha } \biggl( \frac{\xi}{x^'} \biggr) </math>
<math>~ \Rightarrow ~~~~~ \frac{d\ln[ (\xi^2 \sin^2\xi)x^']^{-1/(2\alpha)}}{d\ln\xi} </math>	<math>~=</math>	<math> ~1 + \frac{\sigma^2}{2\alpha } \biggl( \frac{\xi}{x^'} \biggr) \, . </math>

[Comment from J. E. Tohline on 6 April 2015: I'm not sure what else to make of this.]

Second Guess (n1)

Adopting the generic rewriting of the LHS, and leaving the RHS fully generic as well, we have,

<math>~ \frac{x^'}{\xi} \biggl[\frac{d\ln[ (\xi^2 \sin^2\xi)x^']}{d\ln\xi} \biggr] </math>	<math>~=</math>	<math> ~- \biggl[ \frac{2\alpha}{\xi} \frac{d}{d\xi}\biggl( \frac{\sin\xi}{\xi} \biggr) + \sigma^2 \biggr] \biggl( \frac{\xi}{\sin\xi}\biggr) x </math>
<math>~\Rightarrow ~~~~~ \frac{x^'}{x} \biggl[\frac{d\ln[ (\xi^2 \sin^2\xi)x^']}{d\ln\xi} \biggr] </math>	<math>~=</math>	<math> ~- 2\alpha\biggl( \frac{\xi}{\sin\xi}\biggr) \frac{d}{d\xi}\biggl( \frac{\sin\xi}{\xi} \biggr) ~- \sigma^2\biggl( \frac{\xi^2}{\sin\xi}\biggr) </math>
<math>~\Rightarrow ~~~~~ \frac{d\ln(x)}{d\ln \xi} \biggl[\frac{d\ln[ (\xi^2 \sin^2\xi)x^']}{d\ln\xi} \biggr] </math>	<math>~=</math>	<math> ~- 2\alpha\biggl[ \frac{d\ln(\sin\xi/\xi)}{d\ln \xi} \biggr] ~- \sigma^2\biggl( \frac{\xi^3}{\sin\xi}\biggr) \, . </math>
<math> ~\Rightarrow ~~~~ - \sigma^2 </math>	<math>~=</math>	<math>~ \biggl( \frac{\sin\xi}{\xi^3}\biggr) \biggl\{\frac{d\ln(x)}{d\ln \xi} \biggl[\frac{d\ln[ (\xi^2 \sin^2\xi)x^']}{d\ln\xi} \biggr] + 2\alpha\biggl[ \frac{d\ln(\sin\xi/\xi)}{d\ln \xi} \biggr] \biggr\} \, . </math>

[Comment from J. E. Tohline on 6 April 2015: I'm not sure what else to make of this.]

Third Guess (n1)

Let's rewrite the polytropic (n = 1) wave equation as follows:

<math> ~\sin\xi \biggl[ \xi^2 x^{} + 2\xi x^' - 2\alpha x \biggr] + \cos\xi \biggl[ 2\xi^2 x^' + 2\alpha \xi x \biggr] +\sigma^2 \xi^3 x = 0 \, . </math>

It is difficult to determine what term in the adiabatic wave equation will cancel the term involving <math>~\sigma^2</math> because its leading coefficient is <math>~\xi^3</math> and no other term contains a power of <math>~\xi</math> that is higher than two. After thinking through various trial eigenvector expressions, <math>~x(\xi)</math>, I have determined that a function of the following form has a chance of working because the second derivative of the function generates a leading factor of <math>~\xi^3</math> while the function itself does not introduce any additional factors of <math>~\xi</math> into the term that contains <math>~\sigma^2</math>:

<math>~x</math>	<math>~=</math>	<math> ~[a\sin^2(\xi^{5/2}) + b\sin(\xi^{5/2})\cos(\xi^{5/2}) + c\cos^2(\xi^{5/2})] [ A\sin\xi + B\cos\xi] </math>
<math>~\Rightarrow ~~~ x^'</math>	<math>~=</math>	<math> ~[a\sin^2(\xi^{5/2}) + b\sin(\xi^{5/2})\cos(\xi^{5/2}) + c\cos^2(\xi^{5/2})] \cdot \frac{d[ A\sin\xi + B\cos\xi]}{d\xi} </math>
		<math> ~+ \frac{d[a\sin^2(\xi^{5/2}) + b\sin(\xi^{5/2})\cos(\xi^{5/2}) + c\cos^2(\xi^{5/2})]}{d\xi} \cdot [ A\sin\xi + B\cos\xi] </math>
	<math>~=</math>	<math> ~[a\sin^2(\xi^{5/2}) + b\sin(\xi^{5/2})\cos(\xi^{5/2}) + c\cos^2(\xi^{5/2})] \cdot \frac{d[ A\sin\xi + B\cos\xi]}{d\xi} </math>
		<math> ~+ [ A\sin\xi + B\cos\xi] \biggl\{5a \xi^{3/2} \sin(\xi^{5/2}) \cos(\xi^{5/2}) + b\biggl[ \frac{5}{2} \xi^{3/2}\cos^2(\xi^{5/2}) - \frac{5}{2} \xi^{3/2}\sin^2(\xi^{5/2}) \biggr] - 5c \xi^{3/2} \sin(\xi^{5/2}) \cos(\xi^{5/2}) \biggr\} </math>
	<math>~=</math>	<math> ~[a\sin^2(\xi^{5/2}) + b\sin(\xi^{5/2})\cos(\xi^{5/2}) + c\cos^2(\xi^{5/2})] \cdot \frac{d[ A\sin\xi + B\cos\xi]}{d\xi} </math>
		<math> ~+ [ A\sin\xi + B\cos\xi] \biggl\{5(a-c) \xi^{3/2} \sin(\xi^{5/2}) \cos(\xi^{5/2}) + \frac{5b}{2} \xi^{3/2}\biggl[ 1 - 2\sin^2(\xi^{5/2}) \biggr] \biggr\} </math>
<math>~\Rightarrow ~~~ x^{}</math>	<math>~=</math>	<math> ~[a\sin^2(\xi^{5/2}) + b\sin(\xi^{5/2})\cos(\xi^{5/2}) + c\cos^2(\xi^{5/2})] \cdot \frac{d^2[ A\sin\xi + B\cos\xi]}{d^2\xi} </math>
		<math>~+ \biggl\{5(a-c) \xi^{3/2} \sin(\xi^{5/2}) \cos(\xi^{5/2}) + \frac{5b}{2} \xi^{3/2}\biggl[ 1 - 2\sin^2(\xi^{5/2}) \biggr] \biggr\} \cdot \frac{d[ A\sin\xi + B\cos\xi]}{d\xi} </math>
		<math> ~+ [ A\sin\xi + B\cos\xi] \biggl\{ \frac{15}{2}(a-c) \xi^{1/2} \sin(\xi^{5/2}) \cos(\xi^{5/2}) +\frac{25}{2}(a-c) \xi^{3} \cos^2(\xi^{5/2}) - \frac{25}{2}(a-c) \xi^{3} \sin^2(\xi^{5/2}) </math>
		<math> + \frac{15b}{4} \xi^{1/2}\biggl[ 1 - 2\sin^2(\xi^{5/2}) \biggr] - 25b \xi^{3}\sin(\xi^{5/2}) \cos(\xi^{5/2}) \biggr\} </math>
	<math>~=</math>	<math> ~[a\sin^2(\xi^{5/2}) + b\sin(\xi^{5/2})\cos(\xi^{5/2}) + c\cos^2(\xi^{5/2})] \cdot \frac{d^2[ A\sin\xi + B\cos\xi]}{d^2\xi} </math>
		<math>~+ \biggl\{5(a-c) \xi^{3/2} \sin(\xi^{5/2}) \cos(\xi^{5/2}) + \frac{5b}{2} \xi^{3/2}\biggl[ 1 - 2\sin^2(\xi^{5/2}) \biggr] \biggr\} \cdot \frac{d[ A\sin\xi + B\cos\xi]}{d\xi} </math>
		<math> ~+ [ A\sin\xi + B\cos\xi] \biggl\{ \frac{15}{4}\xi^{1/2} \biggl[ 2(a-c) \sin(\xi^{5/2}) \cos(\xi^{5/2}) + b\biggl( 1 - 2\sin^2(\xi^{5/2}) \biggr) \biggr] </math>
		<math>~+ \frac{25}{2} \xi^3 \biggl[ - 2b \sin(\xi^{5/2}) \cos(\xi^{5/2}) +(a-c) \biggl( 1- 2\sin^2(\xi^{5/2}) \biggr) \biggr] \biggr\} </math>

[Comment from J. E. Tohline on 9 April 2015: I'm not sure what else to make of this.]

[Additional comment from J. E. Tohline on 15 April 2015: It is perhaps worth mentioning that there is a similarity between the argument of the trigonometric function being used in this "third guess" and the Lane-Emden function derived by Srivastava for <math>~n=5</math> polytropes; and also a similarity between Srivastava's function and the functional form of the LHS that we constructed, above, in connection with our "second guess."]

Fourth Guess (n1)

Again, working with the polytropic (n = 1) wave equation written in the following form,

<math> ~\sin\xi \biggl[ \xi^2 x^{} + 2\xi x^' - 2\alpha x \biggr] + \cos\xi \biggl[ 2\xi^2 x^' + 2\alpha \xi x \biggr] +\sigma^2 \xi^3 x = 0 \, . </math>

Now, let's try:

which means,

<math>~x^' </math>	<math>~=</math>	<math> ~b_1 \sin\xi + b_1 \xi \cos\xi + 2c_2 \xi \cos\xi - c_2\xi^2 \sin\xi </math>
	<math>~=</math>	<math> ~(b_1 - c_2\xi^2 ) \sin\xi + (b_1 + 2c_2)\xi \cos\xi \, , </math>
<math>~x^{} </math>	<math>~=</math>	<math> ~(- 2c_2\xi ) \sin\xi + (b_1 - c_2\xi^2 ) \cos\xi + (b_1 + 2c_2 )\cos\xi - (b_1 + 2c_2 )\xi \sin\xi </math>
	<math>~=</math>	<math> ~-(2c_2+b_1 + 2c_2 ) \xi \sin\xi + (2b_1 + 2c_2 - c_2\xi^2 ) \cos\xi \, . </math>

The LHS of the wave equation then becomes,

LHS	<math>~=</math>	<math> ~\sin\xi \biggl\{ \xi^2 \biggl[ -(2c_2+b_1 + 2c_2 ) \xi \sin\xi + (2b_1 + 2c_2 - c_2\xi^2 ) \cos\xi \biggr] + 2\xi \biggl[ (b_1 - c_2\xi^2 ) \sin\xi + (b_1 + 2c_2)\xi \cos\xi \biggr] - 2\alpha \biggl[ a_0 + (b_1 \xi) \sin\xi + (c_2 \xi^2) \cos\xi \biggr] \biggr\} </math>
		<math> + \cos\xi \biggl\{ 2\xi^2 \biggl[ (b_1 - c_2\xi^2 ) \sin\xi + (b_1 + 2c_2)\xi \cos\xi \biggr] + 2\alpha \xi \biggl[ a_0 + (b_1 \xi) \sin\xi + (c_2 \xi^2) \cos\xi \biggr] \biggr\} +\sigma^2 \xi^3 \biggl[ a_0 + b_1 \xi \sin\xi + c_2 \xi^2 \cos\xi \biggr] </math>
	<math>~=</math>	<math> ~\sin\xi \biggl\{ \biggl[ -(2c_2+b_1 + 2c_2 ) \xi^3 \sin\xi + (2b_1 + 2c_2 - c_2\xi^2 ) \xi^2\cos\xi \biggr] + \biggl[ 2(b_1 - c_2\xi^2 )\xi \sin\xi + 2(b_1 + 2c_2)\xi^2 \cos\xi \biggr] - 2\alpha \biggl[ a_0 + (b_1 \xi) \sin\xi + (c_2 \xi^2) \cos\xi \biggr] \biggr\} </math>
		<math> + \cos\xi \biggl\{ \biggl[ 2(b_1 - c_2\xi^2 )\xi^2 \sin\xi + 2(b_1 + 2c_2)\xi^3 \cos\xi \biggr] + \biggl[ 2a_0\alpha \xi + 2b_1\alpha \xi^2 \sin\xi + 2c_2 \alpha \xi^3 \cos\xi \biggr] \biggr\} +\sigma^2 \biggl[ a_0\xi^3 + b_1 \xi^4 \sin\xi + c_2 \xi^5 \cos\xi \biggr] </math>
	<math>~=</math>	<math> ~\sin\xi \biggl\{- 2\alpha a_0 + \biggl[ -(2c_2+b_1 + 2c_2 ) \xi^3 + 2(b_1 - c_2\xi^2 )\xi - 2\alpha (b_1 \xi) \biggr]\sin\xi + \biggl[ (2b_1 + 2c_2 - c_2\xi^2 ) \xi^2 + 2(b_1 + 2c_2)\xi^2 - 2\alpha (c_2 \xi^2) \biggr] \cos\xi \biggr\} </math>
		<math> + \cos\xi \biggl\{ + 2a_0\alpha \xi + \biggl[ 2(b_1 - c_2\xi^2 )\xi^2 + 2b_1\alpha \xi^2 \biggr] \sin\xi + \biggl[ 2(b_1 + 2c_2)\xi^3 + 2c_2 \alpha \xi^3\biggr] \cos\xi \biggr\} +\sigma^2 \biggl[ a_0\xi^3 + b_1 \xi^4 \sin\xi + c_2 \xi^5 \cos\xi \biggr] </math>
	<math>~=</math>	<math> ~\sigma^2 a_0 \xi^3 + \biggl[ -(2c_2+b_1 + 2c_2 ) \xi^3 + 2(b_1 - c_2\xi^2 )\xi - 2\alpha (b_1 \xi) \biggr]\sin^2\xi + \biggl[ 2(b_1 + 2c_2)\xi^3 + 2c_2 \alpha \xi^3\biggr] \biggl(1-\sin^2\xi \biggr) </math>
		<math> + \biggl[ (2b_1 + 2c_2 - c_2\xi^2 ) \xi^2 + 2(b_1 + 2c_2)\xi^2 - 2\alpha (c_2 \xi^2) \biggr] \sin\xi \cos\xi + \biggl[ 2(b_1 - c_2\xi^2 )\xi^2 + 2b_1\alpha \xi^2 \biggr] \sin\xi \cos\xi </math>
		<math> +\sigma^2 \biggl[ b_1 \xi^4 \sin\xi + c_2 \xi^5 \cos\xi \biggr] + 2a_0\alpha \xi\cos\xi - 2\alpha a_0 \sin\xi </math>
	<math>~=</math>	<math> ~\biggl[ \sigma^2 a_0 + 2(b_1 + 2c_2) + 2c_2 \alpha \biggr]\xi^3 + \biggl\{+ 2(b_1 )\xi - 2\alpha (b_1 \xi) +[-2c_2 - 2(b_1 + 2c_2) - 2c_2 \alpha -(2c_2+b_1 + 2c_2 )] \xi^3 \biggr\} \sin^2\xi </math>
		<math> + \biggl\{ [ (2b_1 + 2c_2 ) + 2(b_1 + 2c_2) - 2\alpha (c_2 ) + 2(b_1 ) + 2b_1\alpha ] \xi^2 -3c_2\xi^4 \biggr\} \sin\xi \cos\xi </math>
		<math>~+ \sin\xi \biggl[\sigma^2b_1 \xi^4 - 2\alpha a_0 \biggr] + \xi \cos\xi \biggl[\sigma^2 c_2 \xi^4 + 2a_0\alpha \biggr] </math>
	<math>~=</math>	<math> ~[ \sigma^2 a_0 + 2(b_1 + 2c_2) + 2c_2 \alpha ]\xi^3 + \biggl\{2 b_1(1-\alpha) - [2c_2(5+\alpha) + 3b_1] \xi^2 \biggr\} \xi \sin^2\xi </math>
		<math> + \biggl\{ 2(3-\alpha)( b_1+c_2 ) -3c_2\xi^2 \biggr\} \xi^2 \sin\xi \cos\xi +\sin\xi \biggl[\sigma^2b_1 \xi^4 - 2\alpha a_0 \biggr] + \xi \cos\xi \biggl[\sigma^2 c_2 \xi^4 + 2a_0\alpha \biggr] \, . </math>

Fifth Guess (n1)

Along a similar line of reasoning, let's try a function of the form,

where <math>~x_s, x_c, x_1, x_2,</math> and <math>~x_3</math> are five separate, as yet, unspecified (polynomial?) functions of <math>~\xi</math>. This also means that,

and,

<math>~x^{}</math>

Hence the LHS of the polytropic (n = 1) wave equation becomes,

LHS	<math>~=</math>	<math> ~~\sin\xi \biggl\{ \xi^2 \biggl[~(x_s^{} - 2x_c^{'} - x_s)\sin\xi + (x_c^{} + 2x_s^' -x_c)\cos\xi + (x_1^{} -2x_3^' -2x_1 + 2x_2)\sin^2\xi + (x_2^{} + 2x_3^'+ 2x_1 - 2x_2)\cos^2\xi + (x_3^{} + 4x_1^' -4x_2^' - 4x_3)\sin\xi \cos\xi \biggr] </math>
		<math> + 2\xi \biggl[~(x_s^' - x_c)\sin\xi + (x_c^' + x_s)\cos\xi + (x_1^' - x_3)\sin^2\xi + (x_2^' + x_3)\cos^2\xi + (x_3^' + 2x_1 -2x_2)\sin\xi \cos\xi \biggr] </math>
		<math> - 2\alpha \biggl[ x_s \sin\xi + x_c \cos\xi + x_1 \sin^2\xi + x_2 \cos^2\xi + x_3 \sin\xi \cos\xi\biggr] \biggr\} </math>
		<math> + \cos\xi \biggl\{ 2\xi^2 \biggl[~(x_s^' - x_c)\sin\xi + (x_c^' + x_s)\cos\xi + (x_1^' - x_3)\sin^2\xi + (x_2^' + x_3)\cos^2\xi + (x_3^' + 2x_1 -2x_2)\sin\xi \cos\xi \biggr] </math>
		<math> + 2\alpha \xi \biggl[ x_s \sin\xi + x_c \cos\xi + x_1 \sin^2\xi + x_2 \cos^2\xi + x_3 \sin\xi \cos\xi\biggr] \biggr\} </math>
		<math> +\sigma^2 \xi^3 \biggl\{ x_s \sin\xi + x_c \cos\xi + x_1 \sin^2\xi + x_2 \cos^2\xi + x_3 \sin\xi \cos\xi \biggr\} </math>
	<math>~=</math>	<math> ~\biggl[(x_s^{} - 2x_c^{'} - x_s)\xi^2 + 2\xi (x_s^' - x_c) -2\alpha x_s + \sigma^2 \xi^3 x_1 \biggr]\sin^2\xi + \biggl[(x_c^{} + 2x_s^' -x_c)\xi^2 + 2\xi (x_c^' + x_s) - 2\alpha x_c + 2\xi^2 (x_s^' - x_c) + 2\alpha \xi x_s + \sigma^2 \xi^3 x_3 \biggr] \sin\xi \cos\xi </math>
		<math> +\biggl[ (x_1^{} -2x_3^' -2x_1 + 2x_2)\xi^2 + 2\xi (x_1^' - x_3) - 2\alpha x_1 \biggr] \sin^3\xi + \biggl[(x_2^{} + 2x_3^'+ 2x_1 - 2x_2)\xi^2 + 2\xi (x_2^' + x_3) - 2\alpha x_2 + 2\xi^2 (x_3^' + 2x_1 -2x_2)+ 2\alpha \xi x_3 \biggr]\sin\xi \cos^2\xi </math>
		<math> + \biggl[(x_3^{} + 4x_1^' -4x_2^' - 4x_3)\xi^2 + 2\xi (x_3^' + 2x_1 -2x_2) - 2\alpha x_3 + 2\xi^2 (x_1^' - x_3) + 2\alpha \xi x_1 \biggr] \sin^2\xi \cos\xi </math>
		<math> + \biggl[2\xi^2 (x_c^' + x_s ) + 2\alpha \xi x_c + \sigma^2 \xi^3 x_2 \biggr]\cos^2\xi + \biggl[2\xi^2 (x_2^' + x_3)+ 2\alpha \xi x_2 \biggr]\cos^3\xi + \sigma^2 \xi^3 x_s \sin\xi + \sigma^2 \xi^3 x_c \cos\xi </math>
	<math>~=</math>	<math> ~\biggl[(x_s^{} - 2x_c^{'} - x_s)\xi^2 + 2\xi (x_s^' - x_c) -2\alpha x_s + \sigma^2 \xi^3 x_1 \biggr]\sin^2\xi + \biggl[(x_c^{} + 2x_s^' -x_c)\xi^2 + 2\xi (x_c^' + x_s) - 2\alpha x_c + 2\xi^2 (x_s^' - x_c) + 2\alpha \xi x_s + \sigma^2 \xi^3 x_3 \biggr] \sin\xi \cos\xi </math>
		<math> +\biggl[ (x_1^{} -2x_3^' -2x_1 + 2x_2)\xi^2 + 2\xi (x_1^' - x_3) - 2\alpha x_1 + \sigma^2 \xi^3 x_s \biggr] \sin\xi </math>
		<math> + \biggl[(x_2^{} + 2x_3^'+ 2x_1 - 2x_2)\xi^2 + 2\xi (x_2^' + x_3) - 2\alpha x_2 + 2\xi^2 (x_3^' + 2x_1 -2x_2)+ 2\alpha \xi x_3 - (x_1^{} -2x_3^' -2x_1 + 2x_2)\xi^2 - 2\xi (x_1^' - x_3) + 2\alpha x_1 \biggr]\sin\xi \cos^2\xi </math>
		<math> + \biggl[(x_3^{} + 4x_1^' -4x_2^' - 4x_3)\xi^2 + 2\xi (x_3^' + 2x_1 -2x_2) - 2\alpha x_3 + 2\xi^2 (x_1^' - x_3) + 2\alpha \xi x_1 -2\xi^2 (x_2^' + x_3) - 2\alpha \xi x_2 \biggr] \sin^2\xi \cos\xi </math>
		<math> + \biggl[2\xi^2 (x_c^' + x_s ) + 2\alpha \xi x_c + \sigma^2 \xi^3 x_2 \biggr]\cos^2\xi + \biggl[2\xi^2 (x_2^' + x_3)+ 2\alpha \xi x_2 + \sigma^2 \xi^3 x_c \biggr]\cos\xi </math>

So, the five chosen (polynomial?) functions of <math>~\xi</math> must simultabeously satisfy the following, seven 2^nd-order ODEs:

<math>~\sin\xi </math>	:	<math>~(x_1^{} -2x_3^' -2x_1 + 2x_2)\xi^2 + 2\xi (x_1^' - x_3) - 2\alpha x_1 + \sigma^2 \xi^3 x_s =0</math>
<math>~\sin^2 \xi</math>	:	<math>~(x_s^{} - 2x_c^{'} - x_s)\xi^2 + 2\xi (x_s^' - x_c) -2\alpha x_s + \sigma^2 \xi^3 x_1 =0</math>
<math>~\sin^2\xi \cos\xi</math>	:	<math>~(x_3^{} + 4x_1^' -4x_2^' - 4x_3)\xi^2 + 2\xi (x_3^' + 2x_1 -2x_2) - 2\alpha x_3 + 2\xi^2 (x_1^' - x_3) + 2\alpha \xi x_1 -2\xi^2 (x_2^' + x_3) - 2\alpha \xi x_2 =0</math>
<math>~\sin\xi \cos\xi</math>	:	<math>~(x_c^{} + 2x_s^' -x_c)\xi^2 + 2\xi (x_c^' + x_s) - 2\alpha x_c + 2\xi^2 (x_s^' - x_c) + 2\alpha \xi x_s + \sigma^2 \xi^3 x_3 =0</math>
<math>~\sin\xi \cos^2\xi</math>	:	<math>~(x_2^{} + 2x_3^'+ 2x_1 - 2x_2)\xi^2 + 2\xi (x_2^' + x_3) - 2\alpha x_2 + 2\xi^2 (x_3^' + 2x_1 -2x_2)+ 2\alpha \xi x_3 - (x_1^{} -2x_3^' -2x_1 + 2x_2)\xi^2 - 2\xi (x_1^' - x_3) + 2\alpha x_1 =0</math>
<math>~\cos^2\xi</math>	:	<math>~2\xi^2 (x_c^' + x_s ) + 2\alpha \xi x_c + \sigma^2 \xi^3 x_2 =0</math>
<math>~\cos\xi</math>	:	<math>~2\xi^2 (x_2^' + x_3)+ 2\alpha \xi x_2 + \sigma^2 \xi^3 x_c =0</math>

Example 1

Let's work on the coefficient of the <math>~\cos\xi</math> term:

<math>~x_c</math>	<math>~=</math>	<math>~\xi^\beta (A_c)</math>
<math>~x_2</math>	<math>~=</math>	<math>~\xi^\beta (C_2 \xi^2)</math>
<math>~x_3</math>	<math>~=</math>	<math>~\xi^\beta (B_3 \xi)</math>
<math>~\Rightarrow~</math> Coefficient of "<math>~\cos\xi</math>" term	<math>~=</math>	<math>~\xi^\beta [2\xi^2 (2C_2\xi + (B_3 \xi))+ 2\alpha \xi (C_2 \xi^2) + \sigma^2 \xi^3 (A_c)]</math>
	<math>~=</math>	<math>~\xi^{\beta+3} [2(2C_2 + B_3 )+ 2\alpha C_2 + \sigma^2 (A_c)]</math>
<math>~\Rightarrow ~~~~ \sigma^2</math>	<math>~=</math>	<math>~- \frac{2}{A_c} \biggl[B_3 + (2+\alpha) C_2 \biggr]</math>

Sixth Guess (n1)

Rationale

From our review of the properties of <math>~n=1</math> polytropic spheres, we know that the equilibrium density distribution is given by the sinc function, namely,

where,

<math>~\xi \equiv \pi \biggl(\frac{r_0}{R_0} \biggr) \, .</math>

The total mass is,

<math>~M_\mathrm{tot} = \frac{4}{\pi} \cdot \rho_c R_0^3 \, ,</math>

and the fractional mass enclosed within a given radius, <math>~r</math>, is,

<math>~\frac{M_r(\xi)}{M_\mathrm{tot}}</math>

Let's guess that, during the fundamental mode of radial oscillation, the sinc-function profile is preserved as the system's total radius varies. In particular, we will assume that the system's time-varying radius is,

<math>R = R_0 \biggl( 1 + \frac{\delta R}{R_0} \biggr) = R_0 ( 1 + \epsilon_R) \, ,</math>

and seek to determine how the displacement vector, <math>~\epsilon \equiv \delta r/r_0</math>, varies with <math>~r_0</math> in order to preserve the overall sinc-function profile. As is usual, we will only examine small perturbations away from equilibrium, that is, we will assume that everywhere throughout the configuration, <math>~|\epsilon| \ll 1 </math>.

Let's begin by defining a new dimensionless coordinate,

<math>~\eta \equiv \pi \biggl(\frac{r}{R} \biggr) = \pi \biggl[\frac{r_0(1+\epsilon)}{R_0(1+\epsilon_R)} \biggr] \approx \xi (1 + \epsilon) \, ,</math>

and recognize that, in the new perturbed state, the fractional mass enclosed within a given radius, <math>~r</math>, is,

<math>~\frac{M_r(\eta)}{M_\mathrm{tot}}</math>

In order to associate each mass shell in the perturbed configuration with its corresponding mass shell in the unperturbed, equilibrium state, we need to set the two <math>~M_r</math> functions equal to one another, that is, demand that,

<math>~\sin\xi - \xi \cos\xi</math>	<math>~=</math>	<math>~\sin\eta - \eta \cos\eta</math>
	<math>~\approx</math>	<math>~\sin[\xi(1+\epsilon)] - \xi(1+\epsilon) \cos[\xi(1+\epsilon)]</math>
	<math>~=</math>	<math>~\biggl[ \sin\xi \cos(\xi\epsilon) + \cos\xi \sin (\xi\epsilon) \biggr] - \xi(1+\epsilon) \biggl[ \cos\xi \cos(\xi\epsilon) - \sin\xi \sin (\xi\epsilon) \biggr]</math>
	<math>~\approx</math>	<math>~\sin\xi \biggl[1 - \frac{1}{2}(\xi\epsilon)^2 \biggr] + (\xi\epsilon)\cos\xi - \xi(1+\epsilon) \cos\xi \biggl[1 - \frac{1}{2}(\xi\epsilon)^2 \biggr] + \xi^2 \epsilon(1+\epsilon) \sin\xi </math>
	<math>~\approx</math>	<math> ~\sin\xi -\xi\cos\xi - \frac{1}{2}(\xi\epsilon)^2 \sin\xi + (\xi\epsilon)\cos\xi - (\xi \epsilon) \cos\xi + \frac{1}{2} \xi^3 \epsilon^2\cos\xi + \xi^2 \epsilon \sin\xi + (\xi \epsilon)^2\sin\xi </math>
<math>~\Rightarrow~~~~- \xi^2 \epsilon \sin\xi </math>	<math>~\approx</math>	<math>~ \frac{(\xi \epsilon)^2}{2} \biggl[\xi \cos\xi+ \sin\xi \biggr] </math>
<math>~\Rightarrow~~~~\frac{1}{\epsilon}</math>	<math>~\approx</math>	<math>~- \frac{1}{2} \biggl[\xi \cdot \frac{\cos\xi}{\sin\xi} + 1 \biggr] </math>
<math>~\Rightarrow~~~~\epsilon</math>	<math>~\approx</math>	<math>~- 2\biggl[1 + \xi \cdot \frac{\cos\xi}{\sin\xi} \biggr]^{-1} = - 2\sin\xi \biggl[\sin\xi + \xi \cos\xi \biggr]^{-1} \, . </math>

Resulting Polytropic Wave Equation

So, let's try,

<math>~x</math>	<math>~=</math>	<math>~ 2\sin\xi \biggl[\sin\xi + \xi \cos\xi \biggr]^{-1} </math>
	<math>~=</math>	<math>~ \biggl[\sin\xi + \xi \cos\xi \biggr]^{-3} 2\sin\xi \biggl[\sin^2\xi + 2\xi \sin\xi \cos\xi + \xi^2 \cos^2\xi \biggr] \, , </math>

in which case,

<math>~x^'</math>	<math>~=</math>	<math>~ 2\cos\xi \biggl[\sin\xi + \xi \cos\xi \biggr]^{-1} -2\sin\xi \biggl[\sin\xi + \xi \cos\xi \biggr]^{-2}\biggl[2\cos\xi - \xi \sin\xi \biggr] </math>
	<math>~=</math>	<math>~\biggl[\sin\xi + \xi \cos\xi \biggr]^{-2}\biggl\{ 2\cos\xi \biggl[\sin\xi + \xi \cos\xi \biggr] -2\sin\xi \biggl[2\cos\xi - \xi \sin\xi \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\biggl[\sin\xi + \xi \cos\xi \biggr]^{-2}\biggl[ 2\cos\xi \sin\xi + 2\xi \cos^2\xi -4\sin\xi \cos\xi + 2\xi \sin^2\xi \biggr] </math>
	<math>~=</math>	<math>~ 2\biggl[\sin\xi + \xi \cos\xi \biggr]^{-2}\biggl[\xi-\sin\xi \cos\xi \biggr] </math>
	<math>~=</math>	<math>~ \biggl[\sin\xi + \xi \cos\xi \biggr]^{-3} 2 \biggl[\xi \sin\xi + \xi^2 \cos\xi -\sin^2\xi \cos\xi - \xi \sin\xi \cos^2\xi \biggr] \, , </math>

and,

<math>~x^{}</math>	<math>~=</math>	<math>~2 \biggl[\sin\xi + \xi \cos\xi \biggr]^{-2}\biggl[1-\cos^2\xi + \sin^2\xi \biggr] - 4\biggl[\sin\xi + \xi \cos\xi \biggr]^{-3}\biggl[\xi-\sin\xi \cos\xi \biggr]\biggl[2\cos\xi - \xi\sin\xi \biggr] </math>
	<math>~=</math>	<math>~4 \biggl[\sin\xi + \xi \cos\xi \biggr]^{-3}\biggl\{ \sin^2\xi \biggl[\sin\xi + \xi \cos\xi \biggr] - \biggl[\xi-\sin\xi \cos\xi \biggr]\biggl[2\cos\xi - \xi\sin\xi \biggr] \biggr\} </math>
	<math>~=</math>	<math>~4 \biggl[\sin\xi + \xi \cos\xi \biggr]^{-3}\biggl[ \sin^3\xi + \xi \sin\xi \cos\xi + \xi^2 \sin\xi -2\xi \cos\xi - \xi\sin^2\xi \cos\xi + 2\sin\xi \cos^2\xi \biggr] </math>

Graphical Reassessment

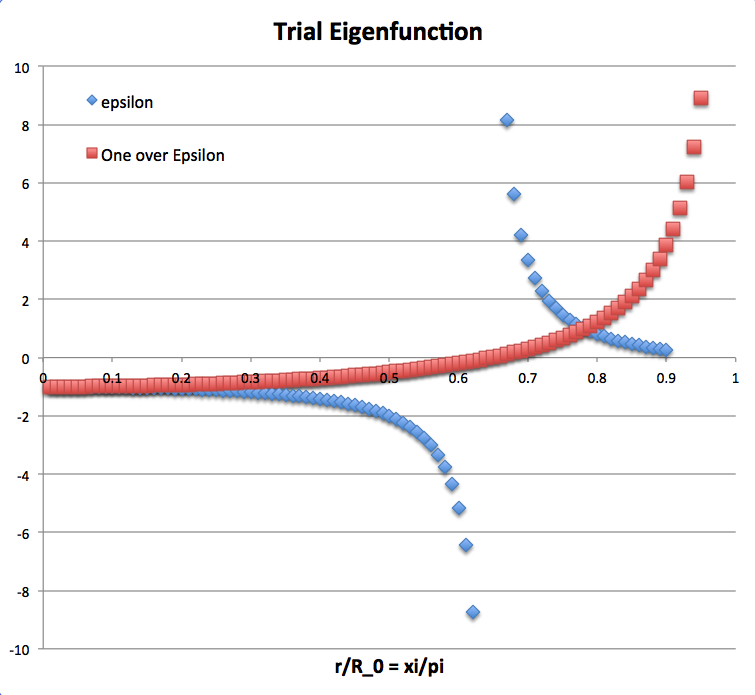
Before plowing ahead and plugging these expressions into the polytropic wave equation, I plotted the trial eigenfunction, <math>~\epsilon(\xi/\pi)</math> (see the blue curve in the accompanying "Trial Eigenfunction" figure), and noticed that it passes through <math>~\pm \infty</math> midway through the configuration. This is a very unphysical behavior. On the other hand, the inverse of this function (see the red curve) exhibits a relatively desirable behavior because it increases monotonically from negative one at the center. As plotted, however, the function has one node. In searching for the eigenfunction of the fundamental mode of oscillation, it might be better to add "1" to the inverse of the function and thereby get rid of all nodes. (Keep in mind, however, that the red curve might be displaying the eigenfunction associated with the first overtone.)

Let's therefore try,

<math>~x = 1 + \frac{1}{\epsilon} = 1 - \frac{1}{2} \biggl[\xi \cdot \frac{\cos\xi}{\sin\xi} + 1 \biggr] = \frac{1}{2} \biggl[1- \xi \cdot \frac{\cos\xi}{\sin\xi} \biggr] \, .</math>

In this case we have,

<math>~x^'</math>	<math>~=</math>	<math>~ \frac{1}{2}\biggl[\xi - \frac{\cos\xi}{\sin\xi} + \xi \cdot \frac{\cos^2\xi}{\sin^2\xi} \biggr] </math>
	<math>~=</math>	<math>~ \frac{1}{2\sin^2\xi}\biggl[\xi \sin^2\xi - \sin\xi \cos\xi + \xi \cos^2\xi \biggr] \, , </math>
	<math>~=</math>	<math>~ \frac{1}{2\sin^2\xi}\biggl[\xi - \sin\xi \cos\xi \biggr] \, , </math>

and,

<math>~x^{}</math>	<math>~=</math>	<math>~ - \frac{\cos\xi}{\sin^3\xi}\biggl[\xi - \sin\xi \cos\xi \biggr] + \frac{1}{2\sin^2\xi}\biggl[1 - \cos^2\xi + \sin^2\xi \biggr] </math>
	<math>~=</math>	<math>~ 1 - \frac{\cos\xi}{\sin^3\xi}\biggl[\xi - \sin\xi \cos\xi \biggr] \, . </math>

Now let's plug these expressions into the polytropic (n = 1) wave equation, namely,

<math>~-\sigma^2 \xi^3 x</math>

<math>~ \sin\xi \biggl[ \xi^2 x^{} + 2\xi x^' - 2\alpha x \biggr] + \cos\xi \biggl[ 2\xi^2 x^' + 2\alpha \xi x \biggr] \, . </math>

The first term inside the square brackets on the right-hand-side gives,

<math>~\xi^2 x^{} + 2\xi x^' - 2\alpha x </math>	<math>~=</math>	<math>~ \xi^2 - \frac{\cos\xi}{\sin^3\xi}\biggl(\xi^3 - \xi^2\sin\xi \cos\xi \biggr) + \frac{1}{\sin^2\xi}\biggl(\xi^2 - \xi \sin\xi \cos\xi \biggr) - \alpha \biggl(1- \xi \cdot \frac{\cos\xi}{\sin\xi} \biggr) </math>
	<math>~=</math>	<math>~ \frac{1}{\sin^3\xi} \biggl[ \xi^2 \sin^3\xi - \cos\xi (\xi^3 - \xi^2\sin\xi \cos\xi ) + \sin\xi (\xi^2 - \xi \sin\xi \cos\xi ) - \alpha (\sin^3\xi - \xi \cos\xi \sin^2\xi ) \biggr] </math>
	<math>~=</math>	<math>~ \frac{1}{\sin^3\xi} \biggl[ \xi^2 \sin\xi (1-\cos^2\xi) + \xi^2\sin\xi \cos^2\xi - \xi^3 \cos\xi + \xi^2\sin\xi - \xi \sin^2\xi \cos\xi - \alpha \sin^3\xi + \alpha \xi \cos\xi \sin^2\xi \biggr] </math>
	<math>~=</math>	<math>~ - \alpha + \frac{1}{\sin^3\xi} \biggl[ 2\xi^2 \sin\xi - \xi^3 \cos\xi - \xi \sin^2\xi \cos\xi + \alpha \xi \cos\xi \sin^2\xi \biggr] \, ; </math>

and the second term inside the square brackets on the right-hand-side gives,

<math>~2\xi^2 x^' + 2\alpha \xi x </math>

<math>~ \frac{1}{\sin^2\xi}\biggl(\xi^3 - \xi^2 \sin\xi \cos\xi \biggr) +\frac{\alpha}{\sin\xi} \biggl(\xi \sin\xi - \xi^2 \cos\xi \biggr) \, . </math>

Put together, then, we have,

RHS	<math>~=</math>	<math>\frac{1}{\sin^2\xi} \biggl[ 2\xi^2 \sin\xi - \xi^3 \cos\xi - \xi \sin^2\xi \cos\xi + \alpha \xi \cos\xi \sin^2\xi \biggr] + \frac{\cos\xi}{\sin^2\xi}\biggl(\xi^3 - \xi^2 \sin\xi \cos\xi \biggr) -\alpha\sin\xi + \alpha \cdot \frac{\cos\xi}{\sin\xi} \biggl(\xi \sin\xi - \xi^2 \cos\xi \biggr) </math>
	<math>~=</math>	<math>\frac{1}{\sin^2\xi} \biggl[ 2\xi^2 \sin\xi - \xi^3 \cos\xi - \xi \sin^2\xi \cos\xi + \alpha \xi \cos\xi \sin^2\xi + \xi^3\cos\xi - \xi^2 \sin\xi \cos^2\xi \biggr] + \frac{\alpha}{\sin\xi} \biggl[ -\sin^2\xi + \xi \sin\xi \cos\xi - \xi^2 \cos^2\xi \biggr] </math>
	<math>~=</math>	<math>\frac{\xi}{\sin\xi} \biggl[ 2\xi - \sin\xi \cos\xi - \xi \cos^2\xi \biggr] - \frac{\alpha}{\sin\xi} \biggl[ \sin^2\xi+\xi^2 \cos^2\xi \biggr] </math>
	<math>~=</math>	<math> \frac{\xi^2}{\sin\xi} \biggl[ 1+\sin^2\xi - \biggl(\frac{\sin\xi}{\xi}\biggr) \cos\xi - \alpha \biggl( \frac{\sin^2\xi}{\xi^2} +\cos^2\xi \biggr)\biggr] \, , </math>

and,

LHS

<math> ~-\frac{\xi \sigma^2}{2} \biggl( \frac{ \xi^2 }{\sin\xi} \biggr) \biggl[\sin\xi - \xi \cos\xi \biggr] \, . </math>

If our trial eigenfunction is a proper solution to the polytropic wave equation, then the difference of these two expressions should be zero. Let's see:

<math>\frac{\sin\xi}{\xi^2} \biggl( \mathrm{RHS} - \mathrm{LHS} \biggr)</math>

<math> ~ 1+\sin^2\xi - \biggl(\frac{\sin\xi}{\xi}\biggr) \cos\xi - \alpha \biggl( \frac{\sin^2\xi}{\xi^2} +\cos^2\xi \biggr) + \frac{\xi \sigma^2}{2} \biggl[\sin\xi - \xi \cos\xi \biggr] \, . </math>

This expression clearly is not zero, so our trial eigenfunction is not a good one. However, the terms in the wave equation did combine somewhat to give a fairly compact — albeit nonzero — expression. So we may be on the right track!

New Idea Involving Logarithmic Derivatives

Simplistic Layout

Let's begin, again, with the relevant LAWE, as provided above. After dividing through by <math>~x</math>, we have,

<math> (\sin\xi )\frac{\xi^2}{x} \cdot \frac{d^2x}{d\xi^2} + 2 \biggl[ \sin\xi + \xi \cos \xi \biggr] \frac{\xi}{x} \cdot \frac{dx}{d\xi} + \biggl[ \sigma^2 \xi^3 - 2\alpha ( \sin\xi - \xi \cos \xi ) \biggr] = 0 \, , </math>

where,

<math>~\sigma^2</math>	<math>~\equiv</math>	<math> ~\frac{\omega^2}{2\pi G\rho_c \gamma_g} \, , </math>
<math>~\alpha</math>	<math>~\equiv</math>	<math> ~3-\frac{4}{\gamma_g} \, . </math>

Now, in addition to recognizing that,

in a separate context, we showed that, quite generally,

<math>~ \frac{d}{d\ln\xi} \biggl[ \frac{d\ln x}{d\ln \xi} \biggr] - \biggl[ 1 - \frac{d\ln x}{d\ln \xi} \biggr]\cdot \frac{d\ln x}{d\ln \xi} \, . </math>

Hence, if we assume that the eigenfunction is a power-law of <math>~\xi</math>, that is, assume that,

then the logarithmic derivative of <math>~x</math> is a constant, namely,

and the two key derivative terms will be,

and

In this case, the LAWE is no longer a differential equation but, instead, takes the form,

<math>~-\sigma^2 \xi^3</math>	<math>~=</math>	<math>~ c_0(c_0-1) \sin\xi + 2c_0 [ \sin\xi + \xi \cos \xi ] - 2\alpha ( \sin\xi - \xi \cos \xi ) </math>
	<math>~=</math>	<math>~ \sin\xi [c_0(c_0-1) +2c_0 -2\alpha ] + \xi \cos \xi [2(c_0+\alpha) ] </math>
	<math>~=</math>	<math>~ \sin\xi [c_0^2 + c_0 -2\alpha ] + \xi \cos \xi [2(c_0+\alpha) ] \, . </math>

Now, the cosine term will go to zero if <math>~c_0 = -\alpha</math>; and the sine term will go to zero if,

<math>~\alpha</math>	<math>~=</math>	<math>~3 </math>
<math>~\Rightarrow ~~~ \gamma_g</math>	<math>~=</math>	<math>~\infty \, . </math>

If these two — rather strange — conditions are met, then we have a marginally unstable configuration because, <math>~\sigma^2 = 0</math>. This, in and of itself, is not very physically interesting. However, it may give us a clue regarding how to more generally search for a physically reasonable radial eigenfunction.

More general Assumption

Try,

<math>~x</math>	<math>~=</math>	<math>~\xi^{c_0} \biggl[a_0 + b_0\sin\xi + d_0 \xi\cos\xi \biggr] </math>
<math>~\Rightarrow ~~~\frac{dx}{d\xi}</math>	<math>~=</math>	<math>~\xi^{c_0} \frac{d}{d\xi}\biggl[a_0 + b_0\sin\xi + d_0 \xi\cos\xi \biggr] + c_0\xi^{c_0-1} \biggl[a_0 + b_0\sin\xi + d_0 \xi\cos\xi \biggr] </math>
	<math>~=</math>	<math>~\xi^{c_0} \biggl[ b_0\cos\xi - d_0 \xi\sin\xi +d_0\cos\xi\biggr] + c_0\xi^{c_0-1} \biggl[a_0 + b_0\sin\xi + d_0 \xi\cos\xi \biggr] </math>
<math>~\Rightarrow ~~~\frac{d\ln x}{d\ln \xi}</math>	<math>~=</math>	<math>~\xi \biggl[ b_0\cos\xi - d_0 \xi\sin\xi +d_0\cos\xi\biggr]\biggl[a_0 + b_0\sin\xi + d_0 \xi\cos\xi \biggr]^{-1} + c_0 </math>
	<math>~=</math>	<math>~\biggl[ (b_0+d_0)\xi\cos\xi - d_0 \xi^2\sin\xi \biggr]\biggl[a_0 + b_0\sin\xi + d_0 \xi\cos\xi \biggr]^{-1} + c_0 </math>

Another Viewpoint

Development

Multiplying through the above LAWE by <math>~(x \xi^{-3})</math> gives,

<math>~ \frac{\sin\xi }{\xi} \cdot \frac{d^2x}{d\xi^2} + 2 \biggl[\frac{ \sin\xi + \xi \cos \xi }{\xi^2}\biggr] \frac{dx}{d\xi} + \biggl[ \sigma^2 - 2\alpha \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr]x </math>

Notice that,

<math>~\frac{d}{d\xi}\biggl[\frac{\sin\xi}{\xi}\biggr]</math>	<math>~=</math>	<math>~ - \frac{\sin\xi}{\xi^2} + \frac{\cos\xi}{\xi} </math>
	<math>~=</math>	<math>~ \biggl[ \frac{\xi\cos\xi - \sin\xi }{\xi^2} \biggr] \, . </math>

And, hence,

<math>~\frac{d^2}{d\xi^2}\biggl[\frac{\sin\xi}{\xi}\biggr]</math>

<math>~ \frac{d}{d\xi}\biggl[ \frac{\cos\xi }{\xi} - \frac{\sin\xi }{\xi^2} \biggr] </math>

<math>~

-\frac{\cos\xi}{\xi^2} -\frac{\sin\xi}{\xi} + \frac{2\sin\xi}{\xi^3} - \frac{\cos\xi}{\xi^2}

</math>

<math>~ -\frac{\sin\xi}{\xi} + 2\biggl[ \frac{\sin\xi -\xi\cos\xi}{\xi^3} \biggr] \, . </math>

So, we can write,

<math>~\frac{d^2}{d\xi^2} \biggl\{ \biggl( \frac{\sin\xi}{\xi}\biggr)x \biggr\}</math>

<math>~\frac{d}{d\xi} \biggl\{

\biggl(\frac{\sin\xi}{\xi}\biggr)\frac{dx}{d\xi}  + x\frac{d}{d\xi} \biggl[ \biggl(\frac{\sin\xi}{\xi}\biggr) \biggr]

\biggr\}</math>

<math>~ \frac{\sin\xi}{\xi} \cdot \frac{d^2 x}{d\xi^2} + 2\frac{dx}{d\xi} \cdot \biggl[\frac{d}{d\xi}\biggr(\frac{\sin\xi}{\xi}\biggr) \biggr] + x \cdot \frac{d^2}{d\xi^2} \biggl(\frac{\sin\xi}{\xi}\biggr) </math>

<math>~ \frac{\sin\xi}{\xi} \cdot \frac{d^2 x}{d\xi^2} + 2\frac{dx}{d\xi} \cdot \biggl[ \frac{\xi\cos\xi - \sin\xi }{\xi^2} \biggr] + x \cdot \biggl\{ -\frac{\sin\xi}{\xi} + 2\biggl[ \frac{\sin\xi -\xi\cos\xi}{\xi^3} \biggr] \biggr\} \, . </math>

This means that we can rewrite the LAWE as,

<math>~0</math>	<math>~=</math>	<math>~ \frac{d^2}{d\xi^2} \biggl\{ \biggl( \frac{\sin\xi}{\xi}\biggr)x \biggr\} - 2\frac{dx}{d\xi} \cdot \biggl[ \frac{\xi\cos\xi - \sin\xi }{\xi^2} \biggr] - x \cdot \biggl\{ -\frac{\sin\xi}{\xi} + 2\biggl[ \frac{\sin\xi -\xi\cos\xi}{\xi^3} \biggr] \biggr\} + 2 \biggl[\frac{ \sin\xi + \xi \cos \xi }{\xi^2}\biggr] \frac{dx}{d\xi} + \biggl[ \sigma^2 - 2\alpha \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr]x </math>
	<math>~=</math>	<math>~ \frac{d^2}{d\xi^2} \biggl\{ \biggl( \frac{\sin\xi}{\xi}\biggr)x \biggr\} + 4 \biggl[\frac{ \sin\xi }{\xi^2}\biggr] \frac{dx}{d\xi} + \biggl\{ \frac{\sin\xi}{\xi} + \sigma^2 - 2(1+\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr\} \cdot x \, . </math>

We recognize, also, that,

<math>~\frac{1}{\xi} \cdot \frac{d}{d\xi}\biggl[ \biggl(\frac{\sin\xi}{\xi} \biggr) x \biggr]</math>	<math>~=</math>	<math>~ \biggl[ \frac{\xi\cos\xi - \sin\xi }{\xi^3} \biggr]x + \biggl(\frac{\sin\xi}{\xi^2} \biggr)\frac{dx}{d\xi} \, . </math>
<math>~\Rightarrow ~~~ 4\biggl(\frac{\sin\xi}{\xi^2} \biggr)\frac{dx}{d\xi} </math>	<math>~=</math>	<math>~ \frac{4}{\xi} \cdot \frac{d}{d\xi}\biggl[ \biggl(\frac{\sin\xi}{\xi} \biggr) x \biggr] + 4\biggl[ \frac{\sin\xi - \xi\cos\xi }{\xi^3} \biggr]x \, . </math>

So the LAWE becomes,

<math>~0</math>	<math>~=</math>	<math>~ \frac{d^2}{d\xi^2} \biggl\{ \biggl( \frac{\sin\xi}{\xi}\biggr)x \biggr\} + \frac{4}{\xi} \cdot \frac{d}{d\xi}\biggl[ \biggl(\frac{\sin\xi}{\xi} \biggr) x \biggr] + 4\biggl[ \frac{\sin\xi - \xi\cos\xi }{\xi^3} \biggr]x + \biggl\{ \frac{\sin\xi}{\xi} + \sigma^2 - 2(1+\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr\} \cdot x </math>
	<math>~=</math>	<math>~ \frac{d^2}{d\xi^2} \biggl\{ \biggl( \frac{\sin\xi}{\xi}\biggr)x \biggr\} + \frac{4}{\xi} \cdot \frac{d}{d\xi}\biggl[ \biggl(\frac{\sin\xi}{\xi} \biggr) x \biggr] + \biggl\{ \frac{\sin\xi}{\xi} + \sigma^2 + [4- 2(1+\alpha)] \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr\} \cdot x </math>
	<math>~=</math>	<math>~ \frac{d^2 \Upsilon}{d\xi^2} + \frac{4}{\xi} \cdot \frac{d\Upsilon}{d\xi} + \Upsilon + \biggl[ \sigma^2 + 2(1-\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr] \cdot x \, , </math>

where we have introduced the new, modified eigenfunction,

<math>\Upsilon \equiv \biggl( \frac{\sin\xi}{\xi} \biggr) x \, .</math>

Alternatively, the LAWE may be written as,

<math>~ \frac{d^2 \Upsilon}{d\xi^2} + \frac{4}{\xi} \cdot \frac{d\Upsilon}{d\xi} + \biggl[ \sigma^2 + 2(1-\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) + \frac{\sin\xi}{\xi} \biggr] \cdot x \, ; </math>

or,

<math>~0</math>	<math>~=</math>	<math>~ \frac{\xi^2}{\Upsilon} \cdot \frac{d^2 \Upsilon}{d\xi^2} + \frac{4\xi}{\Upsilon} \cdot \frac{d\Upsilon}{d\xi} + \biggl[ \sigma^2 + 2(1-\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) + \frac{\sin\xi}{\xi} \biggr] \cdot \frac{\xi^3}{\sin\xi} </math>
	<math>~=</math>	<math>~ \frac{\xi^2}{\Upsilon} \cdot \frac{d^2 \Upsilon}{d\xi^2} + \frac{4\xi}{\Upsilon} \cdot \frac{d\Upsilon}{d\xi} + \biggl[ \sigma^2 \biggl(\frac{\xi^3}{\sin\xi} \biggr) + 2(1-\alpha) \biggl( 1 - \xi \cot \xi \biggr) + \xi^2 \biggr] </math>

Now, if we adopt the homentropic convention that arises from setting, <math>~\gamma = (n+1)/n</math>, then for our <math>~n=1</math> polytropic configuration, we should set, <math>~\gamma = 2</math> and, hence, <math>~\alpha = 1</math>. This will mean that the lat term in this LAWE naturally goes to zero. Hence, we have,

<math>~- \sigma^2 x</math>

<math>~ \frac{d^2 \Upsilon}{d\xi^2} + \frac{4}{\xi} \cdot \frac{d\Upsilon}{d\xi} + \Upsilon \, ; </math>

or,

<math>~ \frac{d^2 \Upsilon}{d\xi^2} + \frac{4}{\xi} \cdot \frac{d\Upsilon}{d\xi} + \biggl[1 + \sigma^2 \biggl(\frac{\xi}{\sin\xi}\biggr) \biggr] \Upsilon \, ; </math>

or,

<math>~ \frac{\xi^2}{\Upsilon} \cdot \frac{d^2 \Upsilon}{d\xi^2} + \frac{4\xi}{\Upsilon} \cdot \frac{d\Upsilon}{d\xi} + \biggl[\xi^2 + \sigma^2 \biggl(\frac{\xi^3}{\sin\xi}\biggr) \biggr] \, . </math>

Does this help?

Check for Mistakes

Given the definition of <math>~\Upsilon</math>, its first derivative is,

<math>~\frac{d\Upsilon}{d\xi} </math>

<math>~ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{dx}{d\xi} +x\biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \, , </math>

and its second derivative is,

<math>~\frac{d^2\Upsilon}{d\xi^2} </math>	<math>~=</math>	<math>~\frac{d}{d\xi} \biggl\{ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{dx}{d\xi} +x\biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \biggr\} </math>
	<math>~=</math>	<math>~ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{d^2x}{d\xi^2} + 2 \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \cdot \frac{dx}{d\xi} + x \cdot \frac{d}{d\xi} \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] </math>
	<math>~=</math>	<math>~ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{d^2x}{d\xi^2} + 2 \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \cdot \frac{dx}{d\xi} + x \biggl[ -\frac{\sin\xi}{\xi} - \frac{2\cos\xi}{\xi^2} + \frac{2\sin\xi}{\xi^3} \biggr] </math>

Hence, the "upsilon" LAWE becomes,

<math>~-\sigma^2 x</math>	<math>~=</math>	<math>~ \frac{d^2 \Upsilon}{d\xi^2} + \frac{4}{\xi} \cdot \frac{d\Upsilon}{d\xi} + \Upsilon + \biggl[ 2(1-\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr] \cdot x </math>
	<math>~=</math>	<math>~ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{d^2x}{d\xi^2} + 2 \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \cdot \frac{dx}{d\xi} + x \biggl[ -\frac{\sin\xi}{\xi} - \frac{2\cos\xi}{\xi^2} + \frac{2\sin\xi}{\xi^3} \biggr] + \frac{4}{\xi} \cdot \biggl\{ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{dx}{d\xi} +x\biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \biggr\} + \biggl[\frac{\sin\xi}{\xi} + 2(1-\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr] \cdot x </math>
	<math>~=</math>	<math>~ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{d^2x}{d\xi^2} + \biggl\{\biggl( \frac{4\sin\xi}{\xi^2} \biggr) + 2 \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \biggr\}\cdot \frac{dx}{d\xi} + \biggl[ -\frac{\sin\xi}{\xi} - \frac{2\cos\xi}{\xi^2} + \frac{2\sin\xi}{\xi^3} + \frac{4\cos\xi}{\xi^2} - \frac{4\sin\xi}{\xi^3} + \frac{\sin\xi}{\xi} + 2(1-\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr] \cdot x </math>
	<math>~=</math>	<math>~ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{d^2x}{d\xi^2} + \biggl[ \frac{2\cos\xi}{\xi} + \frac{2\sin\xi}{\xi^2} \biggr] \cdot \frac{dx}{d\xi} + \biggl[- 2\biggl( \frac{\sin\xi -\xi\cos\xi}{\xi^3} \biggr) + (2-2\alpha) \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr] \cdot x </math>
	<math>~=</math>	<math>~ \biggl( \frac{\sin\xi}{\xi} \biggr) \frac{d^2x}{d\xi^2} + 2\biggl[ \frac{\sin\xi}{\xi^2} + \frac{\cos\xi}{\xi} \biggr] \cdot \frac{dx}{d\xi} + \biggl[-2\alpha \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr] \cdot x \, . </math>

This should be compared with the first expression, above, namely,

<math>~ \frac{\sin\xi }{\xi} \cdot \frac{d^2x}{d\xi^2} + 2 \biggl[\frac{ \sin\xi + \xi \cos \xi }{\xi^2}\biggr] \frac{dx}{d\xi} + \biggl[ \sigma^2 - 2\alpha \biggl( \frac{\sin\xi - \xi \cos \xi}{\xi^3} \biggr) \biggr]x \, , </math>

and it matches! Q.E.D.

Motivated by Yabushita's Discovery

Initial Exploration

This subsection is being developed following our realization — see the accompanying overview — that the eigenfunction is known analytically for marginally unstable, pressure-truncated configurations having <math>~3 \le n \le \infty</math>. Specifically, from the work of Yabushita (1975) we have the following,

Exact Solution to the Isothermal LAWE
<math>~\sigma_c^2 = 0</math>	and	<math>~x = 1 - \biggl( \frac{1}{\xi e^{-\psi}}\biggr) \frac{d\psi}{d\xi} \, .</math>

And from our own recent work, we have discovered the following,

Precise Solution to the Polytropic LAWE
<math>~\sigma_c^2 = 0</math>	and	<math>~x_P \equiv \frac{3(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{1}{\xi \theta^{n}}\biggr) \frac{d\theta}{d\xi}\biggr] </math>

if the adiabatic exponent is assigned the value, <math>~\gamma_g = (n+1)/n</math>, in which case the parameter, <math>~\alpha = (3-n)/(n+1)</math>. Using this polytropic displacement function as a guide, let's try for the case of <math>~n=1</math>, an expression of the form,

<math>~x</math>	<math>~=</math>	<math>~A - B\biggl[ \biggl( \frac{1}{\xi \theta}\biggr) \frac{d\theta}{d\xi} \biggr]</math>
	<math>~=</math>	<math>~A - B \biggl[ \biggl( \frac{1}{\sin\xi}\biggr) \frac{d}{d\xi} \biggl( \frac{\sin\xi}{\xi} \biggr)\biggr]</math>
	<math>~=</math>	<math>~A - B \biggl( \frac{1}{\sin\xi}\biggr) \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] </math>
	<math>~=</math>	<math>~A + \frac{B}{\xi^2} \biggl( 1-\frac{\xi \cos\xi}{\sin\xi} \biggr) \, ,</math>

in which case,

<math>~\frac{dx}{d\xi}</math>	<math>~=</math>	<math>~- B \biggl\{ \biggl( \frac{- \cos\xi}{\sin^2\xi}\biggr) \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] +\biggl( \frac{1}{\sin\xi}\biggr) \biggl[ -\frac{\sin\xi}{\xi} - \frac{\cos\xi}{\xi^2} - \frac{\cos\xi}{\xi^2} + \frac{2\sin\xi}{\xi^3} \biggr] \biggr\}</math>
	<math>~=</math>	<math>~- \frac{B}{\xi^3} \biggl\{ \biggl( \frac{\cos\xi}{\sin^2\xi}\biggr) \biggl[ - \xi^2 \cos\xi + \xi \sin\xi \biggr] +\biggl[ 2 -\xi^2 - \frac{2\xi\cos\xi}{\sin\xi} \biggr] \biggr\} </math>
	<math>~=</math>	<math>~ - \frac{B}{\xi^3} \biggl\{ 2 -\xi^2 - \frac{\xi\cos\xi}{\sin\xi} - \frac{\xi^2 \cos^2\xi}{\sin^2\xi} \biggr\} \, , </math>

What if, instead, we try the more generalized form,

<math>~A + \frac{B}{(\lambda \xi)^2} \biggl[ 1-\frac{\lambda \xi \cos(\lambda \xi)}{\sin(\lambda \xi)} \biggr] \, .</math>

Then we have,

<math>~\frac{1}{\lambda B} \cdot \frac{dx}{d\xi}</math>

<math>~ - \frac{1}{ (\lambda \xi)^3} \biggl\{ 2 - (\lambda \xi)^2 - \frac{\lambda \xi\cos(\lambda \xi)}{\sin(\lambda \xi)} - \frac{(\lambda \xi)^2 \cos^2(\lambda\xi)}{\sin^2(\lambda\xi)} \biggr\} \, , </math>

Probably this also means,

<math>~\frac{1}{\lambda^2 B} \cdot \frac{dx}{d\xi}</math>

<math>~ \frac{1}{(\lambda\xi)^4} \biggl\{ 6 - 2 (\lambda \xi)^2 - \frac{2 \lambda \xi\cos(\lambda\xi)}{\sin(\lambda\xi)} - \frac{2(\lambda\xi)^2 \cos^2(\lambda\xi)}{\sin^2(\lambda\xi)} - \frac{2(\lambda\xi)^3 \cos(\lambda\xi)}{\sin(\lambda\xi)} - \frac{2(\lambda\xi)^3 \cos^3(\lambda\xi)}{\sin^3(\lambda\xi)} \biggr\} \, . </math>

Let's check against the more general derivation, which gives after recognizing that, <math>~B \leftrightarrow (3-n)/(n-1)</math>,

<math>~\frac{dx}{d\xi}</math>	<math>~=</math>	<math>~ \biggl(\frac{3-n}{n-1}\biggr) \biggl\{ \frac{1}{\xi} + \frac{n(\theta^')^2 }{\xi \theta^{n+1}} + \frac{3\theta^' }{\xi^2 \theta^{n}} \biggr\} </math>
	<math>~=</math>	<math>~ \frac{B}{\xi^3} \biggl\{ \xi^2 + \xi^2 \biggl( \frac{\xi}{\sin\xi}\biggr)^2 \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr]^2 + \frac{3\xi^2}{\sin\xi} \biggl[ \frac{\cos\xi}{\xi} - \frac{\sin\xi}{\xi^2} \biggr] \biggr\} </math>
	<math>~=</math>	<math>~ \frac{B}{\xi^3} \biggl\{ \xi^2 + 3\biggl[ \frac{\xi \cos\xi}{\sin\xi} - 1 \biggr] + \biggl[ \frac{\xi \cos\xi}{\sin\xi} - 1 \biggr]^2 \biggr\} </math>
	<math>~=</math>	<math>~ \frac{B}{\xi^3} \biggl\{ \xi^2 + 3\biggl[ \frac{\xi \cos\xi}{\sin\xi} - 1 \biggr] + \biggl[ \biggl(\frac{\xi \cos\xi}{\sin\xi}\biggr)^2 - 2\biggl(\frac{\xi \cos\xi}{\sin\xi}\biggr) + 1 \biggr] \biggr\} </math>
	<math>~=</math>	<math>~ \frac{B}{\xi^3} \biggl\{ \xi^2 + \biggl[ \frac{\xi \cos\xi}{\sin\xi} - 2 \biggr] + \biggl(\frac{\xi \cos\xi}{\sin\xi}\biggr)^2 \biggr\} \, . </math>

This matches the preceding, direct derivation.

Also,

<math>~\frac{d^2x}{d\xi^2}</math>	<math>~=</math>	<math>~\frac{3B}{\xi^4} \biggl\{ \biggl( \frac{\cos\xi}{\sin^2\xi}\biggr) \biggl[ - \xi^2 \cos\xi + \xi \sin\xi \biggr] +\biggl[ 2 -\xi^2 - \frac{2\xi\cos\xi}{\sin\xi} \biggr] \biggr\} </math>
		<math>~- \frac{B}{\xi^3} \biggl\{ \biggl[- \frac{1}{\sin\xi} - \frac{2\cos^2\xi}{\sin^3\xi} \biggr] \biggl[ - \xi^2 \cos\xi + \xi \sin\xi \biggr] + \biggl( \frac{\cos\xi}{\sin^2\xi}\biggr) \biggl[ - 2\xi \cos\xi + \sin\xi + \xi^2 \sin\xi + \xi \cos\xi \biggr] </math>
		<math>~ +\biggl[ -2\xi - \frac{2\cos\xi}{\sin\xi} + \frac{2\xi\sin\xi}{\sin\xi} + \frac{2\xi\cos^2\xi}{\sin^2\xi}\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{B}{\xi^4} \biggl\{ \biggl( \frac{3\cos\xi}{\sin^2\xi}\biggr) \biggl[ - \xi^2 \cos\xi + \xi \sin\xi \biggr] +\biggl[ 6 - 3\xi^2 - \frac{6\xi\cos\xi}{\sin\xi} \biggr] + \biggl[\frac{1}{\sin\xi} + \frac{2\cos^2\xi}{\sin^3\xi} \biggr] \biggl[ - \xi^3 \cos\xi + \xi^2 \sin\xi \biggr] </math>
		<math>~ + \biggl( \frac{\cos\xi}{\sin^2\xi}\biggr) \biggl[ 2\xi^2 \cos\xi - \xi \sin\xi - \xi^3 \sin\xi - \xi^2 \cos\xi \biggr] +\biggl[ 2\xi^2 + \frac{2\xi \cos\xi}{\sin\xi} - \frac{2\xi^2\sin\xi}{\sin\xi} - \frac{2\xi^2\cos^2\xi}{\sin^2\xi}\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{B}{\xi^4} \biggl\{ \biggl[ - \frac{3\xi^2 \cos^2\xi}{\sin^2\xi} + \frac{3\xi \cos\xi}{\sin\xi} \biggr] +\biggl[ 6 - 3\xi^2 - \frac{6\xi\cos\xi}{\sin\xi} \biggr] + \biggl[ - \frac{\xi^3 \cos\xi}{\sin\xi} + \xi^2 \biggr] + \biggl[ - \frac{2\xi^3 \cos^3\xi}{\sin^3\xi} + \frac{2\xi^2 \cos^2\xi}{\sin^2\xi} \biggr] </math>
		<math>~ + \biggl[ \frac{2\xi^2 \cos^2\xi}{\sin^2\xi} - \frac{\xi \cos\xi}{\sin\xi} - \frac{\xi^3 \cos\xi}{\sin\xi} - \frac{\xi^2 \cos^2\xi}{\sin^2\xi} \biggr] +\biggl[ 2\xi^2 + \frac{2\xi \cos\xi}{\sin\xi} - \frac{2\xi^2\sin\xi}{\sin\xi} - \frac{2\xi^2\cos^2\xi}{\sin^2\xi}\biggr] \biggr\} </math>
	<math>~=</math>	<math>~ \frac{B}{\xi^4} \biggl\{ 6 - 2\xi^2 - \frac{2\xi\cos\xi}{\sin\xi} - \frac{2\xi^2 \cos^2\xi}{\sin^2\xi} - \frac{2\xi^3 \cos\xi}{\sin\xi} - \frac{2\xi^3 \cos^3\xi}{\sin^3\xi} \biggr\} \, . </math>

Let's also check this against the more general derivation, which gives after again recognizing that, <math>~B \leftrightarrow (3-n)/(n-1)</math>,

<math>\frac{d^2 x}{d\xi^2}</math>	<math>~=</math>	<math>~ \biggl(\frac{n-3}{n-1}\biggr) \biggl\{ \frac{4}{\xi^2} + \frac{2n(\theta^')}{\xi \theta} + \frac{12\theta^' }{\xi^3 \theta^{n}}+ \frac{8n(\theta^')^2}{\xi^2 \theta^{n+1}} + (n+1) \frac{n(\theta^')^3 }{\xi \theta^{n+2}} \biggr\} </math>
	<math>~=</math>	<math>~-B \biggl\{ \frac{4}{\xi^2} + \frac{2}{\xi \theta} \biggl[ \frac{\sin\xi}{\xi^2}\biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr)\biggr] + \frac{12}{\xi^3 \theta}\biggl[ \frac{\sin\xi}{\xi^2}\biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr)\biggr] + \frac{8 }{\xi^2 \theta^{2}} \biggl[ \frac{\sin\xi}{\xi^2}\biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr)\biggr]^2 + \frac{2 }{\xi \theta^{3}} \biggl[ \frac{\sin\xi}{\xi^2}\biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr)\biggr]^3\biggr\} </math>
	<math>~=</math>	<math>~-\frac{B}{\xi^4} \biggl\{ 4\xi^2 + 2\xi^2 \biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr) + 12\biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr) + 8 \biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr)^2 + 2\biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr)^3\biggr\} </math>
	<math>~=</math>	<math>~-\frac{2B}{\xi^4} \biggl\{ 2\xi^2 + \frac{\xi^3\cos\xi}{\sin\xi} - \xi^2+ \frac{6\xi\cos\xi}{\sin\xi} - 6 + \frac{4\xi^2\cos^2\xi}{\sin^2\xi} - \frac{8\xi\cos\xi}{\sin\xi} + 4 + \biggl(\frac{\xi^2\cos^2\xi}{\sin^2\xi} - \frac{2\xi\cos\xi}{\sin\xi} + 1\biggr) \biggl(\frac{\xi\cos\xi}{\sin\xi} - 1\biggr)\biggr\} </math>
	<math>~=</math>	<math>~-\frac{2B}{\xi^4} \biggl\{-2 + \xi^2 - \frac{2\xi\cos\xi}{\sin\xi} + \frac{\xi^3\cos\xi}{\sin\xi} + \frac{4\xi^2\cos^2\xi}{\sin^2\xi} - \biggl(\frac{\xi^2\cos^2\xi}{\sin^2\xi} - \frac{2\xi\cos\xi}{\sin\xi} + 1\biggr) + \frac{\xi^3\cos^3\xi}{\sin^3\xi} - \frac{2\xi^2\cos^2\xi}{\sin^2\xi} + \frac{\xi\cos\xi}{\sin\xi} \biggr\} </math>
	<math>~=</math>	<math>~-\frac{2B}{\xi^4} \biggl\{-3 + \xi^2 + \frac{\xi\cos\xi}{\sin\xi} + \frac{\xi^3\cos\xi}{\sin\xi} + \frac{\xi^2\cos^2\xi}{\sin^2\xi} + \frac{\xi^3\cos^3\xi}{\sin^3\xi} \biggr\} </math>
	<math>~=</math>	<math>~ \frac{B}{\xi^4} \biggl\{6 -2 \xi^2 - \frac{2\xi\cos\xi}{\sin\xi} - \frac{2\xi^2\cos^2\xi}{\sin^2\xi} - \frac{2\xi^3\cos\xi}{\sin\xi} - \frac{2\xi^3\cos^3\xi}{\sin^3\xi} \biggr\} \, . </math>

A cross-check with the first attempt to derive this second derivative expression initially unveiled a couple of coefficient errors. These have now been corrected and both expressions agree.

Succinct Demonstration

Given that, for <math>~n=1</math>, we should set <math>~\gamma_\mathrm{g} = (n+1)/n = 2 \Rightarrow \alpha = (3-4/\gamma_\mathrm{g}) = +1</math>, and,

<math>~Q \equiv - \frac{d\ln\theta}{d\ln\xi}</math>

<math>~ - \frac{\xi^2}{\sin\xi} \cdot \frac{d}{d\xi}\biggl[ \frac{\sin\xi}{\xi}\biggr] = 1 - \xi \cot\xi \, . </math>

If we then employ the displacement function,

<math>~A + \frac{B}{\xi^2} \biggl[ 1 - \xi \cot\xi \biggr] \, ,</math>

the LAWE becomes,

LAWE	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[4 - (n+1)Q \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + (n+1)\biggl[ \biggl(\frac{\sigma_c^2}{6\gamma_g } \biggr) \frac{\xi^2}{\theta} -\alpha Q\biggr] \frac{ x}{\xi^2} </math>
	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[4 - 2Q \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ \biggl(\frac{\sigma_c^2}{6 } \biggr) \frac{\xi^3}{\sin\xi} - 2Q\biggr] \frac{ x}{\xi^2} </math>
	<math>~=</math>	<math>~ \frac{d^2x}{d\xi^2} + \biggl[2 + \frac{2\xi\cos\xi}{\sin\xi} \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[- 2 + \frac{2\xi\cos\xi}{\sin\xi} \biggr] \frac{ x}{\xi^2} + \biggl[ \biggl(\frac{\sigma_c^2}{6 } \biggr) \frac{\xi}{\sin\xi} \biggr] x </math>
	<math>~=</math>	<math>~ \frac{2B}{\xi^4} \biggl\{3 - \xi^2 - \frac{\xi\cos\xi}{\sin\xi} - \biggl(\frac{\xi\cos\xi}{\sin\xi} \biggr)^2 - \frac{\xi^3\cos\xi}{\sin\xi} - \biggl( \frac{\xi\cos\xi}{\sin\xi} \biggr)^3 \biggr\} </math>
		<math>~ + \frac{2B}{\xi^4}\biggl[1 + \frac{\xi\cos\xi}{\sin\xi} \biggr] \biggl\{ \xi^2 - 2 + \frac{\xi \cos\xi}{\sin\xi} + \biggl(\frac{\xi \cos\xi}{\sin\xi}\biggr)^2 \biggr\} </math>
		<math>~ + \biggl[- 2 + \frac{2\xi\cos\xi}{\sin\xi} \biggr] \biggl[ \frac{A}{\xi^2} + \frac{B}{\xi^4} \biggl( 1-\frac{\xi \cos\xi}{\sin\xi} \biggr)\biggr] + \biggl[ \biggl(\frac{\sigma_c^2}{6 } \biggr) \frac{\xi}{\sin\xi} \biggr] x </math>
	<math>~=</math>	<math>~ \frac{2B}{\xi^4} \biggl\{3 - \xi^2 - \frac{\xi\cos\xi}{\sin\xi} - \biggl(\frac{\xi\cos\xi}{\sin\xi} \biggr)^2 - \frac{\xi^3\cos\xi}{\sin\xi} - \biggl( \frac{\xi\cos\xi}{\sin\xi} \biggr)^3 </math>
		<math>~ + \xi^2\biggl( \frac{\xi\cos\xi}{\sin\xi} \biggr) - 2\biggl( \frac{\xi\cos\xi}{\sin\xi} \biggr) + \biggl(\frac{\xi \cos\xi}{\sin\xi} \biggr)^2 + \biggl(\frac{\xi \cos\xi}{\sin\xi}\biggr)^3 + \xi^2 - 2 + \frac{\xi \cos\xi}{\sin\xi} + \biggl(\frac{\xi \cos\xi}{\sin\xi}\biggr)^2 \biggr\} </math>
		<math>~ - \frac{2B}{\xi^4} \biggl[ 1 - \frac{2\xi\cos\xi}{\sin\xi} + \biggl(\frac{\xi \cos\xi}{\sin\xi} \biggr)^2 \biggr] + \frac{2A}{\xi^2}\biggl[\frac{\xi\cos\xi}{\sin\xi} -1\biggr] + \biggl[ \biggl(\frac{\sigma_c^2}{6 } \biggr) \frac{\xi}{\sin\xi} \biggr] x </math>
	<math>~=</math>	<math>~ \frac{2A}{\xi^2}\biggl[\frac{\xi\cos\xi}{\sin\xi} -1\biggr] + \biggl[ \biggl(\frac{\sigma_c^2}{6 } \biggr) \frac{\xi}{\sin\xi} \biggr] x </math>

Pretty amazing degree of cancelation! So the above-hypothesized displacement function does satisfy the <math>~n=1</math>, polytropic LAWE — for any value of the coefficient, <math>~B</math> — if we set <math>~A = 0</math> and <math>~\sigma_c^2=0</math>. If we set <math>~B = 3</math>, the function will be normalized such that it goes to unity at the center. In summary, then, we have,

<math>~x_P\biggr|_{n=1}</math>

<math>~\frac{3}{\xi^2} \biggl[ 1 - \xi \cot\xi \biggr] \, .</math>

What About Bipolytropes?

Here we will try to find an analytic expression for the radial displacement function, <math>~x</math>, for a bipolytropic envelope whose polytropic index is, <math>~n_e = 1</math>. As in the above succinct derivation, the relevant LAWE is,

LAWE	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[4 - (n+1)Q \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + (n+1)\biggl[ \biggl(\frac{\sigma_c^2}{6\gamma_g } \biggr) \frac{\xi^2}{\theta} -\alpha Q\biggr] \frac{ x}{\xi^2} </math>
	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[4 - 2Q \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ \biggl(\frac{\sigma_c^2}{6 } \biggr) \frac{\xi^3}{\sin\xi} - 2Q\biggr] \frac{ x}{\xi^2} </math>
	<math>~=</math>	<math>~ \frac{d^2x}{d\xi^2} + \biggl[2 + \frac{2\xi\cos\xi}{\sin\xi} \biggr] \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[- 2 + \frac{2\xi\cos\xi}{\sin\xi} \biggr] \frac{ x}{\xi^2} + \biggl[ \biggl(\frac{\sigma_c^2}{6 } \biggr) \frac{\xi}{\sin\xi} \biggr] x </math>

First Attempt

Let's try,

<math>~ A + \frac{B}{(\xi - F)^2} \biggl[1 - (\xi-D) \cot(\xi-C) \biggr] \, . </math>

First, note that,

<math>~\frac{d}{d\xi}\biggl[\cot(\xi - C) \biggr]</math>	<math>~=</math>	<math>~\frac{d}{d\xi}\biggl[ \frac{ \cos(\xi - C) }{ \sin(\xi - C)}\biggr]</math>
	<math>~=</math>	<math>~ - \biggl[ 1 + \cot^2(\xi - C)\biggr] \, . </math>

Hence,

<math>~\frac{dx}{d\xi}</math>	<math>~=</math>	<math>~ - \frac{2B}{(\xi-F)^3} \biggl[1 - (\xi-D) \cot(\xi-C) \biggr] - \frac{B}{(\xi-F)^2} \biggl\{ \cot(\xi-C) - (\xi-D) [1 + \cot^2(\xi-C) ] \biggr\} </math>
	<math>~=</math>	<math>~ - \frac{B}{(\xi-F)^3} \biggl\{\biggl[2 - 2(\xi-D) \cot(\xi-C) \biggr] - (\xi-F) \biggl[ \cot(\xi-C) - (\xi-D) [1 + \cot^2(\xi-C) ]\biggr] \biggr\} </math>
	<math>~=</math>	<math>~ - \frac{B}{(\xi-F)^3} \biggl\{ 2 - \cot(\xi-C)\biggl[ 2(\xi-D) + (\xi-F) \biggr] + (\xi-F) (\xi-D) [1 + \cot^2(\xi-C) ] \biggr\} </math>
	<math>~=</math>	<math>~ - \frac{B}{(\xi-F)^3} \biggl\{ 2 - \biggl[3\xi - (2D +F) \biggr] \cot(\xi-C) + [ \xi^2 - (D+F)\xi + FD] + [ \xi^2 - (D+F)\xi + FD]\cot^2(\xi-C) \biggr\} </math>
	<math>~=</math>	<math>~ - \frac{B}{(\xi-F)^3} \biggl\{ [ \xi^2 - (D+F)\xi + FD+2] - \biggl[3\xi - (2D +F) \biggr] \cot(\xi-C) + [ \xi^2 - (D+F)\xi + FD]\cot^2(\xi-C) \biggr\} \, . </math>

And,

<math>~\frac{d^2x}{d\xi^2}</math>	<math>~=</math>	<math>~ \frac{3B}{(\xi-F)^4} \biggl\{ [ \xi^2 - (D+F)\xi + FD+2] - \biggl[3\xi - (2D +F) \biggr] \cot(\xi-C) + [ \xi^2 - (D+F)\xi + FD]\cot^2(\xi-C) \biggr\} </math>
		<math>~ - \frac{B}{(\xi-F)^3} \biggl\{ [ 2\xi - (D+F)] - 3 \cot(\xi-C) - \biggl[3\xi - (2D +F) \biggr] \frac{d \cot(\xi-C)}{d\xi} + [ 2\xi - (D+F)]\cot^2(\xi-C) + \biggl[ \xi^2 - (D+F)\xi + FD \biggr] \frac{d \cot^2(\xi-C) }{d\xi} \biggr\} </math>
<math>~\Rightarrow ~~~\biggl[ \frac{(\xi-F)^4}{B} \biggr] \frac{d^2x}{d\xi^2}</math>	<math>~=</math>	<math>~ 3[ \xi^2 - (D+F)\xi + FD+2] - 3\biggl[3\xi - (2D +F) \biggr] \cot(\xi-C) + 3[ \xi^2 - (D+F)\xi + FD]\cot^2(\xi-C) </math>
		<math>~ - (\xi-F)[ 2\xi - (D+F)] + 3 (\xi-F) \cot(\xi-C) - (\xi-F)[ 2\xi - (D+F)]\cot^2(\xi-C) </math>
		<math>~ + (\xi-F)\biggl[3\xi - (2D +F) \biggr] \frac{d \cot(\xi-C)}{d\xi} - (\xi-F)\biggl[ \xi^2 - (D+F)\xi + FD \biggr] \frac{d \cot^2(\xi-C) }{d\xi} </math>
	<math>~=</math>	<math>~ 3[ \xi^2 - (D+F)\xi + FD+2] - (\xi-F)[ 2\xi - (D+F)] + \biggl\{3 (\xi-F) - 3\biggl[3\xi - (2D +F) \biggr] \biggr\} \cot(\xi-C) </math>
		<math>~ + \biggl\{ 3[ \xi^2 - (D+F)\xi + FD] - (\xi-F)[ 2\xi - (D+F)] \biggr\}\cot^2(\xi-C) </math>
		<math>~ - (\xi-F)\biggl[3\xi - (2D +F) \biggr] \biggl[ 1 + \cot^2(\xi - C)\biggr] + (\xi-F)\biggl[ \xi^2 - (D+F)\xi + FD \biggr] 2\cot(\xi-C)\biggl[ 1 + \cot^2(\xi - C)\biggr] </math>
	<math>~=</math>	<math>~ 3[ \xi^2 - (D+F)\xi + FD+2] - (\xi-F)[ 2\xi - (D+F)] - (\xi-F) [3\xi - (2D +F) ] + \biggl\{3 (\xi-F) - 3 [3\xi - (2D +F) ] + 2 (\xi-F) [ \xi^2 - (D+F)\xi + FD ] \biggr\} \cot(\xi-C) </math>
		<math>~ + \biggl\{ 3[ \xi^2 - (D+F)\xi + FD] - (\xi-F)[ 2\xi - (D+F)] - (\xi-F) [3\xi - (2D +F) ] \biggr\}\cot^2(\xi-C) + 2 (\xi-F) [ \xi^2 - (D+F)\xi + FD ] \cot^3(\xi - C) \, . </math>

Let's set <math>~C = D = F</math> and see if these expressions match the ones above.

<math>~\frac{dx}{d\xi} \biggr|_{C=D=F}</math>

<math>~ - \frac{B}{\xi^3} \biggl\{ 2 + \xi^2 - 3\xi \cot\xi + \xi^2 \cot^2\xi \biggr\} \, . </math>

<math>~\frac{\xi^4}{B} \cdot \frac{d^2x}{d\xi^2} \biggr\|_{C=D=F}</math>	<math>~=</math>	<math>~ 3[ \xi^2 +2] - (\xi)[ 2\xi ] - \xi [3\xi ] + \biggl\{3 (\xi) - 3 [3\xi ] + 2 \xi [ \xi^2 ] \biggr\} \cot(\xi) </math>
		<math>~ + \biggl\{ 3[ \xi^2 ] - \xi[ 2\xi ] - \xi [3\xi ] \biggr\}\cot^2\xi + 2 \xi [ \xi^2 ] \cot^3\xi </math>
	<math>~=</math>	<math>~ 3 \xi^2 +6- 2\xi^2 - 3\xi^2 + \biggl[ 3 \xi - 9\xi + 2 \xi^3 \biggr] \cot(\xi) + \biggl[ 3\xi^2 - 2\xi^2 - 3\xi^2 \biggr] \cot^2\xi + 2 \xi^3 \cot^3\xi </math>
	<math>~=</math>	<math>~ 6- 2\xi^2 + [ - 6\xi + 2 \xi^3 ] \cot(\xi) - 2\xi^2 \cot^2\xi + 2 \xi^3 \cot^3\xi </math>

Second Attempt

Up to this point we have been rather cavalier about the use of <math>~\xi</math> (and <math>~\xi_i</math>) to represent the envelope's dimensionless radius (and interface location). Let's switch to <math>~\eta</math>,

<math>\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta</math>

<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} + \frac{1}{2\pi \theta_i^4} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr\} x \, . </math>

and, throughout the envelope we have,

<math>~\frac{\rho^}{P^}</math>	<math>~=</math>	<math>~ \biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{-1}_i \phi(\eta)^{-1} \, ; </math>
<math>~\frac{M_r^}{r^}</math>	<math>~=</math>	<math>~ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \theta^{-1}_i \biggl( \frac{2}{\pi} \biggr)^{1/2} \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr) \biggl[ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta \biggr]^{-1} = 2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta_i \eta \biggl(- \frac{d\phi}{d\eta} \biggr) \, . </math>

Hence, the LAWE relevant to the envelope is,

<math>~0</math>	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -\biggl[ \frac{\rho^}{P^}\biggr] \biggl[ \frac{ M_r^}{(r^)} \biggr] \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} + \frac{1}{2\pi \theta_i^4} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl[ \frac{\rho^}{ P^ } \biggr] \biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_e }{(r^)^2} \biggl[ \frac{M_r^}{r^*} \biggr] \biggr\} x </math>
	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -\biggl[ \biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{-1}_i \phi(\eta)^{-1} \biggr] \biggl[ 2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta_i \eta \biggl(- \frac{d\phi}{d\eta} \biggr) \biggr] \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} + \frac{1}{2\pi \theta_i^4} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl[ \biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{-1}_i \phi(\eta)^{-1} \biggr] \biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\alpha_e \biggl[ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta \biggr]^{-2} \biggl[2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta_i \eta \biggl(- \frac{d\phi}{d\eta} \biggr) \biggr] \biggr\} x </math>
	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 - \biggl[ \frac{2 \eta}{\phi} \biggl(- \frac{d\phi}{d\eta} \biggr) \biggr] \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} + \frac{1}{2\pi \theta_i^5 \phi} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\alpha_e \biggl[ \biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{5}_i (4\pi) \eta^{-1} \biggr] \biggl(- \frac{d\phi}{d\eta} \biggr) \biggr\} x </math>
	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 - \biggl[ \frac{2 \eta}{\phi} \biggl(- \frac{d\phi}{d\eta} \biggr) \biggr] \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} + \frac{1}{2\pi \theta_i^5 \phi} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} \biggr\} x ~-~ \alpha_e \biggl[ \frac{2\eta}{\phi} \biggl(- \frac{d\phi}{d\eta} \biggr) \biggr] \frac{x}{\eta^2} \, . </math>

If we assume that, <math>~\alpha_e = (3 - 4/2) = 1</math> and <math>~\sigma_c^2 = 0</math>, then the relevant envelope LAWE is,

<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -2Q \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} ~-~ \biggl[ 2 Q \biggr] \frac{x}{\eta^2} \, , </math>

where,

<math>~ Q \equiv - \frac{d \ln \phi}{ d\ln \eta} \, . </math>

Now consider the,

Precise Solution to the Polytropic LAWE
<math>~x_P</math>	<math>~=</math>	<math>~\frac{b(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{1}{\eta \phi^{n}}\biggr) \frac{d\phi}{d\eta}\biggr]</math>
	<math>~=</math>	<math>~-b\biggl[ \biggl( \frac{1}{\eta \phi}\biggr) \frac{d\phi}{d\eta}\biggr]</math>
	<math>~=</math>	<math>~\frac{b}{\eta^2}\biggl[ -\frac{d\ln \phi}{d\ln \eta}\biggr] </math>
	<math>~=</math>	<math>~\frac{bQ}{\eta^2} \, .</math>

From our accompanying discussion, we recall that the most general solution to the <math>n=1</math> Lane-Emden equation can be written in the form,

<math> \phi = A \biggl[ \frac{\sin(\eta - B)}{\eta} \biggr] \, , </math>

where <math>A</math> and <math>B</math> are constants whose values can be obtained from our accompanying parameter table. The first derivative of this function is,

<math> \frac{d\phi}{d\eta} = \frac{A}{\eta^2} \biggl[ \eta\cos(\eta-B) - \sin(\eta-B) \biggr] \, . </math>

Hence,

<math>~Q = -\frac{d\ln\phi}{d\ln\eta}</math>	<math>~=</math>	<math>~ - \frac{\eta}{\phi} \cdot \frac{A}{\eta^2} \biggl[ \eta\cos(\eta-B) - \sin(\eta-B) \biggr] </math>
	<math>~=</math>	<math>~ \biggl[1- \eta\cot(\eta-B) \biggr] </math>
<math>~\Rightarrow ~~~ x_P</math>	<math>~=</math>	<math>~ \frac{b}{\eta^2} \biggl[1- \eta\cot(\eta-B) \biggr] \, . </math>

What is this in terms of the dimensionless radius, <math>~r^*/R^*</math>? Well,

<math>\frac{~r^}{R^}</math>	<math>~=</math>	<math>\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta \biggl[\frac{\sqrt{2\pi}~\theta_i^2}{\eta_s} \biggl(\frac{\mu_e}{\mu_c}\biggr)\biggr]</math>
	<math>~=</math>	<math>\frac{\eta}{\eta_s} = \frac{\eta}{(\pi + B)} </math>
<math>~\Rightarrow ~~~ \eta</math>	<math>~=</math>	<math>\frac{~r^}{R^}\biggl(\pi + B \biggr) \, .</math>

Also,

<math>~\eta-B</math>	<math>~=</math>	<math>\frac{~r^}{R^}\biggl(\pi + B \biggr) -B = \pi \biggl( \frac{r^}{R^}\biggr) - B\biggl[1-\biggl( \frac{r^}{R^}\biggr)\biggr]</math>
	<math>~=</math>	<math>\pi + \pi \biggl[ \biggl( \frac{r^}{R^}\biggr)-1\biggr] - B\biggl[1-\biggl( \frac{r^}{R^}\biggr)\biggr]</math>
	<math>~=</math>	<math>\pi - (\pi + B)\biggl[1-\biggl( \frac{r^}{R^}\biggr)\biggr] \, .</math>

[12 January 2019]: Here's what appears to work pretty well, empirically:

<math>~x_P</math>	<math>~=</math>	<math>~ \frac{1}{\eta^2} \biggl\{1- \eta\cot[\eta-(\pi - 0.8)] \biggr\} \, . </math>
<math>~\eta</math>	<math>~=</math>	<math>\frac{~r^}{R^}\biggl(\pi - 0.6\pi \biggr) \, .</math>

Let's work through the analytic derivatives again. Keeping in mind that,

<math>~\frac{d}{d\eta}\biggl[\cot(\eta - B) \biggr]</math>

<math>~ - \biggl[ 1 + \cot^2(\eta - B)\biggr] \, , </math>

and starting with the guess,

<math>~ \frac{b}{\eta^2} \biggl[1- \eta\cot(\eta-B) \biggr] \, , </math>

we have,

<math>~ \frac{dx_P}{d\eta}</math>	<math>~=</math>	<math>~ -\frac{2b}{\eta^3} \biggl[1- \eta\cot(\eta-B) \biggr] - \frac{b}{\eta^2} \biggl\{ \cot(\eta-B) - \eta \biggl[ 1 + \cot^2(\eta - B)\biggr] \biggr\} </math>
<math>~ \Rightarrow ~~~ \biggl( \frac{\eta^3}{b} \biggr) \frac{dx_P}{d\eta}</math>	<math>~=</math>	<math>~ - \biggl[2- 2\eta\cot(\eta-B) \biggr] - \biggl\{ \eta \cot(\eta-B) - \eta^2 \biggl[ 1 + \cot^2(\eta - B)\biggr] \biggr\} </math>
	<math>~=</math>	<math>~ \eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B) \, . </math>

The second derivative then gives,

<math>~ \frac{d^2x_P}{d\eta^2}</math>	<math>~=</math>	<math>~ \frac{d}{d\eta}\biggl\{ \frac{b}{\eta^3} \biggl[ \eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~ - \frac{3b}{\eta^4} \biggl[ \eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B) \biggr] </math>
		<math>~ +\frac{b}{\eta^3} \biggl\{ 2\eta + \cot(\eta-B) + 2\eta \cot^2(\eta - B) + \eta\frac{d}{d\eta}\biggl[\cot(\eta-B)\biggr] + 2\eta^2\cot(\eta-B) \frac{d}{d\eta} \biggl[ \cot(\eta - B) \biggr] \biggr\} </math>
<math>~\Rightarrow~~~ \frac{d^2x_P}{d\eta^2}</math>	<math>~=</math>	<math>~ \frac{b}{\eta^4} \biggl[ 6 - 3\eta^2 - 3\eta\cot(\eta-B) - 3\eta^2\cot^2(\eta - B) \biggr] </math>
		<math>~ +\frac{b}{\eta^4} \biggl\{ 2\eta^2 + \eta \cot(\eta-B) + 2\eta^2 \cot^2(\eta - B) - \eta^2\biggl[ 1 + \cot^2(\eta - B)\biggr] - 2\eta^3\cot(\eta-B) \biggl[ 1 + \cot^2(\eta - B)\biggr] \biggr\} </math>
<math>~\Rightarrow~~~\frac{\eta^4}{b}\cdot \frac{d^2x_P}{d\eta^2}</math>	<math>~=</math>	<math>~ 6 - 3\eta^2 - 3\eta\cot(\eta-B) - 3\eta^2\cot^2(\eta - B) </math>
		<math>~ ~+~ 2\eta^2 + \eta \cot(\eta-B) + 2\eta^2 \cot^2(\eta - B) -\eta^2 - \eta^2 \cot^2(\eta - B) - 2\eta^3\cot(\eta-B) - 2\eta^3\cot^3(\eta-B) </math>
	<math>~=</math>	<math>~ 2\biggl[ 3 - \eta^2 - (\eta + \eta^3)\cot(\eta-B) - \eta^2\cot^2(\eta - B) - \eta^3\cot^3(\eta-B) \biggr] \, . </math>

Recalling that,

<math>~Q = \biggl[1- \eta\cot(\eta-B) \biggr] \, ,</math>

plugging these expressions into the relevant envelope LAWE gives,

LAWE	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -2Q \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} ~-~ 2 Q \cdot \frac{x}{\eta^2} </math>
	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -2 \biggl[1- \eta\cot(\eta-B) \biggr]\biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \frac{2x}{\eta^2} </math>
	<math>~=</math>	<math>~\frac{b}{\eta^4} \biggl\{ \frac{\eta^4}{b} \cdot \frac{d^2x}{d\eta^2} + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \frac{2\eta^3}{b} \cdot \frac{dx}{d\eta} ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \frac{2\eta^2 x}{b} \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ 3 - \eta^2 - (\eta + \eta^3)\cot(\eta-B) - \eta^2\cot^2(\eta - B) - \eta^3\cot^3(\eta-B) </math>
		<math>~ + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \biggl[\eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B)\biggr] ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \biggl[1- \eta\cot(\eta-B) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ 3 - \eta^2 - (\eta + \eta^3)\cot(\eta-B) - \eta^2\cot^2(\eta - B) - \eta^3\cot^3(\eta-B) + \biggl[\eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B)\biggr] ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] </math>
		<math>~ + \eta\cot(\eta-B) \biggl[\eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B)\biggr] ~+~\eta\cot(\eta-B) \biggl[1- \eta\cot(\eta-B) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ - (\eta + \eta^3)\cot(\eta-B) - \eta^3\cot^3(\eta-B) ~+~2\eta\cot(\eta-B) </math>
		<math>~ + \eta^3\cot(\eta-B) -2 \eta\cot(\eta-B) + \eta^2\cot^2(\eta-B) + \eta^3\cot^3(\eta - B) ~+~\eta\cot(\eta-B) ~-~\eta^2\cot^2(\eta-B) \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ [- \eta \cot(\eta-B) - \eta\cot(\eta-B) ~+~2\eta\cot(\eta-B) ] + [\eta^3\cot(\eta-B) - \eta^3 \cot(\eta-B) ] </math>
		<math>~ + [\eta^2\cot^2(\eta-B) ~-~\eta^2\cot^2(\eta-B) ] + [\eta^3\cot^3(\eta - B) - \eta^3\cot^3(\eta-B) ] \biggr\} </math>
	<math>~=</math>	<math>~0 \, .</math>

Okay. Now let's determine at what value of <math>~\eta</math> the logarithmic derivative of <math>~x_P</math> goes to negative one.

<math>~\frac{d\ln x_P}{d\ln \eta} = \frac{\eta}{x_P} \cdot \frac{dx_P}{d\eta} </math>	<math>~=</math>	<math>~\frac{\eta^3}{b }\biggl[1- \eta\cot(\eta-B) \biggr]^{-1} \cdot \frac{dx_P}{d\eta} </math>
	<math>~=</math>	<math>~ \biggl[1- \eta\cot(\eta-B) \biggr]^{-1} \biggl[ \eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B) \biggr] \, . </math>

Setting this to negative one, we have,

<math>~ -\biggl[1- \eta\cot(\eta-B) \biggr] </math>	<math>~=</math>	<math>~ \biggl[ \eta^2 -2 + \eta\cot(\eta-B) + \eta^2\cot^2(\eta - B) \biggr] </math>
<math>~ \Rightarrow~~~1 </math>	<math>~=</math>	<math>~ \eta^2\biggl[ 1 + \cot^2(\eta - B) \biggr] </math>
	<math>~=</math>	<math>~ \eta^2\biggl[ \frac{1}{\sin^2(\eta - B)} \biggr] </math>
<math>~ \Rightarrow~~~1 </math>	<math>~=</math>	<math>~ \frac{\eta^2}{\sin^2(\eta - B)} \, . </math>

And this occurs when,

<math>~\biggl(\frac{A}{\phi } \biggr)^2 = 1 \, .</math>

Third Attempt

Prior to the Brute-Force Trial Fit

Let's work through the analytic derivatives again. Keeping in mind that,

<math>~\frac{d}{d\eta}\biggl[\cot(\eta - C) \biggr]</math>

<math>~ - \biggl[ 1 + \cot^2(\eta - C)\biggr] \, , </math>

and starting with the guess,

<math>~ \frac{b}{\eta^2} \biggl[1- \eta\cot(\eta-C) \biggr] \, , </math>

we have,

<math>~ \biggl( \frac{\eta^3}{b} \biggr) \frac{dx_P}{d\eta}</math>

and,

<math>~ 2\biggl[ 3 - \eta^2 - (\eta + \eta^3)\cot(\eta-C) - \eta^2\cot^2(\eta - C) - \eta^3\cot^3(\eta-C) \biggr] \, . </math>

Note that the relevant logarithmic derivative is,

<math>~

\biggl( \frac{b}{\eta^2} \biggr)\biggl[ \eta^2 -2 + \eta\cot(\eta-C)  +  \eta^2\cot^2(\eta - C) \biggr]x_P^{-1}

</math>

<math>~ \biggl[ \eta^2 -2 + \eta\cot(\eta-C) + \eta^2\cot^2(\eta - C) \biggr]\biggl[1- \eta\cot(\eta-C) \biggr]^{-1} </math>

If we know the logarithmic slope and the value of <math>~\eta</math> at the interface, then we can solve for

<math>~y_i \equiv \eta_i \cot(\eta_i-C) \, ,</math>

via the quadratic relation,

<math>~(1- y_i ) \biggl[\frac{d\ln x_P}{d\ln\eta}\biggr]_i</math>

<math>~\Rightarrow~~~0</math>

<math>~ \eta_i^2 -2 + y_i + y_i^2 - (1- y_i ) \biggl[\frac{d\ln x_P}{d\ln\eta}\biggr]_i </math>

<math>~

y_i^2

+ y_i \biggl\{1 + \biggl[\frac{d\ln x_P}{d\ln\eta}\biggr]_i\biggr\} +\biggl\{ \eta_i^2 -2 - \biggl[\frac{d\ln x_P}{d\ln\eta}\biggr]_i \biggr\} \, . </math>

(In practice it appears as though the "plus" solution to this quadratic equation is desired if the quantity inside the last set of curly braces is positive; and the "minus" solution is desired if this quantity is negative.) Once the value of <math>~y_i</math> is known, we can solve for the key coefficient, <math>~C</math>, via the relation,

<math>~\tan(\eta_i - C)</math>	<math>~=</math>	<math>~ \frac{\eta_i}{y_i}</math>
<math>~\Rightarrow ~~~C</math>	<math>~=</math>	<math>~\eta_i - \tan^{-1}\biggl(\frac{\eta_i}{y_i}\biggr)\, .</math>

Recalling that,

<math>~Q = \biggl[1- \eta\cot(\eta-B) \biggr] \, ,</math>

plugging these expressions into the relevant envelope LAWE gives,

LAWE	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -2Q \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} ~-~ 2 Q \cdot \frac{x}{\eta^2} </math>
	<math>~=</math>	<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -2 \biggl[1- \eta\cot(\eta-B) \biggr]\biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \frac{2x}{\eta^2} </math>
	<math>~=</math>	<math>~\frac{b}{\eta^4} \biggl\{ \frac{\eta^4}{b} \cdot \frac{d^2x}{d\eta^2} + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \frac{2\eta^3}{b} \cdot \frac{dx}{d\eta} ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \frac{2\eta^2 x}{b} \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ 3 - \eta^2 - (\eta + \eta^3)\cot(\eta-C) - \eta^2\cot^2(\eta - C) - \eta^3\cot^3(\eta-C) </math>
		<math>~ + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \biggl[\eta^2 -2 + \eta\cot(\eta-C) + \eta^2\cot^2(\eta - C)\biggr] ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \biggl[1- \eta\cot(\eta-C) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ 3 - \eta^2 - (\eta + \eta^3)\cot(\eta-C) - \eta^2\cot^2(\eta - C) - \eta^3\cot^3(\eta-C) + \biggl[\eta^2 -2 + \eta\cot(\eta-C) + \eta^2\cot^2(\eta - C)\biggr] ~-~ \biggl[1- \eta\cot(\eta-C) \biggr] </math>
		<math>~ + \eta\cot(\eta-B) \biggl[\eta^2 -2 + \eta\cot(\eta-C) + \eta^2\cot^2(\eta - C)\biggr] ~+~\eta\cot(\eta-B) \biggl[1- \eta\cot(\eta-C) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ (\eta - \eta^3)\cot(\eta-C) - \eta^3\cot^3(\eta-C) + \eta\cot(\eta-B) \biggl[\eta^2 -1 + \eta^2\cot^2(\eta - C) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl\{ (\eta - \eta^3) [ \cot(\eta-C) - \cot(\eta-B) ] + \eta^3 \cot^2(\eta - C) [\cot(\eta-B)- \cot(\eta-C)] \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^4} \biggl[ \cot(\eta-C) - \cot(\eta-B) \biggr] \biggl[ \eta - \eta^3 - \eta^3 \cot^2(\eta - C) \biggr] </math>
	<math>~=</math>	<math>~\frac{2b}{\eta^3} \biggl[ \cot(\eta-C) - \cot(\eta-B) \biggr] \biggl\{ 1 - \eta^2\biggl[1 + \cot^2(\eta - C)\biggr] \biggr\}\, . </math>

This will go to zero if <math>~C = (B-2m\pi), </math> where <math>~m</math> is a positive integer. When <math>~m =1</math>, for example,

Okay. Now let's determine at what value of <math>~\eta</math> the logarithmic derivative of <math>~x_P</math> goes to negative one.

Following Up on the Brute-Force Trial Fit

In an accompanying discussion — see especially Attempt #2 — we have determined by visual inspection that a decent fit to the envelope's eigenfunction is given by the expression,

<math>~x_\mathrm{trial}</math>	<math>~=</math>	<math>~ \frac{b_0}{\Lambda^2} \biggl\{ 1 - \Lambda \biggl[ \frac{\tan(\eta_i - \Lambda - 3\pi/4) + f_\alpha}{1 - f_\alpha \cdot \tan(\eta_i - \Lambda - 3\pi/4)} \biggr] \biggr\} - a_0 </math>
	<math>~=</math>	<math>~ \frac{b_0}{\Lambda^2} \biggl\{ 1 - \Lambda \cot(\Lambda - E)\biggr\} - a_0 \, , </math>

Limiting Parameter Values
	min	max	<math>~\alpha = \alpha_s</math>
<math>~\eta_\mathrm{F}</math>	<math>~\eta_i</math>	<math>~\eta_s</math>	<math>~\frac{8}{\pi} ( \eta_s - \eta_i )^2 + 2\eta_s - \eta_i</math>
<math>~\alpha</math>	<math>~-\frac{\pi}{2}</math>	<math>~-\frac{5\pi}{8}</math>	<math>~\eta_i - \eta_s - \frac{3\pi}{4}</math>
<math>~\Lambda</math>	<math>~\eta_i - \frac{\pi}{4}</math>	<math>~\eta_i - \frac{\pi}{8}</math>	<math>~\eta_s</math>

where, over the range, <math>~\eta_i \le \eta \le \eta_s \, ,</math>

<math>~E</math>	<math>~\equiv</math>	<math>~\eta_i - \frac{5\pi}{4} + \tan^{-1} f_\alpha \, ,</math>
<math>~\Lambda(\eta)</math>	<math>~\equiv</math>	<math>~ \eta_i + g_\mathrm{F} \biggl[ \eta_i - 2\eta_s + \eta \biggr] = \Lambda_0 + g_\mathrm{F}\eta \, ,</math>
<math>~\frac{1}{f_\alpha} = \tan(\alpha_s)</math>	<math>~\equiv</math>	<math>~ \tan[ - (\eta_s - \eta_i + \tfrac{3\pi}{4}) ] \, ,</math>
<math>~g_\mathrm{F}</math>	<math>~\equiv</math>	<math>~ \frac{\pi}{8(\eta_s - \eta_i)} \, .</math>

Let's examine the first and second derivatives of this trial eigenfunction, recognizing that,

<math>~\frac{dx_\mathrm{trial}}{d\eta} = \frac{d\Lambda}{d\eta} \cdot \frac{dx_\mathrm{trial}}{d\Lambda}= g_\mathrm{F} \cdot \frac{dx_\mathrm{trial}}{d\Lambda}</math>

and

<math>~\frac{d^2x_\mathrm{trial}}{d\eta^2} = \frac{d\Lambda}{d\eta} \cdot \frac{d}{d\Lambda} \biggl[ g_\mathrm{F}\cdot \frac{dx_\mathrm{trial}}{d\Lambda} \biggr] = g_\mathrm{F}^2 \cdot \frac{d^2x_\mathrm{trial}}{d\Lambda^2} \, . </math>

and drawing from the derivative expressions already derived, above. For the first derivative, we have,

<math>~\frac{dx_\mathrm{trial}}{d\eta}</math>

<math>~ g_\mathrm{F} \biggl( \frac{b_0}{\Lambda^3} \biggr) \biggl[ \Lambda ^2 -2 + \Lambda\cot(\Lambda-E) + \Lambda^2\cot^2(\Lambda - E) \biggr] \, . </math>

And the second derivative gives,

<math>~\frac{d^2x_\mathrm{trial}}{d\eta^2}</math>

<math>~ g_\mathrm{F}^2 \biggl(\frac{2b_0}{\Lambda^4} \biggr) \biggl[ 3 - \Lambda^2 - (\Lambda + \Lambda^3)\cot(\Lambda-E) - \Lambda^2\cot^2(\Lambda - E) - \Lambda^3\cot^3(\Lambda-E) \biggr] \, . </math>

Hence,

LAWE	<math>~=</math>	<math>~ \frac{d^2x_\mathrm{trial}}{d\eta^2} + \biggl\{ 4 -2Q \biggr\}\frac{1}{\eta} \cdot \frac{dx_\mathrm{trial}}{d\eta} ~-~ 2 Q \cdot \frac{x_\mathrm{trial}}{\eta^2} </math>
	<math>~=</math>	<math>~ \frac{d^2x_\mathrm{trial}}{d\eta^2} + \biggl\{ 4 -2 \biggl[1- \eta\cot(\eta-B) \biggr]\biggr\}\frac{1}{\eta} \cdot \frac{dx_\mathrm{trial}}{d\eta} ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \frac{2x_\mathrm{trial}}{\eta^2} </math>
	<math>~=</math>	<math>~\frac{b_0}{\eta^4} \biggl\{ \frac{\eta^4}{b_0} \cdot \frac{d^2x_\mathrm{trial}}{d\eta^2} + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \frac{2\eta^3}{b_0} \cdot \frac{dx_\mathrm{trial}}{d\eta} ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \frac{2\eta^2 x_\mathrm{trial}}{b_0} \biggr\} </math>
	<math>~=</math>	<math>~\frac{b_0}{\eta^4} \biggl\{ \frac{\eta^4}{b_0} \cdot g_\mathrm{F}^2 \biggl(\frac{2b_0}{\Lambda^4} \biggr) \biggl[ 3 - \Lambda^2 - (\Lambda + \Lambda^3)\cot(\Lambda-E) - \Lambda^2\cot^2(\Lambda - E) - \Lambda^3\cot^3(\Lambda-E) \biggr] </math>
		<math>~ + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \frac{2\eta^3}{b_0} \cdot g_\mathrm{F} \biggl( \frac{b_0}{\Lambda^3} \biggr) \biggl[ \Lambda ^2 -2 + \Lambda\cot(\Lambda-E) + \Lambda^2\cot^2(\Lambda - E) \biggr] </math>
		<math>~ ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \frac{2\eta^2 }{b_0} \cdot \biggl[\frac{b_0}{\Lambda^2} \biggl\{ 1 - \Lambda \cot(\Lambda - E)\biggr\} - a_0\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{b_0}{\eta^4} \biggl\{ g_\mathrm{F}^2 \biggl(\frac{2\eta^4}{\Lambda^4} \biggr) \biggl[ 3 - \Lambda^2 - (\Lambda + \Lambda^3)\cot(\Lambda-E) - \Lambda^2\cot^2(\Lambda - E) - \Lambda^3\cot^3(\Lambda-E) \biggr] </math>
		<math>~ + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \cdot g_\mathrm{F} \biggl( \frac{2\eta^3}{\Lambda^3} \biggr) \biggl[ \Lambda ^2 -2 + \Lambda\cot(\Lambda-E) + \Lambda^2\cot^2(\Lambda - E) \biggr] </math>
		<math>~ ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \biggl[\frac{2\eta^2}{\Lambda^2} [ 1 - \Lambda \cot(\Lambda - E) ] - \frac{2\eta^2 a_0}{b_0} \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{2b_0}{\Lambda^4\eta^2} \biggl\{ g_\mathrm{F}^2 \eta^2 \biggl[ 3 - \Lambda^2 - (\Lambda + \Lambda^3)\cot(\Lambda-E) - \Lambda^2\cot^2(\Lambda - E) - \Lambda^3\cot^3(\Lambda-E) \biggr] </math>
		<math>~ + \biggl[ 1 + \eta\cot(\eta-B) \biggr] \cdot g_\mathrm{F} \Lambda \eta \biggl[ \Lambda ^2 -2 + \Lambda\cot(\Lambda-E) + \Lambda^2\cot^2(\Lambda - E) \biggr] </math>
		<math>~ ~-~ \biggl[1- \eta\cot(\eta-B) \biggr] \biggl[\Lambda^2 [ 1 - \Lambda \cot(\Lambda - E) ] - \frac{a_0\Lambda^4}{b_0} \biggr] \biggr\} </math>

<math>~\Rightarrow~~~\biggl(\frac{\Lambda^4\eta^2}{2b_0}\biggr) \cdot</math> LAWE	<math>~=</math>	<math>~ g_\mathrm{F}^2 \eta^2 \biggl[ 3 - \Lambda^2 - (\Lambda + \Lambda^3)\cot(\Lambda-E) - \Lambda^2\cot^2(\Lambda - E) - \Lambda^3\cot^3(\Lambda-E) \biggr] </math>
		<math>~ + g_\mathrm{F} \Lambda \eta \biggl[ \Lambda ^2 -2 + \Lambda\cot(\Lambda-E) + \Lambda^2\cot^2(\Lambda - E) \biggr] ~-~ \biggl[\Lambda^2 [ 1 - \Lambda \cot(\Lambda - E) ] - \frac{a_0\Lambda^4}{b_0} \biggr] </math>
		<math>~ + \biggl[ \eta\cot(\eta-B) \biggr] \biggl\{ g_\mathrm{F} \Lambda \eta \biggl[ \Lambda ^2 -2 + \Lambda\cot(\Lambda-E) + \Lambda^2\cot^2(\Lambda - E) \biggr] ~+~ \Lambda^2 [ 1 - \Lambda \cot(\Lambda - E) ] - \frac{a_0\Lambda^4}{b_0} \biggr\} </math>

Fourth Attempt

XXXX

If we assume that, <math>~\alpha_e = (3 - 4/2) = 1</math> and <math>~\sigma_c^2 = 0</math>, then the relevant envelope LAWE is,

<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -2Q \biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} ~-~ \biggl[ 2 Q \biggr] \frac{x}{\eta^2} \, , </math>

where,

<math>~Q \equiv - \frac{d \ln \phi}{ d\ln \eta}</math>

<math>~ \biggl[1- \eta\cot(\eta-B_0) \biggr] \, . </math>

Let's work through the analytic derivatives again. Keeping in mind that,

<math>~\frac{d}{d\eta}\biggl[\cot(\eta - B) \biggr]</math>

<math>~ - \biggl[ 1 + \cot^2(\eta - B)\biggr] \, ; </math>

and that the,

Precise Solution to the Polytropic LAWE
<math>~x_P</math>	<math>~=</math>	<math>~\frac{b(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{1}{\eta \phi^{n}}\biggr) \frac{d\phi}{d\eta}\biggr]</math>
	<math>~=</math>	<math>~-b\biggl[ \biggl( \frac{1}{\eta \phi}\biggr) \frac{d\phi}{d\eta}\biggr]</math>
	<math>~=</math>	<math>~\frac{b}{\eta^2}\biggl[ -\frac{d\ln \phi}{d\ln \eta}\biggr] </math>
	<math>~=</math>	<math>~\frac{bQ}{\eta^2} </math>
<math>~\Rightarrow ~~~ x_P</math>	<math>~=</math>	<math>~ \frac{b}{\eta^2} \biggl[1- \eta\cot(\eta-B_0) \biggr] \, . </math>

As we have already tried once, above, let's try a more general form of this expression, namely,

<math>~ A + \frac{C}{(\eta - F)^2} \biggl[1 - (\eta-D) \cot(\eta-B) \biggr] \, . </math>

Hence,

<math>~\frac{dx_Q}{d\eta}</math>	<math>~=</math>	<math>~ \biggl[1 - (\eta-D) \cot(\eta-B) \biggr] \frac{d}{d\eta}\biggl[ \frac{C}{(\eta - F)^2} \biggr] - \frac{C}{(\eta - F)^2} \frac{d}{d\eta} \biggl[ (\eta-D) \cot(\eta-B) \biggr] </math>
	<math>~=</math>	<math>~ \biggl[1 - (\eta-D) \cot(\eta-B) \biggr]\biggl[ \frac{-2C}{(\eta - F)^3} \biggr] - \frac{C}{(\eta - F)^2} \biggl[ \cot(\eta-B) \biggr] + \frac{C(\eta - D)}{(\eta - F)^2}\biggl[1 + \cot^2(\eta - B)\biggr] </math>
	<math>~=</math>	<math>~\frac{C}{(\eta-F)^3} \biggl\{ - 2 + 2(\eta-D) \cot(\eta-B) - (\eta - F) \biggl[ \cot(\eta-B) \biggr] + (\eta - D)(\eta - F) \biggl[1 + \cot^2(\eta - B)\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{C}{(\eta-F)^3} \biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} \, . </math>

And,

<math>~\frac{d^2x_Q}{d\eta^2}</math>	<math>~=</math>	<math>~\biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} \frac{d}{d\eta}\biggl[\frac{C}{(\eta-F)^3} \biggr] </math>
		<math>~+\frac{C}{(\eta-F)^3} \cdot \frac{d}{d\eta} \biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} </math>
	<math>~=</math>	<math>~\biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} \biggl[\frac{-3C}{(\eta-F)^4} \biggr] </math>
		<math>~+\frac{C}{(\eta-F)^3} \biggl\{ [2\eta - (D+F) ] + \cot(\eta-B) - (\eta - 2D + F) \biggl[1 + \cot^2(\eta-B) \biggr] + [2\eta -(D+F) ] \cot^2(\eta - B) - 2[\eta^2 -\eta(D+F) + DF]\cot(\eta - B)\biggl[1 + \cot^2(\eta - B)\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{C}{(\eta-F)^4} \biggl\{ -3[(\eta - D)(\eta - F) - 2] - 3(\eta - 2D + F) \cot(\eta-B) - 3 (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} </math>
		<math>~+\frac{C}{(\eta-F)^3} \biggl\{ [2\eta - (D+F) ] - (\eta - 2D + F) + \cot(\eta-B) - (\eta - 2D + F) \cot^2(\eta-B) + [2\eta -(D+F) ] \cot^2(\eta - B) - 2[\eta^2 -\eta(D+F) + DF]\cot(\eta - B) - 2[\eta^2 -\eta(D+F) + DF]\cot^3(\eta - B) \biggr\} </math>

YYYY

And,

<math>~\frac{d^2 x_Q}{d\eta^2}</math>	<math>~=</math>	<math>~\biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} \frac{d}{d\eta}\biggl[\frac{C}{(\eta-F)^3} \biggr] </math>
		<math>~ +\frac{C}{(\eta-F)^3} \cdot \frac{d}{d\eta}\biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} </math>
	<math>~=</math>	<math>~\biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\}\biggl[\frac{-3C}{(\eta-F)^4} \biggr] </math>
		<math>~ +\frac{C}{(\eta-F)^3} \cdot \biggl\{ \frac{d}{d\eta}\biggl[ (\eta - 2D + F) \cot(\eta-B) \biggr] +\frac{d}{d\eta}\biggl[ (\eta - D)(\eta - F) \cot^2(\eta - B)\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{C}{(\eta-F)^4} \biggl\{ -3[(\eta - D)(\eta - F) - 2] -3(\eta - 2D + F) \cot(\eta-B) -3(\eta - D)(\eta - F) \cot^2(\eta - B) </math>
		<math>~ + (\eta-F)\frac{d}{d\eta}\biggl[ (\eta - 2D + F) \cot(\eta-B) \biggr] +(\eta-F)\frac{d}{d\eta}\biggl[ (\eta - D)(\eta - F) \cot^2(\eta - B)\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{C}{(\eta-F)^4} \biggl\{ -3[(\eta - D)(\eta - F) - 2] -3(\eta - 2D + F) \cot(\eta-B) -3(\eta - D)(\eta - F) \cot^2(\eta - B) </math>
		<math>~ + (\eta-F) \cot(\eta-B) \frac{d}{d\eta}\biggl[ (\eta - 2D + F) \biggr] + (\eta-F) (\eta - 2D + F) \frac{d}{d\eta}\biggl[ \cot(\eta-B) \biggr] </math>
		<math>~ +(\eta-F) \cot^2(\eta - B) \frac{d}{d\eta}\biggl[ \eta^2 -\eta(D+F) + DF \biggr] +(\eta-F) (\eta - D)(\eta - F) \frac{d}{d\eta}\biggl[ \cot^2(\eta - B)\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{C}{(\eta-F)^4} \biggl\{ -3[(\eta - D)(\eta - F) - 2] -3(\eta - 2D + F) \cot(\eta-B) -3(\eta - D)(\eta - F) \cot^2(\eta - B) </math>
		<math>~ + (\eta-F) \cot(\eta-B) - (\eta-F) (\eta - 2D + F) \biggl[ 1 + \cot^2(\eta - B)\biggr] </math>
		<math>~ +(\eta-F) \cot^2(\eta - B) \biggl[ 2\eta - (D+F) \biggr] -2 (\eta-F) (\eta - D)(\eta - F) \cot(\eta - B)\biggl[ 1 + \cot^2(\eta - B)\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{C}{(\eta-F)^4} \biggl\{ -3[(\eta - D)(\eta - F) - 2] - (\eta-F) (\eta - 2D + F) </math>
		<math>~ + \biggl[ (\eta-F) -3(\eta - 2D + F) -2 (\eta-F) (\eta - D)(\eta - F)\biggr] \cot(\eta - B) </math>
		<math>~ +\biggl[ (\eta-F) [ 2\eta - (D+F) ] -3(\eta - D)(\eta - F) -2 (\eta-F) (\eta - D)(\eta - F) \cot(\eta - B) - (\eta-F) (\eta - 2D + F)\biggr] \cot^2(\eta - B) \biggr\} </math>

So the envelope LAWE becomes,

<math>~\frac{(\eta-F)^4}{C} \cdot \mathrm{LAWE}</math>	<math>~=</math>	<math>~ \frac{(\eta-F)^4}{C} \cdot \frac{d^2x_Q}{d\eta^2} + \frac{(\eta-F)^4}{C} \biggl[ 1 + \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta} \cdot \frac{dx_Q}{d\eta} ~-~ \frac{(\eta-F)^4}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \frac{2x_Q}{\eta^2} </math>
	<math>~=</math>	<math>~ \biggl\{ -3[(\eta - D)(\eta - F) - 2] - (\eta-F) (\eta - 2D + F) + \biggl[ (\eta-F) -3(\eta - 2D + F) -2 (\eta-F) (\eta - D)(\eta - F)\biggr] \cot(\eta - B) </math>
		<math>~ +\biggl[ (\eta-F) [ 2\eta - (D+F) ] -3(\eta - D)(\eta - F) -2 (\eta-F) (\eta - D)(\eta - F) \cot(\eta - B) - (\eta-F) (\eta - 2D + F)\biggr] \cot^2(\eta - B) \biggr\} </math>
		<math>~ + (\eta-F) \biggl[ 1 + \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta} \biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) + (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr\} </math>
		<math>~ ~-~ \frac{(\eta-F)^4}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta^2} \biggl\{ A + \frac{C}{(\eta - F)^2} \biggl[1 - (\eta-D) \cot(\eta-B) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~ -3[(\eta - D)(\eta - F) - 2] - (\eta-F) (\eta - 2D + F) + \biggl[ (\eta-F) -3(\eta - 2D + F) -2 (\eta-F) (\eta - D)(\eta - F)\biggr] \cot(\eta - B) </math>
		<math>~ + (\eta-F) \biggl[ 1 + \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta} \biggl\{ [(\eta - D)(\eta - F) - 2] + (\eta - 2D + F) \cot(\eta-B) \biggr\} </math>
		<math>~ +\biggl[ (\eta-F) [ 2\eta - (D+F) ] -3(\eta - D)(\eta - F) -2 (\eta-F) (\eta - D)(\eta - F) \cot(\eta - B) - (\eta-F) (\eta - 2D + F)\biggr] \cot^2(\eta - B) </math>
		<math>~ + (\eta-F) \biggl[ 1 + \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta} \biggl[ (\eta - D)(\eta - F) \cot^2(\eta - B) \biggr] ~-~ (\eta-F)^2 \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta^2} \biggl[1 - (\eta-D) \cot(\eta-B) \biggr] </math>
		<math>~ ~-~ \frac{(\eta-F)^4}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \frac{2A}{\eta^2} \, . </math>

What does this reduce to if <math>~A = D = F = 0</math>.

<math>~\frac{\eta^4}{C} \cdot \mathrm{LAWE}</math>	<math>~=</math>	<math>~ -3[(\eta )(\eta ) - 2] - (\eta) (\eta ) + \biggl[ (\eta) -3(\eta ) -2 (\eta) (\eta )(\eta )\biggr] \cot(\eta - B) </math>
		<math>~ + (\eta) \biggl[ 1 + \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta} \biggl\{ [(\eta )(\eta ) - 2] + (\eta ) \cot(\eta-B) \biggr\} </math>
		<math>~ +\biggl[ (\eta) [ 2\eta ] -3(\eta )(\eta ) -2 (\eta) (\eta )(\eta) \cot(\eta - B) - (\eta-) (\eta )\biggr] \cot^2(\eta - B) </math>
		<math>~ + (\eta) \biggl[ 1 + \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta} \biggl[ (\eta )(\eta ) \cot^2(\eta - B) \biggr] ~-~ (\eta)^2 \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \frac{2}{\eta^2} \biggl[1 - (\eta) \cot(\eta-B) \biggr] </math>
		<math>~ ~-~ \frac{(\eta)^4}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \frac{2A}{\eta^2} \, . </math>
	<math>~=</math>	<math>~ 6 - 4\eta^2 -2 (\eta + \eta^3 ) \cot(\eta - B) + 2 \biggl[ 1 + \eta\cot(\eta-B_0) \biggr] \biggl[ \eta^2 - 2 + \eta \cot(\eta-B) \biggr] - 2\biggl[ \eta^2 + \eta^3 \cot(\eta - B) \biggr] \cot^2(\eta - B) </math>
		<math>~ + 2 \biggl[ 1 + \eta\cot(\eta-B_0) \biggr]\biggl[ \eta^2 \cot^2(\eta - B) \biggr] ~-~2 \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \biggl[1 - \eta \cot(\eta-B) \biggr] ~-~ \frac{2A\eta^2}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] </math>
	<math>~=</math>	<math>~ 6 - 4\eta^2 -2 (\eta + \eta^3 ) \cot(\eta - B) + 2\eta^2 - 4 + 2\eta \cot(\eta-B) + 2 \eta^3 \cot(\eta-B_0) ~-~4 \eta\cot(\eta-B_0) + 2 \eta^2\cot(\eta-B_0) \cot(\eta-B) - 2\eta^2 \cot^2(\eta - B) - 2 \eta^3 \cot^3(\eta - B) </math>
		<math>~ + 2 \eta^2 \cot^2(\eta - B) + 2 \eta^3 \cot(\eta-B_0) \cot^2(\eta - B) -2 + 4 \eta\cot(\eta-B_0) - 2\eta^2\cot^2(\eta-B_0) ~-~ \frac{2A\eta^2}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] </math>
	<math>~=</math>	<math>~ - 2\eta^2 -2 \eta^3 \biggl[ \cot(\eta - B) + \cot^3(\eta - B) \biggr] + \cot(\eta-B_0) \biggl[ 2 \eta^3 + 2 \eta^2 \cot(\eta-B) + 2 \eta^3 \cot^2(\eta - B) \biggr] - 2\eta^2\cot^2(\eta-B_0) ~-~ \frac{2A\eta^2}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] </math>
	<math>~=</math>	<math>~ - 2\eta^2 + 2 \eta^3\biggl[ \cot(\eta-B_0) - \cot(\eta-B)\biggr] \biggl[ 1 + \cot^2(\eta - B)\biggr] + 2 \eta^2 \cot(\eta-B_0)\biggl[ \cot(\eta-B) - \cot(\eta-B_0) \biggr] ~-~ \frac{2A\eta^2}{C} \biggl[ 1- \eta\cot(\eta-B_0) \biggr] \, . </math>

Related Discussions

Radial Oscillations of Uniform-density sphere
Radial Oscillations of Isolated Polytropes
- Setup
- n = 1: Attempt at Formulating an Analytic Solution
- n = 3: Numerical Solution to compare with M. Schwarzschild (1941)
- n = 5: Attempt at Formulating an Analytic Solution

In an accompanying Chapter within our "Ramblings" Appendix, we have played with the adiabatic wave equation for polytropes, examining its form when the primary perturbation variable is an enthalpy-like quantity, rather than the radial displacement of a spherical mass shell. This was done in an effort to mimic the approach that has been taken in studies of the stability of Papaloizou-Pringle tori.

<math>~n=3</math> … M. Schwarzschild (1941, ApJ, 94, 245), Overtone Pulsations of the Standard Model: This work is referenced in §38.3 of [KW94]. It contains an analysis of the radial modes of oscillation of <math>~n=3</math> polytropes, assuming various values of the adiabatic exponent.

<math>~n=2</math> … C. Prasad & H. S. Gurm (1961, MNRAS, 122, 409), Radial Pulsations of the Polytrope, n = 2

<math>~n=\tfrac{3}{2}</math> … D. Lucas (1953, Bul. Soc. Roy. Sci. Liege, 25, 585) … Citation obtained from the Prasad & Gurm (1961) article.

<math>~n=1</math> … L. D. Chatterji (1951, Proc. Nat. Inst. Sci. [India], 17, 467) … Citation obtained from the Prasad & Gurm (1961) article.

Composite Polytropes … M. Singh (1968, MNRAS, 140, 235-240), Effect of Central Condensation on the Pulsation Characteristics

Summary of Known Analytic Solutions … R. Stothers (1981, MNRAS, 197, 351-361), Analytic Solutions of the Radial Pulsation Equation for Rotating and Magnetic Star Models

Interesting Composite! … C. Prasad (1948, MNRAS, 108, 414-416), Radial Oscillations of a Particular Stellar Model

User:Tohline/SSC/Stability/n1PolytropeLAWE

Contents

Radial Oscillations of n = 1 Polytropic Spheres

Groundwork

Search for Analytic Solutions to the LAWE

Setup

Attempt at Deriving an Analytic Eigenvector Solution

First Guess (n1)

Second Guess (n1)

Third Guess (n1)

Fourth Guess (n1)

Fifth Guess (n1)

Example 1

Sixth Guess (n1)

Rationale

Resulting Polytropic Wave Equation

Graphical Reassessment

New Idea Involving Logarithmic Derivatives

Simplistic Layout

More general Assumption

Another Viewpoint

Development

Check for Mistakes

Motivated by Yabushita's Discovery

Initial Exploration

Succinct Demonstration

What About Bipolytropes?

First Attempt

Second Attempt

Third Attempt

Prior to the Brute-Force Trial Fit

Following Up on the Brute-Force Trial Fit

Fourth Attempt

XXXX

YYYY

Related Discussions

Navigation menu

Search