User:Tohline/SSC/UniformDensity

The Stability of Uniform-Density Spheres

The Eigenvalue Problem

Our Approach

As has been derived in an accompanying discussion, the second-order ODE that defines the relevant Eigenvalue problem is,

<math> \frac{d^2x}{d\chi_0^2} + \biggl[\frac{4}{\chi_0} - \biggl(\frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{P_0}{P_c}\biggr)^{-1} \biggl(\frac{g_0}{g_\mathrm{SSC}}\biggr) \biggr] \frac{dx}{d\chi_0} + \biggl(\frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{P_0}{P_c}\biggr)^{-1} \biggl(\frac{1}{\gamma_\mathrm{g}} \biggr)\biggl[\tau_\mathrm{SSC}^2 \omega^2 + (4 - 3\gamma_\mathrm{g})\biggl(\frac{g_0}{g_\mathrm{SSC}}\biggr) \frac{1}{\chi_0} \biggr] x = 0 . </math>

where the dimensionless radius,

<math> \chi_0 \equiv \frac{r_0}{R} , </math>

the characteristic time for dynamical oscillations in spherically symmetric configurations (SSC) is,

<math> \tau_\mathrm{SSC} \equiv \biggl[ \frac{R^2 \rho_c}{P_c} \biggr]^{1/2} , </math>

and the characteristic gravitational acceleration is,

<math> g_\mathrm{SSC} \equiv \frac{P_c}{R \rho_c} . </math>

The Approach Taken by Sterne (1937)

T. E. Sterne (1937) begins his analysis by deriving the

Adiabatic Wave (or Radial Pulsation) Equation

<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math>

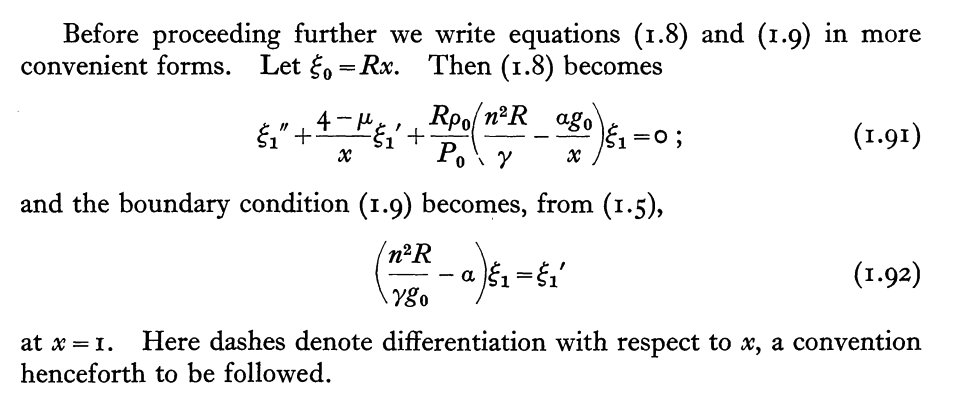
in a manner explicitly designed to reproduce Eddington's pulsation equation — it appears as equation (1.8) in Sterne's paper — and, along with it, the surface boundary condition,

<math>~ r_0 \frac{d\ln x}{dr_0}</math>

<math>~=</math>

<math>~\frac{1}{\gamma_g} \biggl( 4 - 3\gamma_g + \frac{\omega^2 R^3}{GM_\mathrm{tot}}\biggr) </math>        at         <math>~r_0 = R \, ,</math>

which appears in Sterne's paper as equation (1.9). Then, as shown in the following paragraph extracted directly from his paper, Sterne (1937) rewrites both of these expressions in, what he considers to be, "more convenient forms."

Paragraph extracted from T. E. Sterne (1937) "Modes of Radial Oscillation" Monthly Notices of the Royal Astronomical Society, vol. 97, pp. 582 - © Royal Astronomical Society
Notation: Sterne's   Ours <math>~\xi_0 = Rx</math>   <math>~r_0</math> <math>~\xi_1</math>   <math>~x</math> <math>~n^2</math>   <math>~\omega^2</math> <math>~\alpha</math>   <math>~3-4/\gamma_g</math> <math>~\mu</math>   <math>~g_0 \rho_0 r_0/P_0</math> <math>~g_0 R^2</math>   <math>~GM_\mathrm{tot}</math>

Properties of the Equilibrium Configuration

Our Setup

From our derived structure of a uniform-density sphere, in terms of the configuration's radius <math>R</math> and mass <math>M</math>, the central pressure and density are, respectively,

<math>P_c = \frac{3G}{8\pi}\biggl( \frac{M^2}{R^4} \biggr) </math> ,

and

<math>\rho_c = \frac{3M}{4\pi R^3} </math> .

Hence the characteristic time and acceleration are, respectively,

<math> \tau_\mathrm{SSC} = \biggl[ \frac{R^2 \rho_c}{P_c} \biggr]^{1/2} = \biggl[ \frac{2R^3 }{GM} \biggr]^{1/2} = \biggl[ \frac{3}{2\pi G\rho_c} \biggr]^{1/2}, </math>

and,

<math> g_\mathrm{SSC} = \frac{P_c}{R \rho_c} = \biggl( \frac{GM}{2R^2} \biggr) . </math>

The required functions are,

• Density:

<math>\frac{\rho_0(r_0)}{\rho_c} = 1 </math> ;

• Pressure:

<math>\frac{P_0(r_0)}{P_c} = 1 - \chi_0^2 </math> ;

• Gravitational acceleration:

<math> \frac{g_0(r_0)}{g_\mathrm{SSC}} = 2\chi_0 . </math>

So our desired Eigenvalues and Eigenvectors will be solutions to the following ODE:

<math> \frac{1}{(1 - \chi_0^2)} \biggl\{ (1 - \chi_0^2) \frac{d^2x}{d\chi_0^2} + \frac{4}{\chi_0}\biggl[1 - \frac{3}{2}\chi_0^2 \biggr] \frac{dx}{d\chi_0} + \frac{1}{\gamma_\mathrm{g}} \biggl[\tau_\mathrm{SSC}^2 \omega^2 + 2 (4 - 3\gamma_\mathrm{g}) \biggr] x \biggr\} = 0 . </math>

Analytic Solution

First few lowest-order modes

• Mode 0:

<math>x_0 = \mathrm{constant}</math>, in which case,

<math> \omega_0^2 = - 2(4 - 3\gamma_\mathrm{g})\biggl[ \frac{2\pi G\rho_c}{3} \biggr] = 4\pi G \rho_c \biggl[ \gamma_\mathrm{g}- \frac{4}{3} \biggr] </math>

• Mode 1:

<math>x_1 = a + b\chi_0^2</math>, in which case,

<math> \frac{dx}{d\chi_0} = 2b\chi_0; ~~~~ \frac{d^2 x}{d\chi_0^2} = 2b; </math>

<math> \frac{1}{(1 - \chi_0^2)} \biggl\{ 2b (1 - \chi_0^2) + 8b \biggl[1 - \frac{3}{2}\chi_0^2 \biggr] + A_1 \biggl(1 + \frac{b}{a}\chi_0^2 \biggr) \biggr\} = 0 , </math>

where,

<math> A_1 \equiv \frac{a}{\gamma_\mathrm{g}}\biggl[ \biggl( \frac{3}{2\pi G\rho_c} \biggr) \omega_1^2+ 2(4 - 3\gamma_\mathrm{g}) \biggr] . </math>

Therefore,

<math> (A_1 + 10b) + \biggl[ \biggl(\frac{b}{a}\biggr) A_1 - 14b \biggr] \chi_0^2 = 0 , </math>

<math> \Rightarrow ~~~~~ A_1 = - 10b ~~~~~\mathrm{and} ~~~~~ A_1 = 14a </math>

<math> \Rightarrow ~~~~~ \frac{b}{a} = -\frac{7}{5} ~~~~~\mathrm{and} ~~~~~ \frac{A_1}{a} = 14 = \frac{1}{\gamma_\mathrm{g}}\biggl[ \biggl( \frac{3}{2\pi G\rho_c} \biggr) \omega_1^2+ 2(4 - 3\gamma_\mathrm{g}) \biggr] .

</math>

Hence,

<math> \biggl( \frac{3}{2\pi G\rho_c} \biggr) \omega_1^2 = 20\gamma_\mathrm{g} -8 </math>

<math> \Rightarrow ~~~~~ \omega_1^2 = \frac{2}{3}\biggl( 4\pi G\rho_c \biggr) (5\gamma_\mathrm{g} -2) </math>

and, to within an arbitrary normalization factor,

<math> x_1 = 1 - \frac{7}{5}\chi_0^2 . </math>

n=1 Polytrope

This discussion has been moved to another chapter.

© 2014 - 2021 by Joel E. Tohline |   H_Book Home   |   YouTube   | Appendices: | Equations | Variables | References | Ramblings | Images | myphys.lsu | ADS | Recommended citation:   Tohline, Joel E. (2021), The Structure, Stability, & Dynamics of Self-Gravitating Fluids, a (MediaWiki-based) Vistrails.org publication, https://www.vistrails.org/index.php/User:Tohline/citation