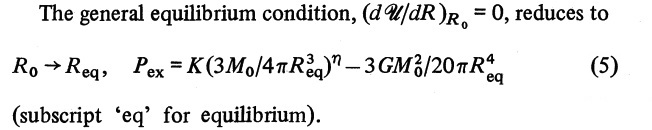Difference between revisions of "User:Tohline/SSC/Virial/Polytropes"
(Create new page discussing virial equilibrium of adiabatic spheres) |
(Move material on bounded adiabatic sphere from another page) |
||
| Line 132: | Line 132: | ||
</math> | </math> | ||
</div> | </div> | ||
==Nonrotating Configuration Embedded in an External Medium== | |||
For a nonrotating configuration <math>(C=J=0)</math> that is embedded in, and is influenced by the pressure <math>P_e</math> of, an external medium, the statement of virial equilibrium is, | |||
<div align="center"> | |||
<math> | |||
(1-\delta_{1\gamma_g})~3(\gamma_g-1) B\chi^{3 -3\gamma_g} +~ \delta_{1\gamma_g} B_I ~-~A\chi^{-1} -~ 3D\chi^3 = 0 \, . | |||
</math> | |||
</div> | |||
===Bounded Adiabatic=== | |||
For adiabatic configurations <math>(\delta_{1\gamma_g} = 0)</math>, equilibrium states exist at radii given by the roots of the following expression: | |||
<div align="center"> | |||
<math> | |||
3(\gamma_g-1) B\chi^{3 -3\gamma_g} ~-~A\chi^{-1} -~ 3D\chi^3 = 0 \, . | |||
</math> | |||
</div> | |||
====Whitworth's (1981) Equivalent Relation==== | |||
This is precisely the same condition that derives from setting equation (3) to zero in [http://adsabs.harvard.edu/abs/1981MNRAS.195..967W Whitworth's] (1981, MNRAS, 195, 967) discussion of the "global gravitational stability for one-dimensional polytropes." The overlap with Whitworth's narative is perhaps clearer after introducing the algebraic expressions for the coefficients <math>A</math>, <math>B</math>, and <math>D</math>, dividing the equation through by <math>(3\chi^3 V_0) = (4\pi R^3)</math>, and rewriting <math>R</math> as <math>R_\mathrm{eq}</math> to obtain, | |||
<div align="center"> | |||
<math> | |||
P_e = K \biggl( \frac{3M}{4\pi R_\mathrm{eq}^3} \biggr)^{\gamma_g} - \biggl( \frac{3GM^2}{20\pi R_\mathrm{eq}^4} \biggr) \, . | |||
</math> | |||
</div> | |||
This exactly matches equation (5) of [http://adsabs.harvard.edu/abs/1981MNRAS.195..967W Whitworth], which reads: | |||
<div align="center"> | |||
<table border="2"> | |||
<tr><td> | |||
[[File:Whitworth1981Eq5.jpg|500px|center|Whitworth (1981, MNRAS, 195, 967)]] | |||
</td></tr> | |||
</table> | |||
</div> | |||
====P-V Diagram==== | |||
Returning to the dimensionless form of this expression and multiplying through by <math>[-\chi/(3D)]</math>, we obtain, | |||
<div align="center"> | |||
<math> | |||
\chi^4 - (\gamma_g - 1)\frac{B}{D} \chi^{4-3\gamma_g} + \frac{A}{3D} = 0 \, . | |||
</math> | |||
</div> | |||
Writing the coefficient, <math>B</math>, in terms of the average sound speed and setting the radial scale factor equal to the equilibrium radius of an isolated adiabatic sphere, that is, setting, | |||
<div align="center"> | |||
<math> | |||
R_0 = \frac{GM}{5\bar{c_s}^2} \, , | |||
</math> | |||
</div> | |||
the equation governing the radii of ''adiabatic'' equilibrium states becomes, | |||
<div align="center"> | |||
<math> | |||
\chi^4 - \frac{1}{\Pi_a} \chi^{(4-3\gamma_g)} + \frac{1}{\Pi_a} = 0 \, , | |||
</math> | |||
</div> | |||
where, | |||
<div align="center"> | |||
<math> | |||
\Pi_a \equiv \frac{4\pi P_e G^3 M^2}{3\cdot 5^3 \bar{c_s}^8} \, . | |||
</math> | |||
</div> | |||
As in the isothermal case, for a given choice of configuration mass and sound speed, this parameter, <math>\Pi_a</math>, can be viewed as a dimensionless external pressure. Alternatively, for a given choice of <math>P_e</math> and <math>\bar{c_s}</math>, <math>\Pi_a^{1/2}</math> can represent a dimensionless mass; or, for a given choice of <math>M</math> and <math>P_e</math>, <math>\Pi_a^{-1/8}</math> can represent a dimensionless sound speed. Here we will view it as a dimensionless external pressure. | |||
Unlike the isothermal case, for an arbitrary value of the adiabatic exponent, <math>\gamma_g</math>, it isn't possible to invert this equation to obtain an analytic expression for <math>\chi</math> as a function of <math>\Pi_a</math>. But we can straightforwardly solve for <math>\Pi_a</math> as a function of <math>\chi</math>. The solution is, | |||
<div align="center"> | |||
<math> | |||
\Pi_a = \frac{\chi^{(4- 3\gamma_g)} - 1}{\chi^4} \, . | |||
</math> | |||
</div> | |||
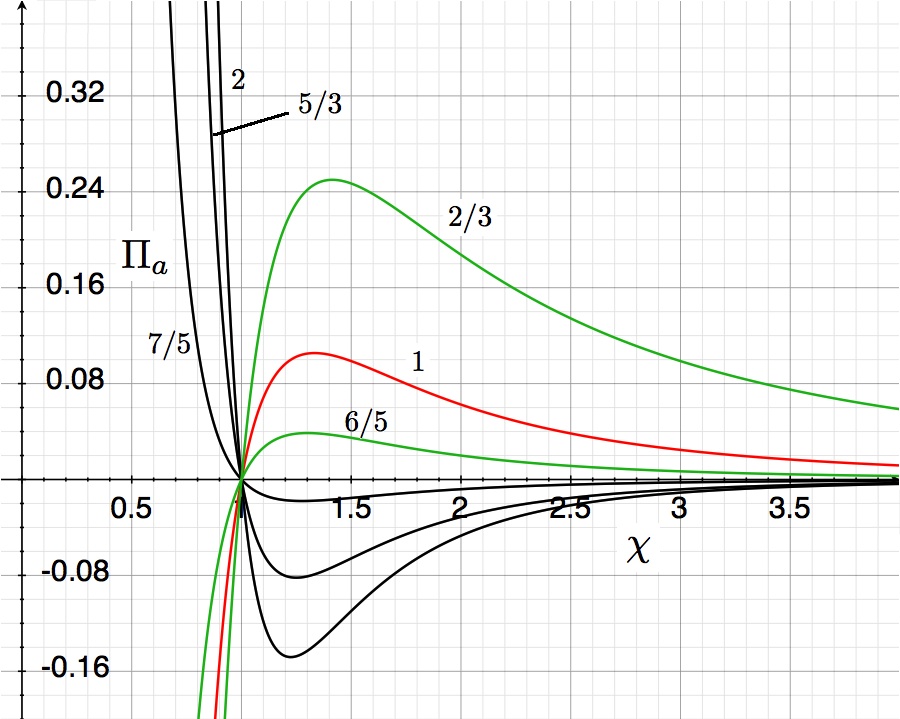
For physically relevant solutions, both <math>\chi</math> and <math>\Pi_a</math> must be nonnegative. Hence, as is illustrated by the curves in Figure 4, the physically allowable range of equilibrium radii is, | |||
<div align="center"> | |||
<math> | |||
1 \le \chi \le \infty \, ~~~~~\mathrm{for}~ \gamma_g < 4/3 \, ; | |||
</math> | |||
<math> | |||
0 < \chi \le 1 \, ~~~~~~\mathrm{for}~ \gamma_g > 4/3 \, . | |||
</math> | |||
</div> | |||
<div align="center"> | |||
<table border="2" cellpadding="8"> | |||
<tr> | |||
<td align="center" colspan="2"> | |||
'''Figure 4:''' <font color="darkblue">Equilibrium Adiabatic P-V Diagram </font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td valign="top" width=450 rowspan="1"> | |||
The curves trace out the function, | |||
<div align="center"> | |||
<math> | |||
\Pi_a = (\chi^{4-3\gamma_g} - 1)/\chi^4 \, , | |||
</math> | |||
</div> | |||
for six different values of <math>\gamma_g</math> (<math>2, ~5/3, ~7/5, ~6/5, ~1, ~2/3</math>, as labeled) and show the dimensionless external pressure, <math>\Pi_a</math>, that is required to construct a nonrotating, self-gravitating, uniform density, adiabatic sphere with an equilibrium radius <math>\chi</math>. The mathematical solution becomes unphysical wherever the pressure becomes negative. | |||
The solid red curve, drawn for the case <math>\gamma_g = 1</math>, is identical to the solid black (isothermal) curve displayed above in Figure 1. | |||
</td> | |||
<td align="center" bgcolor="white"> | |||
[[File:AdabaticBoundedSpheres_Virial.jpg|450px|center|Equilibrium Adiabatic P-R Diagram]] | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Each of the <math>\Pi_a(\chi)</math> curves drawn in Figure 4 exhibits an extremum. In each case this extremum occurs at a configuration radius, <math>\chi_\mathrm{extreme}</math>, given by, | |||
<div align="center"> | |||
<math> | |||
\frac{\partial\Pi_a}{\partial\chi} = 0 \, , | |||
</math> | |||
</div> | |||
that is, where, | |||
<div align="center"> | |||
<math> | |||
4 - 3\gamma_g \chi^{4-3\gamma_g} = 0 ~~~~\Rightarrow ~~~~~ \chi_\mathrm{extreme} = \biggl[ \frac{4}{3\gamma_g} \biggr]^{1/(4-3\gamma_g)} \, . | |||
</math> | |||
</div> | |||
For each value of <math>\gamma_g</math>, the corresponding dimensionless pressure is, | |||
<div align="center"> | |||
<math> | |||
\Pi_a \biggr|_\mathrm{extreme} = \biggl(\frac{4}{3\gamma} - 1 \biggr) \biggl[ \frac{3\gamma_g}{4} \biggr]^{4/(4-3\gamma_g)} \, . | |||
</math> | |||
</div> | |||
Note, first, that for <math>\gamma_g > 4/3</math>, an equilibrium configuration with a positive radius can be constructed for all physically realistic — that is, for all positive — values of <math>\Pi_a</math>. Also, consistent with the behavior of the curves shown in Figure 4, the extremum arises in the regime of physically relevant — ''i.e.,'' positive — pressures only for values of <math>\gamma_g < 4/3</math>; and in each case it represents a ''maximum'' limiting pressure. | |||
====Maximum Mass==== | |||
=====<math>n=5</math> Polytropic===== | |||
When <math>\gamma_a = 6/5</math> — which corresponds to an <math>n=5</math> polytropic configuration — we obtain, | |||
<div align="center"> | |||
<math> | |||
\Pi_\mathrm{max} = \Pi_a\biggr|_\mathrm{extreme}^{(\gamma_g = 6/5)} | |||
= \biggl( \frac{3^{18}}{2^{10}\cdot 5^{10}} \biggr) \, , | |||
</math> | |||
</div> | |||
which corresponds to a maximum mass for pressure-bounded <math>n=5</math> polytropic configurations of, | |||
<div align="center"> | |||
<math>M_\mathrm{max} = \Pi_\mathrm{max}^{1/2} \biggl(\frac{3\cdot 5^3}{2^2\pi} \biggr)^{1/2} \biggl( \frac{\bar{c_s}^8}{G^3 P_e} \biggr)^{1/2} | |||
= \biggl(\frac{3^{19}}{2^{12}\cdot 5^{7}\pi} \biggr)^{1/2} \biggl( \frac{\bar{c_s}^8}{G^3 P_e} \biggr)^{1/2} \, .</math> | |||
</div> | |||
This result can be compared to [[User:Tohline/SSC/Structure/LimitingMasses#Bounded_Isothermal_Sphere_.26_Bonnor-Ebert_Mass|other determinations of the Bonnor-Ebert mass limit]]. | |||
{{LSU_HBook_footer}} | {{LSU_HBook_footer}} | ||
Revision as of 21:31, 9 May 2014
Virial Equilibrium of Adiabatic Spheres

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Review
In an introductory discussion of the virial equilibrium structure of spherically symmetric configurations — see especially the section titled, Energy Extrema — we deduced that a system's equilibrium radius, <math>~R_\mathrm{eq}</math>, measured relative to a reference length scale, <math>~R_0</math>, i.e., the dimensionless equilibrium radius,
<math>~\chi_\mathrm{eq} \equiv \frac{R_\mathrm{eq}}{R_0} \, ,</math>
is given by the root(s) of the following equation:
<math> 2C \chi^{-2} + ~ (1-\delta_{1\gamma_g})~3(\gamma_g-1) B\chi^{3 -3\gamma_g} +~ \delta_{1\gamma_g} B_I ~-~A\chi^{-1} -~ 3D\chi^3 = 0 \, , </math>
where the definitions of the various coefficients are,
|
<math>~A</math> |
<math>~\equiv</math> |
<math>\frac{3}{5} \frac{GM_\mathrm{tot} ^2}{R_0} \cdot \mathfrak{f}_W \, ,</math> |
|
<math>~B</math> |
<math>~\equiv</math> |
<math> \frac{K M_\mathrm{tot} }{(\gamma_g-1)} \biggl( \frac{3M_\mathrm{tot} }{4\pi R_0^3} \biggr)^{\gamma_g - 1} \cdot \mathfrak{f}_A = \frac{\bar{c_s}^2 M_\mathrm{tot} }{(\gamma_g - 1)} \cdot \mathfrak{f}_A \, , </math> |
|
<math>~B_I</math> |
<math>~\equiv</math> |
<math> 3c_s^2 M_\mathrm{tot} \cdot \mathfrak{f}_M \, , </math> |
|
<math>~C</math> |
<math>~\equiv</math> |
<math> \frac{5J^2}{4M_\mathrm{tot} R_0^2} \cdot \mathfrak{f}_T \, , </math> |
|
<math>~D</math> |
<math>~\equiv</math> |
<math> \frac{4}{3} \pi R_0^3 P_e \, . </math> |
Once the pressure exerted by the external medium (<math>~P_e</math>), and the configuration's mass (<math>~M_\mathrm{tot}</math>), angular momentum (<math>~J</math>), and specific entropy (via <math>~K</math>) — or, in the isothermal case, sound speed (<math>~c_s</math>) — have been specified, the values of all of the coefficients are known and <math>~\chi_\mathrm{eq}</math> can be determined.
Isolated, Nonrotating Configuration
For a nonrotating configuration <math>(C=J=0)</math> that is not influenced by the effects of a bounding external medium <math>(D=P_e = 0)</math>, the statement of virial equilibrium is,
<math> (1-\delta_{1\gamma_g})~3(\gamma_g-1) B\chi^{3 -3\gamma_g} +~ \delta_{1\gamma_g} B_I ~-~A\chi^{-1} = 0 \, . </math>
Adiabatic
For adiabatic configurations <math>(\delta_{1\gamma_g} = 0)</math>, one equilibrium state exists for each value of <math>\gamma_g</math> and it occurs where,
<math> 3(\gamma_g-1) B\chi^{3 -3\gamma_g} = A\chi^{-1} \, , </math>
that is, where,
<math> R_\mathrm{eq} = R_0 \chi_\mathrm{eq} = \biggl[ \frac{3(\gamma_g-1) B}{A} \cdot R_0^{(3\gamma_g-4)} \biggr]^{1/(3\gamma_g-4)} = \biggl[ 5\biggl( \frac{3}{4\pi} \biggr)^{\gamma_g-1} \cdot \frac{KM^{(\gamma_g-2)}}{G} \biggr]^{1/(3\gamma_g-4)} \, . </math>
Accordingly, the equilibrium mass-radius relationship for adiabatic configurations of a given specific entropy is,
<math> M^{(\gamma_g - 2)} \propto R_\mathrm{eq}^{(3\gamma_g -4)} \, . </math>
Notice that, for <math>\gamma_g=2</math>, the equilibrium radius depends only on the specific entropy of the gas and is independent of the configuration's mass. Conversely, notice that, for <math>\gamma_g = 4/3</math>, the mass of the configuration is independent of the radius. For <math>\gamma_g</math> > <math> 2</math> or <math>\gamma_g </math>< <math>4/3</math>, configurations with larger mass (but the same specific entropy) have larger equilibrium radii. However, for <math>\gamma_g</math> in the range, <math>2</math> > <math>\gamma_g </math> > <math>4/3</math>, configurations with larger mass have smaller equilibrium radii. Note that the result obtained for the isothermal configuration could have been obtained by setting <math>\gamma_g = 1</math> in this adiabatic solution, because <math>K = c_s^2</math> when <math>\gamma_g = 1</math>.
It is also instructive to write the coefficient <math>B</math> in terms of the average sound speed as defined above. In this case,
<math> R_\mathrm{eq} = R_0 \biggl[ \frac{GM}{5 \bar{c_s}^2 R_0} \biggr]^{1/(4- 3\gamma_g)} \, , </math>
so the equilibrium radius of an isolated, nonrotating, uniform density, adiabatic sphere is,
<math> R_\mathrm{eq} = R_0 = \frac{GM}{5 \bar{c_s}^2 } \, . </math>
Nonrotating Configuration Embedded in an External Medium
For a nonrotating configuration <math>(C=J=0)</math> that is embedded in, and is influenced by the pressure <math>P_e</math> of, an external medium, the statement of virial equilibrium is,
<math> (1-\delta_{1\gamma_g})~3(\gamma_g-1) B\chi^{3 -3\gamma_g} +~ \delta_{1\gamma_g} B_I ~-~A\chi^{-1} -~ 3D\chi^3 = 0 \, . </math>
Bounded Adiabatic
For adiabatic configurations <math>(\delta_{1\gamma_g} = 0)</math>, equilibrium states exist at radii given by the roots of the following expression:
<math> 3(\gamma_g-1) B\chi^{3 -3\gamma_g} ~-~A\chi^{-1} -~ 3D\chi^3 = 0 \, . </math>
Whitworth's (1981) Equivalent Relation
This is precisely the same condition that derives from setting equation (3) to zero in Whitworth's (1981, MNRAS, 195, 967) discussion of the "global gravitational stability for one-dimensional polytropes." The overlap with Whitworth's narative is perhaps clearer after introducing the algebraic expressions for the coefficients <math>A</math>, <math>B</math>, and <math>D</math>, dividing the equation through by <math>(3\chi^3 V_0) = (4\pi R^3)</math>, and rewriting <math>R</math> as <math>R_\mathrm{eq}</math> to obtain,
<math> P_e = K \biggl( \frac{3M}{4\pi R_\mathrm{eq}^3} \biggr)^{\gamma_g} - \biggl( \frac{3GM^2}{20\pi R_\mathrm{eq}^4} \biggr) \, . </math>
This exactly matches equation (5) of Whitworth, which reads:
P-V Diagram
Returning to the dimensionless form of this expression and multiplying through by <math>[-\chi/(3D)]</math>, we obtain,
<math> \chi^4 - (\gamma_g - 1)\frac{B}{D} \chi^{4-3\gamma_g} + \frac{A}{3D} = 0 \, . </math>
Writing the coefficient, <math>B</math>, in terms of the average sound speed and setting the radial scale factor equal to the equilibrium radius of an isolated adiabatic sphere, that is, setting,
<math> R_0 = \frac{GM}{5\bar{c_s}^2} \, , </math>
the equation governing the radii of adiabatic equilibrium states becomes,
<math> \chi^4 - \frac{1}{\Pi_a} \chi^{(4-3\gamma_g)} + \frac{1}{\Pi_a} = 0 \, , </math>
where,
<math> \Pi_a \equiv \frac{4\pi P_e G^3 M^2}{3\cdot 5^3 \bar{c_s}^8} \, . </math>
As in the isothermal case, for a given choice of configuration mass and sound speed, this parameter, <math>\Pi_a</math>, can be viewed as a dimensionless external pressure. Alternatively, for a given choice of <math>P_e</math> and <math>\bar{c_s}</math>, <math>\Pi_a^{1/2}</math> can represent a dimensionless mass; or, for a given choice of <math>M</math> and <math>P_e</math>, <math>\Pi_a^{-1/8}</math> can represent a dimensionless sound speed. Here we will view it as a dimensionless external pressure.
Unlike the isothermal case, for an arbitrary value of the adiabatic exponent, <math>\gamma_g</math>, it isn't possible to invert this equation to obtain an analytic expression for <math>\chi</math> as a function of <math>\Pi_a</math>. But we can straightforwardly solve for <math>\Pi_a</math> as a function of <math>\chi</math>. The solution is,
<math> \Pi_a = \frac{\chi^{(4- 3\gamma_g)} - 1}{\chi^4} \, . </math>
For physically relevant solutions, both <math>\chi</math> and <math>\Pi_a</math> must be nonnegative. Hence, as is illustrated by the curves in Figure 4, the physically allowable range of equilibrium radii is,
<math> 1 \le \chi \le \infty \, ~~~~~\mathrm{for}~ \gamma_g < 4/3 \, ; </math>
<math> 0 < \chi \le 1 \, ~~~~~~\mathrm{for}~ \gamma_g > 4/3 \, . </math>
|
Figure 4: Equilibrium Adiabatic P-V Diagram |
|
|
The curves trace out the function, <math> \Pi_a = (\chi^{4-3\gamma_g} - 1)/\chi^4 \, , </math> for six different values of <math>\gamma_g</math> (<math>2, ~5/3, ~7/5, ~6/5, ~1, ~2/3</math>, as labeled) and show the dimensionless external pressure, <math>\Pi_a</math>, that is required to construct a nonrotating, self-gravitating, uniform density, adiabatic sphere with an equilibrium radius <math>\chi</math>. The mathematical solution becomes unphysical wherever the pressure becomes negative. The solid red curve, drawn for the case <math>\gamma_g = 1</math>, is identical to the solid black (isothermal) curve displayed above in Figure 1. |
|
Each of the <math>\Pi_a(\chi)</math> curves drawn in Figure 4 exhibits an extremum. In each case this extremum occurs at a configuration radius, <math>\chi_\mathrm{extreme}</math>, given by,
<math> \frac{\partial\Pi_a}{\partial\chi} = 0 \, , </math>
that is, where,
<math> 4 - 3\gamma_g \chi^{4-3\gamma_g} = 0 ~~~~\Rightarrow ~~~~~ \chi_\mathrm{extreme} = \biggl[ \frac{4}{3\gamma_g} \biggr]^{1/(4-3\gamma_g)} \, . </math>
For each value of <math>\gamma_g</math>, the corresponding dimensionless pressure is,
<math> \Pi_a \biggr|_\mathrm{extreme} = \biggl(\frac{4}{3\gamma} - 1 \biggr) \biggl[ \frac{3\gamma_g}{4} \biggr]^{4/(4-3\gamma_g)} \, . </math>
Note, first, that for <math>\gamma_g > 4/3</math>, an equilibrium configuration with a positive radius can be constructed for all physically realistic — that is, for all positive — values of <math>\Pi_a</math>. Also, consistent with the behavior of the curves shown in Figure 4, the extremum arises in the regime of physically relevant — i.e., positive — pressures only for values of <math>\gamma_g < 4/3</math>; and in each case it represents a maximum limiting pressure.
Maximum Mass
<math>n=5</math> Polytropic
When <math>\gamma_a = 6/5</math> — which corresponds to an <math>n=5</math> polytropic configuration — we obtain,
<math> \Pi_\mathrm{max} = \Pi_a\biggr|_\mathrm{extreme}^{(\gamma_g = 6/5)} = \biggl( \frac{3^{18}}{2^{10}\cdot 5^{10}} \biggr) \, , </math>
which corresponds to a maximum mass for pressure-bounded <math>n=5</math> polytropic configurations of,
<math>M_\mathrm{max} = \Pi_\mathrm{max}^{1/2} \biggl(\frac{3\cdot 5^3}{2^2\pi} \biggr)^{1/2} \biggl( \frac{\bar{c_s}^8}{G^3 P_e} \biggr)^{1/2} = \biggl(\frac{3^{19}}{2^{12}\cdot 5^{7}\pi} \biggr)^{1/2} \biggl( \frac{\bar{c_s}^8}{G^3 P_e} \biggr)^{1/2} \, .</math>
This result can be compared to other determinations of the Bonnor-Ebert mass limit.

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |