User:Tohline/Appendix/Ramblings/ToroidalCoordinates

Toroidal Configurations and Related Coordinate Systems

Preamble

As I have studied the structure and analyzed the stability of (both self-gravitating and non-self-gravitating) toroidal configurations over the years, I have often wondered whether it might be useful to examine such systems mathematically using a toroidal — or at least a toroidal-like — coordinate system. Is it possible, for example, to build an equilibrium torus for which the density distribution is one-dimensional as viewed from a well-chosen toroidal-like system of coordinates?

I should begin by clarifying my terminology. In volume II (p. 666) of their treatise on Methods of Theoretical Physics, Morse & Feshbach (1953; hereafter MF53) define an orthogonal toroidal coordinate system in which the Laplacian is separable.¹ (See details, below.) It is only this system that I will refer to as the toroidal coordinate system; all other functions that trace out toroidal surfaces but that don't conform precisely to Morse & Feshbach's coordinate system will be referred to as toroidal-like.

I became particularly interested in this idea while working with Howard Cohl (when he was an LSU graduate student). Howie's dissertation research uncovered a Compact Cylindrical Greens Function technique for evaluating Newtonian potentials of rotationally flattened, axisymmetric potentials.^2,3 The technique involves a multipole expansion in terms of half-integer-degree Legendre functions of the <math>2^\mathrm{nd}</math> kind where, if I recall correctly, the argument of this special function (or its inverse) seemed to resemble the radial coordinate of Morse & Feshbach's orthogonal toroidal coordinate system.

Off-center Circle

In what follows, it will be useful to recall the algebraic expression that defines a circle whose center is not positioned at the origin of a Cartesian coordinate system. Specifically, consider a circle of radius "<math>r_c</math>" whose center is located a distance "<math>x_0</math>" along the plus-x axis. The equation for this circle is,

<math> (x-x_0)^2 + y^2 = r_c^2 . </math>

Toroidal Coordinates

Presentation by MF53

The orthogonal toroidal coordinate system <math>(\xi_1,\xi_2,\xi_3=\cos\varphi)</math> discussed by MF53 has the following properties:

<math> \frac{x}{a} </math>	<math> = </math>	<math> \biggl[ \frac{(\xi_1^2 - 1)^{1/2}}{\xi_1 - \xi_2} \biggr]\cos\varphi </math>
<math> \frac{y}{a} </math>	<math> = </math>	<math> \biggl[ \frac{(\xi_1^2 - 1)^{1/2}}{\xi_1 - \xi_2} \biggr]\sin\varphi </math>
<math> \frac{z}{a} </math>	<math> = </math>	<math> \frac{(1-\xi_2^2)^{1/2}}{\xi_1 - \xi_2} </math>
<math> \frac{\varpi}{a} \equiv \biggl[ \biggl(\frac{x}{a}\biggr)^2 + \biggl(\frac{y}{a}\biggr)^2 \biggr]^{1/2} </math>	<math> = </math>	<math> \frac{(\xi_1^2 - 1)^{1/2}}{\xi_1 - \xi_2} </math>
<math> \frac{r}{a} \equiv \biggl[ \biggl(\frac{x}{a}\biggr)^2 + \biggl(\frac{y}{a}\biggr)^2 + \biggl(\frac{z}{a}\biggr)^2\biggr]^{1/2} </math>	<math> = </math>	<math> \biggl[ \frac{\xi_1 + \xi_2}{\xi_1 - \xi_2} \biggr]^{1/2} </math>

According to MF53, the associated scale factors of this orthogonal coordinate system are:

<math> \frac{h_1}{a} </math>	<math> = </math>	<math> \frac{1}{(\xi_1 - \xi_2)(\xi_1^2 - 1)^{1/2}} </math>
<math> \frac{h_2}{a} </math>	<math> = </math>	<math> \frac{1}{(\xi_1 - \xi_2)(1-\xi_2^2)^{1/2}} </math>
<math> \frac{h_3}{a} </math>	<math> = </math>	<math> \biggl[ \frac{(\xi_1^2 - 1)^{1/2}}{\xi_1 - \xi_2} \biggr]\frac{1}{\sin\varphi} </math>

Tohline's Ramblings

My inversion of these coordinate definitions has lead to the following expressions:

<math> \xi_1 </math>	<math> = </math>	<math> \frac{r(r^2 + 1)} {[\chi^2(r^2-1)^2 + \zeta^2(r^2+1)^2]^{1/2}} </math>
<math> \xi_2 </math>	<math> = </math>	<math> \frac{r(r^2 - 1)}{[\chi^2(r^2-1)^2 + \zeta^2(r^2+1)^2]^{1/2}} </math>

where,

<math> \chi \equiv \frac{\varpi}{a} ~~~;~~~\zeta\equiv\frac{z}{a} ~~~\mathrm{and} ~~~ r=(\chi^2 + \zeta^2)^{1/2} . </math>

Apparently the allowed ranges of the two meridional-plane coordinates are:

<math> +1 \leq \xi_1 \leq \infty ~~~\mathrm{and} ~~~ -1 \leq \xi_2 \leq +1 . </math>

Example Toroidal Surfaces

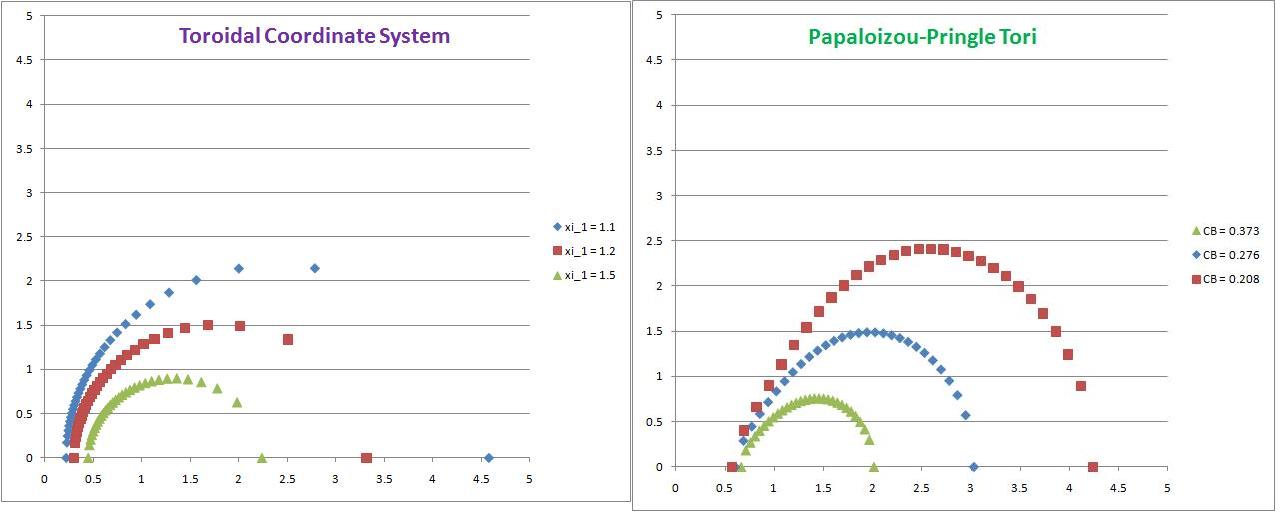
In the accompanying figure labeled "Toroidal Coordinate System," we've outlined three different <math>\xi_1 = \mathrm{constant}</math> meridional contours for the MF53 toroidal coordinate system. The illustrated values are,

<math> \xi_1 </math>	=	1.1		(blue);
<math> \xi_1 </math>	=	1.2		(red);
<math> \xi_1 </math>	=	1.5		(gold).

The inner and outer edges of the toroidal surface in the equatorial plane should be determined by setting <math>\xi_2 = -1</math> (inner) and <math>\xi_2 = +1</math> (outer). Hence,

<math> \chi_\mathrm{inner} </math>	=	<math> \frac{(\xi_1^2 - 1)^{1/2}}{\xi_1 +1} = \biggl[\frac{(\xi_1 - 1)}{(\xi_1 + 1)} \biggr]^{1/2} </math>
<math> \chi_\mathrm{outer} </math>	=	<math> \frac{(\xi_1^2 - 1)^{1/2}}{\xi_1 - 1} = \biggl[\frac{(\xi_1 + 1)}{(\xi_1 - 1)} \biggr]^{1/2} </math>

The equatorial-plane location of the "center" of each torus is,

<math> \chi_0 = \frac{1}{2} (\chi_\mathrm{outer} + \chi_\mathrm{inner}) = \frac{\xi_1}{(\xi_1^2 - 1)^{1/2}} , </math>

and the so-called distortion parameter,

<math> \delta \equiv \frac{\chi_\mathrm{outer}-\chi_\mathrm{inner}}{\chi_0}= \frac{2}{\xi_1} . </math>

Properties of <math>\xi_1 = \mathrm{constant}</math> Toroidal Surfaces
Curve in Figure	<math>\xi_1</math>	<math>\chi_\mathrm{inner}</math>	<math>\chi_\mathrm{outer}</math>	<math>\chi_0</math>	<math>\delta</math>
Blue	1.1	0.218	4.583	2.400	1.818
Red	1.2	0.302	3.317	1.809	1.667
Gold	1.5	0.447	2.236	1.342	1.333

What function <math>\zeta(\varpi)</math> coincides with these <math>\xi_1 = \mathrm{constant}</math> surfaces? (To be answered!)

Off-center Circle

The curves drawn in the above figure labeled "Toroidal Coordinate System" resemble circles whose centers are positioned a distance <math>\chi_0</math> away from the origin. Let's examine whether this is the case by drawing on the familiar expression for such a configuration, as presented above. If this is the case, then the circle will have a radius,

<math> r_c </math>	<math> = </math>	<math> \chi_\mathrm{outer} - \chi_0 </math>
	<math> = </math>	<math> \biggl[\frac{(\xi_1 + 1)}{(\xi_1 - 1)} \biggr]^{1/2} - \frac{\xi_1}{(\xi_1^2 - 1)^{1/2}} </math>
	<math> = </math>	<math> \frac{1}{(\xi_1^2 - 1)^{1/2}} </math>

and the algebraic expression describing the circle will take the form,

<math> (\chi - \chi_0)^2 + \zeta^2 = r_c^2 = (\xi_1^2 - 1)^{-1} . </math>

Let's evaluate the left-hand-side of this expression to see if it indeed reduces to <math>(\xi_1^2 - 1)^{-1}</math>.

LHS	=	<math> \biggl\{ \biggl[ \frac{(\xi_1^2 - 1)^{1/2}}{\xi_1 - \xi_2} \biggr] - \frac{\xi_1}{(\xi_1^2 - 1)^{1/2}} \biggr\}^2 + \biggl[ \frac{(1-\xi_2^2)^{1/2}}{\xi_1 - \xi_2} \biggr]^2 </math>
	=	<math> \frac{1}{(\xi_1 - \xi_2)^2 (\xi_1^2 - 1)} \biggl\{ (\xi_1^2 - 1) - \xi_1 (\xi_1-\xi_2) \biggr\}^2 + \frac{(1-\xi_2^2)}{(\xi_1 - \xi_2)^2} </math>
	=	<math> \frac{(\xi_1 \xi_2 - 1)^2}{(\xi_1 - \xi_2)^2 (\xi_1^2 - 1)} + \frac{(1-\xi_2^2)}{(\xi_1 - \xi_2)^2} </math>
	=	<math> \frac{1}{(\xi_1 - \xi_2)^2 (\xi_1^2 - 1)} \biggl[(\xi_1 \xi_2 - 1)^2 + (\xi_1^2 - 1)(1-\xi_2^2) \biggr] </math>
	=	<math> \frac{1}{(\xi_1 - \xi_2)^2 (\xi_1^2 - 1)} \biggl[ \xi_1^2 \xi_2^2 - 2\xi_1 \xi_2 + 1 ) + (\xi_1^2 - 1 -\xi_1^2 \xi_2^2 + \xi_2^2) \biggr] </math>
	=	<math> \frac{\xi_1^2 - 2\xi_1 \xi_2 + \xi_2^2}{(\xi_1 - \xi_2)^2 (\xi_1^2 - 1)} </math>
	=	<math> \frac{1}{(\xi_1^2 - 1)} </math>

Yes! So this means that the "<math>\xi_1 = \mathrm{constant}</math>" toroidal contours can be described by the off-center circle expression,

<math> (\chi - \chi_0)^2 + \zeta^2 = (\chi_\mathrm{outer} - \chi_0)^2 </math>

or,

<math> \biggl[ \chi - \frac{\xi_1}{(\xi_1^2 - 1)^{1/2}} \biggr]^2 + \zeta^2 = \frac{1}{(\xi_1^2 - 1)} </math>

It also means that, while <math>\xi_1</math> is the official radial coordinate of MF53's toroidal coordinate system, the actual radius of the relevant cross-sectional circle is,

<math> r_c </math>

<math> = </math>

<math> \frac{1}{(\xi_1^2 - 1)^{1/2}} . </math>

Papaloizou-Pringle Tori

Summary of Structure

As derived elsewhere, the accretion tori constructed by Papaloizou & Pringle (1984; hereafter PP84) have the following surface properties. For a given choice of the dimensionless Bernoulli constant, <math>C_\mathrm{B}^'</math>,

<math> \chi_\mathrm{inner} = \frac{1}{1 + \sqrt{1-2C_\mathrm{B}^'}} ; </math>

<math> \chi_\mathrm{outer} = \frac{1}{1 - \sqrt{1-2C_\mathrm{B}^'}} ; </math>

<math> \chi_0 = \frac{1}{2} (\chi_\mathrm{outer} + \chi_\mathrm{inner}) = \frac{1}{2C_\mathrm{B}^'} ; </math>

<math> \delta \equiv \frac{\chi_\mathrm{outer} - \chi_\mathrm{inner}}{\chi_0} = 2\sqrt{1-2C_\mathrm{B}^'} . </math>

So if I want to construct PP84 tori that are approximately the same size/shape as the MF53 tori illustrated above, I should choose values of the dimensionless Bernoulli constant as follows:

<math> {\chi_0}\biggr|_\mathrm{PP84} = {\chi_0}\biggr|_\mathrm{MF53} </math>

<math> \Rightarrow~~~~ \frac{1}{2C_\mathrm{B}^'}=\frac{\xi_1}{(\xi_1^2-1)^{1/2}} </math>

<math> \Rightarrow~~~~ C_\mathrm{B}^'= \frac{(\xi_1^2-1)^{1/2}}{2\xi_1} </math>

In the accompanying figure labeled "Papaloizou-Pringle Tori," we've drawn three different <math>C_\mathrm{B}^' = \mathrm{constant}</math> meridional contours for the PP84 tori where the values of the dimensionless Bernoulli constants have been chosen to produce values of <math>\chi_0</math> that are identical to the values displayed by the three MF53 tori shown above. The following table details properties of these three PP84 tori that have been constructed in an effort to facilitate comparison with the table shown above for MF53 tori.

Properties of <math>C_\mathrm{B}^' = \mathrm{constant}</math> PP84 Toroidal Surfaces
Curve in Figure	<math>C_\mathrm{B}^'</math>	<math>\chi_\mathrm{inner}</math>	<math>\chi_\mathrm{outer}</math>	<math>\chi_0</math>	<math>\delta</math>
Red	0.208	0.567	4.234	2.400	1.528
Blue	0.276	0.599	3.019	1.809	1.338
Gold	0.373	0.665	2.019	1.342	1.009

CCGF Expansion

The Compact Cylindrical Green's Function expansion that Cohl & Tohline (1999; hereafter CT99) use to express the Newtonian potential, includes a special function whose dimensionless argument is,

<math> \chi_\mathrm{CT99} \equiv \frac{R^2 + {R'}^2 + (z - z')^2}{2R R'} . </math>

References

1. Morse, P.M. & Feshmach, H. 1953, Methods of Theoretical Physics — Volumes I and II
2. Cohl, H.S. & Tohline, J.E. 1999, ApJ, 527, 86-101
3. Cohl, H.S., Rau, A.R.P., Tohline, J.E., Browne, D.A., Cazes, J.E. & Barnes, E.I. 2001, Phys. Rev. A, 64, 052509

 

© 2014 - 2021 by Joel E. Tohline |   H_Book Home   |   YouTube   | Appendices: | Equations | Variables | References | Ramblings | Images | myphys.lsu | ADS | Recommended citation:   Tohline, Joel E. (2021), The Structure, Stability, & Dynamics of Self-Gravitating Fluids, a (MediaWiki-based) Vistrails.org publication, https://www.vistrails.org/index.php/User:Tohline/citation