Revision as of 00:35, 30 May 2018

Relationships Between Toroidal Functions

Wong Toroidal Coordinates

This chapter has been put together in an effort to lay the groundwork for an evaluation of Wong's (1973) derived expression for the gravitational potential both inside and outside of a uniform-density, axisymmetric torus. After multiplying his expression by the negative of <math>~G</math>, then replacing his total charge, <math>~q</math>, with the total mass, <math>~M</math>, Wong's interior (i.e., <math>~\eta^' > \eta_0</math>) solution is,

<math>~U(\eta^',\theta^')\biggr\|_{\mathrm{for}~\eta^' \ge \eta_0}</math>	<math>~=</math>	<math>~- \frac{2^{5 / 2} a^2 G}{3} \biggl[ \frac{1}{2\pi^2 a^2}\biggl(\frac{M}{a}\biggr) \frac{\sinh^3 \eta_0}{\cosh\eta_0} \biggr] \biggl\{ - \frac{3\pi^2}{2^{5/ 2}} \biggl[ \frac{\sinh^2\eta^'}{(\cosh \eta^' - \cos \theta^')^2} \biggr] +~ (\cosh \eta^' - \cos \theta^')^{1 / 2} </math>
		<math>~ \times \sum\limits_{n=0}^\infty \epsilon_n \cos(n\theta^') Q_{n-1 / 2}(\cosh\eta^') B_n(\cosh\eta_0) \biggr\} \, , </math>
Wong (1973), Eq. (2.65)

where,

<math>~B_n(\cosh\eta_0)</math>	<math>~\equiv</math>	<math>~ (n+\tfrac{1}{2})P_{n+1/2} (\cosh\eta_0)Q^2_{n-1/2} (\cosh\eta_0) - (n-\tfrac{3}{2})P_{n-1/2} (\cosh\eta_0)Q^2_{n+1/2} (\cosh\eta_0) \, . </math>
Wong (1973), Eq. (2.62)

Summary

Suppose you want to evaluate the potential of a uniform-density torus whose major radius is, <math>~R</math>, and minor cross-sectional radius is, <math>~d</math>. Evaluation of the potential can be relatively easily expressed in terms of a toroidal coordinate system, <math>~(\eta,\theta)</math>, whose "origin" is at a distance, <math>~a</math>, from the symmetry axis, where,

<math>~a^2 \equiv R^2 - d^2 ~~~\Rightarrow ~~~ \frac{a^2}{d^2} = \frac{R^2}{d^2} - 1\, .</math>

When expressed in terms of cylindrical coordinates, the meridional-plane location at which the potential is to be evaluated is, <math>~(\varpi, z)</math>, and in toroidal coordinates the location is determined as follows:

<math>~\rho_1^2</math>	<math>~\equiv</math>	<math>~(\varpi - a)^2 + z^2</math>
<math>~\rho_2^2</math>	<math>~\equiv</math>	<math>~(\varpi + a)^2 + z^2</math>
<math>~\eta</math>	<math>~=</math>	<math>~\ln\biggl( \frac{\rho_2}{\rho_1} \biggr)</math>
<math>~\cos\theta</math>	<math>~=</math>	<math>~\frac{ (\rho_1^2 + \rho_2^2) -4a^2 }{ 2\rho_1 \rho_2 }</math>

The surface of the uniform-density torus is defined by the toroidal "radial" coordinate, <math>~\eta_0</math>, such that,

<math>~\cosh\eta_0</math>	<math>~=</math>	<math>~\frac{R}{d} \, ,</math>
<math>~\sinh\eta_0</math>	<math>~=</math>	<math>~[ \cosh^2\eta_0 - 1 ]^{1 / 2} = \frac{a}{d} \, ,</math>
<math>~\tanh\eta_0 = \frac{\sinh\eta_0}{\cosh\eta_0}</math>	<math>~=</math>	<math>~\frac{a}{R} \, .</math>

The volume of a torus is,

When this is rewritten in terms of our toroidal coordinate system, we have,

<math>~V</math>	<math>~=</math>	<math>~2\pi^2 a^3 \biggl( \frac{R}{a} \biggr) \biggl( \frac{d}{a} \biggr)^2</math>
	<math>~=</math>	<math>~2\pi^2 a^3 \biggl( \frac{\cosh\eta_0}{\sinh^3\eta_0} \biggr) \, .</math>

Expressions for the relevant toroidal functions are as follows:

<math>~P_{-\frac{1}{2}}(\cosh\eta)</math>	<math>~=</math>	<math>~ \biggl[ \frac{\pi}{2} \cdot \cosh\frac{\eta}{2} \biggr]^{-1} K\biggl( \tanh\frac{\eta}{2} \biggr) \, , </math>
<math>~P_{+\frac{1}{2}}(\cosh\eta)</math>	<math>~=</math>	<math>~ \frac{2}{\pi}~e^{\eta/2} ~E(\sqrt{1-e^{-2\eta} }) \, , </math>
<math>~Q_{-\frac{1}{2}}(\cosh\eta)</math>	<math>~=</math>	<math>~ 2e^{-\eta/2}K(e^{-\eta}) \, , </math>
<math>~Q_{+\frac{1}{2}}(\chi)</math>	<math>~=</math>	<math>~ \chi \mu K(\mu) - (1+\chi)\mu E(\mu) \, </math>

where, <math>~\chi \equiv \cosh\eta</math>; <math>~K</math> and <math>~E</math> are, respectively, complete elliptic integrals of the first and second kind; and,

<math>~\equiv</math>

Then, for <math>~m \ge 2</math> , the recurrence relation (for <math>~P_{m-\frac{1}{2} }</math> as well as for <math>~Q_{m-\frac{1}{2} }</math>) is,

<math>~ 4\biggl[ \frac{m-1}{2m-1}\biggr] \chi Q_{m-\frac{3}{2}}(\chi) - \biggl[ \frac{2m-3}{2m-1}\biggr] Q_{m-\frac{5}{2}}(\chi) \, . </math>

Given that the portion of the leading term in Wong's expression that sits inside the square brackets is equivalent to the density, <math>~\rho_0 = M/V</math>, of the torus material — that is, given that,

<math>~\frac{1}{2\pi^2 a^2}\biggl(\frac{M}{a}\biggr) \frac{\sinh^3 \eta_0}{\cosh\eta_0} </math>

<math>~\frac{\rho_0 V}{2\pi^2 a^3} \biggl[ \frac{\sinh^3 \eta_0}{\cosh\eta_0} \biggr] </math>

— a reasonable dimensionless version of Wong's expression could be obtained by dividing through by the quantity, <math>~(G\rho_0 a^2) </math>. We prefer, instead, to normalize Wong's expression to the quantity, <math>~GM/R</math> , in which case the dimensionless version of the expression becomes,

<math>~\frac{U(\eta^',\theta^')}{GM/R} \biggr\|_{\mathrm{for}~\eta^' \ge \eta_0}</math>	<math>~=</math>	<math>~- \frac{2^{5 / 2} }{3} \biggl[ \frac{1}{2\pi^2}\biggl(\frac{R}{a}\biggr) \frac{\sinh^3 \eta_0}{\cosh\eta_0} \biggr] \biggl\{ - \frac{3\pi^2}{2^{5/ 2}} \biggl[ \frac{\sinh^2\eta^'}{(\cosh \eta^' - \cos \theta^')^2} \biggr] +~ (\cosh \eta^' - \cos \theta^')^{1 / 2} </math>
		<math>~ \times \sum\limits_{n=0}^\infty \epsilon_n \cos(n\theta^') Q_{n-1 / 2}(\cosh\eta^') B_n(\cosh\eta_0) \biggr\} </math>
	<math>~=</math>	<math>~- \frac{2^{3 / 2} \sinh^2\eta_0 }{3\pi^2}\biggl\{ - \frac{3\pi^2}{2^{5/ 2}} \biggl[ \frac{\sinh^2\eta^'}{(\cosh \eta^' - \cos \theta^')^2} \biggr] +~ (\cosh \eta^' - \cos \theta^')^{1 / 2} </math>
		<math>~ \times \sum\limits_{n=0}^\infty \epsilon_n \cos(n\theta^') Q_{n-1 / 2}(\cosh\eta^') B_n(\cosh\eta_0) \biggr\} </math>
	<math>~=</math>	<math>~\sinh^2\eta_0 \biggl\{ \frac{\sinh^2\eta^'}{2 (\cosh \eta^' - \cos \theta^')^2} ~- ~\frac{2^{3 / 2} }{3\pi^2} (\cosh \eta^' - \cos \theta^')^{1 / 2} \sum\limits_{n=0}^\infty \epsilon_n \cos(n\theta^') Q_{n-1 / 2}(\cosh\eta^') B_n(\cosh\eta_0) \biggr\} \, . </math>

Prior to the Integration

The Green's function written in toroidal coordinates is,

<math>~ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=-\infty}^{\infty} e^{im(\phi - \phi^')}Q_{m- 1 / 2}(\chi) \, , </math>

where,

<math>~\chi \equiv \frac{\varpi^2 + (\varpi^')^2 + (z - z^')^2}{2\varpi \varpi^'} \, ,</math>

and, <math>~Q_{m - 1 / 2}</math> is the zero-order, half-(odd)integer degree, Llegendre function of the second kind — also referred to as a toroidal function of zeroth order. Hence, a valid expression for the gravitational potential is,

<math>~ \Phi_B(\varpi,\phi,z)</math>	<math>~=</math>	<math>~ -G \int \frac{1}{\|\vec{x}^{~'} - \vec{x}\|} ~\rho(\vec{x}^{~'}) d^3x^' </math>
	<math>~=</math>	<math>~ -G \int \biggl\{ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=-\infty}^{\infty} e^{im(\phi - \phi^')}Q_{m- 1 / 2}(\chi) \biggr\}~ \rho(\varpi^',\phi^',z^') \varpi^' d\varpi^' d\phi^' dz^' </math>
	<math>~=</math>	<math>~ -\frac{G}{\pi \sqrt{\varpi}} \int \rho(\varpi^',\phi^',z^') \sqrt{\varpi^'} d\varpi^' d\phi^' dz^' \sum_{m=0}^{\infty} \epsilon_m \cos[m(\phi - \phi^')] Q_{m- 1 / 2}(\chi) \, , </math>

where, <math>~\epsilon_m</math> is the Neumann factor, that is, <math>~\epsilon_0=1</math> and <math>~\epsilon_m = 2</math> for <math>~m\ge 1</math>.

Wong (1973) states that in toroidal coordinates the Green's function is,

<math>~\frac{1}{\|~\vec{r} - {\vec{r}}^{~'} ~\|} </math>	<math>~=</math>	<math>~ \frac{1}{\pi a} \biggl[ (\cosh\eta - \cos\theta)(\cosh \eta^' - \cos\theta^') \biggr]^{1 /2 } \sum\limits_{m,n} (-1)^m \epsilon_m \epsilon_n ~\frac{\Gamma(n-m+\tfrac{1}{2})}{\Gamma(n + m + \tfrac{1}{2})} </math>
		<math>~ \times \cos[m(\psi - \psi^')][\cos[n(\theta - \theta^')] ~\begin{cases}P^m_{n-1 / 2}(\cosh\eta) ~Q^m_{n-1 / 2}(\cosh\eta^') ~~~\eta^' > \eta \\P^m_{n-1 / 2}(\cosh\eta^') ~Q^m_{n-1 / 2}(\cosh\eta)~~~\eta^' < \eta \end{cases}\, , </math>
Wong (1973), Eq. (2.53)

where, <math>~P^m_{n-1 / 2}, Q^m_{n-1 / 2}</math> are "Legendre functions of the first and second kind with order <math>~n - \tfrac{1}{2}</math> and degree <math>~m</math> (toroidal harmonics)," and <math>~\epsilon_m</math> is the Neumann factor, that is, <math>~\epsilon_0 = 1</math> and <math>~\epsilon_m = 2</math> for all <math>~m \ge 1</math>,

<math>~\eta</math>	<math>~=</math>	<math>~\ln\biggl(\frac{r_1}{r_2} \biggr) \, ,</math>
<math>~\cos\theta</math>	<math>~=</math>	<math>~\frac{(r_1^2 + r_2^2 - 4a^2)}{2r_1 r_2} \, ,</math>
<math>~\tan\psi</math>	<math>~=</math>	<math>~\frac{y}{x} \, ,</math>

and,

<math>~r_1^2 </math>	<math>~\equiv</math>	<math>~[(x^2 + y^2)^{1 / 2} + a]^2 + z^2 \, ,</math>
<math>~r_2^2 </math>	<math>~\equiv</math>	<math>~[(x^2 + y^2)^{1 / 2} - a]^2 + z^2 \, ,</math>

and <math>~\theta</math> has the same sign as <math>~z</math>.

Hence, the potential, <math>~U({\vec{r}}~')</math>, at a point <math>~{\vec{r}}~'</math> due to an arbitrary mass distribution, <math>~\rho({\vec{r}})</math>, is,

<math>~U({\vec{r}}~')</math>	<math>~=</math>	<math>~-G \iiint \frac{\rho(\vec{r}) d^3r}{\|~\vec{r} - {\vec{r}}^{~'} ~\|} \, .</math>
	<math>~=</math>	<math>~-G \rho_0 a^3 \iiint \frac{1}{\|~\vec{r} - {\vec{r}}^{~'} ~\|} \biggl[ \frac{\Theta(\upsilon) \sinh\eta}{(\cosh\eta - \cos\theta)^3} \biggr] d\eta~ d\theta~ d\psi~ \, .</math>
	<math>~=</math>	<math>~-G \rho_0 a^3\iiint \biggl[ \frac{\Theta(\upsilon) \sinh\eta}{(\cosh\eta - \cos\theta)^3} \biggr]~d\eta~ d\theta~ d\psi~ </math>
		<math>~\times \frac{1}{\pi a} \biggl[ (\cosh\eta - \cos\theta)(\cosh \eta^' - \cos\theta^') \biggr]^{1 /2 } \sum\limits_{m,n} (-1)^m \epsilon_m \epsilon_n ~\frac{\Gamma(n-m+\tfrac{1}{2})}{\Gamma(n + m + \tfrac{1}{2})} </math>
		<math>~ \times \cos[m(\psi - \psi^')][\cos[n(\theta - \theta^')] ~\begin{cases}P^m_{n-1 / 2}(\cosh\eta) ~Q^m_{n-1 / 2}(\cosh\eta^') ~~~\eta^' > \eta \\P^m_{n-1 / 2}(\cosh\eta^') ~Q^m_{n-1 / 2}(\cosh\eta)~~~\eta^' < \eta \end{cases} \biggr\} </math>

This should be compared with,

<math>~ \Phi_B(\varpi,\phi,z)</math>	<math>~=</math>	<math>~ -G \iiint \frac{1}{\|\vec{x}^{~'} - \vec{x}\|} ~\rho(\vec{x}^{~'}) d^3x^' </math>
	<math>~=</math>	<math>~ -G \iiint \biggl\{ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=-\infty}^{\infty} e^{im(\phi - \phi^')}Q_{m- 1 / 2}(\chi) \biggr\}~ \rho(\varpi^',\phi^',z^') \varpi^' d\varpi^' d\phi^' dz^' </math>
	<math>~=</math>	<math>~ -\frac{G}{\pi \sqrt{\varpi}} \iiint\rho(\varpi^',\phi^',z^') \sqrt{\varpi^'} d\varpi^' d\phi^' dz^' \sum_{m=0}^{\infty} \epsilon_m \cos[m(\phi - \phi^')] Q_{m- 1 / 2}(\chi) \, , </math>

The above expression for the potential of a uniform-density torus has been obtained by Wong (1973) from the (double) integral expression,

<math>~U(\eta^',\theta^')</math>	<math>~=</math>	<math>~ 2\rho_0 a^2 (\cosh \eta^' - \cos \theta^')^{1 / 2} \sum\limits_n \epsilon_n \int_{\eta_0}^\infty d\eta \int_{-\pi}^{\pi} \biggl[\frac{\cos[n(\theta - \theta^')]}{(\cosh\eta - \cos\theta)^{5 / 2}} \biggr]d\theta </math>
		<math>~ \times \sinh\eta ~\begin{cases}P_{n-1 / 2}(\cosh\eta) ~Q_{n-1 / 2}(\cosh\eta^') ~~~\eta^' > \eta \\P_{n-1 / 2}(\cosh\eta^') ~Q_{n-1 / 2}(\cosh\eta)~~~\eta^' < \eta \end{cases}\, </math>
Wong (1973), Eq. (2.55)

which is valid for any azimuthal angle, <math>~\psi^'</math>. Notice that the step function, <math>~\Theta(\upsilon)</math>, no longer explicitly appears in this expression for the Coulomb (or gravitational) potential; it has been used to establish the specific limits on the "radial" coordinate integration. Next, he completes the integration over the angle, <math>~\theta</math>, to obtain,

CT99 Coordinates

Eureka! Via his dogged efforts and an extraordinarily in-depth investigation of this problem, in 1999 Howard S. Cohl discovered that, in cylindrical coordinates, the relevant Green's function can be written in a much more compact and much more practical form. Specifically, he realized that,

<math>~ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=-\infty}^{\infty} e^{im(\phi - \phi^')}Q_{m- 1 / 2}(\chi) \, , </math>

where,

<math>~\chi \equiv \frac{\varpi^2 + (\varpi^')^2 + (z - z^')^2}{2\varpi \varpi^'} \, ,</math>

H. S. Cohl & J. E. Tohline (1999), p. 88, Eqs. (15) & (16)
See also: Selvaggi, Salon & Chari (2007) §II, eq. (5)
and the DLMF's definition of Toroidal Functions, <math>~Q_{m - 1 / 2}^{0}</math>

and, <math>~Q_{m - 1 / 2}</math> is the zero-order, half-(odd)integer degree, Llegendre function of the second kind — also referred to as a toroidal function of zeroth order; see additional details, below. Hence, anywhere along the boundary of our cylindrical-coordinate mesh, a valid expression for the gravitational potential is,

<math>~ \Phi_B(\varpi,\phi,z)</math>	<math>~=</math>	<math>~ -G \int \frac{1}{\|\vec{x}^{~'} - \vec{x}\|} ~\rho(\vec{x}^{~'}) d^3x^' </math>
	<math>~=</math>	<math>~ -G \int \biggl\{ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=-\infty}^{\infty} e^{im(\phi - \phi^')}Q_{m- 1 / 2}(\chi) \biggr\}~ \rho(\varpi^',\phi^',z^') \varpi^' d\varpi^' d\phi^' dz^' </math>
	<math>~=</math>	<math>~ -\frac{G}{\pi \sqrt{\varpi}} \int \rho(\varpi^',\phi^',z^') \sqrt{\varpi^'} d\varpi^' d\phi^' dz^' \sum_{m=0}^{\infty} \epsilon_m \cos[m(\phi - \phi^')] Q_{m- 1 / 2}(\chi) \, , </math>
H. S. Cohl & J. E. Tohline (1999), p. 88, Eq. (18)

where, <math>~\epsilon_m</math> is the Neumann factor, that is, <math>~\epsilon_0=1</math> and <math>~\epsilon_m = 2</math> for <math>~m\ge 1</math>. Following this discovery, most of my research group's 3D numerical modeling of self-gravitating fluids has been conducted using Toroidal functions instead of Spherical Harmonics to evaluate the boundary potential on our cylindrical-coordinate meshes.

In our accompanying statement of this problem, we have written,

<math>~- \frac{2G}{R_*^{1 / 2}} \int\int \varpi^{1 / 2} \mu K(\mu) \rho(\varpi, Z) d\varpi dZ \, ,</math>

where, <math>~K(\mu)</math> is the complete elliptic integral of the first kind, and,

<math>~ \biggl[\frac{4R_*\varpi}{(R_* + \varpi)^2 + (Z_* - Z)^2} \biggr] \, . </math>

Observer Toroidal Coordinates

Our Mucking Around

Begins on p. 332 of M. Abramowitz & I. A. Stegun (1995)

Recurrence Relations

According to M. Abramowitz & I. A. Stegun (1995), both <math>~P_\nu^\mu</math> and <math>~Q_\nu^\mu</math> satisfy the same recurrence relations.

<math>~P_\nu^{\mu+1}(z)</math>	<math>~=</math>	<math>~ (z^2 - 1)^{- 1 / 2} \biggl[ (\nu - \mu)z P_\nu^\mu(z) - (\nu + \mu)P_{\nu - 1}^\mu(z) \biggr] \, ; </math>
<math>~(z^2-1) \frac{dP_\nu^{\mu}(z)}{dz}</math>	<math>~=</math>	<math>~ (\nu + \mu)(\nu - \mu +1_(z^2-1)^{1 / 2} P_\nu^{\mu - 1}(z) - \mu zP_\nu^\mu(z) \, ; </math>
<math>~(\nu - \mu + 1)P_{\nu + 1}^{\mu}(z)</math>	<math>~=</math>	<math>~ (2\nu+1)zP_\nu^\mu (z) -(\nu + \mu)P_{\nu-1}^\mu(z) \, ; </math>
<math>~(z^2-1) \frac{dP_\nu^{\mu}(z)}{dz}</math>	<math>~=</math>	<math>~ \nu z P_\nu^{\mu }(z) - (\nu + \mu)P_{\nu-1}^\mu(z) \, . </math>

According to equation (14) of A. B. Basset (1893, American Journal of Mathematics, vol. 15, No. 4, pp. 287 - 302),

<math>~ (n-m+\tfrac{1}{2})P_{n+1}^m(\nu) -2n\nu P_n^m(\nu) + \frac{(n-\tfrac{1}{2})^2}{(n-m-\tfrac{1}{2})}P_{n-1}^m(\nu) </math>	<math>~=</math>	<math>~ \biggl[ \frac{m^2}{n-m-\tfrac{1}{2}} \biggr]P_{n-1}^m(\nu) </math>
<math>~\Rightarrow~~~ (n-m+\tfrac{1}{2})P_{n+1}^m(\nu) </math>	<math>~=</math>	<math>~ 2n\nu P_n^m(\nu) + \biggl[ \frac{m^2}{n-m-\tfrac{1}{2}} \biggr]P_{n-1}^m(\nu) - \frac{(n-\tfrac{1}{2})^2}{(n-m-\tfrac{1}{2})}P_{n-1}^m(\nu) </math>
	<math>~=</math>	<math>~ 2n\nu P_n^m(\nu) + \biggl[ \frac{m^2 - (n-\tfrac{1}{2})^2}{n-m-\tfrac{1}{2}} \biggr]P_{n-1}^m(\nu) </math>

After replacing, <math>~n</math>, with <math>~(n + \tfrac{1}{2})</math>,

<math>~\Rightarrow~~~ (n-m+1)P_{n+3 / 2}^m(\nu) </math>	<math>~=</math>	<math>~ (2n+1)\nu P_{n+1 / 2}^m(\nu) + \biggl[ \frac{m^2 - n^2}{n-m} \biggr]P_{n-1 / 2}^m(\nu) </math>
	<math>~=</math>	<math>~ (2n+1)\nu P_{n+1 / 2}^m(\nu) - (n+m)P_{n-1 / 2}^m(\nu) \, . </math>

The coefficients of this last expression precisely match the coefficients in the above expression provided by M. Abramowitz & I. A. Stegun (1995), but the subscript notation is off by <math>~\tfrac{1}{2}</math>. This inconsistency most likely should be blamed on the notation adopted by Basset (1893). At the top of his p. 289 — which is a couple of pages before his equation (14) — Basset says: A toroidal function is an associated function of degree <math>~n - \tfrac{1}{2}</math> and order <math>~m</math>; and the notation which ought in strictness to be adopted for the two kinds of toroidal functions is <math>~P_{n-1 / 2}^m</math> and <math>~Q_{n-1 / 2}^m</math>; but as these functions rarely if ever occur in an investigation which also involves associated functions of integral degree <math>~n</math>, it will be generally sufficient to employ the suffix <math>~n</math> instead of <math>~n - \tfrac{1}{2}</math>. Thus, we probably should have shifted the subscript notation in his equation (14) by "-½" before incorporating our additional replacement everywhere of <math>~n</math> by <math>~(n + \tfrac{1}{2})</math>.

If we set <math>~\mu = 0</math> in the M. Abramowitz & I. A. Stegun (1995) recurrence relation, then replace <math>~\nu</math> everywhere with <math>~\nu - \tfrac{1}{2}</math>, we obtain,

<math>~(\nu + 1)P_{\nu + 1}(z)</math>	<math>~=</math>	<math>~ (2\nu+1)zP_\nu (z) -(\nu )P_{\nu-1}(z) </math>
<math>~\nu \rightarrow \nu - \tfrac{1}{2} ~~~\Rightarrow ~~~ (\nu + \tfrac{1}{2})P_{\nu + 1 / 2}(z)</math>	<math>~=</math>	<math>~ (2\nu)zP_{\nu - 1 / 2} (z) -(\nu - \tfrac{1}{2})P_{\nu-3 / 2}(z) </math>
Mult. thru by 2 <math>~~~\Rightarrow ~~~ (2\nu + 1)P_{\nu + 1 / 2}(z)</math>	<math>~=</math>	<math>~ (4\nu)zP_{\nu - 1 / 2} (z) -(2\nu - 1)P_{\nu-3 / 2}(z) </math>

Independently, from equation (56) of Basset's (1888, Cambridge: Beighton, Bell and Co.) A Treatise on Hydrodynamics, we have,

This matches the Abramowitz & Stegun expression if, as before, we employ the mapping, <math>~n \rightarrow n-\tfrac{1}{2}</math>, in the subscripts only; also, note that, due to what must have been a typesetting error, the coefficient, <math>~C</math>, in Basset's expression must be replaced by the independent variable, <math>~\nu</math>.

From equations (57) - (60) of Basset's (1888) Hydrodynamics, we also obtain,

<math>~P_{-1 / 2} </math>	<math>~=</math>	<math>~ 2 \sqrt{k} ~F \, ; </math>
<math>~P_{+1 / 2} </math>	<math>~=</math>	<math>~ \frac{2}{ \sqrt{k}}~ E \, ; </math>
<math>~Q_{-1 / 2} </math>	<math>~=</math>	<math>~ 2 \sqrt{k} ~F \, ; </math>
<math>~Q_{+1 / 2} </math>	<math>~=</math>	<math>~ \frac{2}{ \sqrt{k}}~[ F - E] \, ; </math>

where,

<math>~\equiv</math>

and

<math>~\equiv</math>

Toroidal Functions

Relationship between one another, as per equation (8) in A. Gil, J. Segura, & N. M. Temme (2000, JCP, 161, 204 - 217):

<math>~Q_{n-1 / 2}^m (\lambda)</math>

<math>~(-1)^n \frac{\pi^{3/2}}{\sqrt{2} \Gamma(n-m+1 / 2)} (x^2-1)^{1 / 4} P_{m-1 / 2}^n(x) \, , </math>

where, <math>~\lambda \equiv x/\sqrt{x^2-1}</math>. Note that the relationship between <math>~\lambda</math> and <math>~x</math> is the same as the relationship between <math>~\cosh\alpha</math> and <math>~\coth\alpha</math>, that is,

<math>~\coth\alpha</math>

<math>~\frac{\pm \cosh\alpha}{ \sqrt{\cosh^2\alpha - 1}} \, ;</math>

or

<math>~\cosh\alpha</math>

<math>~\frac{1}{\sqrt{1-\tanh^2\alpha}} </math>

<math>~\frac{\pm \coth\alpha}{ \sqrt{\coth^2\alpha - 1 }} \, .</math>

Relation to Elliptic Integrals

<math>~P_{-1 / 2}(z)</math>	<math>~=</math>	<math>~ \frac{2}{\pi} \biggl[\frac{2}{z+1}\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{z-1}{z+1}} \biggr) \, ; </math>
<math>~P_{-1 / 2}(\cosh\eta)</math>	<math>~=</math>	<math>~ \biggl[\frac{\pi}{2}~\cosh\biggl(\frac{\eta}{2}\biggr)\biggr]^{-1} ~K\biggl( \tanh \frac{\eta}{2} \biggr) \, ; </math>

Proof that these are the same expressions:

From standard relationships between hyperbolic functions, we know that,

<math>~ \biggl[ 1 - \tanh^2u \biggr]^{1 / 2} </math>

So, if we let <math>~u \equiv \eta/2</math> and make the association,

<math>~\tanh u</math>	<math>~~~\leftrightarrow~~~</math>	<math>~ \sqrt{\frac{z-1}{z+1}} </math>
<math>~\Rightarrow ~~~ \frac{1}{\cosh u}</math>	<math>~=</math>	<math>~ \biggl[1 - \frac{z-1}{z+1} \biggr]^{1 / 2} </math>	<math>~=</math>	<math>~ \biggl[\frac{2}{z+1} \biggr]^{1 / 2} \, . </math>

Also,

<math>~2\biggl[\frac{z+1}{2}\biggr] - 1 = z \, .</math>

Q.E.D.

<math>~Q_{-1 / 2}(z)</math>	<math>~=</math>	<math>~ \biggl[\frac{2}{z+1}\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{2}{z+1} }\biggr) \, ; </math>
<math>~Q_{-1 / 2}(\cosh\eta)</math>	<math>~=</math>	<math>~2 e^{- \eta / 2} ~K(e^{-\eta} ) \, ; </math>

Proof that these are the same expressions:

Copying the Whipple's formula from §14.19 of DLMF,

<math>~\boldsymbol{Q}^{m}_{n-\frac{1}{2}}\left(\cosh\xi\right)</math>

<math>~ \frac{\Gamma\left(m-n+ \tfrac{1}{2}\right)}{\Gamma\left(m+n+\tfrac{1}{2}\right)}\left(\frac{\pi}{2 \sinh\xi}\right)^{1 / 2}P^{n}_{m-\frac{1}{2}}\left(\coth\xi\right) \, , </math>

then setting <math>~m = n = 0</math>, we have,

<math>~\boldsymbol{Q}^{0}_{-\frac{1}{2}}\left(\cosh\xi\right)</math>

<math>~ \frac{\Gamma\left(\tfrac{1}{2}\right)}{\Gamma\left(\tfrac{1}{2}\right)}\left(\frac{\pi}{2\sinh\xi}\right)^{1 / 2}P^{0}_{-\frac{1}{2}}\left(\coth\xi\right) \, . </math>

Step #1: Associate … <math>z \leftrightarrow \cosh\xi</math>. Then,

<math>~\boldsymbol{Q}^{0}_{-\frac{1}{2}}\left(\cosh\xi\right)</math>

<math>~ \biggl(\frac{\pi}{2} \biggr)^{1/2} \left[\frac{1}{\sqrt{z^2-1}}\right]^{1 / 2} P^{0}_{-\frac{1}{2}}\biggl( \frac{z}{\sqrt{z^2-1}} \biggr) \, . </math>

Step #2: Now making the association … <math>\Lambda \leftrightarrow z/\sqrt{z^2-1}</math>, we can write,

<math>~P_{-1 / 2}(\Lambda)</math>	<math>~=</math>	<math>~ \frac{2}{\pi} \biggl[\frac{2}{\Lambda+1}\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{\Lambda-1}{\Lambda+1}} \biggr) </math>
	<math>~=</math>	<math>~ \frac{2}{\pi} \biggl[\frac{2\sqrt{z^2-1} }{z+\sqrt{z^2-1} }\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{z-\sqrt{z^2-1} }{z+\sqrt{z^2-1} }} \biggr) \, . </math>

Step #3: Again, making the association … <math>z \leftrightarrow \cosh\xi</math>, means,

<math>~P_{-1 / 2}(\Lambda)</math>	<math>~=</math>	<math>~ \frac{2}{\pi} \biggl[\frac{2\sinh\xi }{\cosh\xi+\sinh\xi }\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{\cosh\xi-\sinh\xi }{\cosh\xi+\sinh\xi }} \biggr) </math>
<math>~\Rightarrow ~~~ \boldsymbol{Q}^{0}_{-\frac{1}{2}}\left(\cosh\xi\right)</math>	<math>~=</math>	<math>~\biggl[ \frac{\pi}{2\sinh\xi} \biggr]^{ 1 / 2} \frac{2}{\pi} \biggl[\frac{2\sinh\xi }{\cosh\xi+\sinh\xi }\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{\cosh\xi-\sinh\xi }{\cosh\xi+\sinh\xi }} \biggr) </math>
	<math>~=</math>	<math>~ \frac{2}{\sqrt{\pi}} \biggl[\frac{1 }{\cosh\xi+\sinh\xi }\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{\cosh^2\xi-\sinh^2\xi }{(\cosh\xi+\sinh\xi)^2 }} \biggr) </math>
	<math>~=</math>	<math>~ \frac{2}{\sqrt{\pi}} \biggl[\frac{1 }{\cosh\xi+\sinh\xi }\biggr]^{1 / 2} ~K\biggl( \frac{1}{\cosh\xi+\sinh\xi } \biggr) </math>
	<math>~=</math>	<math>~ \frac{2}{\sqrt{\pi}} ~e^{-\xi/2} ~K( e^{-\xi}) \, , </math>

which, apart from the leading factor of <math>~\pi^{-1 / 2}</math>, exactly matches the above expression.

Note: From Howard Cohl's online overview — see, also, below — we find that the Whipple formula is slightly different from the one (quoted above) drawn from DLMF. According to Cohl the Whipple formula should be,

<math>~Q_{n- 1 / 2}^m(\cosh\alpha)</math>

<math>~ (-1)^m \Gamma (m - n + \tfrac{1}{2} )\biggl( \frac{\pi}{2\sinh\alpha} \biggr)^{1 / 2} P^{n}_{m - 1 / 2}(\coth\alpha) \, . </math>

The DLMF expression needs to be multiplied by <math>~(-1)^m\Gamma (m + n + \tfrac{1}{2} )</math> in order to match the expression provided by Cohl; for the case being considered here of <math>~m=n=0</math>, this factor is precisely <math>~\Gamma(\tfrac{1}{2}) = \sqrt{\pi}</math> — see, for example, Wikipedia's discussion of the gamma function — which cancels this confusing factor of <math>~\pi^{-1 / 2}</math>.

<math>~P_{+1 / 2}(z)</math>	<math>~=</math>	<math>~ \frac{2}{\pi} \biggl[ z + \sqrt{z^2-1} \biggr]^{1 / 2} ~E\biggl( \sqrt{ \frac{2(z^2-1)^{1 / 2}}{z + (z^2-1)^{1 / 2}}} \biggr) \, ; </math>
<math>~P_{+ 1 / 2}(\cosh\eta)</math>	<math>~=</math>	<math>~ \frac{2}{\pi}~e^{\eta/2} ~E\biggl( \sqrt{1-e^{-2\eta}} \biggr) \, ; </math>

Proof that these are the same expressions:

If we associate,

<math>~e^\eta</math>	<math>~~~\leftrightarrow~~~</math>	<math>~ z + \sqrt{z^2-1} </math>
<math>~\Rightarrow ~~~1 - e^{-2\eta}</math>	<math>~=</math>	<math>~ 1 - \frac{1}{[z + \sqrt{z^2-1}]^2} </math>
	<math>~=</math>	<math>~ \frac{2z^2 + 2z\sqrt{z^2-1} -2}{[z + \sqrt{z^2-1}]^2} </math>
	<math>~=</math>	<math>~ \frac{2[z + \sqrt{z^2-1}] \sqrt{z^2-1}}{[z + \sqrt{z^2-1}]^2} </math>
	<math>~=</math>	<math>~ \frac{2\sqrt{z^2-1}}{[z + \sqrt{z^2-1}]} \, . </math>

It also means that,

<math>~\cosh \eta = \tfrac{1}{2}[e^\eta + e^{-\eta}]</math>	<math>~=</math>	<math>~ \frac{1}{2}\biggl[z + \sqrt{z^2-1} + \frac{1}{z + \sqrt{z^2-1}} \biggr] </math>
	<math>~=</math>	<math>~ \frac{1}{2}\biggl[\frac{z^2 + 2z\sqrt{z^2-1} + (z^2-1) + 1}{z + \sqrt{z^2-1}} \biggr] </math>
	<math>~=</math>	<math>~ \frac{1}{2}\biggl[\frac{2z^2 + 2z\sqrt{z^2-1} }{z + \sqrt{z^2-1}} \biggr] </math>
	<math>~=</math>	<math>~ z \, . </math>

Q.E.D.

<math>~z \biggl[\frac{2}{z+1}\biggr]^{1 / 2} ~K\biggl( \sqrt{ \frac{2}{z+1} }\biggr) - \biggl[2(z+1)\biggr]^{1 / 2} E\biggl( \sqrt{\frac{2}{z+1}} \biggr) \, . </math>

When the argument, <math>~x</math>, lies in the range, <math>~-1 < x < 1</math>:

<math>~P_{-1 / 2}(x)</math>	<math>~=</math>	<math>~ \frac{2}{\pi} ~K\biggl( \sqrt{ \frac{1-x}{2} } \biggr) \, ; </math>
<math>~P_{-1 / 2}(\cos\theta)</math>	<math>~=</math>	<math>~ \frac{2}{\pi} ~K\biggl( \sin \frac{\theta}{2}\biggr) \, ; </math>
<math>~Q_{-1 / 2}(x)</math>	<math>~=</math>	<math>~ K\biggl( \sqrt{ \frac{1+x}{2} } \biggr) \, ; </math>
<math>~P_{+1 / 2}(x)</math>	<math>~=</math>	<math>~ \frac{2}{\pi} \biggl[2E\biggl( \sqrt{ \frac{1-x}{2} } \biggr) - ~K\biggl( \sqrt{ \frac{1-x}{2} } \biggr) \biggr] \, ; </math>
<math>~Q_{+ 1 / 2}(x)</math>	<math>~=</math>	<math>~ K\biggl( \sqrt{ \frac{1+x}{2} } \biggr) - 2E\biggl( \sqrt{ \frac{1+x}{2} } \biggr)\, ; </math>

Piece Together

When <math>~\mu = 0</math>, and <math>~\nu = (m- 3/ 2)</math>, the recurrence relation should be …

<math>~(m - \tfrac{1}{2})P_{m-1 / 2}(z)</math>	<math>~=</math>	<math>~ [2m-2]zP_{m-3 / 2} (z) -(m - \tfrac{3}{2} )P_{m - 5 / 2} (z) </math>
<math>~\Rightarrow ~~~(2m -1)P_{m - 1 / 2}(z)</math>	<math>~=</math>	<math>~ 4(m-1)zP_{m - 3 /2 } (z) - (2m -3)P_{m-5 / 2} (z) </math>
<math>~\Rightarrow ~~~P_{m - 1 / 2}(z)</math>	<math>~=</math>	<math>~\biggl[ \frac{ 4(m-1)zP_{m - 3 /2 } (z) - (2m -3)P_{m-5 / 2} (z) }{(2m -1)} \biggr] \, , </math>

for all <math>~m \ge 2</math>.

Overview by Howard Cohl

This subsection is drawn verbatim from Howard Cohl's online overview of toroidal functions.

… These last two expressions allow us to express toroidal functions of a certain kind (first or second, respectively) with argument hyperbolic cosine, as a direct proportionality in terms of the toroidal function of the other kind (second or first, respectively) with argument hyperbolic cotangent. The Whipple formulae may also be expressed as follows:

<math>~Q^n_{m- 1 / 2}(\cosh\alpha)</math>	<math>~=</math>	<math>~(-1)^n ~\Gamma(n-m + \tfrac{1}{2}) \biggl[ \frac{\pi}{2\sinh\alpha} \biggr]^{1 / 2} P^m_{n- 1 / 2}(\coth\alpha)\, , </math>
… and …
<math>~Q^n_{m- 1 / 2}(\coth\alpha)</math>	<math>~=</math>	<math>~(-1)^m ~\frac{\pi}{\Gamma(m-n + \tfrac{1}{2})} \biggl[ \frac{\pi \sinh\alpha}{2} \biggr]^{1 / 2} P^m_{n- 1 / 2}(\cosh\alpha) \, . </math>

These interesting formulae have the property that they can relate Legendre functions of the first and second kinds directly in terms of each other. The only hitch is that you need a different argument to relate them. The way it works is as such. The Legendre functions of the first kind generally are well-behaved near the origin and blow up at positive infinity. Consequently the Legendre functions of the second kind blow up at unity and exponentially converges towards zero for large values of the argument. The relevant domain for toroidal functions is from 1 to infinity. The standard hyperbolic argument for these functions are naturally chosen to be the hyperbolic cosine since it ranges from 1 to infinity. The Whipple formulae relate the Legendre functions with argument 1 to infinity, cosh, to a reversed range given by the hyperbolic cotangent function. the hyperbolic cotangent function ranges from infinity at unity to unity at infinity. At what point alpha does cosh alpha equal coth alpha? The point alpha is given by

<math>~\alpha</math>

Therefore, <math>~e^\alpha</math> and <math>~e^{-\alpha}</math> are given, respectively, by

<math>~e^\alpha</math>

and

<math>~e^{-\alpha}</math>

The value that <math>~\cosh \alpha</math> and <math>~\coth \alpha</math> obtain at <math>~\alpha</math> is given by

<math>~\cosh\alpha = \coth\alpha</math>

The value that <math>~1/\cosh\alpha</math> and <math>~\tanh \alpha</math> obtain at <math>~\alpha</math> is given by

<math>~\frac{1}{\cosh\alpha} = \tanh\alpha</math>

Finally, <math>~\sinh \alpha</math> and it's inverse are given respectively by unity,

<math>~\sinh\alpha = \frac{1}{\sinh\alpha} </math>

We now see that the value at which the argument of the Legendre functions inversely maps the entire domain is given by cosh alpha = coth alpha ~ 1.4142356. By using the Whipple formulae for ring functions, we can inversely map the entire domain from 1 to infinity about this point cosh alpha, the square root of 2, and take full advantage of this new symmetry for Legendre functions. There being previously more definite and indefinite integrals tabulated for the Legendre function of the first kind than for the Legendre function of the second kind. In fact, this new transformation, when applied to toroidal functions yields distinct expressions which relate correspondingly the complete elliptic integrals of the first and second kind, which don't seem to be related to the linear and quadratic transformations of hypergeometric functions.

Note that, as shown above, Gil, et al. (2000) state:

<math>~Q_{n-1 / 2}^m (\lambda)</math>

<math>~(-1)^n \frac{\pi^{3/2}}{\sqrt{2} \Gamma(n-m+1 / 2)} (x^2-1)^{1 / 4} P_{m-1 / 2}^n(x) \, , </math>

where, <math>~\lambda \equiv x/\sqrt{x^2-1}</math>. Hence, if we swap the indexes, <math>~m\leftrightarrow n</math>, and make the assignments, <math>~x \leftrightarrow \cosh\alpha</math> and <math>~\lambda \leftrightarrow \coth\alpha</math>, this last relation becomes,

<math>~Q_{m-1 / 2}^n (\coth\alpha)</math>	<math>~=</math>	<math>~(-1)^m \frac{\pi^{3/2}}{\sqrt{2} \Gamma(m-n+1 / 2)} (\cosh^2\alpha-1)^{1 / 4} P_{n-1 / 2}^m(\cosh\alpha) </math>
	<math>~=</math>	<math>~(-1)^m \frac{\pi}{ \Gamma(m-n+1 / 2)} \biggl[ \frac{\pi\sinh\alpha }{2} \biggr]^{1 / 2} P_{n-1 / 2}^m(\cosh\alpha) </math>

Drawn From Discussion of Solving the Poisson Equation

The following has been copied (May 2018) from an accompanying chapter that presents the integral representation of the Poisson equation in terms of toroidal functions.

Table 5: Green's Function in Terms of
Zero Order, Half-(Odd)Integer Degree, Associated Legendre Functions of the Second Kind, <math>~Q^0_{m-1 / 2}(\chi)</math>
(also referred to as Toroidal Functions)

<math>~ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=-\infty}^{\infty} e^{im(\phi - \phi^')}Q_{m- 1 / 2}(\chi) </math>

where:

<math>~\chi \equiv \frac{\varpi^2 + (\varpi^')^2 + (z - z^')^2}{2\varpi \varpi^'}</math>

H. S. Cohl & J. E. Tohline (1999), p. 88, Eqs. (15) & (16)
See also the DLMF's definition of Toroidal Functions, <math>~Q_{m - 1 / 2}^{0}</math>

Note that, according to, for example, equation (8.731.5) of Gradshteyn & Ryzhik (1994),

Hence, the Green's function can straightforwardly be rewritten in terms of a simpler summation over just non-negative values of the index, <math>~m</math>.

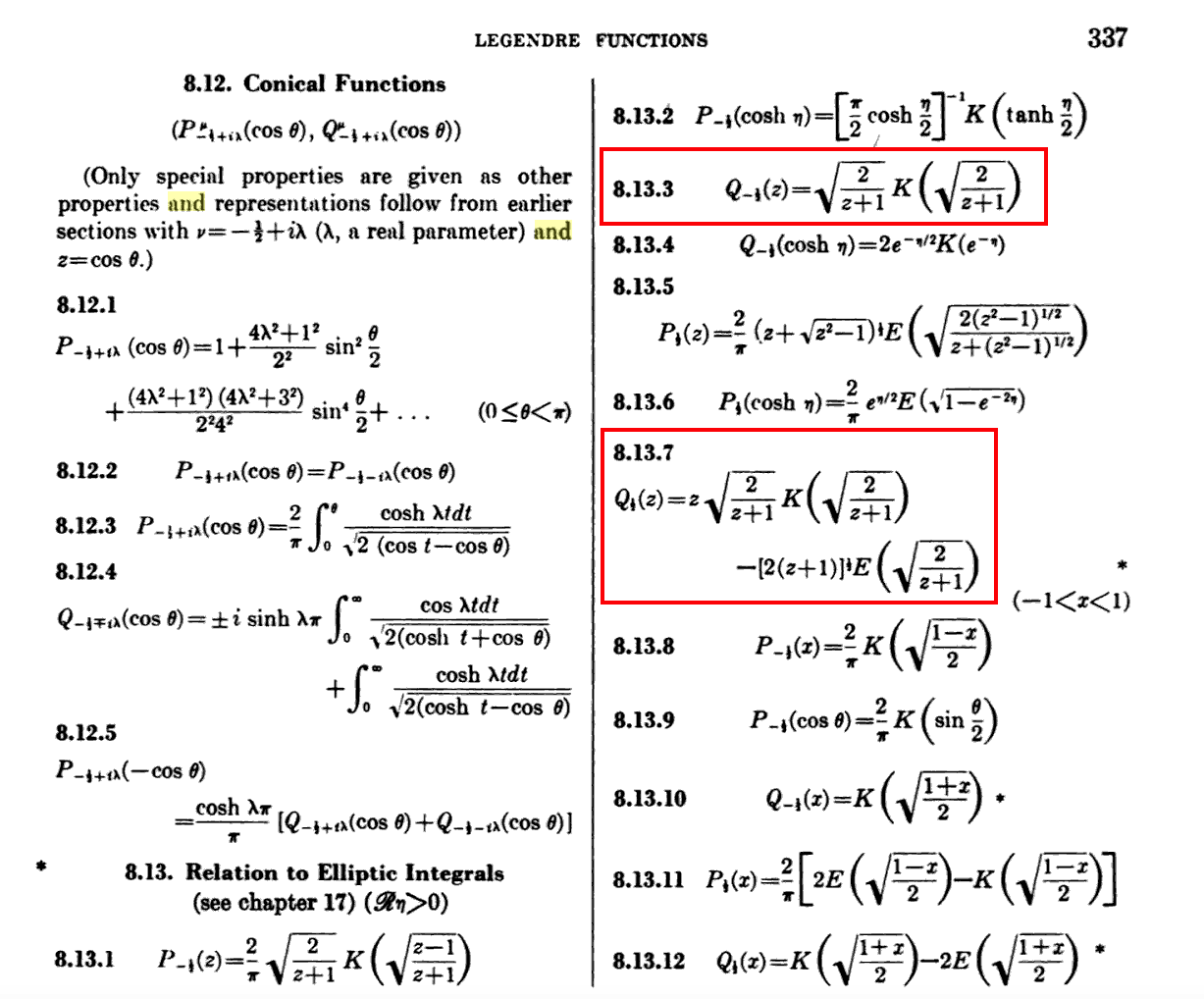
Referencing equations (8.13.3) and (8.13.7), respectively, of Abramowitz & Stegun (1965), we see that for the smallest two values of the non-negative index, <math>~m</math>, the function, <math>~Q_{m- 1 / 2}(\chi)</math>, can be rewritten in terms of, the more familiar, complete elliptic integrals of the first and second kind. Specifically,

for <math>~m = 0</math>,
<math>~Q_{m- 1 / 2}(\chi) ~\rightarrow~ Q_{-1 / 2}</math>	<math>~=</math>	<math>~ \mu K(\mu) \, , </math>
and, for <math>~m = 1</math>,
<math>~Q_{m- 1 / 2}(\chi) ~\rightarrow~ Q_{+ 1 / 2}</math>	<math>~=</math>	<math>~ \chi \mu K(\mu) - (1+\chi)\mu E(\mu) \, , </math>
where,
<math>~\mu \equiv \biggl[ \frac{2}{1+\chi} \biggr]^{1 / 2}</math>	<math>~=</math>	<math>~ \biggl[ \frac{4\varpi \varpi^'}{(\varpi + \varpi^')^2 + (z - z^')^2} \biggr]^{1 / 2} \, . </math>

Excerpt from p. 337 of M. Abramowitz & I. A. Stegun (1995)

Finally, equation (8.5.3) from Abramowitz & Stegun (1965) or equation (8.832.4) of Gradshteyn & Ryzhik (1994) — also see equation (2) of Gil, Segura & Temme (2000) — provide the recurrence relation for all other values of the index, <math>~m</math>. Specifically, for all <math>~m \ge 2</math>,

<math>~Q_{m - 1 / 2}(\chi) = 4\biggl[\frac{m-1}{2m-1}\biggr] \chi Q_{m- 3 / 2}(\chi) - \biggl[ \frac{2m-3}{2m-1}\biggr] Q_{m- 5 / 2}(\chi) \, .</math>

Excerpt from p. 490 of W. Guatschi (1965, Communications of the ACM, 8, 488 - 492)

Just Compare Green Functions

According to CT99, the Green's function written in toroidal coordinates is,

<math>~ \frac{1}{\|\vec{x} - \vec{x}^{~'}\|}</math>	<math>~=</math>	<math>~ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=-\infty}^{\infty} e^{im(\phi - \phi^')}Q_{m- 1 / 2}(\chi) \, , </math>
	<math>~=</math>	<math>~ \frac{1}{\pi \sqrt{\varpi \varpi^'}} \sum_{m=0}^{\infty} \epsilon_m \cos[m(\phi - \phi^')] Q_{m- 1 / 2}(\chi) \, , </math>

where,

<math>~\chi \equiv \frac{\varpi^2 + (\varpi^')^2 + (z - z^')^2}{2\varpi \varpi^'} \, ,</math>

and, <math>~Q_{m - 1 / 2}</math> is the zero-order, half-(odd)integer degree, Llegendre function of the second kind — also referred to as a toroidal function of zeroth order.

From the Dyson-Wong Toroid chapter,

<math>~\varpi</math>	<math>~=</math>	<math>~\frac{a \sinh\eta }{(\cosh\eta - \cos\theta)} \, ,</math>
<math>~z</math>	<math>~=</math>	<math>~\frac{a \sin\theta}{(\cosh\eta - \cos\theta)} \, .</math>

Hence, we can rewrite the Green's function as,

<math>~ \frac{1}{\|\vec{x} - \vec{x}^{~'}\|}</math>	<math>~=</math>	<math>~ \frac{1}{\pi} \biggl[ \frac{a \sinh\eta^' }{(\cosh\eta^' - \cos\theta^')} \frac{a \sinh\eta }{(\cosh\eta - \cos\theta)}\biggr]^{- 1 / 2} \sum_{m=0}^{\infty} \epsilon_m \cos[m(\phi - \phi^')] Q_{m- 1 / 2}(\chi) </math>
	<math>~=</math>	<math>~ \frac{1}{\pi a} [\sinh\eta^' \sinh\eta]^{- 1 / 2} \biggl[ (\cosh\eta^' - \cos\theta^') (\cosh\eta - \cos\theta)\biggr]^{1 / 2} \sum_{m=0}^{\infty} \epsilon_m \cos[m(\phi - \phi^')] Q_{m- 1 / 2}(\chi) </math>

Wong (1973) states that in toroidal coordinates the Green's function is,

<math>~\frac{1}{\|~\vec{r} - {\vec{r}}^{~'} ~\|} </math>	<math>~=</math>	<math>~ \frac{1}{\pi a} \biggl[ (\cosh\eta - \cos\theta)(\cosh \eta^' - \cos\theta^') \biggr]^{1 /2 } \sum\limits_{m,n} (-1)^m \epsilon_m \epsilon_n ~\frac{\Gamma(n-m+\tfrac{1}{2})}{\Gamma(n + m + \tfrac{1}{2})} </math>
		<math>~ \times \cos[m(\psi - \psi^')][\cos[n(\theta - \theta^')] ~\begin{cases}P^m_{n-1 / 2}(\cosh\eta) ~Q^m_{n-1 / 2}(\cosh\eta^') ~~~\eta^' > \eta \\P^m_{n-1 / 2}(\cosh\eta^') ~Q^m_{n-1 / 2}(\cosh\eta)~~~\eta^' < \eta \end{cases}\, , </math>
Wong (1973), Eq. (2.53)

where, <math>~P^m_{n-1 / 2}, Q^m_{n-1 / 2}</math> are "Legendre functions of the first and second kind with order <math>~n - \tfrac{1}{2}</math> and degree <math>~m</math> (toroidal harmonics)," and <math>~\epsilon_m</math> is the Neumann factor, that is, <math>~\epsilon_0 = 1</math> and <math>~\epsilon_m = 2</math> for all <math>~m \ge 1</math>,

<math>~\eta</math>	<math>~=</math>	<math>~\ln\biggl(\frac{r_1}{r_2} \biggr) \, ,</math>
<math>~\cos\theta</math>	<math>~=</math>	<math>~\frac{(r_1^2 + r_2^2 - 4a^2)}{2r_1 r_2} \, ,</math>
<math>~\tan\psi</math>	<math>~=</math>	<math>~\frac{y}{x} \, ,</math>

and,

<math>~r_1^2 </math>	<math>~\equiv</math>	<math>~[(x^2 + y^2)^{1 / 2} + a]^2 + z^2 \, ,</math>
<math>~r_2^2 </math>	<math>~\equiv</math>	<math>~[(x^2 + y^2)^{1 / 2} - a]^2 + z^2 \, ,</math>

and <math>~\theta</math> has the same sign as <math>~z</math>.

Note that:

<math>~\cosh\eta</math>	<math>~=</math>	<math>~\frac{1}{2}\biggl[ e^\eta + e^{-\eta} \biggr] = ~\frac{1}{2}\biggl[ \frac{r_1}{r_2} + \frac{r_2}{r_1}\biggr]</math>
	<math>~=</math>	<math>~\frac{1}{2}\biggl[ \frac{\sqrt{[ \varpi + a]^2 + z^2}}{\sqrt{[ \varpi - a]^2 + z^2}} + \frac{\sqrt{[ \varpi - a]^2 + z^2}}{\sqrt{[ \varpi + a]^2 + z^2}}\biggr]</math>
<math>~\Rightarrow ~~~ 4\cosh^2\eta</math>	<math>~=</math>	<math>~\biggl\{ \frac{ [( \varpi + a)^2 + z^2]^{1 / 2} }{ [( \varpi - a)^2 + z^2]^{1 / 2}} + \frac{[( \varpi - a)^2 + z^2]^{1 / 2}}{ [( \varpi + a)^2 + z^2]^{1 / 2} }\biggr\}^2</math>
<math>~\Rightarrow ~~~ 4\cosh^2\eta~ [( \varpi + a)^2 + z^2] [( \varpi - a)^2 + z^2]</math>	<math>~=</math>	<math>~\biggl\{ [( \varpi + a)^2 + z^2] + [( \varpi - a)^2 + z^2] \biggr\}^2</math>
<math>~\Rightarrow ~~~ 4\cosh^2\eta~ [ \varpi^2 + 2a\varpi + a^2 + z^2] [ \varpi^2 -2a\varpi + a^2 + z^2]</math>	<math>~=</math>	<math>~4 [ \varpi^2 + a^2 + z^2]^2</math>
<math>~\Rightarrow ~~~ \frac{ [ \varpi^2 + a^2 + z^2]^2}{\cosh^2\eta} </math>	<math>~=</math>	<math>~ [ \varpi^4 -2a\varpi^3 + a^2\varpi^2 + \varpi^2 z^2 + 2a\varpi^3 - 4a^2\varpi^2 +2a^3\varpi + 2a\varpi z^2 + a^2\varpi^2 - 2a^3\varpi + a^4 + a^2z^2 + \varpi^2 z^2 -2a\varpi z^2 +a^2z^2 + z^4 ] </math>
	<math>~=</math>	<math>~ [ \varpi^4 + 2\varpi^2 z^2 - 2a^2\varpi^2 + a^4 + 2a^2z^2 + z^4 ] </math>

@@ Line 2,155: / Line 2,155: @@
 </font>
+Note that, as shown above, [http://adsabs.harvard.edu/abs/2000JCoPh.161..204G Gil, ''et al.''  (2000)] state:
+<div align="center">
+<table border="0" cellpadding="5" align="center">
+<tr>
+  <td align="right">
+<math>~Q_{n-1 / 2}^m (\lambda)</math>
+  </td>
+  <td align="center">
+<math>~=</math>
+  </td>
+  <td align="left">
+<math>~(-1)^n
+\frac{\pi^{3/2}}{\sqrt{2} \Gamma(n-m+1 / 2)} (x^2-1)^{1 / 4} P_{m-1 / 2}^n(x) \, ,
+</math>
+  </td>
+</tr>
+</table>
+</div>
+where, <math>~\lambda \equiv x/\sqrt{x^2-1}</math>.  Hence, if we swap the indexes, <math>~m\leftrightarrow n</math>, and make the assignments, <math>~x \leftrightarrow \cosh\alpha</math> and <math>~\lambda \leftrightarrow \coth\alpha</math>, this last relation becomes,
+<div align="center">
+<table border="0" cellpadding="5" align="center">
+<tr>
+  <td align="right">
+<math>~Q_{m-1 / 2}^n (\coth\alpha)</math>
+  </td>
+  <td align="center">
+<math>~=</math>
+  </td>
+  <td align="left">
+<math>~(-1)^m
+\frac{\pi^{3/2}}{\sqrt{2} \Gamma(m-n+1 / 2)} (\cosh^2\alpha-1)^{1 / 4} P_{n-1 / 2}^m(\cosh\alpha)
+</math>
+  </td>
+</tr>
+<tr>
+  <td align="right">
+&nbsp;
+  </td>
+  <td align="center">
+<math>~=</math>
+  </td>
+  <td align="left">
+<math>~(-1)^m
+\frac{\pi}{ \Gamma(m-n+1 / 2)} \biggl[ \frac{\pi\sinh\alpha }{2} \biggr]^{1 / 2} P_{n-1 / 2}^m(\cosh\alpha)
+</math>
+  </td>
+</tr>
+</table>
+</div>
 =Drawn From Discussion of Solving the Poisson Equation=

Difference between revisions of "User:Tohline/Appendix/Mathematics/ToroidalFunctions"

Revision as of 00:35, 30 May 2018

Contents

Relationships Between Toroidal Functions

Wong Toroidal Coordinates

Prior to the Integration

CT99 Coordinates

Observer Toroidal Coordinates

Our Mucking Around

Recurrence Relations

Toroidal Functions

Piece Together

Overview by Howard Cohl

Drawn From Discussion of Solving the Poisson Equation

Just Compare Green Functions

See Also

Navigation menu

Search