Revision as of 22:51, 10 June 2017

Ledoux's Variational Principle

All of the discussion in this chapter will build upon our derivation elsewhere of the,

LAWE: Linear Adiabatic Wave (or Radial Pulsation) Equation

<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math>

We will draw heavily from the papers published by Ledoux & Pekeris (1941) and by S. Chandrasekhar (1964), as well as from pp. 458-474 of the review by P. Ledoux & Th. Walraven (1958) in explaining how the variational principle can be used to identify the eigenvector of the fundamental mode of radial oscillation in spherically symmetric configurations. In an associated "Ramblings" appendix, we provide various derivations that support this chapter's relatively abbreviated presentation.

Ledoux and Pekeris (1941)

Historically, by the 1940s, the LAWE was a relatively familiar one to astrophysicists. For example, the opening paragraph of a 1941 paper by Ledoux & Pekeris (1941, ApJ, 94, 124), reads:

Paragraph extracted from P. Ledoux & C. L. Pekeris (1941)

"Radial Pulsations of Stars"

ApJ, vol. 94, pp. 124-135 © American Astronomical Society

If we divide their equation (1) through by <math>~Xr = \Gamma_1 P r</math> and recognize that,

<math> \frac{dX}{dr} = \frac{dX}{dm}\frac{dm}{dr} = - \Gamma_1 g_0 \rho \, , </math>

we obtain,

<math> \frac{d^2\xi}{dr^2} + \biggl[ \frac{4}{r} - \frac{g_0 \rho}{P} \biggr] \frac{d\xi}{dr} +\frac{\rho}{\Gamma_1 P} \biggl[ \sigma^2 + (4 - 3\Gamma_1) \frac{g_0}{r} \biggr] \xi = 0 \, . </math>

Clearly, this 2^nd-order, ordinary differential equation is the same as our derived LAWE, but with a more general definition of the adiabatic exponent that allows consideration of a situation where the total pressure is a sum of both gas and radiation pressure.

Multiplying this last equation through by <math>~\Gamma_1 P r^4</math>, and recognizing that,

<math>~(r^4 \Gamma_1 P)\frac{d^2\xi}{dr^2} </math>

<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \frac{d\xi}{dr} \cdot \frac{d}{dr} \biggl[ r^4 \Gamma_1 P\biggr] </math>

we can write,

<math>~0</math>	<math>~=</math>	<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \frac{d\xi}{dr} \cdot \frac{d}{dr} \biggl[ r^4 \Gamma_1 P\biggr] + ( \Gamma_1 P r^4 ) \biggl[ \frac{4}{r} - \frac{g_0 \rho}{P} \biggr] \frac{d\xi}{dr} +\rho \biggl[ \sigma^2 r^4 + (4 - 3\Gamma_1) g_0 r^3\biggr] \xi </math>
	<math>~=</math>	<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \biggl[4r^3\Gamma_1 P + \Gamma_1 r^4 \frac{dP}{dr} \biggr] \frac{d\xi}{dr} + \biggl[ 4 r^3\Gamma_1 P + \Gamma_1 r^4 \frac{dP}{dr}\biggr] \frac{d\xi}{dr} +\biggl[ \sigma^2 \rho r^4 - (4 - 3\Gamma_1) r^3 \frac{dP}{dr} \biggr] \xi </math>
	<math>~=</math>	<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] +\biggl[ \sigma^2 \rho r^4 + (3\Gamma_1 - 4) r^3 \frac{dP}{dr} \biggr] \xi </math>
	<math>~=</math>	<math>~ \frac{d}{dr}\biggl[ \Gamma_1 P r^4 ~\frac{d\xi}{dr} \biggr] +\biggl[ \sigma^2 r^4 \rho + 4 Gm (r ) r \rho + 3\Gamma_1 r^3 \frac{dP}{dr} \biggr] \xi \, . </math>

Assuming that <math>~\Gamma_1</math> is uniform throughout the configuration, this last expression is the same as equation (3) of Ledoux & Pekeris (1941), while the next-to-last expression is identical to equation (58.1) of Ledoux & Walraven (1958).

Stability Based on Variational Principle

Here we derive the Lagrangian directly from the governing LAWE. We begin with the next-to-last derived form of the LAWE that appears above in our review of the paper by Ledoux & Pekeris (1941) and, following the guidance provided at the top of p. 666 of S. Chandrasekhar (1964, ApJ, 139, 664), we multiply the LAWE through by the fractional displacement, <math>~\xi</math>. This gives, what we will henceforth refer to as, the,

Foundational Variational Relation
<math>~\sigma^2 \rho r^4 \xi^2</math>	<math>~=</math>	<math>~ -\xi \cdot \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) \, . </math>

Chandrasekhar's Approach

Next, in an effort to adopt the notation used by Chandrasekhar (1964), we make the substitution, <math>~\xi \rightarrow \psi/r^3</math>, and regroup terms to obtain,

<math>~\frac{\sigma^2 \rho \psi^2}{r^2}</math>	<math>~=</math>	<math>~ - \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d}{dr} \biggl( \frac{\psi}{r^3} \biggr) \biggr] - (3\Gamma_1 - 4) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr) </math>
	<math>~=</math>	<math>~ - \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r \Gamma_1 P ~\frac{d\psi}{dr} -3 \Gamma_1 P \psi ~\biggr] - (3\Gamma_1 - 4) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr) </math>
	<math>~=</math>	<math>~ (4-3\Gamma_1 ) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr) - \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r \Gamma_1 P ~\frac{d\psi}{dr} \biggr] + 3 \Gamma_1 \biggl( \frac{\psi^2}{r^3}\biggr) \frac{dP}{dr} +3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr} </math>
	<math>~=</math>	<math>~ 4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr) +3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr} - \biggl\{ \frac{d}{dr}\biggl[ r \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}\biggr] -r\Gamma_1 P ~\frac{d\psi}{dr} \cdot \frac{d}{dr}\biggl( \frac{\psi}{r^3}\biggr) \biggr\} </math>
	<math>~=</math>	<math>~ 4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr) +3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr} + \frac{\Gamma_1 P}{r^2} \biggl[\frac{d\psi}{dr} \biggr]^2 - \biggl[\frac{3\Gamma_1 P\psi}{r^3}\biggr]\frac{d\psi}{dr} - \frac{d}{dr}\biggl[\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr] </math>
	<math>~=</math>	<math>~ 4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr) + \frac{\Gamma_1 P}{r^2} \biggl[\frac{d\psi}{dr} \biggr]^2 - \frac{d}{dr}\biggl[ \frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr] \, . </math>

Multiplying through by <math>~dr</math>, and integrating over the volume gives,

<math>~\int_0^R (\sigma^2 \rho \psi^2)\frac{dr}{r^2}</math>

<math>~ \int_0^R \biggl[ \Gamma_1 P \biggl(\frac{d\psi}{dr} \biggr)^2 + \frac{4\psi^2}{r} \biggl( \frac{dP}{dr} \biggr) \biggr]\frac{dr}{r^2} - \biggl[\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr]_0^R \, , </math>

which is identical to equation (49) of Chandrasekhar (1964), if the last term — the difference of the central and surface boundary conditions — is set to zero.

Note that if we shift from the variable, <math>~\psi</math>, back to the fractional displacement function, <math>~\xi</math>, the last term in this expression may be written as,

<math>~\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr)</math>	<math>~=</math>	<math>~ \Gamma_1 P r \xi \frac{d}{dr} \biggl[r^3 \xi\biggr] </math>
	<math>~=</math>	<math>~ \Gamma_1 P r \xi \biggl[3r^2 \xi + r^3 \frac{d\xi}{dr}\biggr] </math>
	<math>~=</math>	<math>~ \Gamma_1 P r^3 \xi^2 \biggl[3 + \frac{d\ln\xi}{d\ln r}\biggr] \, . </math>

So, as is pointed out by Ledoux & Walraven (1958) in connection with their equation (57.31), setting this expression to zero at the surface of the configuration is equivalent to setting the variation of the pressure to zero at the surface. Quite generally, this can be accomplished by demanding that,

<math>~\frac{d\ln\xi}{d\ln r}\biggr|_\mathrm{surface} = -3 \, .</math>

(An accompanying chapter provides a broader discussion of this and other astrophysically reasonable boundary conditions that are associated with solutions to the LAWE.)

Ledoux & Walraven Approach

Returning to the above Foundational Variational Relation, we can also write,

<math>~\sigma^2 \rho r^4 \xi^2</math>	<math>~=</math>	<math>~ -\xi \cdot \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) </math>
	<math>~=</math>	<math>~ r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2 - (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) - \frac{d}{dr}\biggr[r^4 \Gamma_1 P\xi \biggl(\frac{d\xi}{dr}\biggr) \biggr] </math>
<math>~\Rightarrow ~~~ \int_0^R\sigma^2 \rho r^4 \xi^2 dr</math>	<math>~=</math>	<math>~ \int_0^R r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2 dr - \int_0^R (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) dr - \biggr[r^4 \Gamma_1 P\xi \biggl(\frac{d\xi}{dr}\biggr) \biggr]_0^R </math>

If the last term (boundary conditions) is set to zero, then we may also write,

<math>~\sigma^2 </math>

<math>~ \frac{\int_0^R r^4 \Gamma_1 P \bigl(\frac{d\xi}{dr}\bigr)^2 dr - \int_0^R (3\Gamma_1 - 4) r^3 \xi^2 \bigl( \frac{dP}{dr} \bigr) dr}{\int_0^R \rho r^4 \xi^2 dr} \, . </math>

This means that, if the radial profile of the pressure and the density is known throughout a spherically symmetric, equilibrium configuration, and if, furthermore, the eigenfunction, <math>~\xi(r)</math>, of a radial oscillation mode is specified precisely, then this expression will give the (square of the) eigenfrequency of that oscillation mode.

By using formal variational principle techniques to derive this same expression, Ledoux & Walraven (1958) are able to offer a broader interpretation, which is encapsulated by their equation (59.10), viz.,

<math>~\sigma_0^2 </math>

<math>~\mathrm{min}~ \frac{\int_0^R r^4 \Gamma_1 P \bigl(\frac{d\xi}{dr}\bigr)^2 dr - \int_0^R (3\Gamma_1 - 4) r^3 \xi^2 \bigl( \frac{dP}{dr} \bigr) dr}{\int_0^R \rho r^4 \xi^2 dr} \, . </math>

This means that, if the exact radial eigenfunction, <math>~\xi(r)</math>, is not known, various approximate eigenfunctions can be tried. The trial eigenfunction that minimizes the righthand-side of this expression will give the (square of the) eigenfrequency of the fundamental mode of oscillation (subscript zero). Furthermore, via an evaluation of this righthand-side expression, any reasonable trial eigenfunction — for example, <math>~\xi</math> = constant — can provide an upper limit to <math>~\sigma_0^2</math>.

Ledoux & Pekeris Approach

Here we follow the lead of Ledoux & Pekeris (1941). Returning to the integral expression just derived in our discussion of the Ledoux & Walraven approach, and multiplying through by <math>~4\pi</math>, we have,

<math>~\int_0^R 4\pi \sigma^2 \rho r^4 \xi^2 dr</math>

<math>~ \int_0^R 4\pi r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2 dr - \int_0^R (3\Gamma_1 - 4) 4\pi r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) dr - \biggr[4\pi r^3 \Gamma_1 P\xi^2 \biggl(\frac{d\ln \xi}{d\ln r}\biggr) \biggr]_0^R \, . </math>

If we acknowledge that:

at the center of the configuration, <math>~r^3 = 0</math>;
as above, the boundary condition at the surface is <math>~P = P_e</math> while <math>~(d\ln \xi/d\ln r) = -3</math>;
the differential mass element is, <math>~dm = 4\pi r^2 \rho dr</math> and the corresponding differential volume element is, <math>~dV = 4\pi r^2 dr</math>; and
a statement of detailed force balance is, <math>~dP/dr = - Gm\rho/r^2</math>,

this integral relation becomes,

<math>~ \sigma^2 \int_0^R r^2 \xi^2 dm</math>

<math>~ \Gamma_1 \int_0^R \biggl[ r \biggl(\frac{d\xi}{dr}\biggr)\biggr]^2 P dV + (3\Gamma_1 - 4) \int_0^R \xi^2 \biggl( \frac{Gm}{r} \biggr) dm - \biggr[\Gamma_1 \xi_\mathrm{surface}^2 (3P_e V) \biggl(-3\biggr) \biggr] \, . </math>

Now, as we have discussed separately — see, also, p. 64, Equation (12) of [C67] — the gravitational potential energy of the unperturbed configuration is given by the integral,

<math>~W_\mathrm{grav}</math>

<math>~ - \int_0^{M} \biggl( \frac{Gm}{r_0} \biggr) dm \, ;</math>

for adiabatic systems, the internal energy is,

<math> U_\mathrm{int} = \frac{1}{(\Gamma_1-1)} \int_0^R P_0 dV

\, ;</math>

and — see the text at the top of p. 126 of Ledoux & Pekeris (1941) — the moment of inertia of the configuration about its center is,

<math> I = \int_0^M r_0^2 dm

\, .</math>

After inserting these expressions, we have what will henceforth be referred to as the,

Variational Principle's Governing Integral Relation
<math>~ \sigma^2 \int_0^R \xi^2 dI</math>	<math>~=</math>	<math>~ \Gamma_1 (\Gamma_1 - 1) \int_0^R \xi^2 \biggl[ \frac{d\ln\xi}{d\ln r}\biggr]^2 dU_\mathrm{int} - (3\Gamma_1 - 4) \int_0^R \xi^2 dW_\mathrm{grav} + 3^2 \Gamma_1 P_e V \xi_\mathrm{surface}^2 \, . </math>

Free-Energy Analysis

If we assume the simplest approximation for the fundamental-mode eigenfunction, namely, <math>~\xi = \xi_0</math> = constant — that is, homologous expansion/contraction — then this last integral expression gives,

<math>~ \sigma^2 I</math>

<math>~ (4 - 3\Gamma_1) W_\mathrm{grav} + 3^2 \Gamma_1 P_e V \, . </math>

Contrast this result with the following free-energy analysis:

<math>~\mathfrak{G}</math>

<math>~W_\mathrm{grav} + U_\mathrm{int} + P_eV \, ,</math>

where, in terms of the configuration's (generally non-equilibrium) dimensionless radius, <math>~\chi \equiv R/R_0</math>,

<math>~W_\mathrm{grav}</math>	<math>~=</math>	<math>~-a\chi^{-1}</math>
<math>~U_\mathrm{int}</math>	<math>~=</math>	<math>~b\chi^{3-3\Gamma_1}</math>
<math>~V</math>	<math>~=</math>	<math>~\frac{4\pi}{3} \chi^3 \, .</math>

Then,

<math>~\frac{\partial \mathfrak{G}}{\partial \chi}</math>	<math>~=</math>	<math>~+a \chi^{-2} + 3(1-\Gamma_1) b \chi^{2-3\Gamma_1} + 4\pi P_e \chi^{2} </math>
	<math>~=</math>	<math>~\chi^{-1} \biggl[- W_\mathrm{grav} + 3(1-\Gamma_1) U_\mathrm{int} + 3 P_e V \biggr] \, ,</math>

and,

<math>~\frac{\partial^2 \mathfrak{G}}{\partial \chi^2}</math>	<math>~=</math>	<math>~-2a \chi^{-3} + 3(1-\Gamma_1)(2-3\Gamma_1) b \chi^{1-3\Gamma_1} + 8\pi P_e \chi </math>
	<math>~=</math>	<math>~\chi^{-2} \biggl[ 2W_\mathrm{grav} + 3(1-\Gamma_1)(2-3\Gamma_1) U_\mathrm{int}+ 6 P_e V \biggr] \, .</math>

The equilibrium condition occurs when <math>~\partial \mathfrak{G}/\partial \chi = 0</math>, that is, when,

<math>~3(1-\Gamma_1) U_\mathrm{int}</math>

<math>~W_\mathrm{grav} - 3 P_e V \, ,</math>

in which case,

<math>~\chi^2 \cdot \frac{\partial^2 \mathfrak{G}}{\partial \chi^2}</math>	<math>~=</math>	<math>~2W_\mathrm{grav} + (2-3\Gamma_1) (W_\mathrm{grav} - 3P_eV) + 6 P_e V </math>
	<math>~=</math>	<math>~(4-3\Gamma_1)W_\mathrm{grav} + 3^2 \Gamma_1 P_e V \, .</math>

Fantastic! The righthand-side of this "free-energy-based" expression exactly matches the righthand-side of the above expression that has been derived from the variational principle, assuming homologous expansion/contraction (i.e., <math>~\xi</math> = constant). In this case, we can make the direct association,

<math>~\sigma^2 I = \chi^2 \cdot \frac{\partial^2 \mathfrak{G}}{\partial \chi^2} \, .</math>

This also make sense in that the equilibrium configuration should be stable if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} > 0</math> — in which case, <math>~\sigma^2</math> is positive; whereas the equilibrium configuration should be unstable if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} < 0</math> — in which case, <math>~\sigma^2</math> is negative.

Related, Exploratory Ideas

Logarithmic Derivatives

Returning to our above discussion of the Ledoux & Walraven approach, we appreciate that the differential relation governing the Variational Principle is,

<math>~\sigma^2 \rho r^4 \xi^2</math>	<math>~=</math>	<math>~ r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2 - (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) - \frac{d}{dr}\biggr[r^4 \Gamma_1 P\xi \biggl(\frac{d\xi}{dr}\biggr) \biggr] </math>
<math>~\Rightarrow ~~~ \frac{d}{dr}\biggr[r^3 \Gamma_1 P\xi^2 \biggl(\frac{d\ln\xi}{d\ln r}\biggr) \biggr] </math>	<math>~=</math>	<math>~ r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2 - (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) - \sigma^2 \rho r^4 \xi^2 </math>
	<math>~=</math>	<math>~\xi^2 \biggl\{ r^2 \Gamma_1 P \biggl(\frac{d\ln\xi}{d\ln r}\biggr)^2 - (3\Gamma_1 - 4) r^3 \biggl( \frac{dP}{dr} \biggr) - \sigma^2 \rho r^4 \biggr\} </math>
	<math>~=</math>	<math>~(r \xi)^2 P \biggl\{ \Gamma_1 \biggl(\frac{d\ln\xi}{d\ln r}\biggr)^2 - (3\Gamma_1 - 4) \biggl( \frac{d\ln P}{d\ln r} \biggr) - \frac{\sigma^2 \rho r^2}{P} \biggr\} </math>
	<math>~=</math>	<math>~\Gamma_1 (r \xi)^2 P \biggl\{ \biggl(\frac{d\ln\xi}{d\ln r}\biggr)^2 - \alpha \biggl( \frac{d\ln P}{d\ln r} \biggr) - \frac{\sigma^2 \rho r^2}{\Gamma_1 P} \biggr\} \, , </math>

where,

<math>~\alpha \equiv \biggl(3 - \frac{4}{\Gamma_1}\biggr) \, .</math>

Pressure-Truncated Polytropes

Let's start with the integral expression derived in our discussion of the Ledoux & Walraven approach; insert the variable, <math>~x</math>, in place of <math>~\xi</math>; and adopt the boundary conditions,

<math>~r = 0</math> at the center,

along with

<math>~P = P_e~</math>, and <math>\frac{d\ln x}{d\ln r} = -3</math> at the surface (r = R).

That is, let's start with,

<math>~\int_0^R \sigma^2 \rho r^4 x^2 dr</math>

<math>~ \int_0^R r^4 \Gamma_1 P \biggl(\frac{dx}{dr}\biggr)^2 dr - \int_0^R (3\Gamma_1 - 4) r^3 x^2 \biggl( \frac{dP}{dr} \biggr) dr

+3\Gamma_1 P_e R^3 x_\mathrm{surface}^2 \, .

</math>

Next, we'll divide through by the normalization energy, as defined in an accompanying discussion,

<math>~E_\mathrm{norm} = P_\mathrm{norm}R_\mathrm{norm}^3 = \frac{GM_\mathrm{tot}^2}{R_\mathrm{norm}} \, ,</math>

thereby making the integral relation dimensionless:

<math>~ 0 </math>	<math>~=</math>	<math>~ - \biggl[\frac{R_\mathrm{norm}}{GM_\mathrm{tot}^2} \biggr] \int_0^R \sigma^2 \rho r^4 x^2 dr +\biggl[\frac{1}{P_\mathrm{norm}R_\mathrm{norm}^3} \biggr] \int_0^R r^4 \Gamma_1 P \biggl(\frac{dx}{dr}\biggr)^2 dr - \biggl[\frac{1}{P_\mathrm{norm}R_\mathrm{norm}^3} \biggr] \int_0^R (3\Gamma_1 - 4) r^3 x^2 \biggl( \frac{dP}{dr} \biggr) dr + \biggl[\frac{P_e R^3 }{P_\mathrm{norm}R_\mathrm{norm}^3} \biggr] 3\Gamma_1 x_\mathrm{surface}^2 </math>
	<math>~=</math>	<math>~ - \biggl[\frac{R_\mathrm{norm} R^5 \rho_c^2}{M_\mathrm{tot}^2} \biggr] \int_0^R x^2 \biggl( \frac{\sigma^2}{G\rho_c}\biggr) \biggl( \frac{\rho}{\rho_c} \biggr) \biggl(\frac{r}{R}\biggr)^4 \frac{dr}{R} + \biggl[\frac{P_c R^3}{P_\mathrm{norm}R_\mathrm{norm}^3 } \biggr] \int_0^R \biggl( \frac{r}{R}\biggr)^4 \Gamma_1\biggl(\frac{ P }{P_c} \biggr) \biggl[ \frac{dx}{d(r/R)}\biggr]^2 \frac{dr}{R} </math>
		<math>~ - \biggl[\frac{P_c R^3}{P_\mathrm{norm}R_\mathrm{norm}^3} \biggr] \int_0^R (3\Gamma_1 - 4) \biggl( \frac{r}{R} \biggr)^3 x^2 \biggl[ \frac{d(P/P_c)}{d(r/R)} \biggr] \frac{dr}{R} + \biggl[\frac{P_e R^3 }{P_\mathrm{norm}R_\mathrm{norm}^3} \biggr] 3\Gamma_1 x_\mathrm{surface}^2 </math>
	<math>~=</math>	<math>~ - \biggl[ \frac{M}{M_\mathrm{tot}}\biggr]^2 \biggl[\biggl( \frac{3}{4\pi} \biggr) \frac{\rho_c}{\bar\rho} \biggr]^2 \chi^{-1} \int_0^R x^2 \biggl( \frac{\sigma^2}{G\rho_c}\biggr) \biggl( \frac{\rho}{\rho_c} \biggr) \biggl(\frac{r}{R}\biggr)^4 \frac{dr}{R} + \biggl[\frac{P_e }{P_\mathrm{norm}} \biggr] 3\Gamma_1 \chi^3 x_\mathrm{surface}^2 </math>
		<math>~ + \biggl[\frac{P_c}{P_\mathrm{norm} } \biggr] \chi^3 \int_0^R \biggl\{ \biggl( \frac{r}{R}\biggr)^4 \Gamma_1\biggl(\frac{ P }{P_c} \biggr) \biggl[ \frac{dx}{d(r/R)}\biggr]^2 - (3\Gamma_1 - 4) \biggl( \frac{r}{R} \biggr)^3 x^2 \biggl[ \frac{d(P/P_c)}{d(r/R)} \biggr] \biggr\}\frac{dr}{R} \, , </math>

where,

<math>~\chi \equiv \frac{R}{R_\mathrm{norm}} \, .</math>

Note that we will ultimately insert the relation,

<math>~\frac{P_c}{P_\mathrm{norm}} = \biggl[\biggl( \frac{3}{4\pi}\biggr) \frac{\rho_c}{\bar\rho} \biggl( \frac{M}{M_\mathrm{tot}}\biggr)\biggr]^{\Gamma_1} \biggl( \frac{R}{R_\mathrm{norm}}\biggr)^{-3\Gamma_1} \, .</math>

But, for the time being, dividing through by <math>~[P_c/P_\mathrm{norm}]\chi^3</math> gives,

<math>~0</math>	<math>~=</math>	<math>~ - \biggl[\frac{P_c}{P_\mathrm{norm} } \biggr]^{-1} \biggl[ \frac{M}{M_\mathrm{tot}}\biggr]^2 \biggl[\biggl( \frac{3}{4\pi} \biggr) \frac{\rho_c}{\bar\rho} \biggr]^2 \chi^{-4} \int_0^R x^2 \biggl( \frac{\sigma^2}{G\rho_c}\biggr) \biggl( \frac{\rho}{\rho_c} \biggr) \biggl(\frac{r}{R}\biggr)^4 \frac{dr}{R} </math>
		<math>~ + \biggl[\frac{P_e }{P_c} \biggr] 3\Gamma_1 x_\mathrm{surface}^2 + \int_0^R \biggl\{ \biggl( \frac{r}{R}\biggr)^4 \Gamma_1\biggl(\frac{ P }{P_c} \biggr) \biggl[ \frac{dx}{d(r/R)}\biggr]^2 - (3\Gamma_1 - 4) \biggl( \frac{r}{R} \biggr)^3 x^2 \biggl[ \frac{d(P/P_c)}{d(r/R)} \biggr] \biggr\}\frac{dr}{R} \, , </math>

Now let's focus on the second line of this integral energy relation, evaluating it for pressure-truncated polytropic configurations, in which case, <math>~\Gamma_1 \rightarrow (n+1)/n</math>,

<math>~\frac{r}{R} \rightarrow \frac{\xi}{\tilde\xi}</math>

and

<math>~ \frac{P}{P_c} \rightarrow \theta^{n+1} \, . </math>

We have,

Second line of relation	<math>~=</math>	<math>~ \biggl[\frac{P_e }{P_c} \biggr] 3\Gamma_1 x_\mathrm{surface}^2 + \int_0^R \biggl\{ \biggl( \frac{r}{R}\biggr)^4 \Gamma_1\biggl(\frac{ P }{P_c} \biggr) \biggl[ \frac{dx}{d(r/R)}\biggr]^2 - (3\Gamma_1 - 4) \biggl( \frac{r}{R} \biggr)^3 x^2 \biggl[ \frac{d(P/P_c)}{d(r/R)} \biggr] \biggr\}\frac{dr}{R} </math>
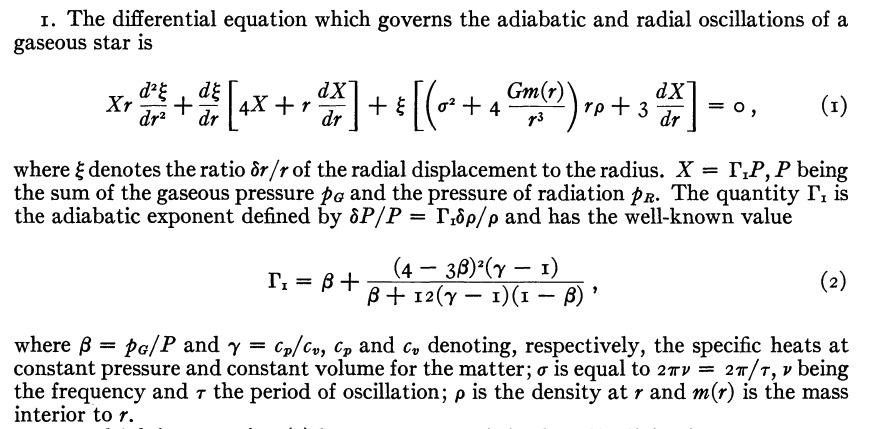
	<math>~=</math>	<math>~ \biggl[\frac{P_e }{P_c} \biggr] \biggl[ \frac{3( n+1)}{n} \biggr] x_\mathrm{surface}^2 + \int_0^R \biggl\{ \biggl( \frac{\xi}{\tilde\xi}\biggr)^4 \biggl(\frac{n+1}{n}\biggr) \theta^{n+1} \biggl[ \frac{dx}{d\xi}\biggr]^2 {\tilde\xi}^2 - \biggl(\frac{3-n}{n}\biggr) \biggl( \frac{\xi}{\tilde\xi} \biggr)^3 x^2 \biggl[ \frac{d\theta^{n+1}}{d\xi} \biggr] \tilde\xi \biggr\}\frac{d\xi}{\tilde\xi} </math>
	<math>~=</math>	<math>~ \biggl[\frac{P_e }{P_c} \biggr] \biggl[ \frac{3( n+1)}{n} \biggr] x_\mathrm{surface}^2 + \frac{1}{n {\tilde\xi}^3}\int_0^R \biggl\{ (n+1) \xi^4 \theta^{n+1} \biggl[ \frac{dx}{d\xi}\biggr]^2 - (3-n) \xi^3 x^2 \biggl[ \frac{d\theta^{n+1}}{d\xi} \biggr] \biggr\}d\xi \, . </math>

Now, let's examine how these terms combine if we guess the analytically defined eigenfunction that applies to marginally unstable, pressure-truncated polytropic configurations, namely,

<math>~x</math>	<math>~=</math>	<math>~\frac{3(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{1}{\xi \theta^{n}}\biggr) \frac{d\theta}{d\xi}\biggr] </math>
<math>~\Rightarrow ~~~ \frac{dx}{d\xi}</math>	<math>~=</math>	<math>~\biggl[\frac{3(n-3)}{2n}\biggr] \biggl\{ \biggl( \frac{1}{\xi \theta^{n}}\biggr) \theta^{} -\biggl( \frac{1}{\xi^2 \theta^{n}}\biggr) \theta^' - \frac{n(\theta^')^2}{\xi \theta^{(n+1)}} \biggr\} </math>

Difference between revisions of "User:Tohline/SSC/VariationalPrinciple"