Difference between revisions of "User:Tohline/SSC/Stability/BiPolytrope0 0"
(→Piecing Together: Insert 2 panels for Figure 1) |
|||
| Line 428: | Line 428: | ||
Here we illustrate how the two segments of the eigenfunction can be successfully pieced together for the specific case of <math>~(\ell,j) = (2,1)</math>. | Here we illustrate how the two segments of the eigenfunction can be successfully pieced together for the specific case of <math>~(\ell,j) = (2,1)</math>. | ||
STEP 1: Choose a value of the adiabatic exponent for the envelope, <math>~\gamma_e</math>. Then, the values of both <math>~\alpha_e</math> and <math>~c_0</math> are known as well; actually, because it is the root of a quadratic equation, <math>~c_0</math> can, in general, take on one of a ''pair'' of values. We will elaborate on this further, below. | <font color="red"><b>STEP 1:</b></font> Choose a value of the adiabatic exponent for the envelope, <math>~\gamma_e</math>. Then, the values of both <math>~\alpha_e</math> and <math>~c_0</math> are known as well; actually, because it is the root of a quadratic equation, <math>~c_0</math> can, in general, take on one of a ''pair'' of values. We will elaborate on this further, below. | ||
STEP 2: Acknowledging that the value of <math>~q</math> has yet to be determined, fix the value of the leading, overall scaling coefficient, <math>~b_0</math>, such that <math>~x_\mathrm{env} = 1</math> at the interface, that is, at <math>~\xi = 1</math>. For the case of <math>~\ell=2</math>, this means that, throughout the envelope, the eigenfunction is, | <font color="red"><b>STEP 2:</b></font> Acknowledging that the value of <math>~q</math> has yet to be determined, fix the value of the leading, overall scaling coefficient, <math>~b_0</math>, such that <math>~x_\mathrm{env} = 1</math> at the interface, that is, at <math>~\xi = 1</math>. For the case of <math>~\ell=2</math>, this means that, throughout the envelope, the eigenfunction is, | ||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 479: | Line 479: | ||
are also both known. | are also both known. | ||
STEP 3: Recognizing that this segment of the eigenfunction will only satisfy the envelope's LAWE if we restrict our discussion to equilibrium models for which <math>~g^2 = \mathcal{B} = (1+2q^3)^{-1}</math>, we must insert this same restriction on <math>~g^2</math> into the core's eigenfunction. At the same time, we should fix the value of the leading, overall scaling coefficient, <math>~a_0</math>, such that <math>~x_\mathrm{core} = 1</math> at the interface <math>~(\xi = 1)</math>. For the case of <math>~j=1</math>, this means that, throughout the core, the eigenfunction is, | <font color="red"><b>STEP 3:</b></font> Recognizing that this segment of the eigenfunction will only satisfy the envelope's LAWE if we restrict our discussion to equilibrium models for which <math>~g^2 = \mathcal{B} = (1+2q^3)^{-1}</math>, we must insert this same restriction on <math>~g^2</math> into the core's eigenfunction. At the same time, we should fix the value of the leading, overall scaling coefficient, <math>~a_0</math>, such that <math>~x_\mathrm{core} = 1</math> at the interface <math>~(\xi = 1)</math>. For the case of <math>~j=1</math>, this means that, throughout the core, the eigenfunction is, | ||
<div align="center"> | <div align="center"> | ||
| Line 499: | Line 499: | ||
</div> | </div> | ||
STEP 4: Now we need to ensure that the first derivative with respect to the radial coordinate, <math>~\xi</math>, of both eigenfunction segments match at the interface <math>~(\xi = 1)</math>. The mathematical relation that accomplishes this is, | <font color="red"><b>STEP 4:</b></font> Now we need to ensure that the first derivative with respect to the radial coordinate, <math>~\xi</math>, of both eigenfunction segments match at the interface <math>~(\xi = 1)</math>. The mathematical relation that accomplishes this is, | ||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 612: | Line 612: | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
<font color="red"><b>STEP 5:</b></font> Finally, for each choice of <math>~\gamma_e</math>, the physically relevant value of the core's adiabatic exponent is set by demanding that the ''dimensional'' eigenfrequencies of the envelope and core precisely match. That is, we demand that, | |||
<div align="center"> | |||
<math>~\omega^2_\mathrm{env} = \omega^2_\mathrm{core} \, .</math> | |||
</div> | |||
From [[#Eigenvector|above]], we know that, for the core, | |||
<div align="center"> | |||
<math>~3\omega^2_\mathrm{core}\biggr|_\mathrm{j=1} = 2\pi \gamma_c G \rho_c [ 20 - 3/\gamma_c] \, ;</math> | |||
</div> | |||
whereas, for the envelope, | |||
<div align="center"> | |||
<math>~3\omega^2_\mathrm{env}\biggr|_\mathrm{\ell=2} = 2\pi \gamma_e G \rho_e [ 3(\alpha_e + 5c_0 + 22)] \, .</math> | |||
</div> | |||
By demanding that these frequencies be identical, we conclude that, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ 20\gamma_c - 3</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~3\gamma_e \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(\alpha_e + 5c_0 + 22 \biggr)</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \Rightarrow ~~~ \gamma_c </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{3}{20}\biggl\{ 1 + \biggl(\frac{4}{3-\alpha_e}\biggr) \biggl[\alpha_e + 5(\sqrt{1+\alpha_e}-1) + 22 \biggr]\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr\}\, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Hence, the eigenfrequency that corresponds to the ''specific'' eigenfunction that is displayed in the right-hand panel of Figure 1, we have, | |||
<div align="center"> | |||
<math>~\gamma_c = 2.004437 \, .</math> | |||
</div> | </div> | ||
Revision as of 20:32, 17 December 2016
Radial Oscillations of a Zero-Zero Bipolytrope
This is a chapter that summarizes an accompanying, detailed derivation.

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
In a separate chapter on astrophysical interesting equilibrium structures, we have derived analytical expressions that define the equilibrium properties of bipolytropic configurations having <math>~(n_c, n_e) = (0, 0)</math>, that is, bipolytropes in which both the core and the envelope are uniform in density, but the densities in the two regions are different from one another. Letting <math>~R</math> be the radius and <math>~M_\mathrm{tot}</math> be the total mass of the bipolytrope, these configurations are fully defined once any two of the following three key parameters have been specified: The envelope-to-core density ratio, <math>~\rho_e/\rho_c</math>; the radial location of the envelope/core interface, <math>~q \equiv r_i/R</math>; and, the fractional mass that is contained within the core, <math>~\nu \equiv M_\mathrm{core}/M_\mathrm{tot}</math>. These three parameters are related to one another via the expression,
|
<math>~\frac{\rho_e}{\rho_c}</math> |
<math>~=</math> |
<math>~\frac{q^3}{\nu} \biggl( \frac{1-\nu}{1-q^3} \biggr) \, .</math> |
Equilibrium configurations can be constructed that have a wide range of parameter values; specifically,
<math>~0 \le q \le 1 \, ;</math> <math>~0 \le \nu \le 1 \, ;</math> and, <math>~0 \le \frac{\rho_e}{\rho_c} \le 1 \, .</math>
(We recognize from buoyancy arguments that any configuration in which the envelope density is larger than the core density will be Rayleigh-Taylor unstable, so we restrict our astrophysical discussion to structures for which <math>~\rho_e < \rho_c</math>.)
By employing the linear stability analysis techniques described in an accompanying chapter, we should, in principle, be able to identify a wide range of eigenvectors — that is, radial eigenfunctions and accompanying eigenfrequencies — that are associated with adiabatic radial oscillation modes in any one of these equilibrium, bipolytropic configurations. Using numerical techniques, Murphy & Fiedler (1985), for example, have carried out such an analysis of bipolytropic structures having <math>~(n_c, n_e) = (1,5)</math>. A pair of linear adiabatic wave equations (LAWEs) must be solved — one tuned to accommodate the properties of the core and another tuned to accommodate the properties of the envelope — then the pair of eigenfunctions must be matched smoothly at the radial location of the interface; the identified core- and envelope-eigenfrequencies must simultaneously match.
After identifying the precise form of the LAWEs that apply to the case of <math>~(n_c, n_e) = (0,0)</math> bipolytropes, we discovered that, for a restricted range of key parameters, the pair of equations can both be solved analytically.
Two Separate LAWEs
In an accompanying discussion, we derived the so-called,
Adiabatic Wave (or Radial Pulsation) Equation
|
<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math> |
For both regions of the bipolytrope, we define the dimensionless (Lagrangian) radial coordinate,
<math>~\xi \equiv \frac{r_0}{r_i} \, .</math>
So, the interface is, by definition, located at <math>~\xi = 1</math>; and, the surface is necessarily at <math>~\xi = q^{-1}</math>. As the material in the bipolytrope's core (envelope) is compressed/de-compressed during a radial oscillation, we will assume that heating/cooling occurs in a manner prescribed by an adiabat of index <math>~\gamma_c ~(\gamma_e)</math>; in general, <math>~\gamma_e \ne \gamma_c</math>. For convenience, we will also adopt the frequently used shorthand "alpha" notation,
<math>~\alpha_c \equiv 3 - \frac{4}{\gamma_c} \, ,</math> and <math>~\alpha_e \equiv 3 - \frac{4}{\gamma_e} \, .</math>
The Core's LAWE
After adopting, for convenience, the function notation,
|
<math>~g^2</math> |
<math>~\equiv</math> |
<math> 1 + \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \biggl( 1-q \biggr) + \frac{\rho_e}{\rho_c} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] \, , </math> |
we have deduced that, for the core, the LAWE may be written in the form,
|
<math>~0</math> |
<math>~=</math> |
<math>~ (1 - \eta^2)\frac{d^2x}{d\eta^2} + ( 4 - 6\eta^2 ) \frac{1}{\eta} \cdot \frac{dx}{d\eta} + \mathfrak{F}_\mathrm{core} x \, . </math> |
where,
<math>~\eta \equiv \frac{\xi}{g} \, ,</math> and <math>~\mathfrak{F}_\mathrm{core} \equiv \frac{3\omega_\mathrm{core}^2}{2\pi G\gamma_c \rho_c} - 2\alpha_c\, .</math>
Not surprisingly, this is identical in form to the eigenvalue problem that was first presented — and solved analytically — by Sterne (1937) in connection with his examination of radial oscillations in isolated uniform-density spheres. As is demonstrated below, for the core of our zero-zero bipolytrope, we can in principle adopt any one of the polynomial eigenfunctions and corresponding eigenfrequencies derived by Sterne.
The Envelope's LAWE
Subsequently, we also have deduced that, for the envelope, the governing LAWE becomes,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \biggl[ 1 + \frac{(g^2-\mathcal{B}) \xi}{\mathcal{A}} - \mathcal{D} \xi^3\biggr] \frac{d^2x}{d\xi^2} + \biggl\{ 3 + \frac{4(g^2-\mathcal{B}) \xi}{\mathcal{A}} - 6\mathcal{D} \xi^3 \biggr\} \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ \mathcal{D} \biggl(\frac{\rho_c}{\rho_e}\biggr) \biggl( \mathfrak{F}_\mathrm{env} + 2\alpha_e -2\alpha_e\frac{\rho_e}{\rho_c} \biggr)\xi^3 -\alpha_e \biggr]\frac{x}{\xi^2} \, , </math> |
where,
|
<math>~\mathcal{A}</math> |
<math>~\equiv</math> |
<math>~2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \, ; </math> |
|
<math>~\mathcal{B}</math> |
<math>~\equiv</math> |
<math>~1 + 2\biggl(\frac{\rho_e}{\rho_c}\biggr) - 3\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 \, , </math> |
|
<math>~\mathcal{D}</math> |
<math>~\equiv</math> |
<math>~\frac{1}{\mathcal{A}}\biggl( \frac{\rho_e}{\rho_c}\biggr)^2 = \biggl[ \frac{\rho_e/\rho_c}{2(1-\rho_e/\rho_c)} \biggr] \, , </math> |
|
<math>~\mathfrak{F}_\mathrm{env}</math> |
<math>~\equiv</math> |
<math>~\frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} - 2\alpha_e \, . </math> |
We have been unable to demonstrate that this governing equation can be solved analytically for arbitrary pairs of the key model parameters, <math>~q</math> and <math>~\rho_e/\rho_c</math>. But, if we choose parameter value pairs that satisfy the constraint,
<math>~g^2 = \mathcal{B} </math> <math>~\Rightarrow</math> <math>~g = \frac{1}{1+2q^3} \, ,</math> and, <math>~q^3 = \mathcal{D} = \biggl[ \frac{\rho_e/\rho_c}{2(1-\rho_e/\rho_c)} \biggr] \, ,</math>
then the LAWE that is relevant to the envelope simplifies. Specifically, it takes the form,
|
<math>~0</math> |
<math>~=</math> |
<math>~ ( 1 - q^3 \xi^3 ) \frac{d^2x}{d\xi^2} + ( 3 - 6q^3 \xi^3 ) \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]\frac{x}{\xi^2} </math> |
|
|
<math>~=</math> |
<math>~\frac{x}{\xi^2}\biggl\{ ( 1 - q^3 \xi^3 ) \biggl[ \frac{d}{d\ln\xi} \biggl( \frac{d\ln x}{d\ln \xi} \biggr) - \biggl( 1 - \frac{d\ln x}{d\ln \xi} \biggr)\cdot \frac{d\ln x}{d\ln \xi}\biggr] + ( 3 - 6q^3 \xi^3 ) \frac{d\ln x}{d\ln \xi} + \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr] \biggr\} \, . </math> |
Shortly after deriving this last expression, we realized that one possible solution is a simple power-law eigenfunction of the form,
<math>~x=a_0 \xi^{c_0} \, ,</math>
where the (constant) exponent is one of the roots of the quadratic equation,
<math>~c_0^2 + 2c_0 - \alpha_e = 0 \, ,</math> <math>~\Rightarrow</math> <math>~c_0 = -1 \pm \sqrt{1+\alpha_e} \, .</math>
This power-law eigenfunction must be paired with the associated, dimensionless eigenfrequency parameter,
|
<math>~\mathfrak{F}_\mathrm{env}</math> |
<math>~=</math> |
<math>~c_0(c_0+5) = 3c_0 + \alpha_e</math> |
|
<math>~\Rightarrow ~~~ \frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} </math> |
<math>~=</math> |
<math>~ 3(c_0 + \alpha_e) = 3[\alpha_e -1 \pm \sqrt{1+\alpha_e}] \, .</math> |
Next, we noticed the strong similarities between the mathematical properties of this eigenvalue problem and the one that was studied by C. Prasad (1948, MNRAS, 108, 414-416) in connection with, what we now recognize to be, a closely related problem. Drawing heavily from Prasad's analysis, we discovered an infinite number of eigenfunctions (each, a truncated polynomial expression) and associated eigenfrequencies that satisfy this governing envelope LAWE. The eigenvectors associated with the lowest few modes are tabulated, below.
Eigenvector
Core Segment
|
Mode |
Core Eigenfunction |
Core Eigenfrequency <math>~\frac{3\omega_\mathrm{core}^2}{2\pi \gamma_c G \rho_c} = 2[\alpha_c + j(2j+5)]</math> |
|
<math>~j=0 </math> |
<math>~x_\mathrm{core} = a_0 </math> |
<math>~6-8/\gamma_c</math> |
|
<math>~j=1 </math> |
<math>~x_\mathrm{core} = a_0 \biggl[ 1 - \frac{7}{5}\biggr(\frac{\xi^2}{g^2}\biggr) \biggr]</math> |
<math>~20-8/\gamma_c</math> |
|
<math>~j=2 </math> |
<math>~x_\mathrm{core} = a_0 \biggl[ 1 - \frac{18}{5}\biggr(\frac{\xi^2}{g^2}\biggr) + \frac{99}{35}\biggr(\frac{\xi^2}{g^2}\biggr)^2 \biggr]</math> |
<math>~42-8/\gamma_c</math> |
Envelope Segment
|
Mode |
Envelope Eigenfunction |
Envelope Eigenfrequency <math>~\frac{3\omega_\mathrm{env}^2}{2\pi \gamma_e G \rho_e} = 3[\alpha_e + c_0(2\ell+1) + \ell(3\ell+5)]</math> |
|
<math>~\ell=0 </math> |
<math>~x_\mathrm{env} = b_0 \xi^{c_0}</math> |
<math>~3[\alpha_e + c_0]</math> |
|
<math>~\ell=1 </math> |
<math>~x_\mathrm{env} = b_0 \xi^{c_0}\biggl\{1 + \biggl[ \frac{c_0(c_0+5)-(c_0+3)(c_0+8)}{(c_0+3)(c_0+5) - \alpha_e} \biggr](q\xi)^3 \biggr\}</math> |
<math>~3[\alpha_e + 3c_0 +8]</math> |
|
<math>~\ell=2 </math> |
<math>~x_\mathrm{env} = b_0 \xi^{c_0}\biggl\{1 + \biggl[ \frac{c_0(c_0+5)-(c_0+6)(c_0+11)}{(c_0+3)(c_0+5) - \alpha_e}\biggr](q\xi)^3 + \biggl[ \frac{c_0(c_0+5)-(c_0+6)(c_0+11)}{(c_0+3)(c_0+5) - \alpha_e}\biggr]\biggl[ \frac{(c_0+3)(c_0+8)-(c_0+6)(c_0+11)}{(c_0+6)(c_0+8) - \alpha_e}\biggr](q\xi)^6 \biggr\} </math> |
<math>~3[\alpha_e + 5c_0 +22]</math> |
Piecing Together
Here we illustrate how the two segments of the eigenfunction can be successfully pieced together for the specific case of <math>~(\ell,j) = (2,1)</math>.
STEP 1: Choose a value of the adiabatic exponent for the envelope, <math>~\gamma_e</math>. Then, the values of both <math>~\alpha_e</math> and <math>~c_0</math> are known as well; actually, because it is the root of a quadratic equation, <math>~c_0</math> can, in general, take on one of a pair of values. We will elaborate on this further, below.
STEP 2: Acknowledging that the value of <math>~q</math> has yet to be determined, fix the value of the leading, overall scaling coefficient, <math>~b_0</math>, such that <math>~x_\mathrm{env} = 1</math> at the interface, that is, at <math>~\xi = 1</math>. For the case of <math>~\ell=2</math>, this means that, throughout the envelope, the eigenfunction is,
|
<math>~x_{\ell=2} |_\mathrm{env}</math> |
<math>~=</math> |
<math>~ \xi^{c_0}\biggl[ \frac{ 1 + q^3 A_{21} \xi^{3} + q^6 A_{21}B_{21}\xi^{6} }{ 1 + q^3 A_{21} + q^6 A_{21}B_{21}}\biggr] \, , </math> |
where, the values of the newly introduced coefficients,
|
<math>~A_{21}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \frac{c_0(c_0+5) - (c_0 + 6)(c_0 + 11)}{(c_0 + 3)(c_0+5) - \alpha_e}\biggr] \, ,</math> |
|
<math>~B_{21}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \frac{(c_0+3)(c_0+8) - (c_0 + 6)(c_0 + 11)}{(c_0 + 6)(c_0+8) - \alpha_e}\biggr] \, ,</math> |
are also both known.
STEP 3: Recognizing that this segment of the eigenfunction will only satisfy the envelope's LAWE if we restrict our discussion to equilibrium models for which <math>~g^2 = \mathcal{B} = (1+2q^3)^{-1}</math>, we must insert this same restriction on <math>~g^2</math> into the core's eigenfunction. At the same time, we should fix the value of the leading, overall scaling coefficient, <math>~a_0</math>, such that <math>~x_\mathrm{core} = 1</math> at the interface <math>~(\xi = 1)</math>. For the case of <math>~j=1</math>, this means that, throughout the core, the eigenfunction is,
|
<math>~x_{j=1} |_\mathrm{core}</math> |
<math>~=</math> |
<math>~ \frac{5 - 7 (1+2q^3)^2 \xi^2}{5-7(1+2q^3)^2} \, .</math> |
STEP 4: Now we need to ensure that the first derivative with respect to the radial coordinate, <math>~\xi</math>, of both eigenfunction segments match at the interface <math>~(\xi = 1)</math>. The mathematical relation that accomplishes this is,
|
<math>~\frac{14(1+2q^3)^2}{7(1+2q^3)^2 - 5}</math> |
<math>~=</math> |
<math>~ \frac{c_0 + (c_0 + 3)A_{21}q^3 + (c_0 + 6)A_{21}B_{21} q^6}{1 + A_{21}q^3 + A_{21}B_{21}q^6} \, , </math> |
or, making the notation substitution, <math>~\Chi \equiv q^3</math>, we have equivalently,
|
<math>~a\Chi^4 + b\Chi^3 + c\Chi^2 +d\Chi +e </math> |
<math>~=</math> |
<math>~0 \, ,</math> |
where,
|
<math>~e</math> |
<math>~\equiv</math> |
<math>~ 14- 2c_0 \, ,</math> |
|
<math>~d</math> |
<math>~\equiv</math> |
<math>~28(2-c_0) + (8-2c_0) A_{21} \, ,</math> |
|
<math>~c</math> |
<math>~\equiv</math> |
<math>~28(2-c_0) - 28(1+c_0) A_{21} + 2(1 - c_0)A_{21}B_{21} \, ,</math> |
|
<math>~b</math> |
<math>~\equiv</math> |
<math>~-28A_{21}[(1+c_0) + (4+c_0)B_{21} ] \, ,</math> |
|
<math>~a</math> |
<math>~\equiv</math> |
<math>~- 28 (4+c_0)A_{21}\cdot B_{21} \, .</math> |
The physically relevant (real) root of this quartic equation in <math>~\Chi</math> — see our accompanying detailed presentation — gives us the specific value of the dimensionless interface location, <math>~q</math>, for which both the values and the first derivatives of the two eigenfunction segments match at the interface. Generally, we expect to find different values of <math>~q</math> for each choice (see STEP 1) of <math>~\gamma_e</math>.
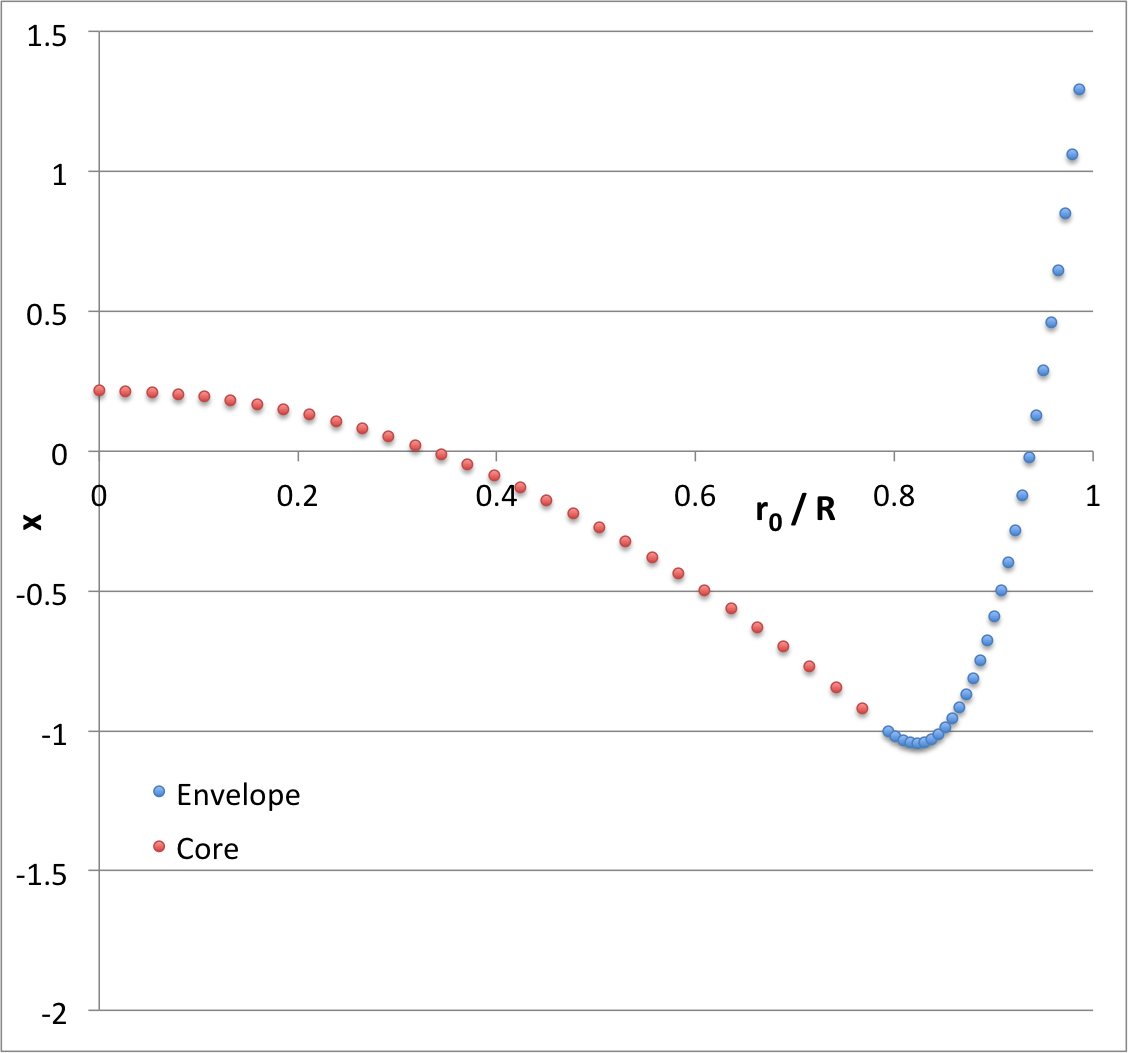
The dark blue curve in the left-hand panel of Figue 1 shows how <math>~q</math> varies with the choice of the envelope's adiabatic exponent over the entire range of physically relevant values, <math>~-1 \le \alpha_e \le 3</math>, if we adopt the "plus" quadratic root for <math>~c_0</math>, that is, <math>~c_0 = [ \sqrt{1+\alpha_e} - 1]</math>. In this plot we have purposely flipped the horizontal axis so that the extreme left <math>~(\alpha_e = +3)</math> represents an incompressible <math>~(n = 0)</math> envelope, while the extreme right represents an isothermal <math>~(\gamma_e = 1)</math> envelope. The green and orange curves, respectively, show as well how the corresponding model parameters, <math>~\nu</math> and <math>~\rho_e/\rho_c</math>, vary with <math>~\alpha_e</math>. The right-hand panel displays one example of an <math>~(\ell, j) = (2,1)</math> eigenfunction that simultaneously satisfies the LAWE of the core and the LAWE of the envelope, and matches smoothly at the interface. This particular plotted solution corresponds to the case of <math>~\alpha_e = -0.35</math>, for which: <math>~\gamma_e =1.1940299</math>; <math>~n_e = 5.1538462</math>; <math>~q = 0.7943853</math>; <math>~\nu =0.6675302</math>; and <math>~\rho_e/\rho_c =0.5006468</math>.
STEP 5: Finally, for each choice of <math>~\gamma_e</math>, the physically relevant value of the core's adiabatic exponent is set by demanding that the dimensional eigenfrequencies of the envelope and core precisely match. That is, we demand that,
<math>~\omega^2_\mathrm{env} = \omega^2_\mathrm{core} \, .</math>
From above, we know that, for the core,
<math>~3\omega^2_\mathrm{core}\biggr|_\mathrm{j=1} = 2\pi \gamma_c G \rho_c [ 20 - 3/\gamma_c] \, ;</math>
whereas, for the envelope,
<math>~3\omega^2_\mathrm{env}\biggr|_\mathrm{\ell=2} = 2\pi \gamma_e G \rho_e [ 3(\alpha_e + 5c_0 + 22)] \, .</math>
By demanding that these frequencies be identical, we conclude that,
|
<math>~ 20\gamma_c - 3</math> |
<math>~=</math> |
<math>~3\gamma_e \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(\alpha_e + 5c_0 + 22 \biggr)</math> |
|
<math>~ \Rightarrow ~~~ \gamma_c </math> |
<math>~=</math> |
<math>~\frac{3}{20}\biggl\{ 1 + \biggl(\frac{4}{3-\alpha_e}\biggr) \biggl[\alpha_e + 5(\sqrt{1+\alpha_e}-1) + 22 \biggr]\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr\}\, .</math> |
Hence, the eigenfrequency that corresponds to the specific eigenfunction that is displayed in the right-hand panel of Figure 1, we have,
<math>~\gamma_c = 2.004437 \, .</math>
Related Discussions

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |