Difference between revisions of "User:Tohline/Appendix/Ramblings/ConcentricEllipsodalT8Coordinates"
| (74 intermediate revisions by the same user not shown) | |||
| Line 1,440: | Line 1,440: | ||
<math>~ | <math>~ | ||
\frac{1}{2^{3 / 2}\kappa_2^2} \biggl\{ \biggl[ 1 + 4\kappa_2^4(\lambda_1^2 - p^2z_0^2 ) \biggr]^{1 / 2} - 1 \biggr\} \, . | \frac{1}{2^{3 / 2}\kappa_2^2} \biggl\{ \biggl[ 1 + 4\kappa_2^4(\lambda_1^2 - p^2z_0^2 ) \biggr]^{1 / 2} - 1 \biggr\} \, . | ||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
=Angle Between Unit Vectors= | |||
We begin by restating that the coordinate, scale factor, and unit vector associated with the normal to our ellipsoidal surface are, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\lambda_1</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(x^2 + q^2y^2 + p^2z^2)^{1 / 2} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~h_1</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\lambda_1 \ell_{3D} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\hat{e}_1</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\hat\imath (x\ell_{3D} ) | |||
+ | |||
\hat\jmath (q^2 y\ell_{3D} ) | |||
+ | |||
\hat{k} (p^2z\ell_{3D} ) \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
In the [[#TableKappa8|Table below titled, "Direction Cosines Components for κ8 Coordinates"]], there are two fully-formed unit vectors that are each orthogonal to the (first) unit vector that is normal to the ellipsoid's surface. Here we will refer to the coordinates of these two fully-formed unit vectors as, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\lambda_2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{xy^{1/q^2}}{z^{2/p^2}}</math> | |||
</td> | |||
<td align="center"> and, </td> | |||
<td align="right"> | |||
<math>~\kappa_2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{(qy)^{1/q^2}}{x} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
The associated scale factors and unit vectors are given by the following expressions: | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~h_{\lambda_2}^{-2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl(\frac{\partial\lambda_2}{\partial x} \biggr)^2 | |||
+ | |||
\biggl(\frac{\partial\lambda_2}{\partial y} \biggr)^2 | |||
+ | |||
\biggl(\frac{\partial\lambda_2}{\partial z} \biggr)^2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl(\frac{\lambda_2}{x} \biggr)^2 | |||
+ | |||
\biggl(\frac{\lambda_2}{q^2y} \biggr)^2 | |||
+ | |||
\biggl(- \frac{2\lambda_2}{p^2z} \biggr)^2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \lambda_2^2 \biggl[ | |||
\frac{q^4y^2p^4z^2 + x^2p^4z^2 + 4x^2 q^4y^2}{x^2 q^4y^2 p^4z^2} | |||
\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ h_{\lambda_2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{x q^2y p^2z}{\lambda_2 \mathcal{D}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ \hat{e}_{\lambda_2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\hat\imath \biggl[ h_{\lambda_2} \biggl(\frac{\partial \lambda_2}{\partial x}\biggr)\biggr] | |||
+ | |||
\hat\jmath \biggl[ h_{\lambda_2} \biggl(\frac{\partial \lambda_2}{\partial y}\biggr)\biggr] | |||
+ | |||
\hat{k} \biggl[ h_{\lambda_2} \biggl(\frac{\partial \lambda_2}{\partial z}\biggr)\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\hat\imath \biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] | |||
+ | |||
\hat\jmath \biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] | |||
- | |||
\hat{k} \biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="1" width="80%" align="center" cellpadding="8"><tr><td align="left"> | |||
With regard to orthogonality, note that, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\hat{e}_1 \cdot \hat{e}_{\lambda_2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ | |||
\hat\imath (x\ell_{3D} ) | |||
+ | |||
\hat\jmath (q^2 y\ell_{3D} ) | |||
+ | |||
\hat{k} (p^2z\ell_{3D} ) | |||
\biggr] \cdot | |||
\biggl\{ | |||
\hat\imath \biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] | |||
+ | |||
\hat\jmath \biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] | |||
- | |||
\hat{k} \biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
(x\ell_{3D} )\biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] | |||
+ | |||
(q^2 y\ell_{3D} )\biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] | |||
- | |||
(p^2z\ell_{3D} )\biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td></tr></table> | |||
And, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~h_{\kappa_2}^{-2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl(\frac{\partial\kappa_2}{\partial x} \biggr)^2 | |||
+ | |||
\biggl(\frac{\partial\kappa_2}{\partial y} \biggr)^2 | |||
+ | |||
\biggl(\frac{\partial\kappa_2}{\partial z} \biggr)^2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl(-\frac{\kappa_2}{x} \biggr)^2 | |||
+ | |||
\biggl(\frac{\kappa_2}{q^2 y} \biggr)^2 | |||
= | |||
\frac{\kappa_2^2}{x^2q^4y^2}\biggl[x^2 + q^4y^2\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~h_{\kappa_2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{xq^2y}{\kappa_2 (x^2 + q^4y^2)^{1 / 2}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~\hat{e}_{\kappa_2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-~\hat\imath \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}}\biggr] | |||
+ | |||
\hat\jmath \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
+ | |||
\hat{k} \biggl[ 0 \biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="1" width="80%" align="center" cellpadding="8"><tr><td align="left"> | |||
Again, note that with regard to orthogonality, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\hat{e}_1 \cdot \hat{e}_{\kappa_2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ | |||
\hat\imath (x\ell_{3D} ) | |||
+ | |||
\hat\jmath (q^2 y\ell_{3D} ) | |||
+ | |||
\hat{k} (p^2z\ell_{3D} ) | |||
\biggr] \cdot | |||
\biggl\{ | |||
-\hat\imath \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}}\biggr] | |||
+ | |||
\hat\jmath \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-~(x\ell_{3D} )\biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
+ | |||
(q^2 y\ell_{3D} )\biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td></tr></table> | |||
From this pair of orthogonality checks, we appreciate that both unit vectors always lie in the plane that is tangent to the surface of our ellipsoid. Next, let's determine the angle, <math>~\alpha</math>, between these two unit vectors as measured in the relevant tangent-plane. | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\cos\alpha \equiv \hat{e}_{\lambda_2} \cdot \hat{e}_{\kappa_2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl\{ | |||
\hat\imath \biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] | |||
+ | |||
\hat\jmath \biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] | |||
- | |||
\hat{k} \biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] | |||
\biggr\} | |||
\biggl\{ | |||
-\hat\imath \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}}\biggr] | |||
+ | |||
\hat\jmath \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
+ | |||
\hat{k} \biggl[ 0 \biggr] | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}}\biggr]\biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] | |||
+ | |||
\biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr]\biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{p^2 z}{\mathcal{D} (x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ x^2 - q^4y^2 \biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
---- | |||
Let's again visit the unit vector that we know lies in the tangent-plane and is always orthogonal to <math>~\lambda_2</math>, namely, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\hat{e}_{\lambda_3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-~\hat\imath \biggl[ x(2q^4 y^2 + p^4z^2 )\biggr]\frac{\ell_{3D}}{\mathcal{D}} | |||
+ | |||
\hat\jmath \biggl[q^2y(p^4z^2 + 2x^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} | |||
+ | |||
\hat{k} \biggl[p^2z (x^2 - q^4y^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} | |||
\, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
We acknowledge that, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\hat{e}_{\lambda_2} \cdot \hat{e}_{\lambda_3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl\{ | |||
\hat\imath \biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] | |||
+ | |||
\hat\jmath \biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] | |||
- | |||
\hat{k} \biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] | |||
\biggr\} | |||
\biggl\{ | |||
-~\hat\imath \biggl[ x(2q^4 y^2 + p^4z^2 )\biggr]\frac{\ell_{3D}}{\mathcal{D}} | |||
+ | |||
\hat\jmath \biggl[q^2y(p^4z^2 + 2x^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} | |||
+ | |||
\hat{k} \biggl[p^2z (x^2 - q^4y^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-~\biggl[ xq^2yp^2z(2q^4 y^2 + p^4z^2 )\biggr]\frac{\ell_{3D}}{\mathcal{D}^2} | |||
+ | |||
\biggl[x q^2y p^2z(p^4z^2 + 2x^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}^2} | |||
- | |||
\biggl[2xq^2y p^2z (x^2 - q^4y^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}^2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ | |||
-(2q^4 y^2 + p^4z^2 ) | |||
+ | |||
(p^4z^2 + 2x^2) | |||
- | |||
2 (x^2 - q^4y^2) | |||
\biggr] \frac{(x q^2y p^2z) \ell_{3D}}{\mathcal{D}^2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0 \, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,621: | Line 2,175: | ||
</table> | </table> | ||
<span id="TableKappa8"> </span> | |||
<table border="1" cellpadding="8" align="center"> | <table border="1" cellpadding="8" align="center"> | ||
<tr> | <tr> | ||
| Line 1,957: | Line 2,513: | ||
</tr> | </tr> | ||
</table> | </table> | ||
=T9 Coordinates= | |||
==Establish 1<sup>st</sup> and 3<sup>rd</sup> Coordinates== | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\lambda_1</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(x^2 + q^2y^2 + p^2z^2)^{1 / 2} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\lambda_3</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{q^2y}{x^{q^2}} \biggr]^{1/(q^2-1)} | |||
= | |||
q^{2/(q^2-1)} y^{1/(q^2-1)} x^{ q^2/(1-q^2) } | |||
\, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\partial \lambda_3}{\partial x}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{q^2}{1-q^2} \biggr) \frac{\lambda_3}{x} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\partial \lambda_3}{\partial y}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{1}{q^2 - 1} \biggr) \frac{\lambda_3}{y} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~h_3^{-2}</math> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \biggl( \frac{q^2}{1 - q^2 } \biggr) \frac{\lambda_3}{x} \biggr]^2 | |||
+ | |||
\biggl[ \biggl( \frac{1}{q^2 - 1} \biggr) \frac{\lambda_3}{y} \biggr]^2 | |||
= | |||
\frac{\lambda_3^2}{(q^2-1)^2}\biggl[ \frac{x^2 + q^4y^2 }{x^2 y^2} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ h_3</math> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(q^2-1)}{\lambda_3}\biggl[ \frac{x y}{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Hence, the three direction-cosines are, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{31} = h_3 \biggl(\frac{\partial \lambda_3}{\partial x} \biggr)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(q^2-1)}{\lambda_3}\biggl[ \frac{x y}{(x^2 + q^4y^2)^{1 / 2} } \biggr]\biggl( \frac{q^2}{1-q^2} \biggr) \frac{\lambda_3}{x} | |||
= | |||
- \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{32} = h_3 \biggl(\frac{\partial \lambda_3}{\partial y} \biggr)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(q^2-1)}{\lambda_3}\biggl[ \frac{x y}{(x^2 + q^4y^2)^{1 / 2} } \biggr]\biggl( \frac{1}{q^2 - 1} \biggr) \frac{\lambda_3}{y} | |||
= | |||
\biggl[ \frac{x }{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
And the position vector is given by the expression, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathbf{\vec{x}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 (\gamma_{11} x + \gamma_{12} y + \gamma_{13} z) | |||
+ | |||
\mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) | |||
+ | |||
\mathbf{\hat{e}}_3 (\gamma_{31} x + \gamma_{32} y + \gamma_{33} z) | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl[ x^2\ell_{3D} + q^2 y^2 \ell_{3D} + p^2 z^2 \ell_{3D} \biggr] | |||
+ | |||
\mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) | |||
+ | |||
\mathbf{\hat{e}}_3 \biggl[- \frac{q^2xy}{(x^2 + q^4y^2)^{1 / 2} } + \frac{xy }{(x^2 + q^4y^2)^{1 / 2} } \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl[ \lambda_1^2 \ell_{3D} \biggr] | |||
+ | |||
\mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) | |||
- | |||
\mathbf{\hat{e}}_3 \biggl[\frac{(q^2-1) xy }{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
==Guess 2<sup>nd</sup> Coordinate== | |||
===Unspecified Coefficients=== | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\lambda_2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(ax^2 + by^2 + ez^2)^{-1 / 2} (ax^2 + by^2)^{1 / 2}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[\frac{(ax^2 + by^2)}{(ax^2 + by^2 + ez^2)}\biggr]^{1 / 2}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-1 / 2} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\partial \lambda_2}{\partial x}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{1}{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \frac{\partial}{\partial x}\biggl[ez^2 (ax^2 + by^2)^{-1 } \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{ez^2 }{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \biggl( -1\biggr) \biggl[(ax^2 + by^2)^{-2} \biggr]2ax | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{a x e z^2 }{(ax^2 + by^2)^{2}}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{a x e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\partial \lambda_2}{\partial y}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{1}{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \frac{\partial}{\partial y}\biggl[ez^2 (ax^2 + by^2)^{-1} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{ez^2 }{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \biggl( -1\biggr) \biggl[(ax^2 + by^2)^{-2} \biggr]2by | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{by e z^2 }{(ax^2 + by^2)^{2}}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{b y e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\partial \lambda_2}{\partial z}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{1}{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \frac{\partial}{\partial z}\biggl[ez^2 (ax^2 + by^2)^{-1 } \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{ez ~ \lambda_2^3}{(ax^2 + by^2)} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Hence, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~h_2^{-2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[\frac{a x e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}}\biggr]^2 | |||
+ | |||
\biggl[ \frac{b y e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \biggr]^2 | |||
+ | |||
\biggl[\frac{ez ~ \lambda_2^3}{(ax^2 + by^2)} \biggr]^2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[\frac{e z ~\lambda_2^3}{(ax^2 + by^2)^{2}}\biggr]^2 | |||
\biggl[ | |||
(a x z)^2 | |||
+ | |||
(b y z )^2 | |||
+ | |||
(ax^2 + by^2)^2 | |||
\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ h_2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 | |||
\biggr]^{-1 / 2} | |||
\, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
The three direction-cosines are, then, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{21} = h_2 \biggl(\frac{\partial\lambda_2}{\partial x} \biggr)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{a x e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} | |||
\biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 | |||
\biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
axz | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 | |||
\biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{22} = h_2 \biggl(\frac{\partial\lambda_2}{\partial y} \biggr)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{b y e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} | |||
\biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 | |||
\biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
byz | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 | |||
\biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{23} = h_2 \biggl(\frac{\partial\lambda_2}{\partial z} \biggr)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{ez ~ \lambda_2^3}{(ax^2 + by^2)} | |||
\biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 | |||
\biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-(ax^2 + by^2) | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 | |||
\biggr]^{-1 / 2} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="1" cellpadding="9" align="center"> | |||
<tr> | |||
<td align="center" colspan="9">'''Direction Cosine Components for T9 Coordinates'''</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~n</math></td> | |||
<td align="center"><math>~\lambda_n</math></td> | |||
<td align="center"><math>~h_n</math></td> | |||
<td align="center"><math>~\frac{\partial \lambda_n}{\partial x}</math></td> | |||
<td align="center"><math>~\frac{\partial \lambda_n}{\partial y}</math></td> | |||
<td align="center"><math>~\frac{\partial \lambda_n}{\partial z}</math></td> | |||
<td align="center"><math>~\gamma_{n1}</math></td> | |||
<td align="center"><math>~\gamma_{n2}</math></td> | |||
<td align="center"><math>~\gamma_{n3}</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">(1)</td> | |||
<td align="center">(2)</td> | |||
<td align="center">(3)</td> | |||
<td align="center">(4)</td> | |||
<td align="center">(5)</td> | |||
<td align="center">(6)</td> | |||
<td align="center">(7)</td> | |||
<td align="center">(8)</td> | |||
<td align="center">(9)</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~1</math></td> | |||
<td align="center"><math>~(x^2 + q^2 y^2 + p^2 z^2)^{1 / 2} </math></td> | |||
<td align="center"><math>~\lambda_1 \ell_{3D}</math></td> | |||
<td align="center"><math>~\frac{x}{\lambda_1}</math></td> | |||
<td align="center"><math>~\frac{q^2 y}{\lambda_1}</math></td> | |||
<td align="center"><math>~\frac{p^2 z}{\lambda_1}</math></td> | |||
<td align="center"><math>~(x) \ell_{3D}</math></td> | |||
<td align="center"><math>~(q^2 y)\ell_{3D}</math></td> | |||
<td align="center"><math>~(p^2z) \ell_{3D}</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~2A</math></td> | |||
<td align="center">---</td> | |||
<td align="center" bgcolor="pink"><math>~\ell_{3D}(x^2 + q^4 y^2)^{1 / 2}</math></td> | |||
<td align="center" bgcolor="white"><math>~\frac{xp^2z}{(x^2 + q^4y^2)} </math></td> | |||
<td align="center" bgcolor="white"><math>~\frac{q^2 y p^2z}{(x^2 + q^4y^2)}</math></td> | |||
<td align="center" bgcolor="white"><math>~-1</math></td> | |||
<td align="center"><math>~\frac{x p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math></td> | |||
<td align="center"><math>~\frac{q^2 y p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math></td> | |||
<td align="center"><math>~-\frac{(x^2 + q^4 y^2)\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math></td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~2B</math></td> | |||
<td align="center" bgcolor="yellow"><math>~\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-1 / 2}</math></td> | |||
<td align="center"><math>~\biggl[\frac{(ax^2 + by^2)^2}{ez~\lambda_2^3} \biggr]\mathfrak{J}_{2\mathrm{B}}</math></td> | |||
<td align="center"><math>~\frac{ax~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math></td> | |||
<td align="center"><math>~\frac{by~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math></td> | |||
<td align="center"><math>~- \frac{ez ~\lambda_2^3}{(ax^2 + by^2)} </math></td> | |||
<td align="center"><math>~axz ~\mathfrak{J}_{2\mathrm{B}}</math></td> | |||
<td align="center"><math>~byz ~\mathfrak{J}_{2\mathrm{B}}</math></td> | |||
<td align="center"><math>~-(ax^2 + by^2)~\mathfrak{J}_{2\mathrm{B}} </math></td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~3</math></td> | |||
<td align="center"><math>~\biggl[ \frac{q^2y}{x^{q^2}} \biggr]^{1/(q^2-1)}</math></td> | |||
<td align="center"><math>~\frac{(q^2-1)x y }{\lambda_3(x^2 + q^4y^2)^{1 / 2}} </math></td> | |||
<td align="center"><math>~- \biggl( \frac{q^2}{q^2 - 1} \biggr) \frac{\lambda_3}{x}</math></td> | |||
<td align="center"><math>~\biggl( \frac{q^2}{q^2 - 1} \biggr) \frac{\lambda_3}{q^2y}</math></td> | |||
<td align="center"><math>~0</math></td> | |||
<td align="center"><math>~- \frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} </math></td> | |||
<td align="center"><math>~\frac{x }{(x^2 + q^4y^2)^{1 / 2}} </math></td> | |||
<td align="center"><math>~0</math></td> | |||
</tr> | |||
<tr> | |||
<td align="left" colspan="9"> | |||
<table border="0" cellpadding="8" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\ell_{3D}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(x^2 + q^4y^2 + p^4 z^2 )^{- 1 / 2} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{J}^{-2}_0 \equiv \frac{(x^2 + q^4 y^2)}{\ell_{3D}^2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[(xp^2 z)^2 + (q^2 y p^2 z )^2 + (x^2 + q^4y^2)^2\biggr] \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{J}_{2\mathrm{B}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2\biggr]^{-1 / 2} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td> | |||
</tr> | |||
</table> | |||
This table titled, "<i>Direction Cosine Components for T9 Coordinates</i>," contains the following information: | |||
<ol> | |||
<li> | |||
The (first) row labeled, <math>~n = 1</math>, correctly details the scale-factor, <math>~h_1</math>, and the unit vector expression, <math>~\hat{e}_1 = (\hat\imath \gamma_{11} + \hat\jmath \gamma_{12} + \hat{k} \gamma_{13})</math>, that result from the given specification of the <math>~\lambda_1</math> coordinate. By design, the unit vector, <math>~\hat{e}_1</math>, is everywhere normal to the "surface" of the ellipsoid. | |||
</li> | |||
<li> | |||
The (fourth) row labeled, <math>~n = 3</math>, correctly details the scale-factor, <math>~h_3</math>, and the unit vector expression, <math>~\hat{e}_3 = (\hat\imath \gamma_{31} + \hat\jmath \gamma_{32} + \hat{k} \gamma_{33})</math>, that result from the given specification of the <math>~\lambda_2</math> coordinate. By design, this unit vector, <math>~\hat{e}_3</math>, has no vertical component — that is, <math>~\gamma_{33} = 0</math> — and, by design, it is everywhere perpendicular to the "surface-normal" unit vector, <math>~\hat{e}_1</math>. | |||
</li> | |||
<li> | |||
We desire a unit vector, <math>~\hat{e}_2</math>, that is mutually orthogonal to the other two unit vectors; this has been accomplished by examining their cross-product, namely, we have set <math>~\hat{e}_{2\mathrm{A}} = \hat{e}_3 \times \hat{e}_1</math>. Determined in this manner, the expressions for the three direction-cosine components of <math>~\hat{e}_{2\mathrm{A}}</math> have been written in the last three columns of the (second) row labeled, <math>~n=2\mathrm{A}</math>. While we are confident that the correct specification of <math>~\hat{e}_2</math> is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\hat{e}_{2\mathrm{A}} = (\hat\imath \gamma_{21} + \hat\jmath \gamma_{22} + \hat{k} \gamma_{23})</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\hat\imath (xp^2 z)\mathfrak{J}_0 + \hat\jmath (q^2y p^2 z)\mathfrak{J}_0 - \hat{k} (x^2 + q^4y^2) \mathfrak{J}_0 \, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
as yet (18 February 2021), we have been unable to determine an expression for the coordinate, <math>~\lambda_{2\mathrm{A}}(x, y, z)</math>, from which all three of these direction-cosine expressions can be simultaneously derived — hence, the dashes in the second column of row 2A. The expression for <math>~h_{2\mathrm{A}}</math> that has been presented in the third column of row 2A (and framed in pink) is a ''guess'' which, when divided into each of the three direction cosines, gives respectively the three ''guessed'' partial-derivative expressions shown in columns 4, 5, and 6 of row 2A. | |||
</li> | |||
<li> | |||
The second column of row 2B contains a ''guess'' (framed in yellow) for the coordinate expression, <math>~\lambda_{2\mathrm{B}}</math>; this expression contains three unspecified scalar coefficients, <math>~a</math>, <math>~b</math> and <math>~e</math>. The remaining columns of this row contain the three partial derivatives, the associated scale factor, and the three direction cosines that result from this ''guessed'' coordinate expression. If we can find values of the three scalar coefficients that give (row 2B) expressions for the three direction cosines that perfectly match the direction cosines written in row 2A, then we will be able to state that <math>~\lambda_{2\mathrm{B}}</math> is — at least one form of — our sought-after third coordinate expression. | |||
</li> | |||
</ol> | |||
===Yellow-Framed Guess 2B=== | |||
Referencing the 2B table row, above, we are looking for coefficient values that map, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~axz \rightarrow xp^2 z \, ,</math> | |||
</td> | |||
<td align="center"> </td> | |||
<td align="center"> | |||
<math>~byz \rightarrow q^2 yp^2 z \, ,</math> | |||
</td> | |||
<td align="center"> </td> | |||
<td align="left"> | |||
<math>~(ax^2 + by^2) \rightarrow (x^2 + q^4y^2) \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
and that map, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\mathfrak{J}_{2\mathrm{B}} \equiv \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[z^2(a^2 x^2 + b^2 y^2) + (ax^2 + by^2)^2 \biggr]^{-1 / 2} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
into the expression, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{J}_0 \equiv \frac{\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ | |||
(x^2 + q^4y^2 + p^4 z^2)(x^2 + q^4 y^2) | |||
\biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ | |||
p^4 z^2(x^2 + q^4 y^2) | |||
+ | |||
(x^2 + q^4y^2 )^2 | |||
\biggr]^{-1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ | |||
x^2 p^4 z^2 | |||
+ | |||
q^4 y^2 p^4 z^2 | |||
+ | |||
(x^2 + q^4y^2 )^2 | |||
\biggr]^{-1 / 2} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
A portion of these mappings are accomplished by setting <math>~a = p^2</math> and <math>~b = q^2p^2</math>, but this pair of specified coefficient values does not satisfy other mappings. Alternatively, a separate subset of mappings — but, again, not all mappings — is satisfied by setting <math>~a = 1</math> and <math>~b = q^4</math>. So the yellow-framed <font color="red">guess 2B does not provide a correct second-coordinate expression</font>. | |||
===Blue-Framed Guess 2C=== | |||
Let's try, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\lambda_{2\mathrm{B}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(cz)^{2e} (ax^2 + by^2)^{-f} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="1" cellpadding="9" align="center"> | |||
<tr> | |||
<td align="center" colspan="9">'''Direction Cosine Components for Additional T9 Coordinate Guesses'''</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~n</math><br /> <br />(1)</td> | |||
<td align="center"><math>~\lambda_n</math><br /> <br />(2)</td> | |||
<td align="center"><math>~h_n</math><br /> <br />(3)</td> | |||
<td align="center"><math>~\partial \lambda_n/\partial x</math><br /> <br />(4)</td> | |||
<td align="center"><math>~\partial \lambda_n/\partial y</math><br /> <br />(5)</td> | |||
<td align="center"><math>~\partial \lambda_n/\partial z</math><br /> <br />(6)</td> | |||
<td align="center"><math>~\gamma_{n1}</math><br /> <br />(7)</td> | |||
<td align="center"><math>~\gamma_{n2}</math><br /> <br />(8)</td> | |||
<td align="center"><math>~\gamma_{n3}</math><br /> <br />(9)</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~2A</math></td> | |||
<td align="center">---</td> | |||
<td align="center" bgcolor="white">---</td> | |||
<td align="center" bgcolor="white">---</td> | |||
<td align="center" bgcolor="white">---</td> | |||
<td align="center" bgcolor="white">---</td> | |||
<td align="center"><math>~x p^2 z ~\mathfrak{J}_0 </math></td> | |||
<td align="center"><math>~q^2 y p^2 z~\mathfrak{J}_0 </math></td> | |||
<td align="center"><math>~-(x^2 + q^4 y^2)~\mathfrak{J}_0 </math></td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~2B</math></td> | |||
<td align="center" bgcolor="yellow"><math>~\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-1 / 2}</math></td> | |||
<td align="center"><math>~\biggl[\frac{(ax^2 + by^2)^2}{ez~\lambda_2^3} \biggr]\mathfrak{J}_{2\mathrm{B}}</math></td> | |||
<td align="center"><math>~\frac{ax~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math></td> | |||
<td align="center"><math>~\frac{by~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math></td> | |||
<td align="center"><math>~- \frac{ez ~\lambda_2^3}{(ax^2 + by^2)} </math></td> | |||
<td align="center"><math>~axz ~\mathfrak{J}_{2\mathrm{B}}</math></td> | |||
<td align="center"><math>~byz ~\mathfrak{J}_{2\mathrm{B}}</math></td> | |||
<td align="center"><math>~-(ax^2 + by^2)~\mathfrak{J}_{2\mathrm{B}} </math></td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~2C</math></td> | |||
<td align="center" bgcolor="lightblue"><math>~(cz)^{2e} (ax^2 + by^2)^{-f}</math></td> | |||
<td align="center"><math>~\biggl[\frac{z(ax^2 + by^2)}{2e~\lambda_{2\mathrm{C}}} \biggr]\mathfrak{J}_{2\mathrm{C}}</math></td> | |||
<td align="center"><math>~- \frac{2afx~\lambda_{2\mathrm{C}} }{(ax^2 + by^2)}</math></td> | |||
<td align="center"><math>~- \frac{2bfy~\lambda_{2\mathrm{C}} }{(ax^2 + by^2)}</math></td> | |||
<td align="center"><math>~\frac{2e~\lambda_{2\mathrm{C}} }{z}</math></td> | |||
<td align="center"><math>~ -\biggl( \frac{af xz}{e} \biggr)~\mathfrak{J}_{2\mathrm{C}}</math></td> | |||
<td align="center"><math>~- \biggl( \frac{bf yz}{e} \biggr)~\mathfrak{J}_{2\mathrm{C}}</math></td> | |||
<td align="center"><math>~(ax^2 + by^2)~\mathfrak{J}_{2\mathrm{C}} </math></td> | |||
</tr> | |||
<tr> | |||
<td align="left" colspan="9"> | |||
<table border="0" cellpadding="8" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\ell_{3D}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(x^2 + q^4y^2 + p^4 z^2 )^{- 1 / 2} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{J}^{-2}_0 \equiv \frac{(x^2 + q^4 y^2)}{\ell_{3D}^2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[(xp^2 z)^2 + (q^2 y p^2 z )^2 + (x^2 + q^4y^2)^2\biggr] \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{J}_{2\mathrm{B}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2\biggr]^{-1 / 2} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{J}_{2\mathrm{C}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[(x z)^2 \biggl( \frac{fa}{e} \biggr)^2 + (y z )^2\biggl( \frac{fb}{e} \biggr)^2 + (ax^2 + by^2)^2\biggr]^{-1 / 2} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td> | |||
</tr> | |||
</table> | |||
Examining just the expression for <math>~\mathfrak{J}_{2\mathrm{C}}</math>, we see that we definitely need: <math>~a = 1</math> and <math>~b=q^4</math>. Also, we need, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{af}{e}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~p^2</math> | |||
</td> | |||
<td align="center"> <math>~\Rightarrow </math> | |||
<td align="right"> | |||
<math>~\frac{f}{e}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~p^2 \, ;</math> | |||
</td> | |||
</tr> | |||
</table> | |||
and, separately we need, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{fb}{e}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~q^2 p^2</math> | |||
</td> | |||
<td align="center"> <math>~\Rightarrow </math> | |||
<td align="right"> | |||
<math>~\frac{f}{e}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{p^2}{q^2} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
These cannot simultaneously be satisfied. So the blue-framed <font color="red">guess 2C does not provide a correct second-coordinate expression</font>. But we are very close! We need one additional scalar coefficient degree of freedom. | |||
===Next Thought=== | |||
More generally, this leads to an expression for the scale-factor of the form, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~h_2^{-2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\sim</math> | |||
</td> | |||
<td align="left" colspan="9"> | |||
<math>~ | |||
\mathfrak{G}(x, y, z) | |||
\biggl[ | |||
x^2 p^4 z^2 | |||
+ | |||
q^4 y^2 p^4 z^2 | |||
+ | |||
(x^2 + q^4y^2 )^2 | |||
\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ \frac{\partial \lambda_2}{\partial x}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\sim</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
[\mathfrak{G}(x, y, z) ]^{1 / 2} | |||
\biggl[ | |||
x p^2 z | |||
\biggr] \, ; | |||
</math> | |||
</td> | |||
<td align="center"> </td> | |||
<td align="right"> | |||
<math>~\frac{\partial \lambda_2}{\partial y}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\sim</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
[\mathfrak{G}(x, y, z) ]^{1 / 2} | |||
\biggl[ | |||
q^2 y p^2 z | |||
\biggr] \, ; | |||
</math> | |||
</td> | |||
<td align="center"> </td> | |||
<td align="right"> | |||
<math>~\frac{\partial \lambda_2}{\partial z}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\sim</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
[\mathfrak{G}(x, y, z) ]^{1 / 2} | |||
\biggl[ | |||
x^2 + q^4y^2 | |||
\biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
---- | |||
Now, if we set, <math>~a = p^2</math> and <math>~b = q^2p^2</math>, we have, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
( x p^2 z)^2 + (q^2p^2 y z )^2 + (p^2 x^2 + q^2p^2 y^2)^2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
p^4[ q^4 y^2 z^2 + x^2 z^2 + (x^2 + q^2 y^2 )^2] \, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
in which case, | |||
=Complete Orthogonality Check= | =Complete Orthogonality Check= | ||
| Line 2,099: | Line 3,748: | ||
</table> | </table> | ||
</td></tr></table> | </td></tr></table> | ||
and the position vector is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathbf{\vec{x}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat\imath} x + | |||
\mathbf{\hat\jmath} y + | |||
\mathbf{\hat{k}} z | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 (\gamma_{11} x + \gamma_{12} y + \gamma_{13} z) | |||
+ | |||
\mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) | |||
+ | |||
\mathbf{\hat{e}}_3 (\gamma_{31} x + \gamma_{32} y + \gamma_{33} z) \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
==For (κ<sub>1</sub>, κ<sub>2</sub>, κ<sub>3</sub>)== | ==For (κ<sub>1</sub>, κ<sub>2</sub>, κ<sub>3</sub>)== | ||
| Line 2,186: | Line 3,873: | ||
</math> | </math> | ||
<font color="red">Yes!</font> | <font color="red">Yes!</font> | ||
</td> | |||
</tr> | |||
</table> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{32}\gamma_{13} - \gamma_{33}\gamma_{12}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
\biggl[ p^2z \ell_{3D} \biggr] | |||
- | |||
\biggl[ 0\biggr] | |||
\biggl[ q^2y \ell_{3D} \biggr] | |||
= | |||
\biggl[ \frac{xp^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
= | |||
\gamma_{21} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{33}\gamma_{11} - \gamma_{31}\gamma_{13}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ 0\biggr] | |||
\biggl[ x\ell_{3D} \biggr] | |||
+ | |||
\biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
\biggl[ p^2z \ell_{3D} \biggr] | |||
= | |||
\biggl[ \frac{q^2y p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
= | |||
\gamma_{22} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{31}\gamma_{12} - \gamma_{32}\gamma_{11}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
\biggl[ q^2y \ell_{3D} \biggr] | |||
- | |||
\biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
\biggl[ x\ell_{3D} \biggr] | |||
= | |||
-(x^2 + q^4y^2)^{1 / 2}\ell_{3D} | |||
= | |||
\gamma_{23} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{12}\gamma_{23} - \gamma_{13}\gamma_{22}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- \biggl[ q^2y \ell_{3D} \biggr] | |||
\biggl[ (x^2 + q^4y^2)^{1 / 2}\ell_{3D} \biggr] | |||
- | |||
\biggl[ p^2 z\ell_{3D} \biggr] | |||
\biggl[ \frac{q^2y p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- \frac{ q^2 y \ell_{3D}^2 }{(x^2 + q^4y^2)^{1 / 2} } | |||
\biggl[ x^2 + q^4y^2 | |||
+ | |||
p^4 z^2 \biggr] | |||
= | |||
- \frac{ q^2 y }{(x^2 + q^4y^2)^{1 / 2} } | |||
= | |||
\gamma_{31} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{13}\gamma_{21} - \gamma_{11}\gamma_{23}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ p^2z \ell_{3D} \biggr] | |||
\biggl[ \frac{x p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
+ | |||
\biggl[ x \ell_{3D} \biggr] | |||
\biggl[ (x^2 + q^4y^2)^{1 / 2} \ell_{3D}\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{x \ell_{3D}^2 }{ (x^2 + q^4y^2)^{1 / 2} } \biggl[ | |||
x^2 + q^4y^2 + p^4 z^2 | |||
\biggr] | |||
= | |||
\frac{x }{ (x^2 + q^4y^2)^{1 / 2} } | |||
= | |||
\gamma_{32} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{11}\gamma_{22} - \gamma_{12}\gamma_{21}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ x \ell_{3D} \biggr] | |||
\biggl[ \frac{q^2y p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
- | |||
\biggl[ q^2y \ell_{3D} \biggr] | |||
\biggl[ \frac{xp^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] | |||
= | |||
0 | |||
= | |||
\gamma_{33} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
</table> | |||
Given that the prescribed interrelationships between all nine direction cosines are satisfied, we conclude that the <math>~(\kappa_1, \kappa_2, \kappa_3)</math> coordinate system is an orthogonal one. Accordingly, the position vector is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathbf{\vec{x}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl\{ | |||
x^2 \ell_{3D} | |||
+ q^2y^2 \ell_{3D} | |||
+ p^2z^2 \ell_{3D} | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ | |||
\mathbf{\hat{e}}_2 \biggl\{ | |||
x^2 + q^2y^2 - \frac{1}{p^2}(x^2 + q^4 y^2) | |||
\biggr\} \frac{p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+~ | |||
\mathbf{\hat{e}}_3 \biggl\{ | |||
- \frac{xq^2y}{(x^2 + q^4y^2)^{1 / 2}} | |||
+ \frac{xy}{(x^2 + q^4 y^2)^{1 / 2}} | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl[ \kappa_1^2 \ell_{3D} \biggr] | |||
~+~ | |||
\mathbf{\hat{e}}_2 \biggl[ | |||
x^2 + q^2y^2 - \frac{1}{p^2}(x^2 + q^4 y^2) | |||
\biggr] \frac{p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} | |||
~+~ | |||
\mathbf{\hat{e}}_3 | |||
\biggl[ | |||
\frac{(1-q^2)xy}{(x^2 + q^4 y^2)^{1 / 2}} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl[ \kappa_1^2 \ell_{3D} \biggr] | |||
~+~ | |||
\mathbf{\hat{e}}_2 \biggl[ | |||
x^2\biggl(1 - \frac{1}{p^2}\biggr) - q^2y^2 \biggl(q^2 - 1 \biggr) | |||
\biggr] \frac{p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} | |||
~-~ | |||
\mathbf{\hat{e}}_3 | |||
\biggl[ | |||
\frac{(q^2 - 1)xy}{(x^2 + q^4 y^2)^{1 / 2}} \biggr] \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
==For (κ<sub>1</sub>, κ<sub>4</sub>, κ<sub>5</sub>)== | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{42}\gamma_{53} - \gamma_{43}\gamma_{52}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{xq^2y p^2z}{\mathcal{D}} \biggl( \frac{1}{q^2y} \biggr) \biggr] | |||
\biggl[ \frac{p^2 z \ell_{3D}}{\mathcal{D}} \biggl(x^2 - q^4y^2 \biggr) \biggr] | |||
+ | |||
\biggl[ \frac{xq^2 y p^2 z}{\mathcal{D}} \biggl( \frac{2}{p^2z} \biggr) \biggr] | |||
\biggl[ \frac{q^2y \ell_{3D}}{\mathcal{D}}\biggl( p^4 z^2 + 2x^2 \biggr) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \frac{x\ell_{3D}}{\mathcal{D}^2} \biggl[ | |||
(x^2 - q^4y^2) p^4z^2 | |||
+ | |||
( p^4 z^2 + 2x^2 )2q^4y^2 \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \frac{x\ell_{3D}}{\mathcal{D}^2} \biggl[ | |||
x^2p^4z^2 | |||
+ | |||
4x^2 q^4y^2 | |||
+ | |||
q^4y^2 p^4 z^2 \biggr] | |||
= | |||
x\ell_{3D} = \gamma_{11} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{43}\gamma_{51} - \gamma_{41}\gamma_{53}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{xq^2y p^2z}{\mathcal{D}} \biggl( \frac{2}{p^2 z} \biggr) \biggr] | |||
\biggl[ \frac{x \ell_{3D}}{\mathcal{D}} \biggl(2q^4y^2 +p^4z^2\biggr) \biggr] | |||
- | |||
\biggl[ \frac{xq^2 y p^2 z}{\mathcal{D}} \biggl( \frac{1}{x} \biggr) \biggr] | |||
\biggl[ \frac{p^2 z \ell_{3D}}{\mathcal{D}}\biggl( x^2 - q^4y^2 \biggr) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{q^2 y \ell_{3D}}{\mathcal{D}^2} \biggl[ | |||
2 x^2 (2q^4y^2 +p^4z^2 ) | |||
- | |||
p^4 z^2 ( x^2 - q^4y^2) | |||
\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{q^2 y \ell_{3D}}{\mathcal{D}^2} \biggl[ | |||
4x^2 q^4y^2 + x^2 p^4z^2 + q^4y^2 p^4 z^2 | |||
\biggr] | |||
= | |||
q^2y \ell_{3D} | |||
= \gamma_{12} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{41}\gamma_{52} - \gamma_{42}\gamma_{51}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{xq^2y p^2z}{\mathcal{D}} \biggl( \frac{1}{x} \biggr) \biggr] | |||
\biggl[ \frac{q^2 y\ell_{3D}}{\mathcal{D}} \biggl(p^4z^2 + 2x^2\biggr) \biggr] | |||
+ | |||
\biggl[ \frac{xq^2 y p^2 z}{\mathcal{D}} \biggl( \frac{1}{q^2 y} \biggr) \biggr] | |||
\biggl[ \frac{x \ell_{3D}}{\mathcal{D}}\biggl( 2q^4y^2 + p^4z^2 \biggr) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{p^2 z \ell_{3D}}{\mathcal{D}^2} \biggl[ | |||
q^4y^2 (p^4z^2 + 2x^2) | |||
+ | |||
x^2 ( 2q^4y^2 + p^4z^2 ) | |||
\biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{p^2 z \ell_{3D}}{\mathcal{D}^2} \biggl[ | |||
q^4y^2 p^4z^2 + 4x^2 q^4 y^2 | |||
+ x^2p^4z^2 | |||
\biggr] | |||
= | |||
p^2 z \ell_{3D} | |||
= | |||
\gamma_{13} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{52}\gamma_{13} - \gamma_{53}\gamma_{12}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{q^2 y p^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( p^4z^2 + 2x^2 \biggr) \biggr] | |||
- | |||
\biggl[ \frac{q^2 y p^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( x^2 - q^4y^2 \biggr) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{q^2 y p^2 z\ell_{3D}^2}{\mathcal{D}} \biggl[ x^2 + q^4y^2 + p^4z^2 \biggr] | |||
= | |||
\frac{q^2 y p^2 z }{\mathcal{D}} | |||
= | |||
\gamma_{41} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{53}\gamma_{11} - \gamma_{51}\gamma_{13}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{xp^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( x^2 - q^4y^2 \biggr) \biggr] | |||
+ | |||
\biggl[ \frac{xp^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( 2q^4y^2 +p^4z^2\biggr) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{xp^2 z\ell_{3D}^2}{\mathcal{D}} \biggl[ x^2 + q^4y^2 +p^4z^2 \biggr] | |||
= | |||
\frac{xp^2 z}{\mathcal{D}} | |||
= | |||
\gamma_{42} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{51}\gamma_{12} - \gamma_{52}\gamma_{11}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\biggl[ \frac{x q^2y \ell_{3D}^2}{\mathcal{D}} \biggl( 2q^4y^2 +p^4z^2\biggr) \biggr] | |||
- | |||
\biggl[ \frac{x q^2y \ell_{3D}^2}{\mathcal{D}} \biggl( p^4z^2 + 2x^2\biggr) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\frac{2x q^2y \ell_{3D}^2}{\mathcal{D}}\biggl[ x^2 + q^4y^2 +p^4z^2 \biggr] | |||
= | |||
-\frac{2x q^2y }{\mathcal{D}} | |||
= | |||
\gamma_{43} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{12}\gamma_{43} - \gamma_{13}\gamma_{42}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- \biggl[ \frac{2x q^4 y^2 \ell_{3D} }{\mathcal{D}} \biggr] | |||
- \biggl[ \frac{x p^4 z^2 \ell_{3D} }{\mathcal{D}} \biggr] | |||
= | |||
- \biggl[ \frac{x \ell_{3D} }{\mathcal{D}} \biggr] (2q^4 y^2 + p^4z^2) | |||
= \gamma_{51} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{13}\gamma_{41} - \gamma_{11}\gamma_{43}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{q^2 y p^4z^2 \ell_{3D} }{\mathcal{D}} \biggr] | |||
+ | |||
\biggl[ \frac{2x^2 q^2 y \ell_{3D} }{\mathcal{D}} \biggr] | |||
= | |||
\biggl[ \frac{q^2 y \ell_{3D} }{\mathcal{D}} \biggr](p^4z^2 + 2x^2) | |||
= | |||
\gamma_{52} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\gamma_{11}\gamma_{42} - \gamma_{12}\gamma_{41}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{x^2 p^2z \ell_{3D}}{\mathcal{D}} \biggr] | |||
- | |||
\biggl[ \frac{q^4 y^2 p^2 z\ell_{3D} }{\mathcal{D}} \biggr] | |||
= | |||
\biggl[ \frac{p^2z \ell_{3D}}{\mathcal{D}} \biggr](x^2 - q^4y^2) | |||
= | |||
\gamma_{53} \, . | |||
</math> | |||
<font color="red">Yes!</font> | |||
</td> | |||
</tr> | |||
</table> | |||
Given that the prescribed interrelationships between all nine direction cosines are satisfied, we conclude that the <math>~(\kappa_1, \kappa_4, \kappa_5)</math> coordinate system is an orthogonal one. Accordingly, the position vector is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathbf{\vec{x}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 (\gamma_{11} x + \gamma_{12} y + \gamma_{13} z) | |||
+ | |||
\mathbf{\hat{e}}_2 (\gamma_{41} x + \gamma_{42} y + \gamma_{43} z) | |||
+ | |||
\mathbf{\hat{e}}_3 (\gamma_{51} x + \gamma_{52} y + \gamma_{53} z) | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl\{ | |||
x^2\ell_{3D} | |||
+ q^2y^2 \ell_{3D} | |||
+ p^2z^2\ell_{3D} | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ | |||
\mathbf{\hat{e}}_2 \biggl\{ | |||
\frac{xq^2yp^2z}{\mathcal{D}} | |||
+ \frac{x y p^2z}{\mathcal{D}} | |||
- \frac{2 xq^2y z}{\mathcal{D}} | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ | |||
\mathbf{\hat{e}}_3 \biggl\{ | |||
- \frac{\ell_{3D} x^2}{\mathcal{D}} (2q^4y^2 + p^4z^2) | |||
+ \frac{\ell_{3D} q^2 y^2}{\mathcal{D}} (p^4z^2 + 2x^2) | |||
+ \frac{\ell_{3D} p^2z^2}{\mathcal{D}} (x^2 - q^4y^2) | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl\{ | |||
\kappa_1^2 \ell_{3D} | |||
\biggr\} | |||
+ | |||
\mathbf{\hat{e}}_2 \biggl\{ | |||
q^2p^2 + p^2 - 2 q^2 | |||
\biggr\} \frac{xyz}{\mathcal{D}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ | |||
\mathbf{\hat{e}}_3 \biggl\{ | |||
- x^2 (2q^4y^2 + p^4z^2) | |||
+ q^2 y^2 (p^4z^2 + 2x^2) | |||
+ p^2z^2 (x^2 - q^4y^2) | |||
\biggr\} \frac{\ell_{3D}}{\mathcal{D}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\mathbf{\hat{e}}_1 \biggl\{ | |||
\kappa_1^2 \ell_{3D} | |||
\biggr\} | |||
~+~ | |||
\mathbf{\hat{e}}_2 \biggl\{ | |||
q^2p^2 + p^2 - 2 q^2 | |||
\biggr\} \frac{xyz}{\mathcal{D}} | |||
~-~ | |||
\mathbf{\hat{e}}_3 \biggl\{ 2 x^2 q^2y^2(q^2-1) + x^2 p^2z^2(p^2-1) + q^2 y^2 p^2 z^2(q^2 - p^2) | |||
\biggr\} \frac{\ell_{3D}}{\mathcal{D}} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
Latest revision as of 20:52, 24 February 2021
Concentric Ellipsoidal (T8) Coordinates

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Background
Building on our general introduction to Direction Cosines in the context of orthogonal curvilinear coordinate systems, and on our previous development of T3 (concentric oblate-spheroidal) and T5 (concentric elliptic) coordinate systems, here we explore the creation of a concentric ellipsoidal (T8) coordinate system. This is motivated by our desire to construct a fully analytically prescribable model of a nonuniform-density ellipsoidal configuration that is an analog to Riemann S-Type ellipsoids.
Note that, in a separate but closely related discussion, we made attempts to define this coordinate system, numbering the trials up through "T7." In this "T7" effort, we were able to define a set of three, mutually orthogonal unit vectors that should work to define a fully three-dimensional, concentric ellipsoidal coordinate system. But we were unable to figure out what coordinate function, <math>~\lambda_3(x, y, z)</math>, was associated with the third unit vector. In addition, we found the <math>~\lambda_2</math> coordinate to be rather strange in that it was not oriented in a manner that resembled the classic spherical coordinate system. Here we begin by redefining the <math>~\lambda_2</math> coordinate such that its associated <math>~\hat{e}_3</math> unit vector lies parallel to the x-y plane.
Realigning the Second Coordinate
The first coordinate remains the same as before, namely,
|
<math>~\lambda_1^2</math> |
<math>~=</math> |
<math>~x^2 + q^2 y^2 + p^2 z^2 \, .</math> |
This may be rewritten as,
|
<math>~1</math> |
<math>~=</math> |
<math>~\biggl( \frac{x}{a}\biggr)^2 + \biggl( \frac{y}{b}\biggr)^2 + \biggl(\frac{z}{c}\biggr)^2 \, ,</math> |
where,
|
<math>~a = \lambda_1 \, ,</math> |
<math>~b = \frac{\lambda_1}{q} \, ,</math> |
<math>~c = \frac{\lambda_1}{p} \, .</math> |
By specifying the value of <math>~z = z_0 < c</math>, as well as the value of <math>~\lambda_1</math>, we are picking a plane that lies parallel to, but a distance <math>~z_0</math> above, the equatorial plane. The elliptical curve that defines the intersection of the <math>~\lambda_1</math>-constant surface with this plane is defined by the expression,
|
<math>~\lambda_1^2 - p^2z_0^2</math> |
<math>~=</math> |
<math>~x^2 + q^2 y^2 </math> |
|
<math>~\Rightarrow~~~1</math> |
<math>~=</math> |
<math>~\biggl( \frac{x}{a_{2D}}\biggr)^2 + \biggl( \frac{y}{b_{2D}}\biggr)^2 \, ,</math> |
where,
|
<math>~a_{2D} = \biggl(\lambda_1^2 - p^2z_0^2 \biggr)^{1 / 2} \, ,</math> |
<math>~b_{2D} = \frac{1}{q} \biggl(\lambda_1^2 - p^2z_0^2 \biggr)^{1 / 2} \, .</math> |
At each point along this elliptic curve, the line that is tangent to the curve has a slope that can be determined by simply differentiating the equation that describes the curve, that is,
|
<math>~0</math> |
<math>~=</math> |
<math>~\frac{2x dx}{a_{2D}^2} + \frac{2y dy}{b_{2D}^2}</math> |
|
<math>~\Rightarrow~~~\frac{dy}{dx}</math> |
<math>~=</math> |
<math>~- \frac{2x}{a_{2D}^2} \cdot \frac{b_{2D}^2}{2y} = - \frac{x}{q^2y} \, .</math> |
|
<math>~\Rightarrow~~~\Delta y</math> |
<math>~=</math> |
<math>~- \biggl( \frac{x}{q^2y} \biggr)\Delta x \, .</math> |
The unit vector that lies tangent to any point on this elliptical curve will be described by the expression,
|
<math>~\hat{e}_2</math> |
<math>~=</math> |
<math>~ \hat\imath~ \biggl\{ \frac{\Delta x}{[ (\Delta x)^2 + (\Delta y)^2 ]^{1 / 2}} \biggr\} + \hat\jmath~ \biggl\{ \frac{\Delta y}{[ (\Delta x)^2 + (\Delta y)^2 ]^{1 / 2}} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \hat\imath~ \biggl\{ \frac{1}{[ 1 + x^2/(q^4y^2) ]^{1 / 2}} \biggr\} - \hat\jmath~ \biggl\{ \frac{x/(q^2y)}{[ 1 + x^2/(q^4y^2) ]^{1 / 2}} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \hat\imath~ \biggl\{ \frac{q^2y}{[ x^2 + q^4y^2 ]^{1 / 2}} \biggr\} - \hat\jmath~ \biggl\{ \frac{x}{[ x^2 + q^4y^2 ]^{1 / 2}} \biggr\} \, .</math> |
As we have discovered, the coordinate that gives rise to this unit vector is,
|
<math>~\lambda_2</math> |
<math>~=</math> |
<math>~\frac{x}{y^{1/q^2}} \, .</math> |
Other properties that result from this definition of <math>~\lambda_2</math> are presented in the following table.
| Direction Cosine Components for T8 Coordinates | |||||||||||
| <math>~n</math> | <math>~\lambda_n</math> | <math>~h_n</math> | <math>~\frac{\partial \lambda_n}{\partial x}</math> | <math>~\frac{\partial \lambda_n}{\partial y}</math> | <math>~\frac{\partial \lambda_n}{\partial z}</math> | <math>~\gamma_{n1}</math> | <math>~\gamma_{n2}</math> | <math>~\gamma_{n3}</math> | |||
| <math>~1</math> | <math>~(x^2 + q^2 y^2 + p^2 z^2)^{1 / 2} </math> | <math>~\lambda_1 \ell_{3D}</math> | <math>~\frac{x}{\lambda_1}</math> | <math>~\frac{q^2 y}{\lambda_1}</math> | <math>~\frac{p^2 z}{\lambda_1}</math> | <math>~(x) \ell_{3D}</math> | <math>~(q^2 y)\ell_{3D}</math> | <math>~(p^2z) \ell_{3D}</math> | |||
| <math>~2</math> | <math>~\frac{x}{ y^{1/q^2}}</math> | <math>~\frac{1}{\lambda_2}\biggl[\frac{x q^2 y }{(x^2 + q^4y^2)^{1 / 2}}\biggr] </math> | <math>~\frac{\lambda_2}{x}</math> | <math>~-\frac{\lambda_2}{q^2 y}</math> | <math>~0</math> | <math>~\frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~- \frac{x }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~0</math> | |||
| <math>~3</math> | --- | --- | --- | --- | --- | --- | --- | --- | |||
|
|||||||||||
The associated unit vector is, then,
|
<math>~\hat{e}_2</math> |
<math>~=</math> |
<math>~ \hat\imath~\biggl[ \frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} \biggr] - \hat\jmath~\biggl[ \frac{x }{(x^2 + q^4y^2)^{1 / 2}} \biggr] \, . </math> |
It is easy to see that <math>~\hat{e}_2 \cdot \hat{e}_2 = 1</math>. We also see that,
|
<math>~\hat{e}_1 \cdot \hat{e}_2</math> |
<math>~=</math> |
<math>~ x \ell_{3D}\biggl[ \frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} \biggr] - q^2y \ell_{3D} \biggl[ \frac{x }{(x^2 + q^4y^2)^{1 / 2}} \biggr] = 0 \, , </math> |
so it is clear that these first two unit vectors are orthogonal to one another.
Search for the Third Coordinate
Cross Product of First Two Unit Vectors
The cross-product of these two unit vectors should give the third, namely,
|
<math>~\hat{e}_3 = \hat{e}_1 \times \hat{e}_2</math> |
<math>~=</math> |
<math>~ \hat\imath~\biggl[ {e}_{1y}{e}_{2z} - {e}_{1z}{e}_{2y} \biggr] + \hat\jmath~\biggl[ {e}_{1z}{e}_{2x} - {e}_{1x}{e}_{2z} \biggr] + \hat{k}~\biggl[ {e}_{1x}{e}_{2y} - {e}_{1y}{e}_{2x} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \hat\imath~\biggl[ \frac{x p^2z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] + \hat\jmath~\biggl[ \frac{q^2 y p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] - \hat{k}~\biggl[ \frac{x^2\ell_{3D} }{(x^2 + q^4y^2)^{1 / 2}} ~+~ \frac{q^4 y^2\ell_{3D} }{(x^2 + q^4y^2)^{1 / 2}} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggl\{~ \hat\imath~( x p^2z ) + \hat\jmath~(q^2 y p^2z ) - \hat{k}~(x^2 + q^4y^2) ~\biggr\} \, . </math> |
Inserting these component expressions into the last row of the T8 Direction Cosine table gives …
| Direction Cosine Components for T8 Coordinates | |||||||||||
| <math>~n</math> | <math>~\lambda_n</math> | <math>~h_n</math> | <math>~\frac{\partial \lambda_n}{\partial x}</math> | <math>~\frac{\partial \lambda_n}{\partial y}</math> | <math>~\frac{\partial \lambda_n}{\partial z}</math> | <math>~\gamma_{n1}</math> | <math>~\gamma_{n2}</math> | <math>~\gamma_{n3}</math> | |||
| <math>~1</math> | <math>~(x^2 + q^2 y^2 + p^2 z^2)^{1 / 2} </math> | <math>~\lambda_1 \ell_{3D}</math> | <math>~\frac{x}{\lambda_1}</math> | <math>~\frac{q^2 y}{\lambda_1}</math> | <math>~\frac{p^2 z}{\lambda_1}</math> | <math>~(x) \ell_{3D}</math> | <math>~(q^2 y)\ell_{3D}</math> | <math>~(p^2z) \ell_{3D}</math> | |||
| <math>~2</math> | <math>~\frac{x}{ y^{1/q^2}}</math> | <math>~\frac{1}{\lambda_2}\biggl[\frac{x q^2 y }{(x^2 + q^4y^2)^{1 / 2}}\biggr] </math> | <math>~\frac{\lambda_2}{x}</math> | <math>~-\frac{\lambda_2}{q^2 y}</math> | <math>~0</math> | <math>~\frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~- \frac{x }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~0</math> | |||
| <math>~3</math> | --- | --- | --- | --- | --- | <math>~\frac{x p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~\frac{q^2 y p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~-\frac{(x^2 + q^4 y^2)\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | |||
|
|||||||||||
Associated h3 Scale Factor
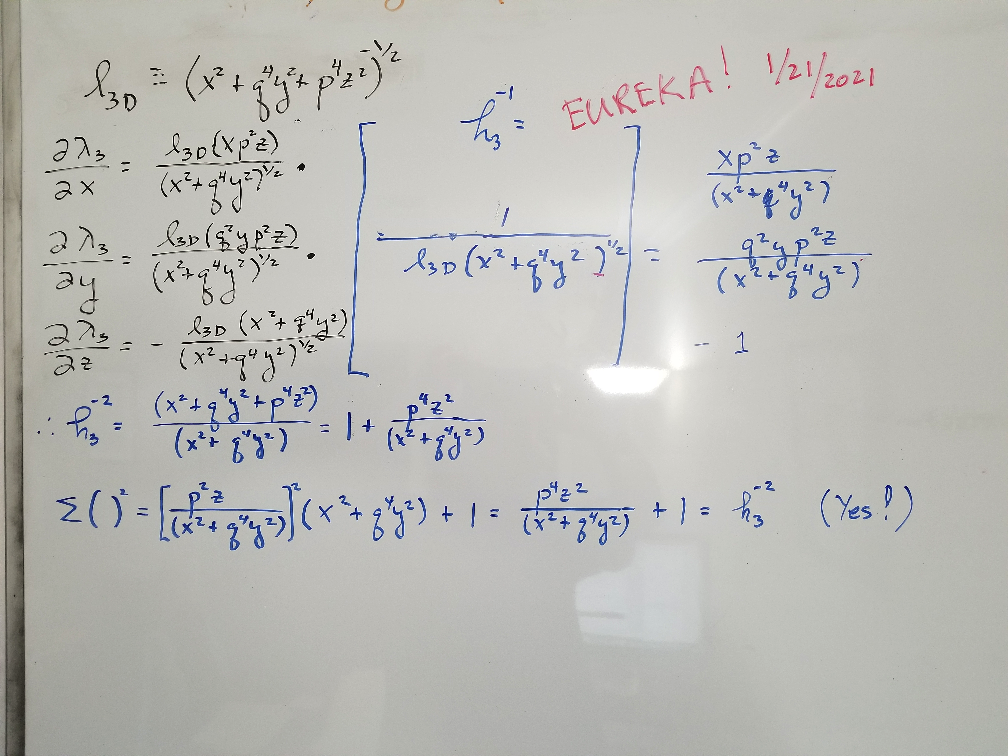 |
After working through various scenarios on my whiteboard today (21 January 2021), I propose that,
|
<math>~\frac{\partial \lambda_3}{\partial x}</math> |
<math>~=</math> |
<math>~\frac{xp^2z}{(x^2 + q^4y^2)} \, ;</math> |
<math>~\frac{\partial \lambda_3}{\partial y}</math> |
<math>~=</math> |
<math>~\frac{q^2 y p^2z}{(x^2 + q^4y^2)} \, ;</math> |
and |
<math>~\frac{\partial \lambda_3}{\partial z}</math> |
<math>~=</math> |
<math>~-1 \, .</math> |
This means that,
|
<math>~h_3^{-2}</math> |
<math>~=</math> |
<math>~\sum_{i=1}^3 \biggl( \frac{\partial \lambda_3}{\partial x_i}\biggr)^2</math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{xp^2z}{(x^2 + q^4y^2)} \biggr]^2 + \biggl[ \frac{q^2 y p^2z}{(x^2 + q^4y^2)} \biggr]^2 + \biggl[ -1 \biggr]^2 </math> |
|
|
<math>~=</math> |
<math>~ \frac{p^4z^2(x^2 + q^4y^2)}{(x^2 + q^4y^2)^2} + 1 </math> |
|
|
<math>~=</math> |
<math>~ \frac{(x^2 + q^4y^2 +p^4z^2)}{(x^2 + q^4y^2)} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{\ell_{3D}^2 (x^2 + q^4y^2)} </math> |
|
<math>~\Rightarrow~~~ h_3</math> |
<math>~=</math> |
<math>~ \ell_{3D} (x^2 + q^4y^2)^{1 / 2} \, . </math> |
This seems to work well because, when combined with the three separate expressions for <math>~\partial \lambda_3/\partial x_i</math>, this single expression for <math>~h_3</math> generates all three components of the third unit vector, that is, all three direction cosines, <math>~\gamma_{3i}</math>. All of the elements of this new "EUREKA moment" result have been entered into the following table.
| Direction Cosine Components for T8 Coordinates | |||||||||||
| <math>~n</math> | <math>~\lambda_n</math> | <math>~h_n</math> | <math>~\frac{\partial \lambda_n}{\partial x}</math> | <math>~\frac{\partial \lambda_n}{\partial y}</math> | <math>~\frac{\partial \lambda_n}{\partial z}</math> | <math>~\gamma_{n1}</math> | <math>~\gamma_{n2}</math> | <math>~\gamma_{n3}</math> | |||
| <math>~1</math> | <math>~(x^2 + q^2 y^2 + p^2 z^2)^{1 / 2} </math> | <math>~\lambda_1 \ell_{3D}</math> | <math>~\frac{x}{\lambda_1}</math> | <math>~\frac{q^2 y}{\lambda_1}</math> | <math>~\frac{p^2 z}{\lambda_1}</math> | <math>~(x) \ell_{3D}</math> | <math>~(q^2 y)\ell_{3D}</math> | <math>~(p^2z) \ell_{3D}</math> | |||
| <math>~2</math> | <math>~\frac{x}{ y^{1/q^2}}</math> | <math>~\frac{1}{\lambda_2}\biggl[\frac{x q^2 y }{(x^2 + q^4y^2)^{1 / 2}}\biggr] </math> | <math>~\frac{\lambda_2}{x}</math> | <math>~-\frac{\lambda_2}{q^2 y}</math> | <math>~0</math> | <math>~\frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~- \frac{x }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~0</math> | |||
| <math>~3</math> | --- | <math>~\ell_{3D}(x^2 + q^4 y^2)^{1 / 2}</math> | <math>~\frac{xp^2z}{(x^2 + q^4y^2)} </math> | <math>~\frac{q^2 y p^2z}{(x^2 + q^4y^2)}</math> | <math>~-1</math> | <math>~\frac{x p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~\frac{q^2 y p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~-\frac{(x^2 + q^4 y^2)\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | |||
|
|||||||||||
What is the Third Coordinate Function, λ3
The remaining $64,000 question is, "What is the actual expression for <math>~\lambda_3(x, y, z)</math> ? "
Notice that the (partial) derivatives of <math>~\lambda_3</math> with respect to <math>~x</math> and <math>~y</math> may be rewritten, respectively, in the form
|
<math>~\biggl( \frac{q^2 y}{p^2z} \biggr) \frac{\partial \lambda_3}{\partial x}</math> |
<math>~=</math> |
<math>~ \frac{q^2 y}{x(1 + \eta^2)} = \frac{\eta}{(1 + \eta^2)} \, , </math> and, |
|
<math>~\biggl( \frac{x}{p^2z} \biggr) \frac{\partial \lambda_3}{\partial y}</math> |
<math>~=</math> |
<math>~ \frac{q^2y}{x(1 + \eta^2)} = \frac{\eta}{(1 + \eta^2)} \, , </math> |
where,
|
<math>~\eta</math> |
<math>~\equiv</math> |
<math>~ \frac{q^2y}{x} ~~~~\Rightarrow~~ \frac{\partial \ln\eta}{\partial \ln x} = -1 \, , ~~~~\frac{\partial \ln\eta}{\partial \ln y} = +1 \, . </math> |
Then, after searching through the CRC Mathematical Handbook's pages of familiar derivative expressions, we appreciate that
|
<math>~\frac{d}{dx_i} \biggl[\frac{1}{\cosh\gamma}\biggr]</math> |
<math>~=</math> |
<math>~- \biggl[ \frac{\tanh\gamma}{\cosh\gamma} \biggr] \frac{d\gamma}{dx_i} \, .</math> |
Hence, it will be useful to adopt the mapping,
|
<math>~\eta</math> |
<math>~~~\rightarrow~~~</math> |
<math>~\sinh \gamma \, ,</math> |
because the right-hand side of both partial-derivative expressions becomes,
|
<math>~\frac{\eta}{(1+\eta^2)}</math> |
<math>~~~\rightarrow~~~</math> |
<math>~\frac{\sinh\gamma}{\cosh^2\gamma} = \frac{\tanh\gamma}{\cosh\gamma} \, .</math> |
Guess A
In particular, this suggests that we set,
|
<math>~\lambda_3</math> |
<math>~=</math> |
<math>~\frac{A}{\cosh\gamma} \, ,</math> |
where,
|
<math>~\gamma</math> |
<math>~\equiv</math> |
<math>~\sinh^{-1}\eta = \pm \cosh^{-1}[\eta^2 + 1]^{1 / 2} \, .</math> |
In other words,
|
<math>~\lambda_3</math> |
<math>~=</math> |
<math>~A[\eta^2 + 1]^{-1 / 2} \, .</math> |
Let's check the first and second partial derivatives.
|
<math>~\frac{\partial \lambda_3}{\partial x}</math> |
<math>~=</math> |
<math>~ - \frac{A}{2} \biggl[ \frac{2\eta}{(\eta^2 + 1)^{3 / 2}} \biggr] \frac{\partial \eta}{\partial x} </math> |
Guess B
What if,
|
<math>~\lambda_3</math> |
<math>~=</math> |
<math>~\frac{1}{2}\ln(1+\eta^2) \, .</math> |
Then,
|
<math>~\frac{d\lambda_3}{d\eta}</math> |
<math>~=</math> |
<math>~ \frac{\eta}{(1+\eta^2)} \, , </math> |
in which case we find,
|
<math>~\frac{\partial\lambda_3}{\partial x_i} </math> |
<math>~=</math> |
<math>~\frac{d\lambda_3}{d\eta} \cdot \frac{\partial\eta}{\partial x_i}</math> |
which means,
|
<math>~\frac{\partial\lambda_3}{\partial x} </math> |
<math>~=</math> |
<math>~ \frac{\eta}{(1+\eta^2)} \biggl[ - \frac{q^2 y}{x^2} \biggr] = - \frac{x}{(x^2 + q^4y^2)} \biggl[ \frac{q^4 y^2}{x^2} \biggr] \, . </math> |
Guess C
What if,
|
<math>~\lambda_3</math> |
<math>~=</math> |
<math>~\frac{1}{2} \ln(1+\eta^{-2}) \, .</math> |
Then,
|
<math>~\frac{d\lambda_3}{d\eta}</math> |
<math>~=</math> |
<math>~- \frac{\eta^{-3}}{(1+\eta^{-2})} = -\frac{ \eta^{-1}}{(1+\eta^{2})} </math> |
in which case we find,
|
<math>~\frac{\partial\lambda_3}{\partial x_i} </math> |
<math>~=</math> |
<math>~\frac{d\lambda_3}{d\eta} \cdot \frac{\partial\eta}{\partial x_i}</math> |
which means,
|
<math>~\frac{\partial\lambda_3}{\partial x} </math> |
<math>~=</math> |
<math>~ -\frac{\eta^{-1}}{(1+\eta^2)} \biggl[ - \frac{q^2 y}{x^2} \biggr] = \frac{x}{(x^2 + q^4y^2)} \, ; </math> |
and,
|
<math>~\frac{\partial\lambda_3}{\partial y} </math> |
<math>~=</math> |
<math>~ -\frac{\eta^{-1}}{(1+\eta^2)} \biggl[ \frac{q^2 }{x} \biggr] = -\frac{x^2}{y(x^2+ q^4y^2)} </math> |
Inverting Coordinate Relations
In a Plane Perpendicular to the Z-Axis
General Case
At a fixed value of <math>~z = z_0</math>, let's invert the <math>~\lambda_1(x, y)</math> and <math>~\lambda_2(x, y)</math> relations to obtain expressions for <math>~x(\lambda_1, \lambda_2)</math> and <math>~y(\lambda_1, \lambda_2)</math>. Perhaps this will help us determine what the third coordinate expression should be.
We start with,
|
<math>~\lambda_1</math> |
<math>~\equiv</math> |
<math>~(x^2 + q^2y^2 + p^2 z_0^2)^{1 / 2} \, ;</math> |
|
<math>~\lambda_2</math> |
<math>~\equiv</math> |
<math>~\frac{x}{y^{1/q^2}} \, .</math> |
This means that,
|
<math>~\ln y</math> |
<math>~=</math> |
<math>~q^2(\ln x - \ln\lambda_2 ) \, ,</math> |
and,
|
<math>~q^2 y^2</math> |
<math>~=</math> |
<math>~(\lambda_1^2 - p^2z_0^2) - x^2</math> |
|
<math>~\Rightarrow~~~2\ln y</math> |
<math>~=</math> |
<math>~\ln\biggl\{ \frac{1}{q^2} \biggl[ (\lambda_1^2 - p^2z_0^2) - x^2 \biggr] \biggr\} \, .</math> |
Together, this gives,
|
<math>~\ln\biggl\{ \frac{1}{q^2} \biggl[ (\lambda_1^2 - p^2z_0^2) - x^2 \biggr] \biggr\} </math> |
<math>~=</math> |
<math>~2q^2(\ln x - \ln\lambda_2 ) </math> |
|
|
<math>~=</math> |
<math>~\ln\biggl[\frac{x}{\lambda_2} \biggr]^{2q^2}</math> |
|
<math>~\Rightarrow~~~(\lambda_1^2 - p^2z_0^2) - x^2 </math> |
<math>~=</math> |
<math>~q^2\biggl[\frac{x}{\lambda_2} \biggr]^{2q^2} </math> |
|
<math>~\Rightarrow~~~ x^2 +q^2\biggl[\frac{x}{\lambda_2} \biggr]^{2q^2} + (p^2z_0^2 - \lambda_1^2) </math> |
<math>~=</math> |
<math>~0 \, .</math> |
What if Axisymmetric (q2 = 1)
In an axisymmetric configuration, <math>~q^2 = 1</math> and <math>~(\lambda_1^2 - p^2z_0^2) = \varpi^2</math>, so this general expression for <math>~x</math> becomes,
|
<math>~0</math> |
<math>~=</math> |
<math>~ x^2 + \biggl[\frac{x}{\lambda_2} \biggr]^{2} -\varpi^2 </math> |
|
|
<math>~=</math> |
<math>~ x^2\biggl[\frac{1 + \lambda_2^2}{\lambda_2^2}\biggr] -\varpi^2 </math> |
|
<math>~\Rightarrow~~~x</math> |
<math>~=</math> |
<math>~ \varpi \biggl[\frac{\lambda_2^2}{1 + \lambda_2^2}\biggr]^{1 / 2} \, . </math> |
Given that, for axisymmetric systems,
|
<math>~x</math> |
<math>~=</math> |
<math>~\varpi \cos\varphi \, ,</math> |
we conclude that when <math>~q^2 = 1</math>,
|
<math>~\biggl[\frac{\lambda_2^2}{1 + \lambda_2^2}\biggr]^{1 / 2}</math> |
<math>~=</math> |
<math>~\cos\varphi </math> |
|
<math>~\Rightarrow~~~\lambda_2^2</math> |
<math>~=</math> |
<math>~\cot^2\varphi </math> |
What if q2 = 2
For example, if we choose <math>~q^2 = 2</math>, we have a quadratic expression for <math>~x^2</math>, namely,
|
<math>~0 </math> |
<math>~=</math> |
<math>~x^2 + 2\biggl[\frac{x}{\lambda_2} \biggr]^{4} + (p^2z_0^2 - \lambda_1^2) </math> |
|
<math>~\Rightarrow ~~~ 0 </math> |
<math>~=</math> |
<math>~x^{4} + \frac{1}{2} \lambda_2^4~ x^2 + \frac{1}{2}\lambda_2^4(p^2z_0^2 - \lambda_1^2) </math> |
|
<math>~\Rightarrow ~~~ 2x^2 </math> |
<math>~=</math> |
<math>~ - \frac{\lambda_2^4}{2} \pm \biggl[ \frac{\lambda_2^8}{4} - 2\lambda_2^4(p^2z_0^2 - \lambda_1^2) \biggr]^{1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ \frac{\lambda_2^4}{2} \biggl\{ \biggl[ 1 - \frac{8(p^2z_0^2 - \lambda_1^2)}{\lambda_2^4} \biggr]^{1 / 2} - 1 \biggr\} </math> |
|
<math>~\Rightarrow ~~~ x^2 </math> |
<math>~=</math> |
<math>~ \frac{\lambda_2^4}{4} \biggl\{ \biggl[ 1 - \frac{8(p^2z_0^2 - \lambda_1^2)}{\lambda_2^4} \biggr]^{1 / 2} - 1 \biggr\} \, . </math> |
Given that, for <math>~q^2 = 2</math>, one of the two defining expression means, <math>~\lambda_2 = x/\sqrt{y}</math>, we also have,
|
<math>~y </math> |
<math>~=</math> |
<math>~ \frac{\lambda_2^2}{4} \biggl\{ \biggl[ 1 - \frac{8(p^2z_0^2 - \lambda_1^2)}{\lambda_2^4} \biggr]^{1 / 2} - 1 \biggr\} \, . </math> |
New 2nd Coordinate
Apparently it will be cleaner to define a new "2nd coordinate," <math>~\kappa_2</math>, such that,
|
<math>~\kappa_2^{2q^2}</math> |
<math>~\equiv</math> |
<math>~ q^2\biggl[\frac{1}{\lambda_2} \biggr]^{2q^2} </math> |
|
<math>~\Rightarrow~~~\kappa_2</math> |
<math>~\equiv</math> |
<math>~ q^{1/q^2}\biggl[\frac{1}{\lambda_2} \biggr] = q^{1/q^2}\biggl[\frac{y^{1/q^2}}{x} \biggr] = \frac{(qy)^{1/q^2}}{x} \, . </math> |
(With this new definition, <math>~\kappa_2 \sim \tan\varphi</math>; it is exactly this when <math>~q^2 = 1</math>.) Then we can rewrite the last expression from the above general case as,
|
<math>~x^2 +(\kappa_2 x)^{2q^2} - (\lambda_1^2 - p^2z_0^2 ) </math> |
<math>~=</math> |
<math>~0 \, .</math> |
When <math>~q=1</math> (the axisymmetric case), this gives,
|
<math>~x^2(1+\kappa_2^2)</math> |
<math>~=</math> |
<math>~ (\lambda_1^2 - p^2z_0^2 ) </math> |
|
<math>~\Rightarrow~~~\frac{x^2}{(\lambda_1^2 - p^2z_0^2 ) }</math> |
<math>~=</math> |
<math>~ \frac{1}{(1+\kappa_2^2)} \, ,</math> |
which means that <math>~\kappa_2 = \tan\varphi</math>. And, for the case of <math>~q^2 = 2</math>, after making the substitution,
we find,
|
<math>~x^2 </math> |
<math>~=</math> |
<math>~ \frac{\lambda_2^4}{4} \biggl\{ \biggl[ 1 - \frac{8(p^2z_0^2 - \lambda_1^2)}{\lambda_2^4} \biggr]^{1 / 2} - 1 \biggr\} \, . </math> |
|
<math>~\Rightarrow~~~ x^2 </math> |
<math>~=</math> |
<math>~ \frac{1}{2\kappa_2^4} \biggl\{ \biggl[ 1 + 4\kappa_2^4(\lambda_1^2 - p^2z_0^2 ) \biggr]^{1 / 2} - 1 \biggr\} \, , </math> |
and,
|
<math>~y = \biggl(\frac{\kappa_2^2}{2^{1 / 2}} \biggr) x^2 </math> |
<math>~=</math> |
<math>~\biggl(\frac{\kappa_2^2}{2^{1 / 2}} \biggr) \frac{1}{2\kappa_2^4} \biggl\{ \biggl[ 1 + 4\kappa_2^4(\lambda_1^2 - p^2z_0^2 ) \biggr]^{1 / 2} - 1 \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{2^{3 / 2}\kappa_2^2} \biggl\{ \biggl[ 1 + 4\kappa_2^4(\lambda_1^2 - p^2z_0^2 ) \biggr]^{1 / 2} - 1 \biggr\} \, . </math> |
Angle Between Unit Vectors
We begin by restating that the coordinate, scale factor, and unit vector associated with the normal to our ellipsoidal surface are,
|
<math>~\lambda_1</math> |
<math>~\equiv</math> |
<math>~(x^2 + q^2y^2 + p^2z^2)^{1 / 2} \, ,</math> |
|
<math>~h_1</math> |
<math>~=</math> |
<math>~\lambda_1 \ell_{3D} \, ,</math> |
|
<math>~\hat{e}_1</math> |
<math>~=</math> |
<math>~ \hat\imath (x\ell_{3D} ) + \hat\jmath (q^2 y\ell_{3D} ) + \hat{k} (p^2z\ell_{3D} ) \, . </math> |
In the Table below titled, "Direction Cosines Components for κ8 Coordinates", there are two fully-formed unit vectors that are each orthogonal to the (first) unit vector that is normal to the ellipsoid's surface. Here we will refer to the coordinates of these two fully-formed unit vectors as,
|
<math>~\lambda_2</math> |
<math>~\equiv</math> |
<math>~\frac{xy^{1/q^2}}{z^{2/p^2}}</math> |
and, |
<math>~\kappa_2</math> |
<math>~\equiv</math> |
<math>~\frac{(qy)^{1/q^2}}{x} \, .</math> |
The associated scale factors and unit vectors are given by the following expressions:
|
<math>~h_{\lambda_2}^{-2}</math> |
<math>~=</math> |
<math>~ \biggl(\frac{\partial\lambda_2}{\partial x} \biggr)^2 + \biggl(\frac{\partial\lambda_2}{\partial y} \biggr)^2 + \biggl(\frac{\partial\lambda_2}{\partial z} \biggr)^2 </math> |
|
|
<math>~=</math> |
<math>~ \biggl(\frac{\lambda_2}{x} \biggr)^2 + \biggl(\frac{\lambda_2}{q^2y} \biggr)^2 + \biggl(- \frac{2\lambda_2}{p^2z} \biggr)^2 </math> |
|
|
<math>~=</math> |
<math>~ \lambda_2^2 \biggl[ \frac{q^4y^2p^4z^2 + x^2p^4z^2 + 4x^2 q^4y^2}{x^2 q^4y^2 p^4z^2} \biggr] </math> |
|
<math>~\Rightarrow ~~~ h_{\lambda_2}</math> |
<math>~=</math> |
<math>~ \frac{x q^2y p^2z}{\lambda_2 \mathcal{D}} </math> |
|
<math>~\Rightarrow ~~~ \hat{e}_{\lambda_2}</math> |
<math>~=</math> |
<math>~ \hat\imath \biggl[ h_{\lambda_2} \biggl(\frac{\partial \lambda_2}{\partial x}\biggr)\biggr] + \hat\jmath \biggl[ h_{\lambda_2} \biggl(\frac{\partial \lambda_2}{\partial y}\biggr)\biggr] + \hat{k} \biggl[ h_{\lambda_2} \biggl(\frac{\partial \lambda_2}{\partial z}\biggr)\biggr] </math> |
|
|
<math>~=</math> |
<math>~ \hat\imath \biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] + \hat\jmath \biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] - \hat{k} \biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] \, . </math> |
|
With regard to orthogonality, note that,
|
And,
|
<math>~h_{\kappa_2}^{-2}</math> |
<math>~=</math> |
<math>~ \biggl(\frac{\partial\kappa_2}{\partial x} \biggr)^2 + \biggl(\frac{\partial\kappa_2}{\partial y} \biggr)^2 + \biggl(\frac{\partial\kappa_2}{\partial z} \biggr)^2 </math> |
|
|
<math>~=</math> |
<math>~ \biggl(-\frac{\kappa_2}{x} \biggr)^2 + \biggl(\frac{\kappa_2}{q^2 y} \biggr)^2 = \frac{\kappa_2^2}{x^2q^4y^2}\biggl[x^2 + q^4y^2\biggr] </math> |
|
<math>~\Rightarrow~~~h_{\kappa_2}</math> |
<math>~=</math> |
<math>~ \frac{xq^2y}{\kappa_2 (x^2 + q^4y^2)^{1 / 2}} </math> |
|
<math>~\Rightarrow~~~\hat{e}_{\kappa_2}</math> |
<math>~=</math> |
<math>~ -~\hat\imath \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}}\biggr] + \hat\jmath \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] + \hat{k} \biggl[ 0 \biggr] \, . </math> |
|
Again, note that with regard to orthogonality,
|
From this pair of orthogonality checks, we appreciate that both unit vectors always lie in the plane that is tangent to the surface of our ellipsoid. Next, let's determine the angle, <math>~\alpha</math>, between these two unit vectors as measured in the relevant tangent-plane.
|
<math>~\cos\alpha \equiv \hat{e}_{\lambda_2} \cdot \hat{e}_{\kappa_2}</math> |
<math>~=</math> |
<math>~ \biggl\{ \hat\imath \biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] + \hat\jmath \biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] - \hat{k} \biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] \biggr\} \biggl\{ -\hat\imath \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}}\biggr] + \hat\jmath \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] + \hat{k} \biggl[ 0 \biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ -\biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}}\biggr]\biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] + \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr]\biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{p^2 z}{\mathcal{D} (x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ x^2 - q^4y^2 \biggr] \, . </math> |
Let's again visit the unit vector that we know lies in the tangent-plane and is always orthogonal to <math>~\lambda_2</math>, namely,
|
<math>~\hat{e}_{\lambda_3}</math> |
<math>~=</math> |
<math>~ -~\hat\imath \biggl[ x(2q^4 y^2 + p^4z^2 )\biggr]\frac{\ell_{3D}}{\mathcal{D}} + \hat\jmath \biggl[q^2y(p^4z^2 + 2x^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} + \hat{k} \biggl[p^2z (x^2 - q^4y^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} \, . </math> |
We acknowledge that,
|
<math>~\hat{e}_{\lambda_2} \cdot \hat{e}_{\lambda_3}</math> |
<math>~=</math> |
<math>~ \biggl\{ \hat\imath \biggl[ \frac{q^2y p^2z}{\mathcal{D}} \biggr] + \hat\jmath \biggl[ \frac{xp^2z}{\mathcal{D}} \biggr] - \hat{k} \biggl[ \frac{2x q^2y }{\mathcal{D}} \biggr] \biggr\} \biggl\{ -~\hat\imath \biggl[ x(2q^4 y^2 + p^4z^2 )\biggr]\frac{\ell_{3D}}{\mathcal{D}} + \hat\jmath \biggl[q^2y(p^4z^2 + 2x^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} + \hat{k} \biggl[p^2z (x^2 - q^4y^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ -~\biggl[ xq^2yp^2z(2q^4 y^2 + p^4z^2 )\biggr]\frac{\ell_{3D}}{\mathcal{D}^2} + \biggl[x q^2y p^2z(p^4z^2 + 2x^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}^2} - \biggl[2xq^2y p^2z (x^2 - q^4y^2) \biggr]\frac{\ell_{3D}}{\mathcal{D}^2} </math> |
|
|
<math>~=</math> |
<math>~\biggl[ -(2q^4 y^2 + p^4z^2 ) + (p^4z^2 + 2x^2) - 2 (x^2 - q^4y^2) \biggr] \frac{(x q^2y p^2z) \ell_{3D}}{\mathcal{D}^2} </math> |
|
|
<math>~=</math> |
<math>~ 0 \, . </math> |
Kappa (κ8) Coordinates
|
<math>~\kappa_1</math> |
<math>~\equiv</math> |
<math>~(x^2 + q^2y^2 + p^2 z^2)^{1 / 2} \, ;</math> |
|
<math>~\kappa_3</math> |
<math>~\equiv</math> |
<math>~\tan^{-1}\biggl[ \frac{(qy)^{1/q^2}}{x} \biggr] \, .</math> |
|
<math>~\frac{\partial\kappa_3}{\partial x}</math> |
<math>~=</math> |
<math>~ \biggl[1 + \frac{(qy)^{2/q^2}}{x^2} \biggr]^{-1} \biggl[- \frac{(qy)^{1/q^2}}{x^2} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ -\frac{\sin^2\kappa_3}{(qy)^{1/q^2}} = -\frac{\sin^2\kappa_3}{x\tan\kappa_3} </math> |
|
|
<math>~=</math> |
<math>~ -\frac{\sin\kappa_3 \cos\kappa_3}{x}\, . </math> |
|
<math>~\frac{\partial\kappa_3}{\partial y}</math> |
<math>~=</math> |
<math>~ \biggl[1 + \frac{(qy)^{2/q^2}}{x^2} \biggr]^{-1} \biggl[\frac{q^{1/q^2} y^{1/q^2}}{q^2 x y} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[1 + \frac{(qy)^{2/q^2}}{x^2} \biggr]^{-1} \biggl[\frac{(qy)^{1/q^2}}{x } \biggr] \frac{1}{q^2y} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{q^2y}\biggl[\frac{\tan\kappa_3}{1 + \tan^2\kappa_3 }\biggr]
</math> |
|
|
<math>~=</math> |
<math>~ + \frac{\sin\kappa_3 \cos\kappa_3}{q^2y} \, . </math> |
Therefore,
|
<math>~h_3^{-2}</math> |
<math>~=</math> |
<math>~ \biggl[-\frac{\sin\kappa_3 \cos\kappa_3}{x} \biggr]^2 + \biggl[\frac{\sin\kappa_3 \cos\kappa_3}{q^2y} \biggr]^2 </math> |
|
|
<math>~=</math> |
<math>~(x^2 + q^4y^2) \biggl[\frac{\sin\kappa_3 \cos\kappa_3}{xq^2y} \biggr]^2 </math> |
|
<math>~\Rightarrow~~~ h_3</math> |
<math>~=</math> |
<math>~(x^2 + q^4y^2)^{-1 / 2} \biggl[\frac{xq^2y}{\sin\kappa_3 \cos\kappa_3} \biggr] \, . </math> |
| Direction Cosine Components for κ8 Coordinates | ||||||||||||||
| <math>~n</math> | <math>~\kappa_n</math> | <math>~h_n</math> | <math>~\frac{\partial \kappa_n}{\partial x}</math> | <math>~\frac{\partial \kappa_n}{\partial y}</math> | <math>~\frac{\partial \kappa_n}{\partial z}</math> | <math>~\gamma_{n1}</math> | <math>~\gamma_{n2}</math> | <math>~\gamma_{n3}</math> | ||||||
| <math>~1</math> | <math>~(x^2 + q^2 y^2 + p^2 z^2)^{1 / 2} </math> | <math>~\kappa_1 \ell_{3D}</math> | <math>~\frac{x}{\kappa_1}</math> | <math>~\frac{q^2 y}{\kappa_1}</math> | <math>~\frac{p^2 z}{\kappa_1}</math> | <math>~(x) \ell_{3D}</math> | <math>~(q^2 y)\ell_{3D}</math> | <math>~(p^2z) \ell_{3D}</math> | ||||||
| <math>~2</math> | --- | <math>~\ell_{3D}(x^2 + q^4 y^2)^{1 / 2}</math> | <math>~\frac{xp^2z}{(x^2 + q^4y^2)} </math> | <math>~\frac{q^2 y p^2z}{(x^2 + q^4y^2)}</math> | <math>~-1</math> | <math>~\frac{x p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~\frac{q^2 y p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~-\frac{(x^2 + q^4 y^2)\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | ||||||
| <math>~3</math> | <math>~\tan^{-1}\biggl[ \frac{(qy)^{1/q^2}}{x} \biggr]</math> | <math>~\frac{1}{\sin\kappa_3 \cos\kappa_3}\biggl[\frac{x q^2 y }{(x^2 + q^4y^2)^{1 / 2}}\biggr] </math> | <math>~-\frac{\sin\kappa_3 \cos\kappa_3}{x}</math> | <math>~\frac{\sin\kappa_3 \cos\kappa_3}{q^2 y}</math> | <math>~0</math> | <math>~- \frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~\frac{x }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~0</math> | ||||||
|
||||||||||||||
| <math>~4</math> | <math>~\frac{x y^{1/q^2}}{ z^{2/p^2}}</math> | <math>~\frac{1}{\kappa_4}\biggl[\frac{x q^2 y p^2 z}{ \mathcal{D}}\biggr] </math> | <math>~\frac{\kappa_4}{x}</math> | <math>~\frac{\kappa_4}{q^2 y}</math> | <math>~-\frac{2\kappa_4}{p^2 z}</math> | <math>~\frac{x q^2 y p^2 z}{\mathcal{D}} \biggl(\frac{1}{x}\biggr)</math> | <math>~ \frac{x q^2 y p^2 z}{\mathcal{D}} \biggl(\frac{1}{q^2y}\biggr)</math> | <math>~\frac{x q^2 y p^2 z}{\mathcal{D}} \biggl(-\frac{2}{p^2z}\biggr)</math> | ||||||
| <math>~5</math> | --- | --- | --- | --- | --- | <math>~-\frac{\ell_{3D}}{\mathcal{D}}\biggl[ x(2 q^4y^2 + p^4z^2 ) \biggl]</math> | <math>~\frac{\ell_{3D}}{\mathcal{D}}\biggl[ q^2 y(p^4z^2 + 2x^2 ) \biggl]</math> | <math>~\frac{\ell_{3D}}{\mathcal{D}}\biggl[ p^2z( x^2 - q^4y^2 ) \biggl]</math> | ||||||
Also note …
and the partial derivatives of <math>~A</math> and <math>~B</math> are detailed in an accompanying discussion. |
||||||||||||||
The direction-cosines of the second unit vector — as has already been inserted into the "κ8 coordinates" table — should be obtainable from the first and third unit vectors via the cross product,
|
<math>~\hat{e}_2 = \hat{e}_3 \times \hat{e}_1</math> |
<math>~=</math> |
<math>~ \hat\imath \biggl[ e_{3y}e_{1z} - e_{3z} e_{1y} \biggr] + \hat\jmath \biggl[ e_{3z}e_{1x} - e_{3x} e_{1z} \biggr] + \hat{k} \biggl[ e_{3x}e_{1y} - e_{3y} e_{1x} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \hat\imath \biggl[ \frac{x (p^2z) \ell_{3D} }{(x^2 + q^4y^2)^{1 / 2}} \biggr] + \hat\jmath \biggl[ \frac{q^2 y (p^2z) \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}}\biggr] - \hat{k} \biggl[ \frac{(x^2 + q^4y^2) \ell_{3D} }{(x^2 + q^4y^2)^{1 / 2}} \biggr] \, . </math> |
The other boxes in the n = 2 row have been drawn from our accompanying EUREKA! moment and the n = 3 row of the table that details "Direction Cosine Components for T8 Coordinates."
Attempt 1
Let's try …
|
<math>~\kappa_2</math> |
<math>~\equiv</math> |
<math>~\tan^{-1}\biggl[ \frac{x(qy)^{1/q^2}}{(pz)^{1/p^2}} \biggr] \, ,</math> |
which leads to,
|
<math>~\frac{\partial\kappa_3}{\partial x}</math> |
<math>~=</math> |
<math>~ \biggl[1 + \frac{x^2(qy)^{2/q^2}}{(pz)^{2/p^2}} \biggr]^{-1} \biggl[ \frac{(qy)^{1/q^2}}{(pz)^{1/p^2}} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{x}\biggl[1+\tan^2\kappa_2\biggr]^{-1} \tan\kappa_2 = \frac{\sin\kappa_2 \cos\kappa_2}{x} \, ; </math> |
|
<math>~\frac{\partial\kappa_3}{\partial y}</math> |
<math>~=</math> |
<math>~ \biggl[1 + \frac{x^2(qy)^{2/q^2}}{(pz)^{2/p^2}} \biggr]^{-1} \biggl[ \frac{xq^{1/q^2}(y)^{1/q^2}}{q^2 y(pz)^{1/p^2}} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{q^2y}\biggl[1+\tan^2\kappa_2\biggr]^{-1} \tan\kappa_2 = \frac{\sin\kappa_2 \cos\kappa_2}{q^2y} \, ; </math> |
|
<math>~\frac{\partial\kappa_3}{\partial z}</math> |
<math>~=</math> |
<math>~ - \frac{\sin\kappa_2 \cos\kappa_2}{p^2z} \, . </math> |
Hence,
|
<math>~h_2^{-2}</math> |
<math>~=</math> |
<math>~\sin^2\kappa_2 \cos^2\kappa_2 \biggl[ \frac{1}{x^2} + \frac{1}{q^4y^2} + \frac{1}{p^4z^2} \biggr]</math> |
|
|
<math>~=</math> |
<math>~ \sin^2\kappa_2 \cos^2\kappa_2 \biggl[ \frac{x^2 + q^4 y^2 + p^4 z^2}{x^2 q^4y^2 p^4 z^2} \biggr] = \biggl[ \frac{\sin^2\kappa_2 \cos^2\kappa_2 }{x^2 q^4y^2 p^4 z^2 \ell_{3D}^2} \biggr] </math> |
|
<math>~\Rightarrow~~~ h_2</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x q^2y p^2 z \ell_{3D}}{\sin\kappa_2 \cos\kappa_2 } \biggr] \, . </math> |
The three direction-cosines are, then,
|
<math>~\gamma_{21} = h_2 \biggl(\frac{\partial \kappa_2}{\partial x}\biggr)</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x q^2y p^2 z \ell_{3D}}{\sin\kappa_2 \cos\kappa_2 } \biggr] \frac{\sin\kappa_2 \cos\kappa_2}{x} = q^2y p^2z \ell_{3D} \, . </math> |
T9 Coordinates
Establish 1st and 3rd Coordinates
|
<math>~\lambda_1</math> |
<math>~\equiv</math> |
<math>~(x^2 + q^2y^2 + p^2z^2)^{1 / 2} \, ,</math> |
|
<math>~\lambda_3</math> |
<math>~\equiv</math> |
<math>~ \biggl[ \frac{q^2y}{x^{q^2}} \biggr]^{1/(q^2-1)} = q^{2/(q^2-1)} y^{1/(q^2-1)} x^{ q^2/(1-q^2) } \, . </math> |
|
<math>~\frac{\partial \lambda_3}{\partial x}</math> |
<math>~=</math> |
<math>~ \biggl( \frac{q^2}{1-q^2} \biggr) \frac{\lambda_3}{x} \, , </math> |
|
<math>~\frac{\partial \lambda_3}{\partial y}</math> |
<math>~=</math> |
<math>~ \biggl( \frac{1}{q^2 - 1} \biggr) \frac{\lambda_3}{y} \, , </math> |
|
<math>~h_3^{-2}</math> |
<math>~=</math> |
<math>~ \biggl[ \biggl( \frac{q^2}{1 - q^2 } \biggr) \frac{\lambda_3}{x} \biggr]^2 + \biggl[ \biggl( \frac{1}{q^2 - 1} \biggr) \frac{\lambda_3}{y} \biggr]^2 = \frac{\lambda_3^2}{(q^2-1)^2}\biggl[ \frac{x^2 + q^4y^2 }{x^2 y^2} \biggr] </math> |
|
<math>~\Rightarrow~~~ h_3</math> |
<math>~=</math> |
<math>~ \frac{(q^2-1)}{\lambda_3}\biggl[ \frac{x y}{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, . </math> |
Hence, the three direction-cosines are,
|
<math>~\gamma_{31} = h_3 \biggl(\frac{\partial \lambda_3}{\partial x} \biggr)</math> |
<math>~=</math> |
<math>~ \frac{(q^2-1)}{\lambda_3}\biggl[ \frac{x y}{(x^2 + q^4y^2)^{1 / 2} } \biggr]\biggl( \frac{q^2}{1-q^2} \biggr) \frac{\lambda_3}{x} = - \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, , </math> |
|
<math>~\gamma_{32} = h_3 \biggl(\frac{\partial \lambda_3}{\partial y} \biggr)</math> |
<math>~=</math> |
<math>~ \frac{(q^2-1)}{\lambda_3}\biggl[ \frac{x y}{(x^2 + q^4y^2)^{1 / 2} } \biggr]\biggl( \frac{1}{q^2 - 1} \biggr) \frac{\lambda_3}{y} = \biggl[ \frac{x }{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, . </math> |
And the position vector is given by the expression,
|
<math>~\mathbf{\vec{x}}</math> |
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 (\gamma_{11} x + \gamma_{12} y + \gamma_{13} z) + \mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) + \mathbf{\hat{e}}_3 (\gamma_{31} x + \gamma_{32} y + \gamma_{33} z) </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl[ x^2\ell_{3D} + q^2 y^2 \ell_{3D} + p^2 z^2 \ell_{3D} \biggr] + \mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) + \mathbf{\hat{e}}_3 \biggl[- \frac{q^2xy}{(x^2 + q^4y^2)^{1 / 2} } + \frac{xy }{(x^2 + q^4y^2)^{1 / 2} } \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl[ \lambda_1^2 \ell_{3D} \biggr] + \mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) - \mathbf{\hat{e}}_3 \biggl[\frac{(q^2-1) xy }{(x^2 + q^4y^2)^{1 / 2} } \biggr] \, . </math> |
Guess 2nd Coordinate
Unspecified Coefficients
|
<math>~\lambda_2</math> |
<math>~\equiv</math> |
<math>~(ax^2 + by^2 + ez^2)^{-1 / 2} (ax^2 + by^2)^{1 / 2}</math> |
|
|
<math>~=</math> |
<math>~\biggl[\frac{(ax^2 + by^2)}{(ax^2 + by^2 + ez^2)}\biggr]^{1 / 2}</math> |
|
|
<math>~=</math> |
<math>~\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-1 / 2} \, .</math> |
|
<math>~\frac{\partial \lambda_2}{\partial x}</math> |
<math>~=</math> |
<math>~ -\frac{1}{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \frac{\partial}{\partial x}\biggl[ez^2 (ax^2 + by^2)^{-1 } \biggr] </math> |
|
|
<math>~=</math> |
<math>~ -\frac{ez^2 }{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \biggl( -1\biggr) \biggl[(ax^2 + by^2)^{-2} \biggr]2ax </math> |
|
|
<math>~=</math> |
<math>~ \frac{a x e z^2 }{(ax^2 + by^2)^{2}}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} </math> |
|
|
<math>~=</math> |
<math>~ \frac{a x e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \, , </math> |
|
<math>~\frac{\partial \lambda_2}{\partial y}</math> |
<math>~=</math> |
<math>~ -\frac{1}{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \frac{\partial}{\partial y}\biggl[ez^2 (ax^2 + by^2)^{-1} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ -\frac{ez^2 }{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \biggl( -1\biggr) \biggl[(ax^2 + by^2)^{-2} \biggr]2by </math> |
|
|
<math>~=</math> |
<math>~ \frac{by e z^2 }{(ax^2 + by^2)^{2}}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} </math> |
|
|
<math>~=</math> |
<math>~ \frac{b y e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \, , </math> |
|
<math>~\frac{\partial \lambda_2}{\partial z}</math> |
<math>~=</math> |
<math>~ -\frac{1}{2}\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-3 / 2} \frac{\partial}{\partial z}\biggl[ez^2 (ax^2 + by^2)^{-1 } \biggr] </math> |
|
|
<math>~=</math> |
<math>~ -\frac{ez ~ \lambda_2^3}{(ax^2 + by^2)} \, . </math> |
Hence,
|
<math>~h_2^{-2}</math> |
<math>~=</math> |
<math>~ \biggl[\frac{a x e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}}\biggr]^2 + \biggl[ \frac{b y e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \biggr]^2 + \biggl[\frac{ez ~ \lambda_2^3}{(ax^2 + by^2)} \biggr]^2 </math> |
|
|
<math>~=</math> |
<math>~ \biggl[\frac{e z ~\lambda_2^3}{(ax^2 + by^2)^{2}}\biggr]^2 \biggl[ (a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr] </math> |
|
<math>~\Rightarrow~~~ h_2</math> |
<math>~=</math> |
<math>~ \biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} \, . </math> |
The three direction-cosines are, then,
|
<math>~\gamma_{21} = h_2 \biggl(\frac{\partial\lambda_2}{\partial x} \biggr)</math> |
<math>~=</math> |
<math>~ \frac{a x e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ axz \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} </math> |
|
<math>~\gamma_{22} = h_2 \biggl(\frac{\partial\lambda_2}{\partial y} \biggr)</math> |
<math>~=</math> |
<math>~ \frac{b y e z^2 ~\lambda_2^3}{(ax^2 + by^2)^{2}} \biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ byz \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} </math> |
|
<math>~\gamma_{23} = h_2 \biggl(\frac{\partial\lambda_2}{\partial z} \biggr)</math> |
<math>~=</math> |
<math>~ -\frac{ez ~ \lambda_2^3}{(ax^2 + by^2)} \biggl[\frac{(ax^2 + by^2)^{2}}{e z ~\lambda_2^3}\biggr] \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ -(ax^2 + by^2) \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} \, . </math> |
| Direction Cosine Components for T9 Coordinates | |||||||||||||||||
| <math>~n</math> | <math>~\lambda_n</math> | <math>~h_n</math> | <math>~\frac{\partial \lambda_n}{\partial x}</math> | <math>~\frac{\partial \lambda_n}{\partial y}</math> | <math>~\frac{\partial \lambda_n}{\partial z}</math> | <math>~\gamma_{n1}</math> | <math>~\gamma_{n2}</math> | <math>~\gamma_{n3}</math> | |||||||||
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | |||||||||
| <math>~1</math> | <math>~(x^2 + q^2 y^2 + p^2 z^2)^{1 / 2} </math> | <math>~\lambda_1 \ell_{3D}</math> | <math>~\frac{x}{\lambda_1}</math> | <math>~\frac{q^2 y}{\lambda_1}</math> | <math>~\frac{p^2 z}{\lambda_1}</math> | <math>~(x) \ell_{3D}</math> | <math>~(q^2 y)\ell_{3D}</math> | <math>~(p^2z) \ell_{3D}</math> | |||||||||
| <math>~2A</math> | --- | <math>~\ell_{3D}(x^2 + q^4 y^2)^{1 / 2}</math> | <math>~\frac{xp^2z}{(x^2 + q^4y^2)} </math> | <math>~\frac{q^2 y p^2z}{(x^2 + q^4y^2)}</math> | <math>~-1</math> | <math>~\frac{x p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~\frac{q^2 y p^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~-\frac{(x^2 + q^4 y^2)\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> | |||||||||
| <math>~2B</math> | <math>~\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-1 / 2}</math> | <math>~\biggl[\frac{(ax^2 + by^2)^2}{ez~\lambda_2^3} \biggr]\mathfrak{J}_{2\mathrm{B}}</math> | <math>~\frac{ax~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math> | <math>~\frac{by~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math> | <math>~- \frac{ez ~\lambda_2^3}{(ax^2 + by^2)} </math> | <math>~axz ~\mathfrak{J}_{2\mathrm{B}}</math> | <math>~byz ~\mathfrak{J}_{2\mathrm{B}}</math> | <math>~-(ax^2 + by^2)~\mathfrak{J}_{2\mathrm{B}} </math> | |||||||||
| <math>~3</math> | <math>~\biggl[ \frac{q^2y}{x^{q^2}} \biggr]^{1/(q^2-1)}</math> | <math>~\frac{(q^2-1)x y }{\lambda_3(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~- \biggl( \frac{q^2}{q^2 - 1} \biggr) \frac{\lambda_3}{x}</math> | <math>~\biggl( \frac{q^2}{q^2 - 1} \biggr) \frac{\lambda_3}{q^2y}</math> | <math>~0</math> | <math>~- \frac{q^2 y }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~\frac{x }{(x^2 + q^4y^2)^{1 / 2}} </math> | <math>~0</math> | |||||||||
|
|||||||||||||||||
This table titled, "Direction Cosine Components for T9 Coordinates," contains the following information:
- The (first) row labeled, <math>~n = 1</math>, correctly details the scale-factor, <math>~h_1</math>, and the unit vector expression, <math>~\hat{e}_1 = (\hat\imath \gamma_{11} + \hat\jmath \gamma_{12} + \hat{k} \gamma_{13})</math>, that result from the given specification of the <math>~\lambda_1</math> coordinate. By design, the unit vector, <math>~\hat{e}_1</math>, is everywhere normal to the "surface" of the ellipsoid.
- The (fourth) row labeled, <math>~n = 3</math>, correctly details the scale-factor, <math>~h_3</math>, and the unit vector expression, <math>~\hat{e}_3 = (\hat\imath \gamma_{31} + \hat\jmath \gamma_{32} + \hat{k} \gamma_{33})</math>, that result from the given specification of the <math>~\lambda_2</math> coordinate. By design, this unit vector, <math>~\hat{e}_3</math>, has no vertical component — that is, <math>~\gamma_{33} = 0</math> — and, by design, it is everywhere perpendicular to the "surface-normal" unit vector, <math>~\hat{e}_1</math>.
-
We desire a unit vector, <math>~\hat{e}_2</math>, that is mutually orthogonal to the other two unit vectors; this has been accomplished by examining their cross-product, namely, we have set <math>~\hat{e}_{2\mathrm{A}} = \hat{e}_3 \times \hat{e}_1</math>. Determined in this manner, the expressions for the three direction-cosine components of <math>~\hat{e}_{2\mathrm{A}}</math> have been written in the last three columns of the (second) row labeled, <math>~n=2\mathrm{A}</math>. While we are confident that the correct specification of <math>~\hat{e}_2</math> is,
<math>~\hat{e}_{2\mathrm{A}} = (\hat\imath \gamma_{21} + \hat\jmath \gamma_{22} + \hat{k} \gamma_{23})</math>
<math>~=</math>
<math>~ \hat\imath (xp^2 z)\mathfrak{J}_0 + \hat\jmath (q^2y p^2 z)\mathfrak{J}_0 - \hat{k} (x^2 + q^4y^2) \mathfrak{J}_0 \, , </math>
as yet (18 February 2021), we have been unable to determine an expression for the coordinate, <math>~\lambda_{2\mathrm{A}}(x, y, z)</math>, from which all three of these direction-cosine expressions can be simultaneously derived — hence, the dashes in the second column of row 2A. The expression for <math>~h_{2\mathrm{A}}</math> that has been presented in the third column of row 2A (and framed in pink) is a guess which, when divided into each of the three direction cosines, gives respectively the three guessed partial-derivative expressions shown in columns 4, 5, and 6 of row 2A.
- The second column of row 2B contains a guess (framed in yellow) for the coordinate expression, <math>~\lambda_{2\mathrm{B}}</math>; this expression contains three unspecified scalar coefficients, <math>~a</math>, <math>~b</math> and <math>~e</math>. The remaining columns of this row contain the three partial derivatives, the associated scale factor, and the three direction cosines that result from this guessed coordinate expression. If we can find values of the three scalar coefficients that give (row 2B) expressions for the three direction cosines that perfectly match the direction cosines written in row 2A, then we will be able to state that <math>~\lambda_{2\mathrm{B}}</math> is — at least one form of — our sought-after third coordinate expression.
Yellow-Framed Guess 2B
Referencing the 2B table row, above, we are looking for coefficient values that map,
|
<math>~axz \rightarrow xp^2 z \, ,</math> |
<math>~byz \rightarrow q^2 yp^2 z \, ,</math> |
<math>~(ax^2 + by^2) \rightarrow (x^2 + q^4y^2) \, ,</math> |
and that map,
|
<math>~ \mathfrak{J}_{2\mathrm{B}} \equiv \biggl[(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2 \biggr]^{-1 / 2} </math> |
<math>~=</math> |
<math>~ \biggl[z^2(a^2 x^2 + b^2 y^2) + (ax^2 + by^2)^2 \biggr]^{-1 / 2} \, , </math> |
into the expression,
|
<math>~\mathfrak{J}_0 \equiv \frac{\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}}</math> |
<math>~=</math> |
<math>~ \biggl[ (x^2 + q^4y^2 + p^4 z^2)(x^2 + q^4 y^2) \biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ p^4 z^2(x^2 + q^4 y^2) + (x^2 + q^4y^2 )^2 \biggr]^{-1 / 2} </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ x^2 p^4 z^2 + q^4 y^2 p^4 z^2 + (x^2 + q^4y^2 )^2 \biggr]^{-1 / 2} \, . </math> |
A portion of these mappings are accomplished by setting <math>~a = p^2</math> and <math>~b = q^2p^2</math>, but this pair of specified coefficient values does not satisfy other mappings. Alternatively, a separate subset of mappings — but, again, not all mappings — is satisfied by setting <math>~a = 1</math> and <math>~b = q^4</math>. So the yellow-framed guess 2B does not provide a correct second-coordinate expression.
Blue-Framed Guess 2C
Let's try,
|
<math>~\lambda_{2\mathrm{B}}</math> |
<math>~=</math> |
<math>~(cz)^{2e} (ax^2 + by^2)^{-f} \, .</math> |
| Direction Cosine Components for Additional T9 Coordinate Guesses | ||||||||||||||||||||
| <math>~n</math> (1) |
<math>~\lambda_n</math> (2) |
<math>~h_n</math> (3) |
<math>~\partial \lambda_n/\partial x</math> (4) |
<math>~\partial \lambda_n/\partial y</math> (5) |
<math>~\partial \lambda_n/\partial z</math> (6) |
<math>~\gamma_{n1}</math> (7) |
<math>~\gamma_{n2}</math> (8) |
<math>~\gamma_{n3}</math> (9) |
||||||||||||
| <math>~2A</math> | --- | --- | --- | --- | --- | <math>~x p^2 z ~\mathfrak{J}_0 </math> | <math>~q^2 y p^2 z~\mathfrak{J}_0 </math> | <math>~-(x^2 + q^4 y^2)~\mathfrak{J}_0 </math> | ||||||||||||
| <math>~2B</math> | <math>~\biggl[1 + \frac{ez^2}{(ax^2 + by^2)} \biggr]^{-1 / 2}</math> | <math>~\biggl[\frac{(ax^2 + by^2)^2}{ez~\lambda_2^3} \biggr]\mathfrak{J}_{2\mathrm{B}}</math> | <math>~\frac{ax~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math> | <math>~\frac{by~ez^2 ~\lambda_2^3}{(ax^2 + by^2)^2} </math> | <math>~- \frac{ez ~\lambda_2^3}{(ax^2 + by^2)} </math> | <math>~axz ~\mathfrak{J}_{2\mathrm{B}}</math> | <math>~byz ~\mathfrak{J}_{2\mathrm{B}}</math> | <math>~-(ax^2 + by^2)~\mathfrak{J}_{2\mathrm{B}} </math> | ||||||||||||
| <math>~2C</math> | <math>~(cz)^{2e} (ax^2 + by^2)^{-f}</math> | <math>~\biggl[\frac{z(ax^2 + by^2)}{2e~\lambda_{2\mathrm{C}}} \biggr]\mathfrak{J}_{2\mathrm{C}}</math> | <math>~- \frac{2afx~\lambda_{2\mathrm{C}} }{(ax^2 + by^2)}</math> | <math>~- \frac{2bfy~\lambda_{2\mathrm{C}} }{(ax^2 + by^2)}</math> | <math>~\frac{2e~\lambda_{2\mathrm{C}} }{z}</math> | <math>~ -\biggl( \frac{af xz}{e} \biggr)~\mathfrak{J}_{2\mathrm{C}}</math> | <math>~- \biggl( \frac{bf yz}{e} \biggr)~\mathfrak{J}_{2\mathrm{C}}</math> | <math>~(ax^2 + by^2)~\mathfrak{J}_{2\mathrm{C}} </math> | ||||||||||||
|
||||||||||||||||||||
Examining just the expression for <math>~\mathfrak{J}_{2\mathrm{C}}</math>, we see that we definitely need: <math>~a = 1</math> and <math>~b=q^4</math>. Also, we need,
|
<math>~\frac{af}{e}</math> |
<math>~=</math> |
<math>~p^2</math> |
<math>~\Rightarrow </math> |
<math>~\frac{f}{e}</math> |
<math>~=</math> |
<math>~p^2 \, ;</math> |
and, separately we need,
|
<math>~\frac{fb}{e}</math> |
<math>~=</math> |
<math>~q^2 p^2</math> |
<math>~\Rightarrow </math> |
<math>~\frac{f}{e}</math> |
<math>~=</math> |
<math>~\frac{p^2}{q^2} \, .</math> |
These cannot simultaneously be satisfied. So the blue-framed guess 2C does not provide a correct second-coordinate expression. But we are very close! We need one additional scalar coefficient degree of freedom.
Next Thought
More generally, this leads to an expression for the scale-factor of the form,
|
<math>~h_2^{-2}</math> |
<math>~\sim</math> |
<math>~ \mathfrak{G}(x, y, z) \biggl[ x^2 p^4 z^2 + q^4 y^2 p^4 z^2 + (x^2 + q^4y^2 )^2 \biggr] </math> |
||||||||
|
<math>~\Rightarrow~~~ \frac{\partial \lambda_2}{\partial x}</math> |
<math>~\sim</math> |
<math>~ [\mathfrak{G}(x, y, z) ]^{1 / 2} \biggl[ x p^2 z \biggr] \, ; </math> |
<math>~\frac{\partial \lambda_2}{\partial y}</math> |
<math>~\sim</math> |
<math>~ [\mathfrak{G}(x, y, z) ]^{1 / 2} \biggl[ q^2 y p^2 z \biggr] \, ; </math> |
<math>~\frac{\partial \lambda_2}{\partial z}</math> |
<math>~\sim</math> |
<math>~ [\mathfrak{G}(x, y, z) ]^{1 / 2} \biggl[ x^2 + q^4y^2 \biggr] \, . </math> |
||
Now, if we set, <math>~a = p^2</math> and <math>~b = q^2p^2</math>, we have,
|
<math>~(a x z)^2 + (b y z )^2 + (ax^2 + by^2)^2</math> |
<math>~=</math> |
<math>~ ( x p^2 z)^2 + (q^2p^2 y z )^2 + (p^2 x^2 + q^2p^2 y^2)^2 </math> |
|
|
<math>~=</math> |
<math>~ p^4[ q^4 y^2 z^2 + x^2 z^2 + (x^2 + q^2 y^2 )^2] \, , </math> |
in which case,
Complete Orthogonality Check
If the set of unit vectors is indeed orthogonal, then we must find that,
|
<math>~\gamma_{mn}</math> |
<math>~=</math> |
<math>~M_{mn} \, ,</math> |
where the quantity <math>~M_{mn}</math> is the minor of <math>~\gamma_{mn}</math> in the determinant, <math>~|\gamma_{mn}|</math>. (Note: This last expression is true only for right-handed coordinate systems. If the coordinate system is left-handed, we should find, <math>~\gamma_{mn} = - M_{mn}</math>.) More specifically, for any right-handed, orthogonal curvilinear coordinate system we should find:
|
|
|
and the position vector is,
|
<math>~\mathbf{\vec{x}}</math> |
<math>~=</math> |
<math>~ \mathbf{\hat\imath} x + \mathbf{\hat\jmath} y + \mathbf{\hat{k}} z </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 (\gamma_{11} x + \gamma_{12} y + \gamma_{13} z) + \mathbf{\hat{e}}_2 (\gamma_{21} x + \gamma_{22} y + \gamma_{23} z) + \mathbf{\hat{e}}_3 (\gamma_{31} x + \gamma_{32} y + \gamma_{33} z) \, . </math> |
For (κ1, κ2, κ3)
|
<math>~\gamma_{22}\gamma_{33} - \gamma_{23}\gamma_{32}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{q^2y p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ 0 \biggr] + \biggl[ \frac{( x^2 + q^4y^2 ) \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] = x\ell_{3D} = \gamma_{11} \, . </math> Yes! |
|
<math>~\gamma_{23}\gamma_{31} - \gamma_{21}\gamma_{33}</math> |
<math>~=</math> |
<math>~ \bigg[ (x^2 + q^4y^2)^{1 / 2} \ell_{3D} \biggr] \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}} \biggr] - \biggl[ \frac{xp^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ 0 \biggr] = q^2 y \ell_{3D} = \gamma_{12} \, . </math> Yes! |
|
<math>~\gamma_{21}\gamma_{32} - \gamma_{22}\gamma_{31}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{xp^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] + \biggl[ \frac{q^2 y p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{x^2p^2 z \ell_{3D}}{(x^2 + q^4y^2)} \biggr] + \biggl[ \frac{q^4 y^2 p^2 z \ell_{3D}}{(x^2 + q^4y^2)} \biggr] = p^2 z \ell_{3D} = \gamma_{13} \, . </math> Yes! |
|
<math>~\gamma_{32}\gamma_{13} - \gamma_{33}\gamma_{12}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ p^2z \ell_{3D} \biggr] - \biggl[ 0\biggr] \biggl[ q^2y \ell_{3D} \biggr] = \biggl[ \frac{xp^2 z\ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] = \gamma_{21} \, . </math> Yes! |
|
<math>~\gamma_{33}\gamma_{11} - \gamma_{31}\gamma_{13}</math> |
<math>~=</math> |
<math>~ \biggl[ 0\biggr] \biggl[ x\ell_{3D} \biggr] + \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ p^2z \ell_{3D} \biggr] = \biggl[ \frac{q^2y p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] = \gamma_{22} \, . </math> Yes! |
|
<math>~\gamma_{31}\gamma_{12} - \gamma_{32}\gamma_{11}</math> |
<math>~=</math> |
<math>~ - \biggl[ \frac{q^2y}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ q^2y \ell_{3D} \biggr] - \biggl[ \frac{x}{(x^2 + q^4y^2)^{1 / 2}} \biggr] \biggl[ x\ell_{3D} \biggr] = -(x^2 + q^4y^2)^{1 / 2}\ell_{3D} = \gamma_{23} \, . </math> Yes! |
|
<math>~\gamma_{12}\gamma_{23} - \gamma_{13}\gamma_{22}</math> |
<math>~=</math> |
<math>~ - \biggl[ q^2y \ell_{3D} \biggr] \biggl[ (x^2 + q^4y^2)^{1 / 2}\ell_{3D} \biggr] - \biggl[ p^2 z\ell_{3D} \biggr] \biggl[ \frac{q^2y p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ - \frac{ q^2 y \ell_{3D}^2 }{(x^2 + q^4y^2)^{1 / 2} } \biggl[ x^2 + q^4y^2 + p^4 z^2 \biggr] = - \frac{ q^2 y }{(x^2 + q^4y^2)^{1 / 2} } = \gamma_{31} \, . </math> Yes! |
|
<math>~\gamma_{13}\gamma_{21} - \gamma_{11}\gamma_{23}</math> |
<math>~=</math> |
<math>~ \biggl[ p^2z \ell_{3D} \biggr] \biggl[ \frac{x p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] + \biggl[ x \ell_{3D} \biggr] \biggl[ (x^2 + q^4y^2)^{1 / 2} \ell_{3D}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{x \ell_{3D}^2 }{ (x^2 + q^4y^2)^{1 / 2} } \biggl[ x^2 + q^4y^2 + p^4 z^2 \biggr] = \frac{x }{ (x^2 + q^4y^2)^{1 / 2} } = \gamma_{32} \, . </math> Yes! |
|
<math>~\gamma_{11}\gamma_{22} - \gamma_{12}\gamma_{21}</math> |
<math>~=</math> |
<math>~ \biggl[ x \ell_{3D} \biggr] \biggl[ \frac{q^2y p^2z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] - \biggl[ q^2y \ell_{3D} \biggr] \biggl[ \frac{xp^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} \biggr] = 0 = \gamma_{33} \, . </math> Yes! |
Given that the prescribed interrelationships between all nine direction cosines are satisfied, we conclude that the <math>~(\kappa_1, \kappa_2, \kappa_3)</math> coordinate system is an orthogonal one. Accordingly, the position vector is,
|
<math>~\mathbf{\vec{x}}</math> |
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl\{ x^2 \ell_{3D} + q^2y^2 \ell_{3D} + p^2z^2 \ell_{3D} \biggr\} </math> |
|
|
|
<math>~ + \mathbf{\hat{e}}_2 \biggl\{ x^2 + q^2y^2 - \frac{1}{p^2}(x^2 + q^4 y^2) \biggr\} \frac{p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} </math> |
|
|
|
<math>~ +~ \mathbf{\hat{e}}_3 \biggl\{ - \frac{xq^2y}{(x^2 + q^4y^2)^{1 / 2}} + \frac{xy}{(x^2 + q^4 y^2)^{1 / 2}} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl[ \kappa_1^2 \ell_{3D} \biggr] ~+~ \mathbf{\hat{e}}_2 \biggl[ x^2 + q^2y^2 - \frac{1}{p^2}(x^2 + q^4 y^2) \biggr] \frac{p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} ~+~ \mathbf{\hat{e}}_3 \biggl[ \frac{(1-q^2)xy}{(x^2 + q^4 y^2)^{1 / 2}} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl[ \kappa_1^2 \ell_{3D} \biggr] ~+~ \mathbf{\hat{e}}_2 \biggl[ x^2\biggl(1 - \frac{1}{p^2}\biggr) - q^2y^2 \biggl(q^2 - 1 \biggr) \biggr] \frac{p^2 z \ell_{3D}}{(x^2 + q^4y^2)^{1 / 2}} ~-~ \mathbf{\hat{e}}_3 \biggl[ \frac{(q^2 - 1)xy}{(x^2 + q^4 y^2)^{1 / 2}} \biggr] \, . </math> |
For (κ1, κ4, κ5)
|
<math>~\gamma_{42}\gamma_{53} - \gamma_{43}\gamma_{52}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{xq^2y p^2z}{\mathcal{D}} \biggl( \frac{1}{q^2y} \biggr) \biggr] \biggl[ \frac{p^2 z \ell_{3D}}{\mathcal{D}} \biggl(x^2 - q^4y^2 \biggr) \biggr] + \biggl[ \frac{xq^2 y p^2 z}{\mathcal{D}} \biggl( \frac{2}{p^2z} \biggr) \biggr] \biggl[ \frac{q^2y \ell_{3D}}{\mathcal{D}}\biggl( p^4 z^2 + 2x^2 \biggr) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{x\ell_{3D}}{\mathcal{D}^2} \biggl[ (x^2 - q^4y^2) p^4z^2 + ( p^4 z^2 + 2x^2 )2q^4y^2 \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{x\ell_{3D}}{\mathcal{D}^2} \biggl[ x^2p^4z^2 + 4x^2 q^4y^2 + q^4y^2 p^4 z^2 \biggr] = x\ell_{3D} = \gamma_{11} \, . </math> Yes! |
|
<math>~\gamma_{43}\gamma_{51} - \gamma_{41}\gamma_{53}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{xq^2y p^2z}{\mathcal{D}} \biggl( \frac{2}{p^2 z} \biggr) \biggr] \biggl[ \frac{x \ell_{3D}}{\mathcal{D}} \biggl(2q^4y^2 +p^4z^2\biggr) \biggr] - \biggl[ \frac{xq^2 y p^2 z}{\mathcal{D}} \biggl( \frac{1}{x} \biggr) \biggr] \biggl[ \frac{p^2 z \ell_{3D}}{\mathcal{D}}\biggl( x^2 - q^4y^2 \biggr) \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{q^2 y \ell_{3D}}{\mathcal{D}^2} \biggl[ 2 x^2 (2q^4y^2 +p^4z^2 ) - p^4 z^2 ( x^2 - q^4y^2) \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{q^2 y \ell_{3D}}{\mathcal{D}^2} \biggl[ 4x^2 q^4y^2 + x^2 p^4z^2 + q^4y^2 p^4 z^2 \biggr] = q^2y \ell_{3D} = \gamma_{12} \, . </math> Yes! |
|
<math>~\gamma_{41}\gamma_{52} - \gamma_{42}\gamma_{51}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{xq^2y p^2z}{\mathcal{D}} \biggl( \frac{1}{x} \biggr) \biggr] \biggl[ \frac{q^2 y\ell_{3D}}{\mathcal{D}} \biggl(p^4z^2 + 2x^2\biggr) \biggr] + \biggl[ \frac{xq^2 y p^2 z}{\mathcal{D}} \biggl( \frac{1}{q^2 y} \biggr) \biggr] \biggl[ \frac{x \ell_{3D}}{\mathcal{D}}\biggl( 2q^4y^2 + p^4z^2 \biggr) \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{p^2 z \ell_{3D}}{\mathcal{D}^2} \biggl[ q^4y^2 (p^4z^2 + 2x^2) + x^2 ( 2q^4y^2 + p^4z^2 ) \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{p^2 z \ell_{3D}}{\mathcal{D}^2} \biggl[ q^4y^2 p^4z^2 + 4x^2 q^4 y^2 + x^2p^4z^2 \biggr] = p^2 z \ell_{3D} = \gamma_{13} \, . </math> Yes! |
|
<math>~\gamma_{52}\gamma_{13} - \gamma_{53}\gamma_{12}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{q^2 y p^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( p^4z^2 + 2x^2 \biggr) \biggr] - \biggl[ \frac{q^2 y p^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( x^2 - q^4y^2 \biggr) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{q^2 y p^2 z\ell_{3D}^2}{\mathcal{D}} \biggl[ x^2 + q^4y^2 + p^4z^2 \biggr] = \frac{q^2 y p^2 z }{\mathcal{D}} = \gamma_{41} \, . </math> Yes! |
|
<math>~\gamma_{53}\gamma_{11} - \gamma_{51}\gamma_{13}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{xp^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( x^2 - q^4y^2 \biggr) \biggr] + \biggl[ \frac{xp^2 z\ell_{3D}^2}{\mathcal{D}} \biggl( 2q^4y^2 +p^4z^2\biggr) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{xp^2 z\ell_{3D}^2}{\mathcal{D}} \biggl[ x^2 + q^4y^2 +p^4z^2 \biggr] = \frac{xp^2 z}{\mathcal{D}} = \gamma_{42} \, . </math> Yes! |
|
<math>~\gamma_{51}\gamma_{12} - \gamma_{52}\gamma_{11}</math> |
<math>~=</math> |
<math>~ -\biggl[ \frac{x q^2y \ell_{3D}^2}{\mathcal{D}} \biggl( 2q^4y^2 +p^4z^2\biggr) \biggr] - \biggl[ \frac{x q^2y \ell_{3D}^2}{\mathcal{D}} \biggl( p^4z^2 + 2x^2\biggr) \biggr] </math> |
|
|
<math>~=</math> |
<math>~ -\frac{2x q^2y \ell_{3D}^2}{\mathcal{D}}\biggl[ x^2 + q^4y^2 +p^4z^2 \biggr] = -\frac{2x q^2y }{\mathcal{D}} = \gamma_{43} \, . </math> Yes! |
|
<math>~\gamma_{12}\gamma_{43} - \gamma_{13}\gamma_{42}</math> |
<math>~=</math> |
<math>~ - \biggl[ \frac{2x q^4 y^2 \ell_{3D} }{\mathcal{D}} \biggr] - \biggl[ \frac{x p^4 z^2 \ell_{3D} }{\mathcal{D}} \biggr] = - \biggl[ \frac{x \ell_{3D} }{\mathcal{D}} \biggr] (2q^4 y^2 + p^4z^2) = \gamma_{51} \, . </math> Yes! |
|
<math>~\gamma_{13}\gamma_{41} - \gamma_{11}\gamma_{43}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{q^2 y p^4z^2 \ell_{3D} }{\mathcal{D}} \biggr] + \biggl[ \frac{2x^2 q^2 y \ell_{3D} }{\mathcal{D}} \biggr] = \biggl[ \frac{q^2 y \ell_{3D} }{\mathcal{D}} \biggr](p^4z^2 + 2x^2) = \gamma_{52} \, . </math> Yes! |
|
<math>~\gamma_{11}\gamma_{42} - \gamma_{12}\gamma_{41}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x^2 p^2z \ell_{3D}}{\mathcal{D}} \biggr] - \biggl[ \frac{q^4 y^2 p^2 z\ell_{3D} }{\mathcal{D}} \biggr] = \biggl[ \frac{p^2z \ell_{3D}}{\mathcal{D}} \biggr](x^2 - q^4y^2) = \gamma_{53} \, . </math> Yes! |
Given that the prescribed interrelationships between all nine direction cosines are satisfied, we conclude that the <math>~(\kappa_1, \kappa_4, \kappa_5)</math> coordinate system is an orthogonal one. Accordingly, the position vector is,
|
<math>~\mathbf{\vec{x}}</math> |
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 (\gamma_{11} x + \gamma_{12} y + \gamma_{13} z) + \mathbf{\hat{e}}_2 (\gamma_{41} x + \gamma_{42} y + \gamma_{43} z) + \mathbf{\hat{e}}_3 (\gamma_{51} x + \gamma_{52} y + \gamma_{53} z) </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl\{ x^2\ell_{3D} + q^2y^2 \ell_{3D} + p^2z^2\ell_{3D} \biggr\} </math> |
|
|
|
<math>~ + \mathbf{\hat{e}}_2 \biggl\{ \frac{xq^2yp^2z}{\mathcal{D}} + \frac{x y p^2z}{\mathcal{D}} - \frac{2 xq^2y z}{\mathcal{D}} \biggr\} </math> |
|
|
|
<math>~ + \mathbf{\hat{e}}_3 \biggl\{ - \frac{\ell_{3D} x^2}{\mathcal{D}} (2q^4y^2 + p^4z^2) + \frac{\ell_{3D} q^2 y^2}{\mathcal{D}} (p^4z^2 + 2x^2) + \frac{\ell_{3D} p^2z^2}{\mathcal{D}} (x^2 - q^4y^2) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl\{ \kappa_1^2 \ell_{3D} \biggr\} + \mathbf{\hat{e}}_2 \biggl\{ q^2p^2 + p^2 - 2 q^2 \biggr\} \frac{xyz}{\mathcal{D}} </math> |
|
|
|
<math>~ + \mathbf{\hat{e}}_3 \biggl\{ - x^2 (2q^4y^2 + p^4z^2) + q^2 y^2 (p^4z^2 + 2x^2) + p^2z^2 (x^2 - q^4y^2) \biggr\} \frac{\ell_{3D}}{\mathcal{D}} </math> |
|
|
<math>~=</math> |
<math>~ \mathbf{\hat{e}}_1 \biggl\{ \kappa_1^2 \ell_{3D} \biggr\} ~+~ \mathbf{\hat{e}}_2 \biggl\{ q^2p^2 + p^2 - 2 q^2 \biggr\} \frac{xyz}{\mathcal{D}} ~-~ \mathbf{\hat{e}}_3 \biggl\{ 2 x^2 q^2y^2(q^2-1) + x^2 p^2z^2(p^2-1) + q^2 y^2 p^2 z^2(q^2 - p^2) \biggr\} \frac{\ell_{3D}}{\mathcal{D}} \, . </math> |
See Also

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |