Difference between revisions of "User:Tohline/Appendix/Ramblings/BordeauxSequences"
| (20 intermediate revisions by the same user not shown) | |||
| Line 124: | Line 124: | ||
</table> | </table> | ||
===Model Sequences=== | |||
====Fixed Ω==== | |||
<span id="jSquared">The Maclaurin sequence (MLS) is defined by the relations:</span> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Omega^2 \equiv \frac{\omega_0^2}{4\pi G \rho}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{1}{2}(3 - 2e^2)(1 - e^2)^{1 / 2} \cdot \frac{\sin^{-1} e}{e^3} - \frac{3(1-e^2)}{2e^2} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~j^2 \equiv \biggl( \frac{3}{2^8 \pi^4} \biggr)^{1 / 3} \frac{L_c^2}{G M_c^3 } \cdot R_\mathrm{eq}^{-1} (1-e^2)^{-1 / 6}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{3}{2^8 \pi^4} \biggr)^{1 / 3} \frac{\Omega^2}{G R_\mathrm{eq}(1-e^2)^{1 / 6} } | |||
\biggl[ \biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr) (1 - e^2) G R_\mathrm{eq}^{10} \rho^3 \biggr] | |||
\biggl[ \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr]^{-3} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{2^{24} \pi^9}{3^6 \cdot 5^6}\biggr)^{1 / 3} \biggl( \frac{3^9}{2^{18}\pi^9}\biggr)^{1 / 3} | |||
\biggl( \frac{3}{2^8 \pi^4} \biggr)^{1 / 3} \frac{\Omega^2}{(1-e^2)^{2 / 3} } | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{3^4}{2^2 \cdot 5^6\pi^4}\biggr)^{1 / 3} | |||
\frac{\Omega^2}{(1-e^2)^{2 / 3} } \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
=====Central Object===== | =====Central Object===== | ||
Assume that the central object is exactly a Maclaurin spheroid. Then from [[User:Tohline/Apps/MaclaurinSpheroidSequence#Equilibrium_Angular_Velocity|Figure 1 (and Table 1) of our review of equilibrium models along the Maclaurin spheroid sequence]], we appreciate that all we have to do is specify the eccentricity, <math>~0 \le e \le 1</math>, and <math>~\Omega^2 \equiv \omega_0^2/(4\pi G\rho)</math> is known. For example, if we choose <math>~e = 0.60</math>, then from that Table 1, <math>~\omega_0^2/(2\pi G\rho) = 0.1007~~\Rightarrow~~\Omega^2 \approx 0.05</math>. Other properties of this "central" spheroid — such as its mass, moment of inertia, and angular momentum — are given | Assume that the central object is exactly a Maclaurin spheroid. Then from [[User:Tohline/Apps/MaclaurinSpheroidSequence#Equilibrium_Angular_Velocity|Figure 1 (and Table 1) of our review of equilibrium models along the Maclaurin spheroid sequence]], we appreciate that all we have to do is specify the eccentricity, <math>~0 \le e \le 1</math>, and <math>~\Omega^2 \equiv \omega_0^2/(4\pi G\rho)</math> is known. For example, if we choose <math>~e = 0.60</math>, then from that Table 1, <math>~\omega_0^2/(2\pi G\rho) = 0.1007~~\Rightarrow~~\Omega^2 \approx 0.05</math>. Other properties of this "central" spheroid — such as its mass, moment of inertia, and angular momentum — are given by the following expressions: | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 197: | Line 263: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~4\pi G\biggl( \frac{2^3\pi}{3\cdot 5}\biggr)^2 (1 - e^2) R_\mathrm{eq}^{10} \rho^3 \Omega^2 \, . </math> | <math>~4\pi G\biggl( \frac{2^3\pi}{3\cdot 5}\biggr)^2 (1 - e^2) R_\mathrm{eq}^{10} \rho^3 \Omega^2 </math> | ||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr) (1 - e^2) G R_\mathrm{eq}^{10} \rho^3 \Omega^2 \, . </math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
We note as well that the Keplerian frequency for a massless particle orbiting in the equatorial plane of this central object will be, | We note as well that the (square of the) Keplerian frequency for a massless particle orbiting in the equatorial plane at a distance, <math>~r</math>, from the center of this central object will be, | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 270: | Line 348: | ||
</table> | </table> | ||
For example, when <math>~(e, \Omega^2) = (0.6, 0.05)</math>, we have, <math>~r/R_\mathrm{eq} = (5.3333)^{1 / 3} = 1.747</math>. | For example, when <math>~(e, \Omega^2) = (0.6, 0.05)</math>, we have, <math>~r/R_\mathrm{eq} = (5.3333)^{1 / 3} = 1.747</math>. | ||
=====Surrounding Torus===== | =====Surrounding Torus===== | ||
| Line 278: | Line 355: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{a}{R_\mathrm{eq}} </math> | <math>~\alpha_t \equiv \frac{a}{R_\mathrm{eq}} </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 302: | Line 379: | ||
<math>~2\pi a (\pi b^2)\rho | <math>~2\pi a (\pi b^2)\rho | ||
= | = | ||
2\pi^2 R_\mathrm{eq}^3 \rho \biggl[ \ | 2\pi^2 R_\mathrm{eq}^3 \rho \biggl[ \alpha_t \beta_t^2 \biggr] | ||
\, | \, ,</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
where, | |||
<table border="0" cellpadding="5" align="center"> | |||
Given that | <tr> | ||
<td align="right"> | |||
<math>~\beta_t</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{b}{R_\mathrm{eq}} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Given that <math>~\alpha_t</math> is known once the eccentricity of the central Maclaurin spheroid has been selected and, given that the density of the torus must match the density of the central object, the mass of the torus will only depend on the choice of <math>~0 < \beta_t \le \beta_\mathrm{max}</math>. The maximum allowed value, <math>~\beta_\mathrm{max}</math>, is set by ensuring that equatorial-plane location of the inner edge of the torus is no smaller than the equatorial radius of the central spheroid, <math>~R_\mathrm{eq}</math>. This means, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\beta_\mathrm{max}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 319: | Line 411: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\alpha_t - 1 \, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 334: | Line 426: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
2\pi^2 R_\mathrm{eq}^3 \rho | 2\pi^2 R_\mathrm{eq}^3 \rho \biggl[ \alpha_t \beta_\mathrm{max}^2 \biggr] | ||
\biggl[\ | = | ||
\biggr\}^2 | 2\pi^2 R_\mathrm{eq}^3 \rho ~\alpha_t (\alpha_t - 1)^2 \, . | ||
\, . | </math> | ||
</td> | |||
</tr> | |||
</table> | |||
The moment of inertia of the torus is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~I_t</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~M_t R_\mathrm{eq}^2\biggl[ \biggl(\frac{a}{R_\mathrm{eq}} \biggr)^2 + \frac{3}{4} \biggl( \frac{b}{R_\mathrm{eq}} \biggr)^2 \biggr]</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~2\pi^2 R_\mathrm{eq}^5 \rho \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Hence, the (square of the) angular momentum of the torus is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~L_t^2 = I_t^2 \omega_0^2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ 2\pi^2 R_\mathrm{eq}^5 \rho \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \biggr]^2 \Omega^2 (4\pi G\rho) | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
2^4 \pi^5 \alpha_t^2 \beta_t^4 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr)^2 G R_\mathrm{eq}^{10} \rho^3 \Omega^2 \, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 345: | Line 495: | ||
=====Combined Configuration===== | =====Combined Configuration===== | ||
Given that, for the chosen Maclaurin spheroid, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\bar{a}^3</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~R_\mathrm{eq}^2 Z = R_\mathrm{eq}^3(1-e^2)^{1 / 2} \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
and that the total mass of the system is, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 357: | Line 524: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~M_c + | <math>~M_c + M_t</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 370: | Line 537: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \frac{2^2\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} | ||
+ | + | ||
2\pi^2 R_\mathrm{eq}^3 \rho ~ | 2\pi^2 R_\mathrm{eq}^3 \rho \alpha_t \beta_t^2 | ||
\biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr]^{1 / 3} \biggl\{ \biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr]^{1 / 3} -1 | </math> | ||
\biggr\}^2 \, . | </td> | ||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
2\pi R_\mathrm{eq}^3 \rho \biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr] | |||
\, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
the (square of the) dimensionless total angular momentum of the combined system is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~j_\mathrm{tot}^2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \frac{(L_c + L_t)^2}{GM_\mathrm{tot}^3 \bar{a}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} | |||
\biggl\{ L_c + L_t \biggr\}^2 \biggl\{ M_\mathrm{tot} \biggr\}^{-3}G^{-1} R_\mathrm{eq}^{-1} (1 - e^2)^{-1 / 6} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} | |||
\biggl\{ \biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr)^{1 / 2} (1 - e^2)^{1 / 2} G^{1 / 2} R_\mathrm{eq}^{5} \rho^{3 / 2} \Omega | |||
+ 2^2 \pi^{5 / 2} \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) G^{1 / 2} R_\mathrm{eq}^{5} \rho^{3 / 2} \Omega \biggr\}^2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~\times | |||
\biggl\{ 2\pi R_\mathrm{eq}^3 \rho \biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr] \biggr\}^{-3}G^{-1} R_\mathrm{eq}^{-1} (1 - e^2)^{-1 / 6} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{2^3 \pi^3} | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} | |||
\biggl\{ \biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr)^{1 / 2} (1 - e^2)^{1 / 2} \Omega | |||
+ 2^2 \pi^{5 / 2} \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \Omega \biggr\}^2 | |||
\biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr]^{-3} (1 - e^2)^{-1 / 6} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~2\Omega^2 | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} | |||
\biggl\{ \biggl( \frac{2^2 }{3 \cdot 5}\biggr) (1 - e^2)^{1 / 2} | |||
+ \pi \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \biggr\}^2 | |||
\biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr]^{-3} (1 - e^2)^{-1 / 6} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Notice that when the toroidal component is omitted, this expression reduces to, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~j_c^2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~2\Omega^2 | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} | |||
\biggl\{ \biggl( \frac{2^2}{3 \cdot 5}\biggr) (1 - e^2)^{1 / 2} | |||
\biggr\}^2 | |||
\biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} \biggr]^{-3} (1 - e^2)^{-1 / 6} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~2\Omega^2 | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} | |||
\biggl( \frac{2^4}{3^2 \cdot 5^2}\biggr) | |||
\biggl(\frac{3^3}{2^3}\biggr) | |||
(1 - e^2)^{-3 / 2} (1 - e^2)^{-1 / 6} (1 - e^2) | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\Omega^2 | |||
\biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} | |||
\biggl( \frac{2^2 \cdot 3}{5^2}\biggr) | |||
(1 - e^2)^{-2 / 3} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\Omega^2 | |||
\biggl(\frac{3^4}{2^2 \cdot 5^6 \pi^4}\biggr)^{1 / 3} | |||
(1 - e^2)^{-2 / 3} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
This matches the expression for the isolated Maclaurin spheroid [[#jSquared|derived above]]. | |||
====Fixed Mass Ratio==== | |||
Let's define the mass ratio, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~q</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{M_t}{M_c} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
and build a sequence along which this ratio is held constant. For the problem being considered here, the relevant expression for <math>~q</math> is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~q</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ 2\pi^2 R_\mathrm{eq}^3 \rho \alpha_t \beta_t^2 \biggr] | |||
\biggl[ \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr]^{-1} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{3\pi}{2} \biggr) \alpha_t \beta_t^2 (1 - e^2)^{- 1 / 2}\, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
The sequence is constructed by choosing <math>~q</math> (held fixed), and varying the value of <math>~0 < \beta_t \le \beta_\mathrm{max}</math>; then, for each chosen parameter pair, recognize that, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\alpha_t (1 - e^2)^{- 1 / 2} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl( \frac{2}{3\pi} \biggr) \frac{q}{ \beta_t^2 } \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
that is, from the [[#Surrounding_Torus|above expression for <math>~\alpha_t</math>]], | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr] (1 - e^2)^{- 3 / 2} | |||
</math> | </math> | ||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \biggl( \frac{2}{3\pi} \biggr) \frac{q}{ \beta_t^2 } \biggr]^3</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ | |||
\Omega^2 (1 - e^2) | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{3}\biggl[ \biggl( \frac{3\pi}{2} \biggr) \frac{ \beta_t^2 }{q} \biggr]^3 \, .</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
But, for central models along the MLS, we also must satisfy the relation [[#Model_Sequences|given above]], namely, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Omega^2 </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{1}{2}(3 - 2e^2)(1 - e^2)^{1 / 2} \cdot \frac{\sin^{-1} e}{e^3} - \frac{3(1-e^2)}{2e^2} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Hence, for each <math>~(q, \beta_t)</math> parameter pair, the relevant central-object eccentricity is given by the root of the relation, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\frac{1}{2}(3 - 2e^2)(1 - e^2)^{3 / 2} \cdot \frac{\sin^{-1} e}{e^3} - \frac{3(1-e^2)^2 }{2e^2} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{3}\biggl[ \biggl( \frac{3\pi}{2} \biggr) \frac{ \beta_t^2 }{q} \biggr]^3 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Note that, for the limiting value, <math>~\beta_\mathrm{max} = (\alpha_t - 1)</math>, the relevant relation becomes, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
(\alpha_t - 1)^2\alpha_t (1 - e^2)^{- 1 / 2} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{2q}{3\pi} </math> | |||
</td> | |||
</tr> | |||
</table> | |||
where [[#Surrounding_Torus|again, as above]] | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\alpha_t \equiv \frac{a}{R_\mathrm{eq}} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr]^{1 / 3} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
=See Also= | |||
<ul> | |||
<li>Université de Bordeaux (Part 1): [[User:Tohline/Appendix/Ramblings/Bordeaux|External Gravitational Potential of Toroids]]</li> | |||
<li>Université de Bordeaux (Part 3): [[User:Tohline/Appendix/Ramblings/BordeauxPostDefense|Discussions Following Dissertation Defense]]</li> | |||
</ul> | |||
{{LSU_HBook_footer}} | {{LSU_HBook_footer}} | ||
Latest revision as of 00:00, 17 December 2020
Université de Bordeaux (Part 2)

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Exterior Gravitational Potential of Toroids
J. -M. Huré, B. Basillais, V. Karas, A. Trova, & O. Semerák (2020), MNRAS, 494, 5825-5838 have published a paper titled, The Exterior Gravitational Potential of Toroids. Here we examine how their work relates to the published work by C.-Y. Wong (1973, Annals of Physics, 77, 279), which we have separately discussed in detail.
We discuss this topic in a separate, accompanying chapter.
Spheroid-Ring Systems
Through a research collaboration at the Université de Bordeaux, B. Basillais & J. -M. Huré (2019), MNRAS, 487, 4504-4509 have published a paper titled, Rigidly Rotating, Incompressible Spheroid-Ring Systems: New Bifurcations, Critical Rotations, and Degenerate States.
Key References
Here are some relevant publications:
- Hachisu (1986a, ApJS, 61, 479): A Versatile Method for Obtaining Structures of Rapidly Rotating Stars
- Fujisawa & Eriguchi (2014, MNRAS, 438, L61): Prolate stars due to meridional flows
- Huré, Hersant & Nasello (2018, MNRAS, 475, 63): The equilibrium of overpressurized polytropes
- & Eriguchi (1984, Ap&SS, 99, 71): Fission Sequence and Equilibrium Models of [Rigidly] Rotating Polytropes
- Hachisu, Eriguchi & Nomoto (1986b, ApJ, 311, 214): Fate of merging double white dwarfs. II - Numerical method
- Nishida, Eriguchi & Lanza (1992, ApJ, 401, 618): General Relativistic Structure of Star-Toroid Systems
- Woodward, Sankaran & Tohline (1992 ApJ, 394, 248): Tidal Disruption of a Star by a Massive Disk (The Axisymmetric Roche Problem)
Especially,
- Eriguchi & Hachisu (1983, Prog. Theor. Phys., 69, 1131): Two Kinds of Axially Symmetric Equilibrium Sequences of Self-Gravitating and Rotating Incompressible Fluids: Two-Ring Sequence and Core-Ring Sequence
- Ansorg, Kleinwächter & Meinel (2003, MNRAS, 339, 515): Uniformly rotating axisymmetric fluid configurations bifurcating from highly flattened Maclaurin spheroids
- Hachisu, Eriguchi & Nomoto (1986a, ApJ, 308, 161): Fate of Merging Double White Dwarfs
Key Figures
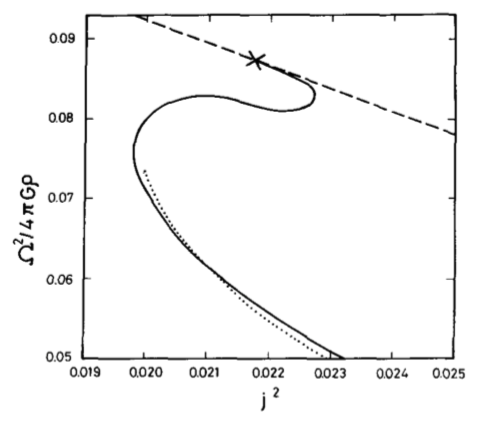
Eriguchi & Sugimoto (1981)
|
Fig. 1 extracted without modification from p. 1873 of Eriguchi & Sugimoto (1981)
"Another Equilibrium Sequence of Self-Gravitating and Rotating Incompressible Fluid"
Progress of Theoretical Physics,
vol. 65, pp. 1870-1875 © Progress of Theoretical Physics |
|
CAPTION (modified here): The squared angular velocity is plotted against <math>~j^2</math> for a segment of the Maclaurin sequence (dashed curve), for the Dyson-Wong sequence (dotted curve), and for the new configurations reported in this 1981 paper by Eriguchi & Sugimoto (solid curve). The "×" mark denotes the neutral point on the Maclaurin sequence against the <math>~P_4(\eta)</math> perturbation. The dotted curve is plotted by using the values which are read from the curve of Fig. 6 of Wong (1974), so it may contain errors to some extent. |
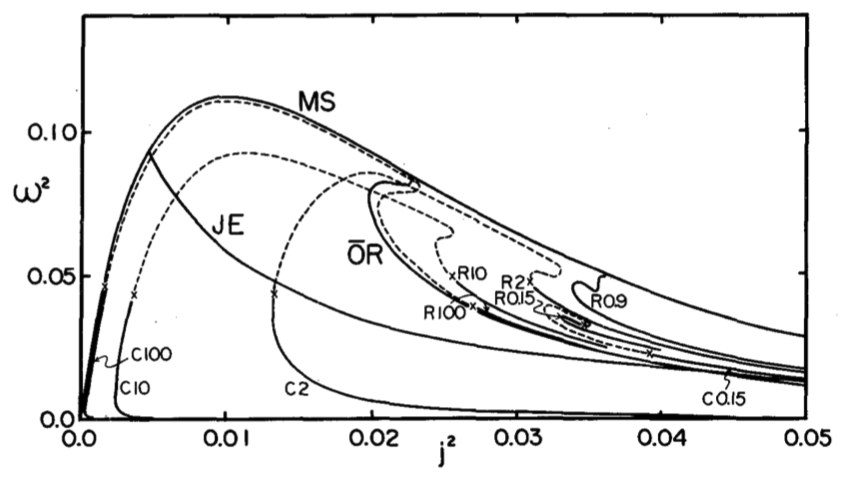
Eriguchi & Hachisu (1983)
|
Fig. 3 extracted without modification from p. 1134 of Eriguchi & Hachisu (1983)
"Two Kinds of Axially Symmetric Equilibrium Sequences of Self-Gravitating and Rotating Incompressible Fluids: Two-Ring Sequence and Core-Ring Sequence" Progress of Theoretical Physics, vol. 69, pp. 1131-1136 © Progress of Theoretical Physics |
|
CAPTION: The angular momentum-angular velocity relations. Solid curves represent uniformly rotating equilibrium sequences.
The number and letter R or C attached to a curve denote mass ratio and two-ring or core-ring sequence, respectively. If differential rotation is allowed, the equilibrium sequences may continue to exist as shown by the dashed curves. |
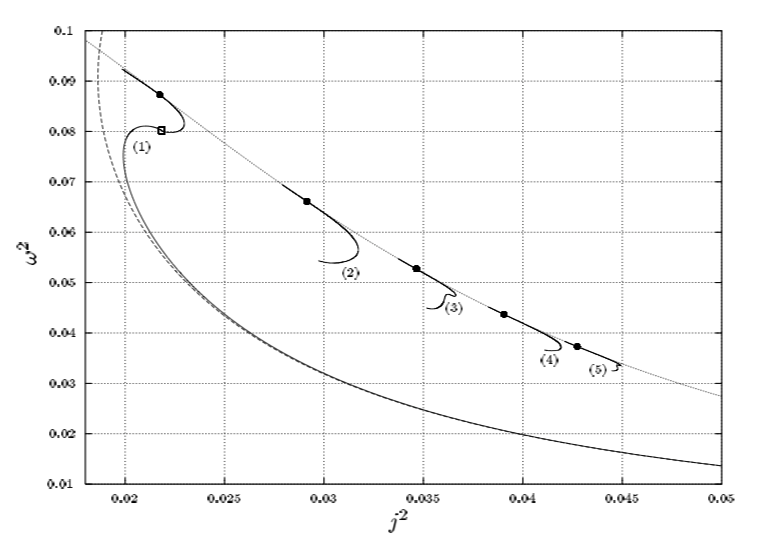
AKM (2003)
|
Fig. 2 extracted without modification from p. 517 of Ansorg, Kleinwächter & Meinel (2003)
"Uniformly rotating axisymmetric fluid configurations bifurcating from highly flattened Maclaurin spheroids"
MNRAS, vol. 339, pp. 515-523 © Royal Astronomical Society |
|
CAPTION: For the first five axisymmetric sequences, <math>~\omega_0^2</math> is plotted against the dimensionless squared angular momentum, <math>~j^2</math>, using the same normalizations as Eriguchi & Hachisu (1983). Dotted and dashed curves again refer to the Maclaurin sequence and the Dyson approximation respectively. The full circles mark the bifurcation points on the Maclaurin sequence, and the open square the transition configuration of spheroidal to toroidal bodies on the Dyson ring sequence. |
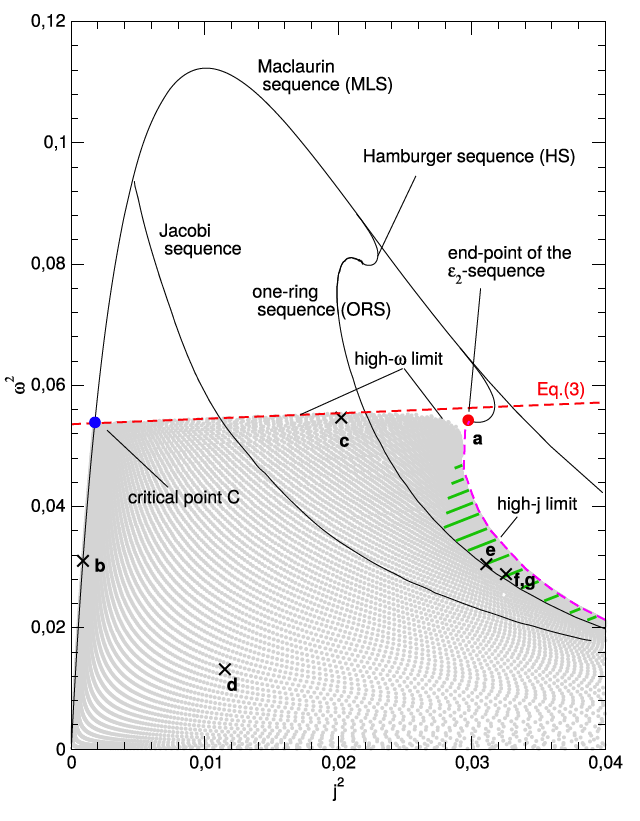
Basillais & Huré (2019)
|
Fig. 4 extracted without modification from p. 4507 of Basillais & Huré (2019)
"Rigidly rotating, incompressible spheroid-ring systems: new bifurcations, critical rotations, and degenerate states"
MNRAS, vol. 487, pp. 4504-4509 © Royal Astronomical Society |
|
CAPTION: The spheroid-ring solutions (grey dots) populate the <math>~\omega_0^2 - j^2</math> diagram in between the MLS, the high-ω limit, and the high-j limit. The MLS, ORS, Jacobi sequence, Hamburger sequence, and ε2-sequence are also shown (plain lines). Points labelled a to f (cross) correspond to equilibria shown in Figure 3; see also Table 1. There is a band of degeneracy rightward to the ORS (green dashed zone). |
Model Sequences
Fixed Ω
The Maclaurin sequence (MLS) is defined by the relations:
|
<math>~\Omega^2 \equiv \frac{\omega_0^2}{4\pi G \rho}</math> |
<math>~=</math> |
<math>~ \frac{1}{2}(3 - 2e^2)(1 - e^2)^{1 / 2} \cdot \frac{\sin^{-1} e}{e^3} - \frac{3(1-e^2)}{2e^2} \, , </math> |
|
<math>~j^2 \equiv \biggl( \frac{3}{2^8 \pi^4} \biggr)^{1 / 3} \frac{L_c^2}{G M_c^3 } \cdot R_\mathrm{eq}^{-1} (1-e^2)^{-1 / 6}</math> |
<math>~=</math> |
<math>~ \biggl( \frac{3}{2^8 \pi^4} \biggr)^{1 / 3} \frac{\Omega^2}{G R_\mathrm{eq}(1-e^2)^{1 / 6} } \biggl[ \biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr) (1 - e^2) G R_\mathrm{eq}^{10} \rho^3 \biggr] \biggl[ \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr]^{-3} </math> |
|
|
<math>~=</math> |
<math>~ \biggl( \frac{2^{24} \pi^9}{3^6 \cdot 5^6}\biggr)^{1 / 3} \biggl( \frac{3^9}{2^{18}\pi^9}\biggr)^{1 / 3} \biggl( \frac{3}{2^8 \pi^4} \biggr)^{1 / 3} \frac{\Omega^2}{(1-e^2)^{2 / 3} } </math> |
|
|
<math>~=</math> |
<math>~ \biggl( \frac{3^4}{2^2 \cdot 5^6\pi^4}\biggr)^{1 / 3} \frac{\Omega^2}{(1-e^2)^{2 / 3} } \, . </math> |
Central Object
Assume that the central object is exactly a Maclaurin spheroid. Then from Figure 1 (and Table 1) of our review of equilibrium models along the Maclaurin spheroid sequence, we appreciate that all we have to do is specify the eccentricity, <math>~0 \le e \le 1</math>, and <math>~\Omega^2 \equiv \omega_0^2/(4\pi G\rho)</math> is known. For example, if we choose <math>~e = 0.60</math>, then from that Table 1, <math>~\omega_0^2/(2\pi G\rho) = 0.1007~~\Rightarrow~~\Omega^2 \approx 0.05</math>. Other properties of this "central" spheroid — such as its mass, moment of inertia, and angular momentum — are given by the following expressions:
|
<math>~M_c</math> |
<math>~=</math> |
<math>~\frac{4\pi}{3} \rho R_\mathrm{eq}^2 Z = \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \, ;</math> |
|
<math>~I_c</math> |
<math>~=</math> |
<math>~\frac{2}{5} M_c R_\mathrm{eq}^2 \, ;</math> |
|
<math>~L_c</math> |
<math>~=</math> |
<math>~I_c \omega_0 = \frac{2}{5} M_c R_\mathrm{eq}^2 \omega_0 </math> |
|
|
<math>~=</math> |
<math>~\frac{2}{5} \biggl[ \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr] R_\mathrm{eq}^2 \omega_0 </math> |
|
|
<math>~=</math> |
<math>~\frac{2^3\pi}{3\cdot 5} (1 - e^2)^{1 / 2} R_\mathrm{eq}^5 \rho \omega_0 </math> |
|
<math>~\Rightarrow ~~~L^2_c</math> |
<math>~=</math> |
<math>~4\pi G\biggl( \frac{2^3\pi}{3\cdot 5}\biggr)^2 (1 - e^2) R_\mathrm{eq}^{10} \rho^3 \Omega^2 </math> |
|
|
<math>~=</math> |
<math>~\biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr) (1 - e^2) G R_\mathrm{eq}^{10} \rho^3 \Omega^2 \, . </math> |
We note as well that the (square of the) Keplerian frequency for a massless particle orbiting in the equatorial plane at a distance, <math>~r</math>, from the center of this central object will be,
|
<math>~\omega_K^2</math> |
<math>~=</math> |
<math>~\frac{GM_c}{r^3}</math> |
|
|
<math>~=</math> |
<math>~\frac{G}{r^3} \biggl[ \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr] \, .</math> |
So, if we force this orbital frequency to also equal the spin-frequency of the Maclaurin spheroid, the radius of the orbit must be,
|
<math>~\Omega^2 (4\pi G\rho)</math> |
<math>~=</math> |
<math>~\frac{G}{r^3} \biggl[ \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr] </math> |
|
<math>~\Rightarrow~~~\Omega^2 </math> |
<math>~=</math> |
<math>~\frac{1}{r^3} \biggl[ \frac{1}{3} R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr] </math> |
|
<math>~\Rightarrow~~~\frac{r}{R_\mathrm{eq}} </math> |
<math>~=</math> |
<math>~\biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr]^{1 / 3} \, .</math> |
For example, when <math>~(e, \Omega^2) = (0.6, 0.05)</math>, we have, <math>~r/R_\mathrm{eq} = (5.3333)^{1 / 3} = 1.747</math>.
Surrounding Torus
We'll assume that the surrounding 2nd object is a thin torus (1) with the same density as the central object, (2) with a major axis, <math>~a</math>, which ensures that the torus is spinning with the Keplerian frequency prescribed by the mass of the central object, (3) and with a minor cross-sectional radius, <math>~b</math>. The second of these constraints means that,
|
<math>~\alpha_t \equiv \frac{a}{R_\mathrm{eq}} </math> |
<math>~=</math> |
<math>~\biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr]^{1 / 3} \, .</math> |
The mass of the torus is given by the expression,
|
<math>~M_t</math> |
<math>~=</math> |
<math>~2\pi a (\pi b^2)\rho = 2\pi^2 R_\mathrm{eq}^3 \rho \biggl[ \alpha_t \beta_t^2 \biggr] \, ,</math> |
where,
|
<math>~\beta_t</math> |
<math>~\equiv</math> |
<math>~\frac{b}{R_\mathrm{eq}} \, .</math> |
Given that <math>~\alpha_t</math> is known once the eccentricity of the central Maclaurin spheroid has been selected and, given that the density of the torus must match the density of the central object, the mass of the torus will only depend on the choice of <math>~0 < \beta_t \le \beta_\mathrm{max}</math>. The maximum allowed value, <math>~\beta_\mathrm{max}</math>, is set by ensuring that equatorial-plane location of the inner edge of the torus is no smaller than the equatorial radius of the central spheroid, <math>~R_\mathrm{eq}</math>. This means,
|
<math>~\beta_\mathrm{max}</math> |
<math>~=</math> |
<math>~\alpha_t - 1 \, .</math> |
So, the maximum torus mass is,
|
<math>~M_\mathrm{max}</math> |
<math>~=</math> |
<math>~ 2\pi^2 R_\mathrm{eq}^3 \rho \biggl[ \alpha_t \beta_\mathrm{max}^2 \biggr] = 2\pi^2 R_\mathrm{eq}^3 \rho ~\alpha_t (\alpha_t - 1)^2 \, . </math> |
The moment of inertia of the torus is,
|
<math>~I_t</math> |
<math>~=</math> |
<math>~M_t R_\mathrm{eq}^2\biggl[ \biggl(\frac{a}{R_\mathrm{eq}} \biggr)^2 + \frac{3}{4} \biggl( \frac{b}{R_\mathrm{eq}} \biggr)^2 \biggr]</math> |
|
|
<math>~=</math> |
<math>~2\pi^2 R_\mathrm{eq}^5 \rho \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \, .</math> |
Hence, the (square of the) angular momentum of the torus is,
|
<math>~L_t^2 = I_t^2 \omega_0^2</math> |
<math>~=</math> |
<math>~ \biggl[ 2\pi^2 R_\mathrm{eq}^5 \rho \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \biggr]^2 \Omega^2 (4\pi G\rho) </math> |
|
|
<math>~=</math> |
<math>~ 2^4 \pi^5 \alpha_t^2 \beta_t^4 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr)^2 G R_\mathrm{eq}^{10} \rho^3 \Omega^2 \, . </math> |
Combined Configuration
Given that, for the chosen Maclaurin spheroid,
|
<math>~\bar{a}^3</math> |
<math>~=</math> |
<math>~R_\mathrm{eq}^2 Z = R_\mathrm{eq}^3(1-e^2)^{1 / 2} \, ,</math> |
and that the total mass of the system is,
|
<math>~M_\mathrm{tot}</math> |
<math>~=</math> |
<math>~M_c + M_t</math> |
|
|
<math>~=</math> |
<math>~ \frac{2^2\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} + 2\pi^2 R_\mathrm{eq}^3 \rho \alpha_t \beta_t^2 </math> |
|
|
<math>~=</math> |
<math>~ 2\pi R_\mathrm{eq}^3 \rho \biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr] \, , </math> |
the (square of the) dimensionless total angular momentum of the combined system is,
|
<math>~j_\mathrm{tot}^2</math> |
<math>~\equiv</math> |
<math>~ \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \frac{(L_c + L_t)^2}{GM_\mathrm{tot}^3 \bar{a}} </math> |
|
|
<math>~=</math> |
<math>~ \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \biggl\{ L_c + L_t \biggr\}^2 \biggl\{ M_\mathrm{tot} \biggr\}^{-3}G^{-1} R_\mathrm{eq}^{-1} (1 - e^2)^{-1 / 6} </math> |
|
|
<math>~=</math> |
<math>~ \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \biggl\{ \biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr)^{1 / 2} (1 - e^2)^{1 / 2} G^{1 / 2} R_\mathrm{eq}^{5} \rho^{3 / 2} \Omega + 2^2 \pi^{5 / 2} \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) G^{1 / 2} R_\mathrm{eq}^{5} \rho^{3 / 2} \Omega \biggr\}^2 </math> |
|
|
|
<math>~\times \biggl\{ 2\pi R_\mathrm{eq}^3 \rho \biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr] \biggr\}^{-3}G^{-1} R_\mathrm{eq}^{-1} (1 - e^2)^{-1 / 6} </math> |
|
|
<math>~=</math> |
<math>~\frac{1}{2^3 \pi^3} \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \biggl\{ \biggl( \frac{2^8 \pi^3}{3^2 \cdot 5^2}\biggr)^{1 / 2} (1 - e^2)^{1 / 2} \Omega + 2^2 \pi^{5 / 2} \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \Omega \biggr\}^2 \biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr]^{-3} (1 - e^2)^{-1 / 6} </math> |
|
|
<math>~=</math> |
<math>~2\Omega^2 \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \biggl\{ \biggl( \frac{2^2 }{3 \cdot 5}\biggr) (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggl( \alpha_t^2 + \frac{3}{4} \beta_t^2 \biggr) \biggr\}^2 \biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} + \pi \alpha_t \beta_t^2 \biggr]^{-3} (1 - e^2)^{-1 / 6} \, . </math> |
Notice that when the toroidal component is omitted, this expression reduces to,
|
<math>~j_c^2</math> |
<math>~=</math> |
<math>~2\Omega^2 \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \biggl\{ \biggl( \frac{2^2}{3 \cdot 5}\biggr) (1 - e^2)^{1 / 2} \biggr\}^2 \biggl[ \frac{2}{3} (1 - e^2)^{1 / 2} \biggr]^{-3} (1 - e^2)^{-1 / 6} </math> |
|
|
<math>~=</math> |
<math>~2\Omega^2 \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \biggl( \frac{2^4}{3^2 \cdot 5^2}\biggr) \biggl(\frac{3^3}{2^3}\biggr) (1 - e^2)^{-3 / 2} (1 - e^2)^{-1 / 6} (1 - e^2) </math> |
|
|
<math>~=</math> |
<math>~\Omega^2 \biggl(\frac{3}{2^8 \pi^4}\biggr)^{1 / 3} \biggl( \frac{2^2 \cdot 3}{5^2}\biggr) (1 - e^2)^{-2 / 3} </math> |
|
|
<math>~=</math> |
<math>~\Omega^2 \biggl(\frac{3^4}{2^2 \cdot 5^6 \pi^4}\biggr)^{1 / 3} (1 - e^2)^{-2 / 3} \, . </math> |
This matches the expression for the isolated Maclaurin spheroid derived above.
Fixed Mass Ratio
Let's define the mass ratio,
|
<math>~q</math> |
<math>~\equiv</math> |
<math>~ \frac{M_t}{M_c} \, , </math> |
and build a sequence along which this ratio is held constant. For the problem being considered here, the relevant expression for <math>~q</math> is,
|
<math>~q</math> |
<math>~=</math> |
<math>~ \biggl[ 2\pi^2 R_\mathrm{eq}^3 \rho \alpha_t \beta_t^2 \biggr] \biggl[ \frac{4\pi}{3} \rho R_\mathrm{eq}^3 (1 - e^2)^{1 / 2} \biggr]^{-1} </math> |
|
|
<math>~=</math> |
<math>~ \biggl( \frac{3\pi}{2} \biggr) \alpha_t \beta_t^2 (1 - e^2)^{- 1 / 2}\, . </math> |
The sequence is constructed by choosing <math>~q</math> (held fixed), and varying the value of <math>~0 < \beta_t \le \beta_\mathrm{max}</math>; then, for each chosen parameter pair, recognize that,
|
<math>~ \alpha_t (1 - e^2)^{- 1 / 2} </math> |
<math>~=</math> |
<math>~\biggl( \frac{2}{3\pi} \biggr) \frac{q}{ \beta_t^2 } \, ,</math> |
that is, from the above expression for <math>~\alpha_t</math>,
|
<math>~ \biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr] (1 - e^2)^{- 3 / 2} </math> |
<math>~=</math> |
<math>~\biggl[ \biggl( \frac{2}{3\pi} \biggr) \frac{q}{ \beta_t^2 } \biggr]^3</math> |
|
<math>~\Rightarrow~~~ \Omega^2 (1 - e^2) </math> |
<math>~=</math> |
<math>~\frac{1}{3}\biggl[ \biggl( \frac{3\pi}{2} \biggr) \frac{ \beta_t^2 }{q} \biggr]^3 \, .</math> |
But, for central models along the MLS, we also must satisfy the relation given above, namely,
|
<math>~\Omega^2 </math> |
<math>~=</math> |
<math>~ \frac{1}{2}(3 - 2e^2)(1 - e^2)^{1 / 2} \cdot \frac{\sin^{-1} e}{e^3} - \frac{3(1-e^2)}{2e^2} \, . </math> |
Hence, for each <math>~(q, \beta_t)</math> parameter pair, the relevant central-object eccentricity is given by the root of the relation,
|
<math>~ \frac{1}{2}(3 - 2e^2)(1 - e^2)^{3 / 2} \cdot \frac{\sin^{-1} e}{e^3} - \frac{3(1-e^2)^2 }{2e^2} </math> |
<math>~=</math> |
<math>~\frac{1}{3}\biggl[ \biggl( \frac{3\pi}{2} \biggr) \frac{ \beta_t^2 }{q} \biggr]^3 \, .</math> |
Note that, for the limiting value, <math>~\beta_\mathrm{max} = (\alpha_t - 1)</math>, the relevant relation becomes,
|
<math>~ (\alpha_t - 1)^2\alpha_t (1 - e^2)^{- 1 / 2} </math> |
<math>~=</math> |
<math>~\frac{2q}{3\pi} </math> |
where again, as above
|
<math>~\alpha_t \equiv \frac{a}{R_\mathrm{eq}} </math> |
<math>~=</math> |
<math>~\biggl[\frac{1}{3\Omega^2} (1 - e^2)^{1 / 2} \biggr]^{1 / 3} \, .</math> |
See Also
- Université de Bordeaux (Part 1): External Gravitational Potential of Toroids
- Université de Bordeaux (Part 3): Discussions Following Dissertation Defense

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |