Difference between revisions of "User:Tohline/SSC/Stability/BiPolytrope0 0"
(→Eigenfunctions: Work through first derivative of core's j=2 eigenfunction) |
|||
| (124 intermediate revisions by the same user not shown) | |||
| Line 2: | Line 2: | ||
<!-- __NOTOC__ will force TOC off --> | <!-- __NOTOC__ will force TOC off --> | ||
=Radial Oscillations of a Zero-Zero Bipolytrope= | =Radial Oscillations of a Zero-Zero Bipolytrope= | ||
This chapter includes the interface-matching condition specified by [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)]. It replaces [[User:Tohline/SSC/Stability/BiPolytrope0_0Old#Radial_Oscillations_of_a_Zero-Zero_Bipolytrope|an earlier overview chapter]], which summarized models in which an incorrect interface matching condition was used. | |||
{{LSU_HBook_header}} | {{LSU_HBook_header}} | ||
== | |||
In a [[User:Tohline/SSC/Structure/BiPolytropes/Analytic0_0#BiPolytrope_with_nc_.3D_0_and_ne_.3D_0|separate chapter on astrophysical interesting ''equilibrium structures'']], we have derived analytical expressions that define the equilibrium properties of bipolytropic configurations having <math>~(n_c, n_e) = (0, 0)</math>, that is, bipolytropes in which both the core and the envelope are uniform in density, but the densities in the two regions are different from one another. Letting <math>~R</math> be the radius and <math>~M_\mathrm{tot}</math> be the total mass of the bipolytrope, these configurations are fully defined once any two of the following three key parameters have been specified: The envelope-to-core density ratio, <math>~\rho_e/\rho_c</math>; the radial location of the envelope/core interface, <math>~q \equiv r_i/R</math>; and, the fractional mass that is contained within the core, <math>~\nu \equiv M_\mathrm{core}/M_\mathrm{tot}</math>. These three parameters are related to one another via the expression, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\rho_e}{\rho_c}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{q^3}{\nu} \biggl( \frac{1-\nu}{1-q^3} \biggr) \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Equilibrium configurations can be constructed that have a wide range of parameter values; specifically, | |||
<div align="center"> | |||
<math>~0 \le q \le 1 \, ;</math> | |||
| |||
<math>~0 \le \nu \le 1 \, ;</math> | |||
| |||
and, | |||
| |||
<math>~0 \le \frac{\rho_e}{\rho_c} \le 1 \, .</math> | |||
</div> | |||
(We recognize from buoyancy arguments that any configuration in which the envelope density is larger than the core density will be Rayleigh-Taylor unstable, so we restrict our astrophysical discussion to structures for which <math>~\rho_e < \rho_c</math>.) | |||
By employing the [[User:Tohline/SSC/Perturbations#Spherically_Symmetric_Configurations_.28Stability_.E2.80.94_Part_II.29|linear stability analysis techniques described in an accompanying chapter]], we should, in principle, be able to identify a wide range of eigenvectors — that is, radial eigenfunctions and accompanying eigenfrequencies — that are associated with adiabatic radial oscillation modes in any one of these equilibrium, bipolytropic configurations. Using numerical techniques, [http://adsabs.harvard.edu/abs/1985PASAu...6..222M Murphy & Fiedler (1985)], for example, have carried out such an analysis of bipolytropic structures having <math>~(n_c, n_e) = (1,5)</math>. A ''pair'' of [[User:Tohline/SSC/Perturbations#2ndOrderODE|linear adiabatic wave equations (LAWEs)]] must be solved — one tuned to accommodate the properties of the core and another tuned to accommodate the properties of the envelope — then the pair of eigenfunctions must be matched smoothly at the radial location of the interface; the identified core- and envelope-eigenfrequencies must simultaneously match. | |||
After identifying the precise form of the LAWEs that apply to the case of <math>~(n_c, n_e) = (0,0)</math> bipolytropes, we discovered that, for a restricted range of key parameters, the pair of equations can both be solved ''analytically''. | |||
==Two Separate LAWEs== | |||
In an [[User:Tohline/SSC/Perturbations#2ndOrderODE|accompanying discussion]], we derived the so-called, | In an [[User:Tohline/SSC/Perturbations#2ndOrderODE|accompanying discussion]], we derived the so-called, | ||
| Line 23: | Line 61: | ||
</div> | </div> | ||
--> | --> | ||
For both regions of the bipolytrope, we define the dimensionless (Lagrangian) radial coordinate, | |||
<div align="center"> | |||
<math>~\xi \equiv \frac{r_0}{r_i} \, .</math> | |||
</div> | |||
So, the interface is, by definition, located at <math>~\xi = 1</math>; and, the surface is necessarily at <math>~\xi = q^{-1}</math>. As the material in the bipolytrope's core (envelope) is compressed/de-compressed during a radial oscillation, we will assume that heating/cooling occurs in a manner prescribed by an adiabat of index <math>~\gamma_c ~(\gamma_e)</math>; in general, <math>~\gamma_e \ne \gamma_c</math>. For convenience, we will also adopt the frequently used shorthand "alpha" notation, | |||
<div align="center"> | |||
<math>~\alpha_c \equiv 3 - \frac{4}{\gamma_c} \, ,</math> | |||
and | |||
<math>~\alpha_e \equiv 3 - \frac{4}{\gamma_e} \, .</math> | |||
</div> | |||
===The Core's LAWE=== | |||
After adopting, for convenience, the function notation, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 29: | Line 80: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~g^2</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 35: | Line 86: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
1 + \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \biggl( 1-q \biggr) + | |||
\frac{\rho_e}{\rho_c} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] \, , | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
in | we [[User:Tohline/SSC/Stability/BiPolytrope0_0Details#Match_Prasad-like_Envelope_Eigenvector_to_the_Core_Eigenvector|have deduced]] that, for the core, the LAWE may be written in the form, | ||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 46: | Line 101: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 52: | Line 107: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ \ | <math>~ | ||
(1 - \eta^2)\frac{d^2x}{d\eta^2} + | |||
( 4 - 6\eta^2 ) \frac{1}{\eta} \cdot \frac{dx}{d\eta} | |||
+ \mathfrak{F}_\mathrm{core} x \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
where, | |||
<div align="center"> | <div align="center"> | ||
<math>~ | <math>~\eta \equiv \frac{\xi}{g} \, ,</math> | ||
<math>~ | and | ||
<math>~\mathfrak{F}_\mathrm{core} \equiv \frac{3\omega_\mathrm{core}^2}{2\pi G\gamma_c \rho_c} - 2\alpha_c\, .</math> | |||
</div> | </div> | ||
Not surprisingly, this is identical in form to the eigenvalue problem that was first presented — and solved analytically — by [[User:Tohline/SSC/UniformDensity#Setup_as_Presented_by_Sterne_.281937.29|Sterne (1937)]] in connection with his examination of radial oscillations in ''isolated'' uniform-density spheres. As is demonstrated below, for the core of our zero-zero bipolytrope, we can in principle adopt any one of the [[User:Tohline/SSC/UniformDensity#Sterne.27s_General_Solution|polynomial eigenfunctions and corresponding eigenfrequencies]] derived by Sterne. | |||
===The Envelope's LAWE=== | |||
Subsequently, we also [[User:Tohline/SSC/Stability/BiPolytrope0_0Details#More_General_Solution|have deduced]] that, for the envelope, the governing LAWE becomes, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 69: | Line 134: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 75: | Line 140: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\biggl( \frac{ | <math>~ | ||
\biggl[ 1 + \frac{(g^2-\mathcal{B}) \xi}{\mathcal{A}} - \mathcal{D} \xi^3\biggr] \frac{d^2x}{d\xi^2} | |||
+ \biggl\{ 3 + \frac{4(g^2-\mathcal{B}) \xi}{\mathcal{A}} - 6\mathcal{D} \xi^3 \biggr\} | |||
\, ,</math> | \frac{1}{\xi} \cdot \frac{dx}{d\xi} | ||
+ \biggl[ | |||
\mathcal{D} \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e | |||
\biggr]\frac{x}{\xi^2} \, , | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
where, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\mathcal{A}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\rho_c \, | <math>~2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \, ; | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 96: | Line 171: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\mathcal{B}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~1 | <math>~1 + 2\biggl(\frac{\rho_e}{\rho_c}\biggr) - 3\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 | ||
\, , | |||
</math> | |||
\, ,</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 113: | Line 185: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\mathcal{D}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{ | <math>~\frac{1}{\mathcal{A}}\biggl( \frac{\rho_e}{\rho_c}\biggr)^2 = \biggl[ \frac{\rho_e/\rho_c}{2(1-\rho_e/\rho_c)} \biggr] | ||
= | \, , | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 128: | Line 199: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\mathfrak{F}_\mathrm{env}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} - 2\alpha_e | ||
\frac | \, . | ||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
<span id="KeyConstraint">We have been unable</span> to demonstrate that this governing equation can be solved analytically for ''arbitrary'' pairs of the key model parameters, <math>~q</math> and <math>~\rho_e/\rho_c</math>. But, if we choose parameter value pairs that satisfy the constraint, | |||
<div align="center"> | |||
<math>~g^2 = \mathcal{B} </math> | |||
<math>~\Rightarrow</math> | |||
<math>~g^2 = \frac{1+8q^3}{(1+2q^3)^2} \, ,</math> | |||
and, | |||
<math>~q^3 = \mathcal{D} = \biggl[ \frac{\rho_e/\rho_c}{2(1-\rho_e/\rho_c)} \biggr] </math> | |||
<math>~\Rightarrow</math> | |||
<math>~\frac{\rho_e}{\rho_c} = \frac{2q^3}{1+2q^3} \, ,</math> | |||
</div> | |||
then the LAWE that is relevant to the envelope simplifies. Specifically, it takes the form, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~0</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 152: | Line 238: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
( 1 - q^3 \xi^3 ) \frac{d^2x}{d\xi^2} + ( 3 - 6q^3 \xi^3 ) \frac{1}{\xi} \cdot \frac{dx}{d\xi} | |||
+ | |||
\frac{ | \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e | ||
\biggr]\frac{x}{\xi^2} | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 167: | Line 254: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{x}{\xi^2}\biggl\{ | ||
( 1 - q^3 \xi^3 ) | |||
\biggl[ \frac{d}{d\ln\xi} \biggl( \frac{d\ln x}{d\ln \xi} \biggr) - \biggl( 1 - \frac{d\ln x}{d\ln \xi} \biggr)\cdot \frac{d\ln x}{d\ln \xi}\biggr] | |||
+ ( 3 - 6q^3 \xi^3 ) \frac{d\ln x}{d\ln \xi} | |||
+ \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e | |||
\biggr] \biggr\} | |||
\, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 174: | Line 266: | ||
</table> | </table> | ||
</div> | </div> | ||
where, | Shortly after deriving this last expression, we realized that one possible solution is a simple power-law eigenfunction of the form, | ||
<div align="center"> | |||
<math>~x=a_0 \xi^{c_0} \, ,</math> | |||
</div> | |||
where the (constant) exponent is one of the roots of the quadratic equation, | |||
<div align="center"> | |||
<math>~c_0^2 + 2c_0 - \alpha_e = 0 \, ,</math> | |||
<math>~\Rightarrow</math> | |||
<math>~c_0 = -1 \pm \sqrt{1+\alpha_e} \, .</math> | |||
</div> | |||
This power-law eigenfunction must be paired with the associated, dimensionless eigenfrequency parameter, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 180: | Line 282: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\mathfrak{F}_\mathrm{env}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~c_0(c_0+5) = 3c_0 + \alpha_e</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 195: | Line 294: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{\ | <math>~\Rightarrow ~~~ \frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 201: | Line 300: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ 3(c_0 + \alpha_e) = 3[\alpha_e -1 \pm \sqrt{1+\alpha_e}] \, .</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
Next, [[User:Tohline/SSC/Stability/BiPolytrope0_0Details#Eureka_Regarding_Prasad.27s_1948_Paper|we noticed]] the strong similarities between the mathematical properties of this eigenvalue problem and the one that was studied by [http://adsabs.harvard.edu/abs/1948MNRAS.108..414P C. Prasad (1948, MNRAS, 108, 414-416)] in connection with, what we now recognize to be, a closely related problem. Drawing heavily from Prasad's analysis, we discovered an infinite number of eigenfunctions (each, a truncated polynomial expression) and associated eigenfrequencies that satisfy this governing envelope LAWE. The eigenvectors associated with the lowest few modes are tabulated, below. | |||
==Eigenvector== | |||
===Core Segment=== | |||
<div align="center" id="Table1"> | |||
<table border="1" cellpadding="8"> | |||
<tr> | |||
<th align="center" colspan="3"><font size="+1">Table 1:</font> Analytically Specifiable Core Eigenvectors</th> | |||
</tr> | |||
<tr> | <tr> | ||
<td align=" | <td align="center" colspan="1"> | ||
Mode | |||
</td> | </td> | ||
<td align="center"> | <td align="center" colspan="1"> | ||
<math> | Core Eigenfunction<br /> | ||
<br /> | |||
<math> | |||
g^2 \equiv 1 + \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \biggl( 1-q \biggr) + | |||
\frac{\rho_e}{\rho_c} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] | |||
</math> | |||
</td> | </td> | ||
<td align=" | <td align="center">Core Eigenfrequency <br /> | ||
<math>~\frac{ | <math>~\frac{3\omega_\mathrm{core}^2}{2\pi \gamma_c G \rho_c} = 2[\alpha_c + j(2j+5)]</math> | ||
\ | |||
</td> | </td> | ||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~j=0 </math> | |||
</td> | |||
<td align="left"> | |||
<math>~x_\mathrm{core} = a_0 </math> | |||
</td> | |||
<td align="center"> | |||
<math>~6-8/\gamma_c</math> | |||
</td> | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="center"> | |||
<math>~j=1 </math> | |||
</td> | |||
<td align="left"> | |||
<math>~=</math> | <math>~x_\mathrm{core} = a_0 \biggl[ 1 - \frac{7}{5}\biggr(\frac{\xi^2}{g^2}\biggr) \biggr]</math> | ||
</td> | |||
<td align="center"> | |||
<math>~ | <math>~20-8/\gamma_c</math> | ||
</td> | |||
\ | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="center"> | |||
| <math>~j=2 </math> | ||
</td> | |||
<td align="left"> | |||
<math>~x_\mathrm{core} = a_0 \biggl[ 1 - \frac{18}{5}\biggr(\frac{\xi^2}{g^2}\biggr) + \frac{99}{35}\biggr(\frac{\xi^2}{g^2}\biggr)^2 \biggr]</math> | |||
</td> | |||
<td align="center"> | |||
<math>~42-8/\gamma_c</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
===Envelope Segment=== | |||
<div align="center" id="Table2"> | |||
<table border="1" cellpadding="8"> | |||
<tr> | |||
<th align="center" colspan="3"><font size="+1">Table 2:</font> Analytically Specifiable Envelope Eigenvectors</th> | |||
</tr> | |||
<tr> | |||
<td align="center" colspan="1"> | |||
Mode | |||
</td> | |||
<td align="center" colspan="1"> | |||
Envelope Eigenfunction<br /> | |||
<br /> | |||
<math>~c_0 \equiv -1 \pm \sqrt{1+\alpha_e}</math> | |||
</td> | |||
<td align="center">Envelope Eigenfrequency<br /> | |||
<math>~\frac{3\omega_\mathrm{env}^2}{2\pi \gamma_e G \rho_e} = 3[\alpha_e + c_0(2\ell+1) + \ell(3\ell+5)]</math><br /><br /><math>~=2\alpha_e + (c_0+3\ell)(c_0+3\ell + 5)</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~\ell=0 </math> | |||
</td> | |||
<td align="left"> | |||
<math>~x_\mathrm{env} = b_0 \xi^{c_0}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~3[\alpha_e + c_0]</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~\ell=1 </math> | |||
</td> | |||
<td align="left"> | |||
<math>~x_\mathrm{env} = b_0 \xi^{c_0}\biggl\{1 + \biggl[ | |||
\frac{c_0(c_0+5)-(c_0+3)(c_0+8)}{(c_0+3)(c_0+5) - \alpha_e} | |||
\biggr](q\xi)^3 \biggr\}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~3[\alpha_e + 3c_0 +8]</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~\ell=2 </math> | |||
</td> | |||
<td align="left"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~x_\mathrm{env} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 248: | Line 432: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~b_0 \xi^{c_0}\biggl\{1 + \biggl[ \frac{c_0(c_0+5)-(c_0+6)(c_0+11)}{(c_0+3)(c_0+5) - \alpha_e}\biggr](q\xi)^3 | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 256: | Line 439: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
+ \biggl[ \frac{c_0(c_0+5)-(c_0+6)(c_0+11)}{(c_0+3)(c_0+5) - \alpha_e}\biggr]\biggl[ \frac{(c_0+3)(c_0+8)-(c_0+6)(c_0+11)}{(c_0+6)(c_0+8) - \alpha_e}\biggr](q\xi)^6 | |||
\biggr\} | |||
</math> | |||
\ | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
<math> | </td> | ||
\ | <td align="center"> | ||
<math>~3[\alpha_e + 5c_0 +22]</math> | |||
</td> | |||
</tr> | |||
</math> | </table> | ||
</ | </div> | ||
===Piecing Together=== | |||
Here we illustrate how the two segments of the eigenfunction can be successfully pieced together for the specific case of <math>~(\ell,j) = (2,1)</math>. | |||
<span id="STEP1"><font color="red"><b>STEP 1:</b></font></span> Choose a value of the adiabatic exponent for the envelope, <math>~\gamma_e</math>. Then, the values of both <math>~\alpha_e</math> and <math>~c_0</math> are known as well; actually, because it is the root of a quadratic equation, <math>~c_0</math> can, in general, take on one of a ''pair'' of values. We will elaborate on this further, below. | |||
<span id="STEP2"><font color="red"><b>STEP 2:</b></font></span> Acknowledging that the value of <math>~q</math> has yet to be determined, fix the value of the leading, overall scaling coefficient, <math>~b_0</math>, such that<sup>†</sup> <math>~x_\mathrm{env} = 1</math> at the interface, that is, at <math>~\xi = 1</math>. For the case of <math>~\ell=2</math>, this means that, throughout the envelope, the eigenfunction is, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 290: | Line 473: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~x_{\ell=2} |_\mathrm{env}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 297: | Line 480: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\ | \xi^{c_0}\biggl[ \frac{ 1 + q^3 A_{2} \xi^{3} + q^6 A_{2}B_{2}\xi^{6} }{ 1 + q^3 A_{2} + q^6 A_{2}B_{2}}\biggr] \, , | ||
+ | |||
+ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
where, the values of the newly introduced coefficients, | |||
<div align="center"> | |||
<table border="0" cellpadding="3" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~A_{2}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\biggl[ \frac{c_0(c_0+5) - (c_0 + 6)(c_0 + 11)}{(c_0 + 3)(c_0+5) - \alpha_e}\biggr] \, ,</math> | ||
( | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 323: | Line 503: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~B_{2}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~\equiv</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\biggl[ \frac{(c_0+3)(c_0+8) - (c_0 + 6)(c_0 + 11)}{(c_0 + 6)(c_0+8) - \alpha_e}\biggr] \, ,</math> | ||
+ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
are also both known. | |||
<span id="STEP3"><font color="red"><b>STEP 3:</b></font></span> Recognizing that this segment of the eigenfunction will only satisfy the envelope's LAWE if we restrict our discussion to equilibrium models for which <math>~g^2 = \mathcal{B} = [(1+8q^3)/(1+2q^3)^{2}]</math>, we must insert this same restriction on <math>~g^2</math> into the core's eigenfunction. At the same time, we should fix the value of the leading, overall scaling coefficient, <math>~a_0</math>, such that<sup>†</sup> <math>~x_\mathrm{core} = 1</math> at the interface <math>~(\xi = 1)</math>. For the case of <math>~j=1</math>, this means that, throughout the core, the eigenfunction is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~x_{j=1} |_\mathrm{core}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 345: | Line 530: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{1 | \frac{5(1+8q^3) - 7 (1+2q^3)^2 \xi^2}{5(1+8q^3)-7(1+2q^3)^2} \, .</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
<span id="STEP4"><font color="red"><b>STEP 4:</b></font></span> Now we need to match the two eigenfunctions at the interface. Following the discussion in §§57 & 58 of [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)], the proper treatment is to ensure that fractional perturbation in the gas pressure (see their equation 57.31), | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{\delta P}{P}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~- \gamma x \biggl( 3 + \frac{d\ln x}{d\ln \xi} \biggr) \, ,</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
is continuous across the interface. That is to say, at the interface <math>~(\xi = 1)</math>, we need to enforce the relation, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~0</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 375: | Line 567: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\biggl[ \gamma_c x_\mathrm{core} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \gamma_e x_\mathrm{env} \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=1}</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 392: | Line 579: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\gamma_e \biggl[ \frac{\gamma_c}{\gamma_e} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=1}</math> | ||
\frac{ | |||
+ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\Rightarrow~~~ \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr|_{\xi=1}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 415: | Line 591: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl( \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr)_{\xi=1} \, .</math> | ||
( | |||
\, | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
In the context of this interface-matching constraint (see their equation 62.1), [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)] state the following: <font color="darkgreen"><b>In the static</b></font> (''i.e.,'' unperturbed equilibrium) <font color="darkgreen"><b>model</b></font> … <font color="darkgreen"><b>discontinuities in <math>~\rho</math> or in <math>~\gamma</math> might occur at some [radius]</b></font>. <font color="darkgreen"><b>In the first case</b></font> — that is, a discontinuity only in density, while <math>~\gamma_e = \gamma_c</math> — the interface conditions <font color="darkgreen"><b>imply the continuity of <math>~\tfrac{1}{x} \cdot \tfrac{dx}{d\xi}</math> at that [radius]. In the second case</b></font> — that is, a discontinuity in the adiabatic exponent — <font color="darkgreen"><b>the dynamical condition may be written</b></font> as above. <font color="darkgreen"><b>This implies a discontinuity of the first derivative at any discontinuity of <math>~\gamma</math></b></font>. | |||
When evaluated at the interface, the logarithmic derivatives of our pair of parameterized eigenfunction expressions are, respectively, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math> | <math>\frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr|_{\xi=1}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 429: | Line 610: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~ | ||
c_0 + \frac{3A_{2}\Chi + 6A_{2}B_{2} \Chi^2}{1 + A_{2}\Chi + A_{2}B_{2}\Chi^2} \, ; | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 435: | Line 618: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math> | <math> \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr|_{\xi=1}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 441: | Line 624: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~\frac{14(1+2\Chi)^2}{7(1+2\Chi)^2 - 5(1+8\Chi)} \, ,</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
where we have made the notation substitution, <math>~\Chi \equiv q^3</math>. Allowing for a step function in the adiabatic exponent at the interface, our interface-matching constraint is, therefore, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | |||
\frac{\gamma_c}{\gamma_e} \biggl[ \frac{14(1+2\Chi)^2}{7(1+2\Chi)^2 - 5(1+8\Chi)} \biggr] | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 457: | Line 643: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ | ||
c_0 + \frac{3A_{2}\Chi + 6A_{2}B_{2} \Chi^2}{1 + A_{2}\Chi + A_{2}B_{2}\Chi^2} | |||
- 3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 467: | Line 652: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 473: | Line 658: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ | ||
g | \frac{\mathfrak{g}_0 + (\mathfrak{g}_0+3)A_{2}\Chi + (\mathfrak{g}_0+6)A_{2}B_{2} \Chi^2}{1 + A_{2}\Chi + A_{2}B_{2}\Chi^2} | ||
\, , | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
where, | |||
<div align="center"> | |||
<math>~\mathfrak{g}_0 \equiv c_0 + 3\biggl(1-\frac{\gamma_c}{\gamma_e} \biggr) \, .</math> | |||
</div> | |||
This can be rewritten as, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~ | ||
0 | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | |||
<td align="left"> | <td align="left"> | ||
<math>\ | <math>~ | ||
\ | [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] [7(1+2\Chi)^2 - 5(1+8\Chi)] | ||
</td> | - 14(\gamma_c/\gamma_e) (1+2\Chi)^2 [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] | ||
</math> | |||
</td> | |||
</tr> | </tr> | ||
| Line 499: | Line 697: | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | |||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ | ||
\ | [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] [2 - 12\Chi + 28\Chi^2 ] | ||
\ | - (14 + 56\Chi + 56 \Chi^2)(\gamma_c/\gamma_e) [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 514: | Line 712: | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | |||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ | ||
\ | 2[\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] | ||
\ | -12\Chi [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] | ||
+ 28\Chi^2 [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\ | - 14(\gamma_c/\gamma_e) [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] | ||
\ | - 56(\gamma_c/\gamma_e)\Chi [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] | ||
- 56 (\gamma_c/\gamma_e)\Chi^2 [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] \, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 546: | Line 740: | ||
</table> | </table> | ||
</div> | </div> | ||
Or we have, equivalently, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~a\Chi^4 + b\Chi^3 + c\Chi^2 +d\Chi +e </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 571: | Line 752: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~0 \, ,</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
where, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~e</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~ 2\mathfrak{g}_0 - 14(\gamma_c/\gamma_e) \, ,</math> | ||
- | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 599: | Line 773: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~d</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~2(\mathfrak{g}_0+3)A_{2} - 12\mathfrak{g}_0 - 14(\gamma_c/\gamma_e)A_{2} - 56(\gamma_c/\gamma_e)</math> | ||
\ | |||
- | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 618: | Line 788: | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~2[\mathfrak{g}_0 + 3 -7(\gamma_c/\gamma_e)]A_{2} - 4[14(\gamma_c/\gamma_e) + 3\mathfrak{g}_0] \, ,</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 633: | Line 797: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~c</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~2(\mathfrak{g}_0+6)A_{2}B_{2} - 12(\mathfrak{g}_0+3)A_{2} + 28\mathfrak{g}_0 - 14(\gamma_c/\gamma_e)A_{2}B_{2} - 56(\gamma_c/\gamma_e)A_{2} - 56(\gamma_c/\gamma_e) </math> | ||
\ | |||
- | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 652: | Line 812: | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~2[\mathfrak{g}_0 + 6 -7(\gamma_c/\gamma_e)] A_{2}B_{2} - 4[9 + 14(\gamma_c/\gamma_e) + 3\mathfrak{g}_0]A_{2} + 28[\mathfrak{g}_0 - 2(\gamma_c/\gamma_e)] \, ,</math> | ||
+ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~b</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~-12(\mathfrak{g}_0+6)A_{2}B_{2} + 28(\mathfrak{g}_0+3)A_{2} - 56(\gamma_c/\gamma_e)A_{2}B_{2} - 56(\gamma_c/\gamma_e)A_{2} </math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 688: | Line 833: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 694: | Line 839: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~- 4[3\mathfrak{g}_0+18+14(\gamma_c/\gamma_e)]A_{2}B_{2} + 28[\mathfrak{g}_0+3-2(\gamma_c/\gamma_e)]A_{2} \, ,</math> | ||
g | </td> | ||
</math> | </tr> | ||
<tr> | |||
<td align="right"> | |||
<math>~a</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~28[\mathfrak{g}_0+6 - 2(\gamma_c/\gamma_e)]A_{2}B_{2} \, .</math> | |||
</td> | </td> | ||
</tr> | |||
</table> | |||
The physically relevant (real) root of this quartic equation in <math>~\Chi</math> — see our [[User:Tohline/SSC/Stability/BiPolytrope0_0Details#Quartic|accompanying detailed presentation]] — gives us the ''specific'' value of the dimensionless interface location, <math>~q</math>, for which the values of the two eigenfunctions match at the interface, and for which the first derivatives of the two eigenfunctions are discontinuous by the properly prescribed amount at the interface. | |||
===Example Solutions=== | |||
<div align="center" id="Table3"> | |||
<table border="1" cellpadding="5" align="center"> | |||
<tr><th align="center" colspan="6"><font size="+1">Table 3:</font> Example Analytic Model Parameters for <math>~(\ell,j) = (2,1)</math><br />NOTE: <math>\mathfrak{F}_\mathrm{core} = 14</math></th></tr> | |||
<tr> | |||
<td align="center">Eigenfunction</td> | |||
<td align="center"><math>~\gamma_c ~(n_c)</math></td> | |||
<td align="center"><math>~\gamma_e</math></td> | |||
<td align="center"><math>~q</math></td> | |||
<td align="center"><math>~\frac{\rho_e}{\rho_c}</math></td> | |||
<td align="center"><math>~\sigma_c^2</math></td> | |||
</tr> | |||
<tr> | |||
<td>[[File:A21.png|center|thumb|100px|Model A21]] | |||
<td align="center"> <math>~\frac{5}{3} ~~\biggl(\frac{3}{2} \biggr)</math></td> | |||
<td align="center">1.1340607</td> | |||
<td align="center">0.6684554</td> | |||
<td align="center">0.3739731</td> | |||
<td align="center">25.333333</td> | |||
</tr> | |||
<tr> | |||
<td>[[File:B21.png|center|thumb|100px|Model B21]] | |||
<td align="center"> <math>~\frac{4}{3} ~~\biggl( 3 \biggr)</math></td> | |||
<td align="center">1.0263212</td> | |||
<td align="center">0.6385711</td> | |||
<td align="center">0.3424445</td> | |||
<td align="center">18.666666</td> | |||
</tr> | |||
<tr> | |||
<td>[[File:C21.png|center|thumb|100px|Model C21]] | |||
<td align="center"> <math>~\frac{6}{5} ~~\biggl( 5 \biggr)</math></td> | |||
<td align="center">1.0028319</td> | |||
<td align="center">0.6187646</td> | |||
<td align="center">0.3214875</td> | |||
<td align="center">16.000000</td> | |||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
It appears as though the eigenvectors (eigenfunction and eigenfrequency) of other radial oscillation modes can be identified by holding all other parameters fixed but changing the value of the quantum number, <math>~\ell</math>, in the [[#OtherSigmas|expression provided below]]. Picking the configuration identified as model '''C21''' in [[#Table3|Table 3]], for example, in addition to the parameter values provided in the table we have, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 708: | Line 906: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~0</math> | <math>~\alpha_e = 3 - \frac{4}{\gamma_e} = -0.9887044</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
and, | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~c_0 = \sqrt{1+\alpha_e} - 1 = -0.8937192 \, ,</math> | ||
\ | |||
- | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
so we expect the variation in (the square of) the eigenfrequency, <math>~\sigma_c</math>, with <math>~\ell</math> to be, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\sigma_c^2</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~12\biggl[\frac{c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) }{(3-\alpha_e) } \biggr]\frac{\rho_e}{\rho_c} </math> | ||
\frac{ | |||
+ \ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 748: | Line 941: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~0.9671938[c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) ] </math> | ||
+ | |||
+ \ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 768: | Line 953: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~0.9671938[-1.8824236 +(3.2125616)\ell + 3\ell^2 ] \, .</math> | ||
+ | |||
+ | |||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
<div align="center" id="Table4"> | |||
<table border="1" cellpadding="5" align="center"> | |||
<tr> | |||
<th align="center" colspan="6"><font size="+1">Table 4:</font> Additional Hypothesized Oscillation Modes for Model C21</th> | |||
</tr> | |||
<tr> | |||
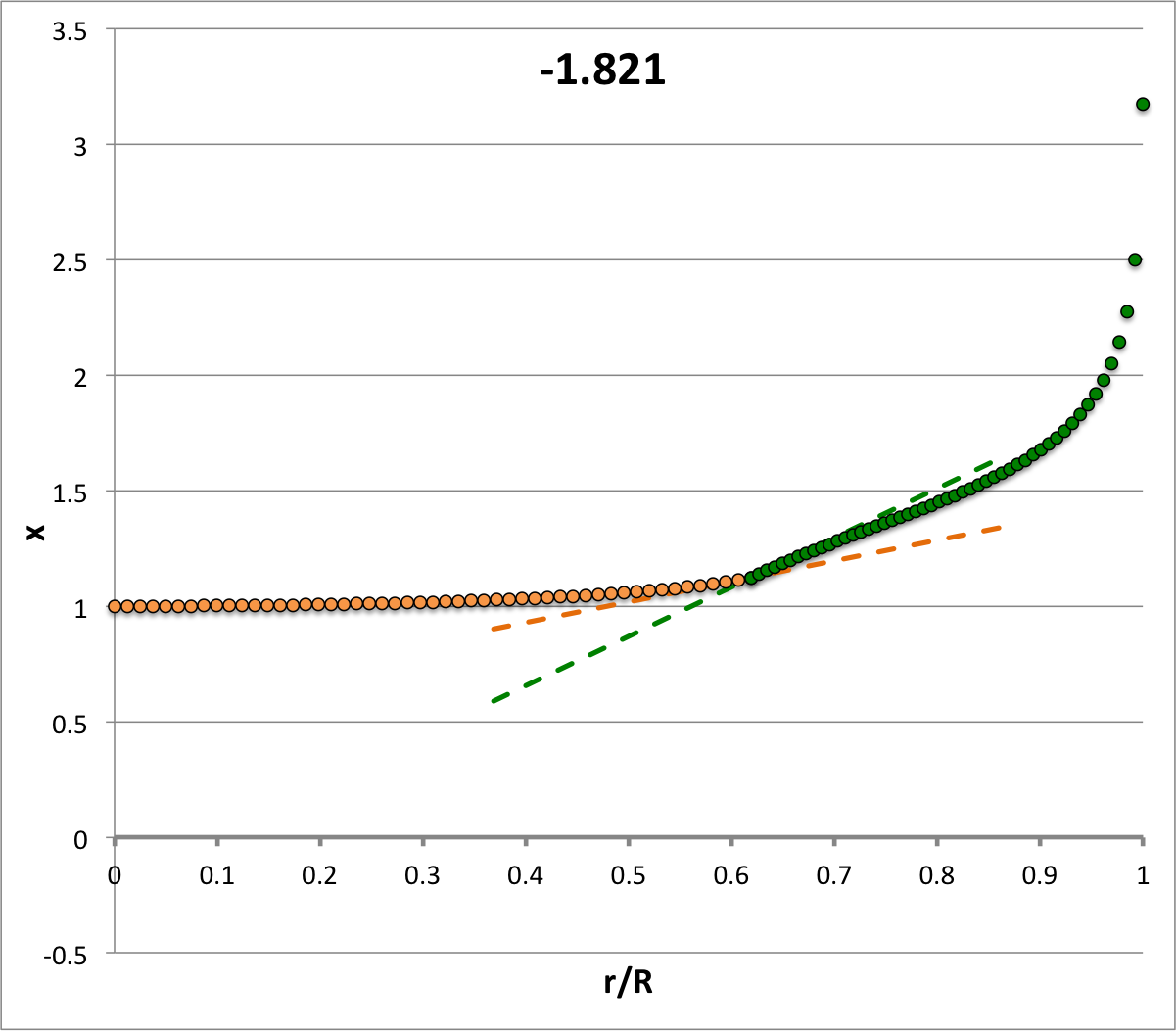
<td align="center"><math>~\ell = 0</math><p></p><math>~\sigma_c^2 = -1.821</math></td> | |||
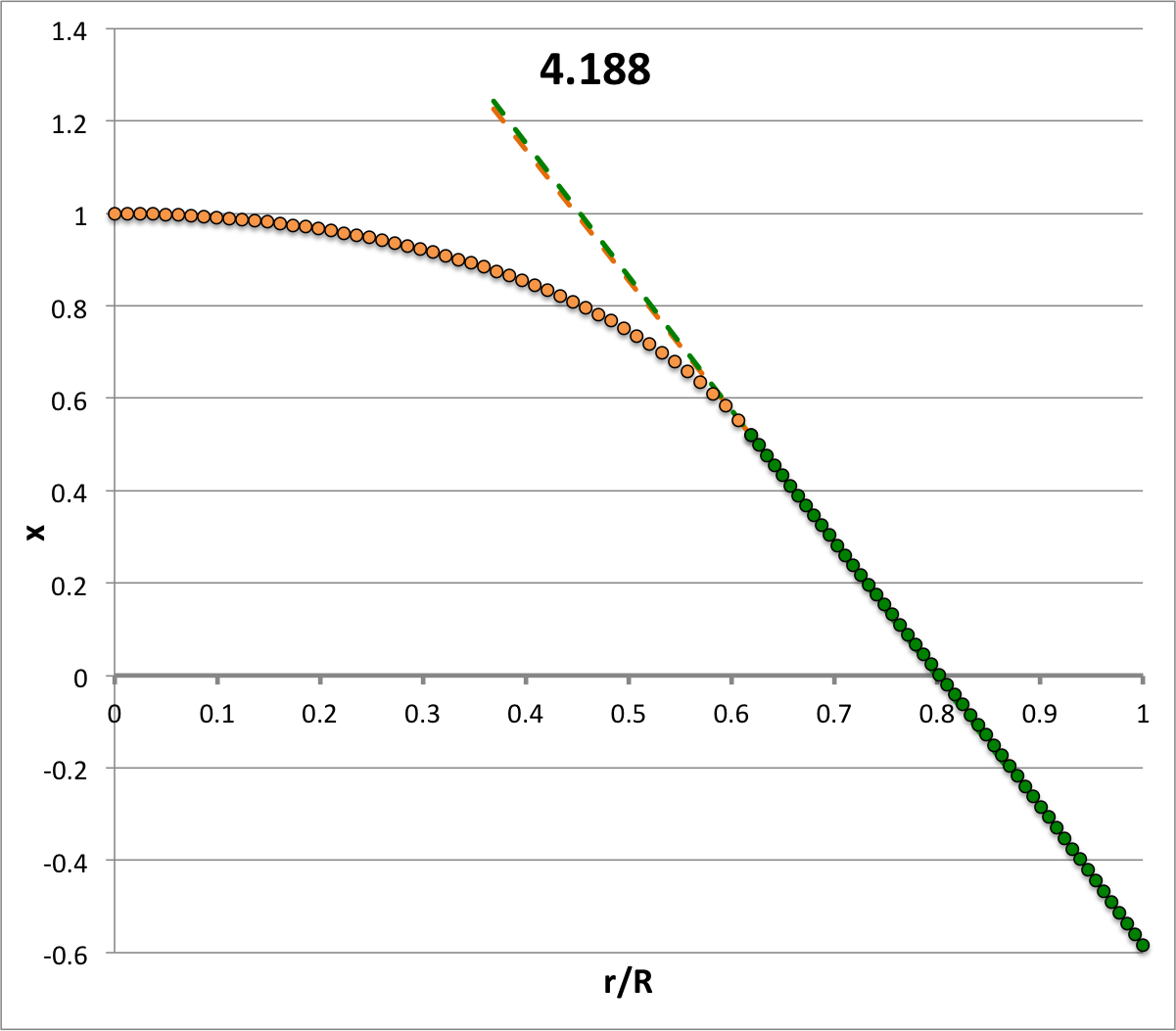
<td align="center"><math>~\ell = 1</math><p></p><math>~\sigma_c^2 = +4.188</math></td> | |||
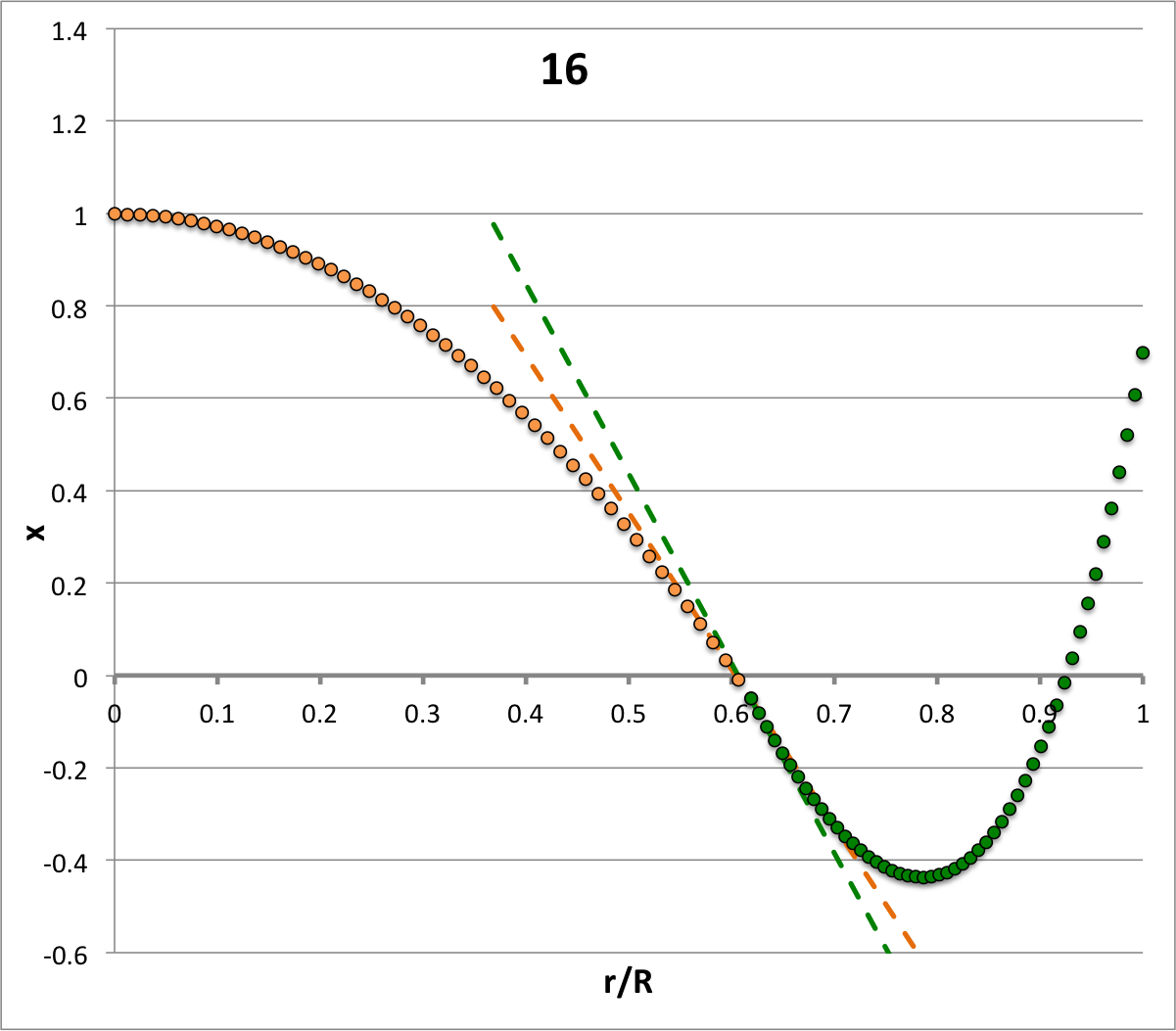
<td align="center"><math>~\ell = 2</math><p></p><math>~\sigma_c^2 = +16</math></td> | |||
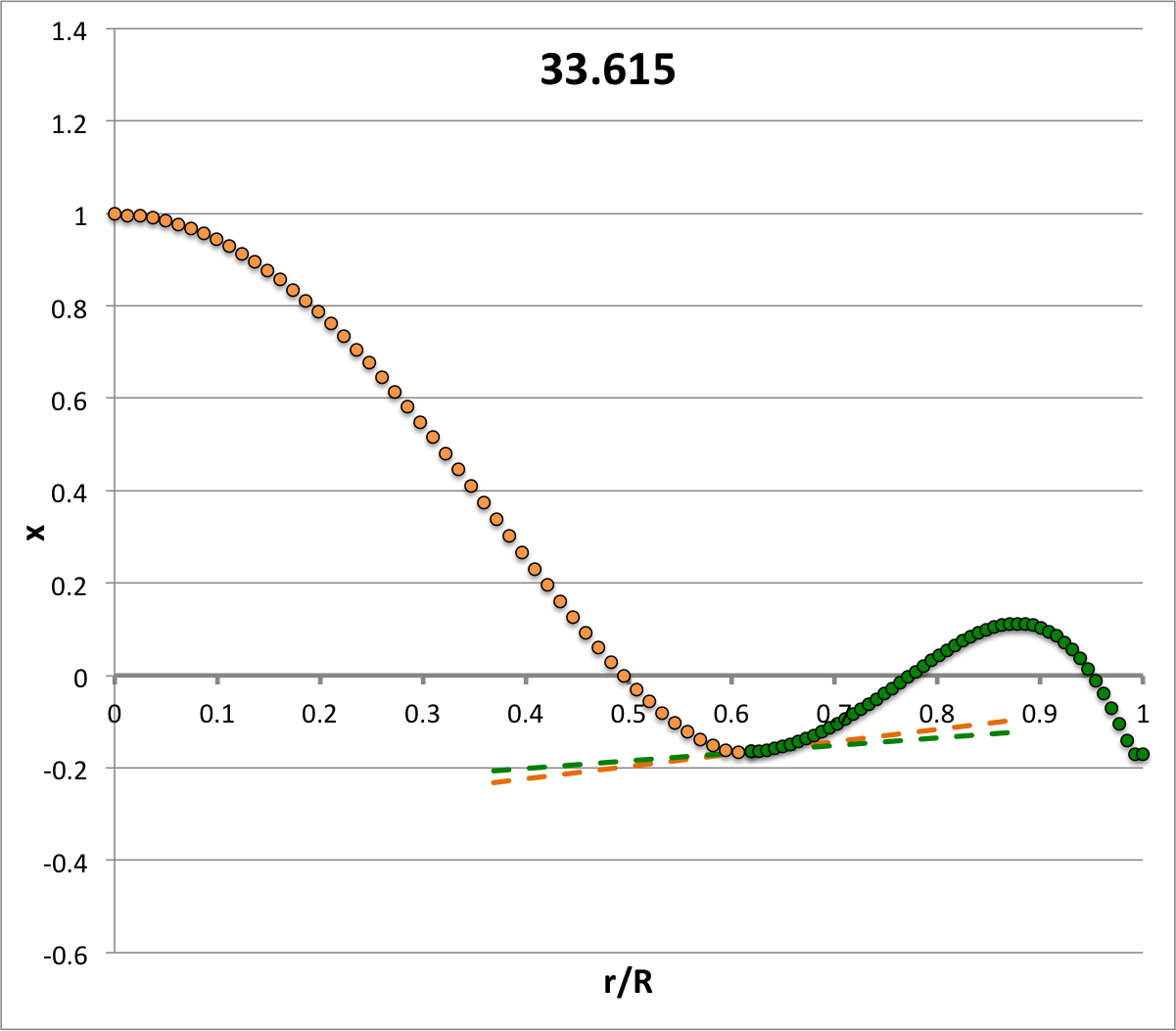
<td align="center"><math>~\ell = 3</math><p></p><math>~\sigma_c^2 = +33.615</math></td> | |||
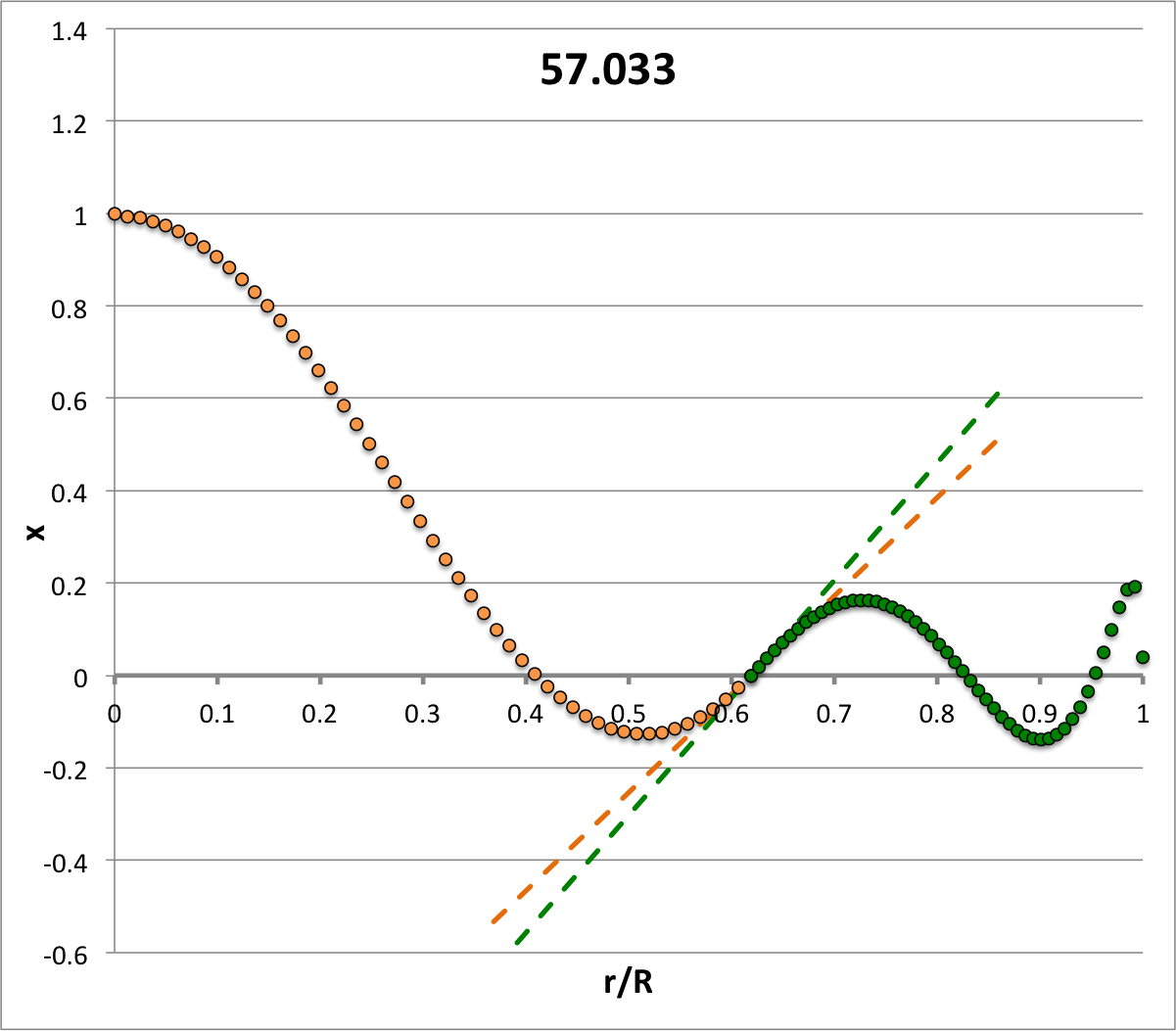
<td align="center"><math>~\ell = 4</math><p></p><math>~\sigma_c^2 = +57.033</math></td> | |||
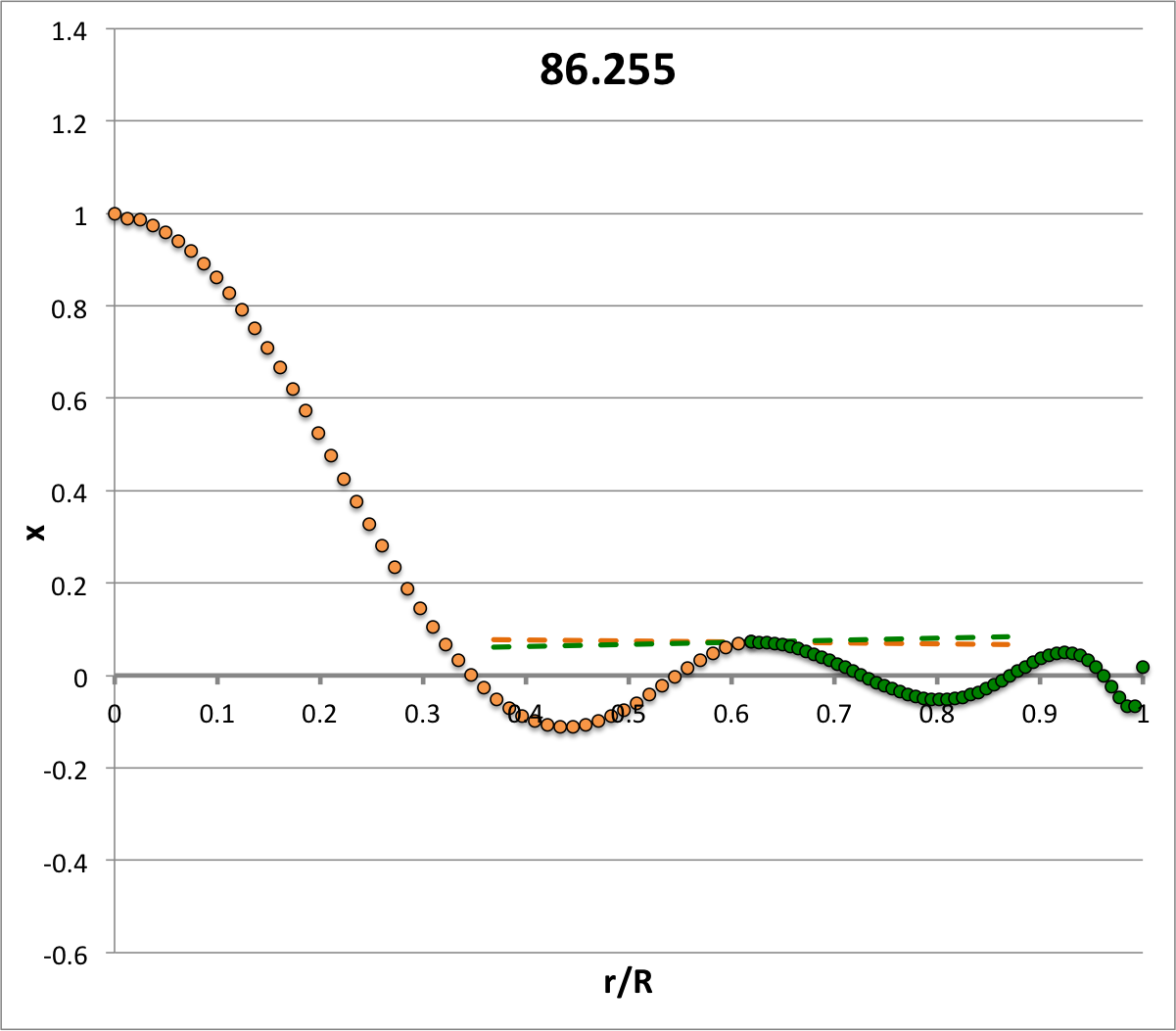
<td align="center"><math>~\ell = 5</math><p></p><math>~\sigma_c^2 = +86.255</math></td> | |||
</tr> | |||
<tr> | <tr> | ||
<td align=" | <td align="center">[[File:C01hypothetical.png|150 px|center]]</td> | ||
<td align="center">[[File:C11hypothetical.png|150 px|center]]</td> | |||
<td align="center">[[File:C21hypothetical.png|150 px|center]]</td> | |||
<td align="center"> | <td align="center">[[File:C31hypothetical.png|150 px|center]]</td> | ||
< | <td align="center">[[File:C41hypothetical.png|150 px|center]]</td> | ||
</td> | <td align="center">[[File:C51hypothetical.png|150 px|center]]</td> | ||
<td align=" | |||
</ | |||
</td> | |||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
Each of the six plots displayed in [[#Table4|Table 4]] (click on a panel in order to view a larger image) was [[User:Tohline/Appendix/Ramblings/NumericallyDeterminedEigenvectors#Numerically_Determined_Eigenvectors_of_a_Zero-Zero_Bipolytrope|generated numerically]] by integrating the LAWE for the core, outward from the center of the configuration to the core/envelope interface, then integrating the LAWE for the envelope, from the interface outward to the surface. At the interface: the ''value'' of the envelope eigenfunction is set to the ''value'' of the eigenfunction of the core; and the ''slope'' of the envelope's eigenfunction (highlighted graphically in each plot by the green, dashed line segment) was based on the slope of the core's eigenfunction (highlighted graphically by the orange, dashed line segment) but shifted in a discontinuous fashion [[#Step4|according to the above "Step 4" discussion]]. Each of the graphically illustrated Table 4 eigenfunctions has been scaled in such a way that the central value is unity; note that the panel labeled <math>~(\ell=2; \sigma_c^2 = +16)</math> displays an eigenfunction that is identical to the ''analytically defined'' eigenfunction displayed as Model C21 in [[#Table3|Table 3]], but it has been rescaled — and by necessity inverted — to provide a central value of unity. | |||
{{LSU_WorkInProgress}} | |||
< | ===Old, Incorrect Solutions=== | ||
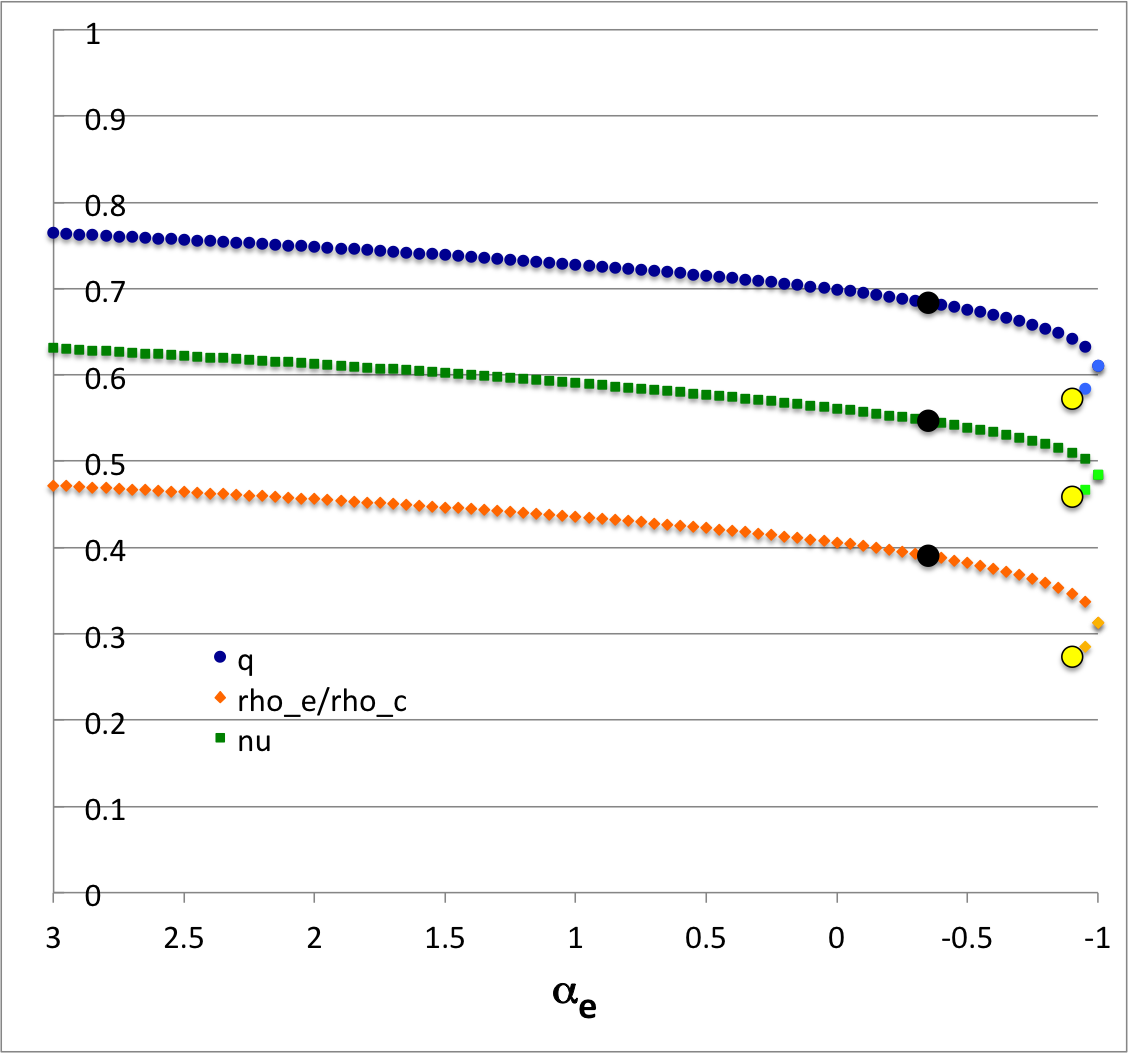
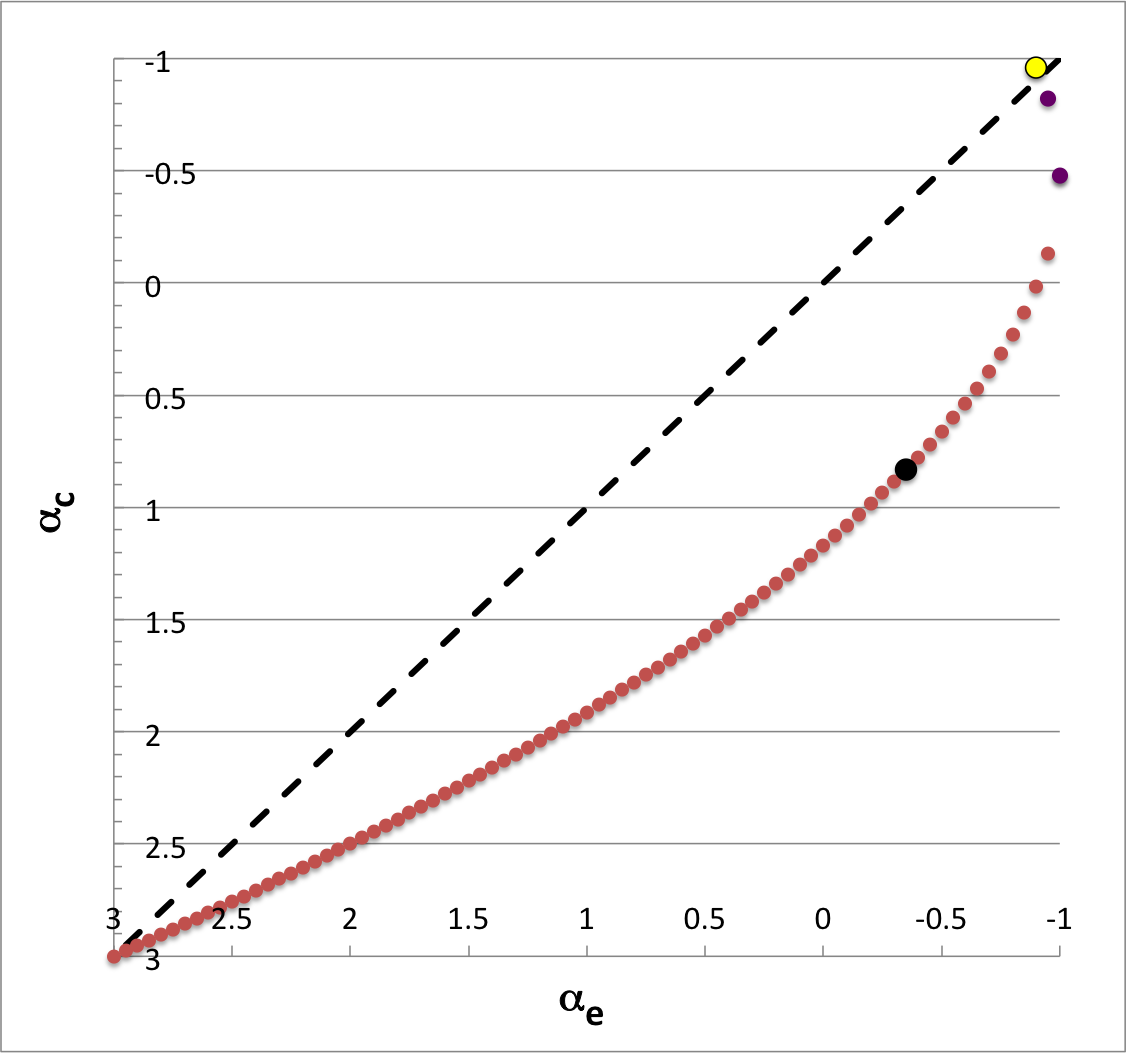
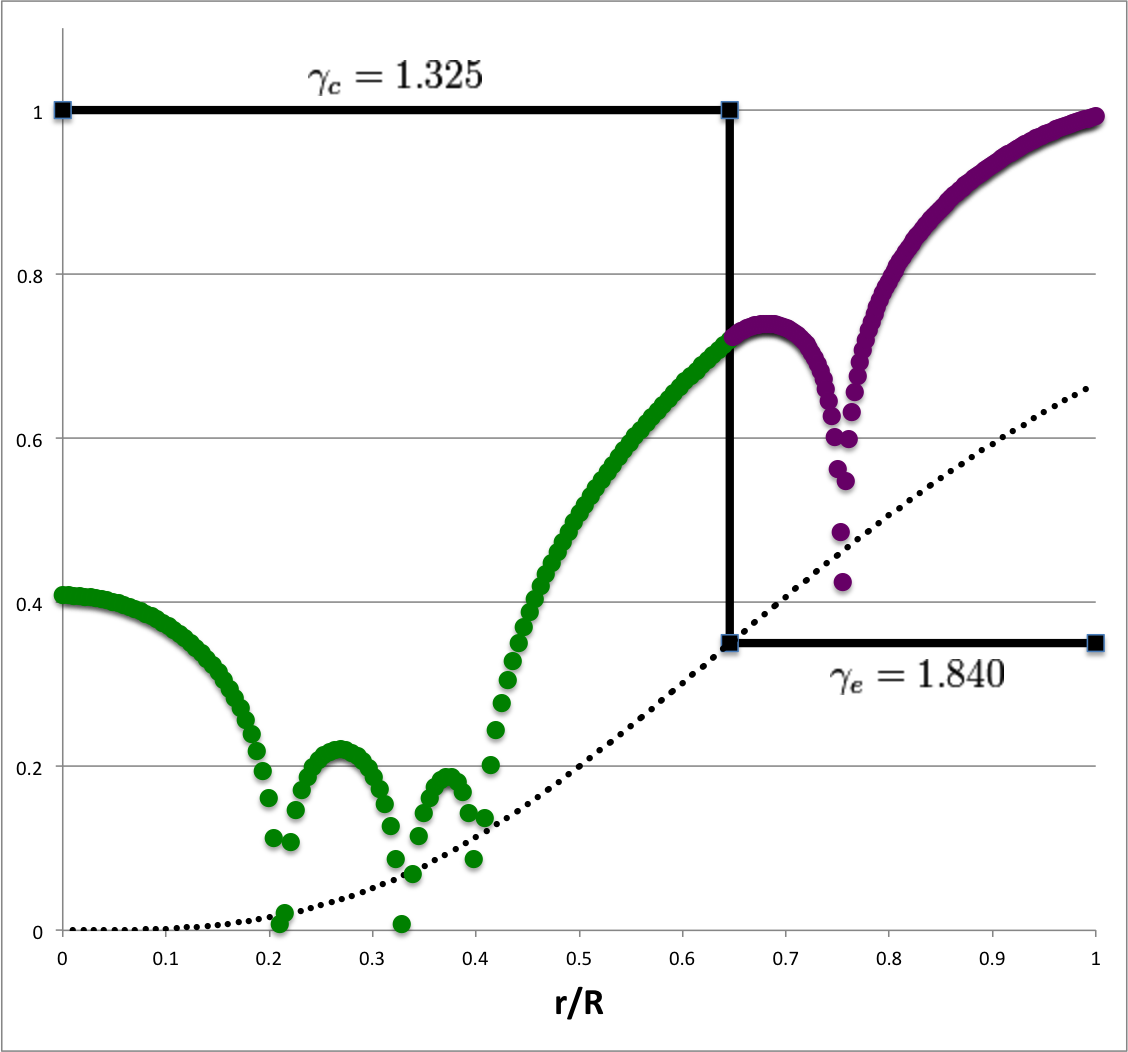
< | As is shown by the plot displayed in the right-hand panel of Figure 1, we have found different values of <math>~q</math> for each choice (STEP 1) of <math>~\gamma_e</math> (or, equivalently, choice of <math>~\alpha_e</math>). In this plot we have purposely flipped the horizontal axis so that the extreme left <math>~(\alpha_e = +3)</math> represents an incompressible <math>~(n = 0)</math> envelope, while the extreme right represents an isothermal <math>~(\gamma_e = 1)</math> envelope. | ||
<!-- | |||
The green and orange curves, respectively, show as well how the corresponding model parameters, <math>~\nu</math> and <math>~\rho_e/\rho_c</math>, vary with <math>~\alpha_e</math>. The right-hand panel displays one example of an <math>~(\ell, j) = (2,1)</math> eigenfunction that simultaneously satisfies the LAWE of the core and the LAWE of the envelope, and matches smoothly at the interface. This ''particular'' plotted solution corresponds to the case of <math>~\alpha_e = -0.35</math>, for which: <math>~\gamma_e =1.1940299</math>; <math>~n_e = 5.1538462</math>; <math>~q = 0.7943853</math>; <math>~\nu =0.6675302</math>; and <math>~\rho_e/\rho_c =0.5006468</math>. | |||
--> | |||
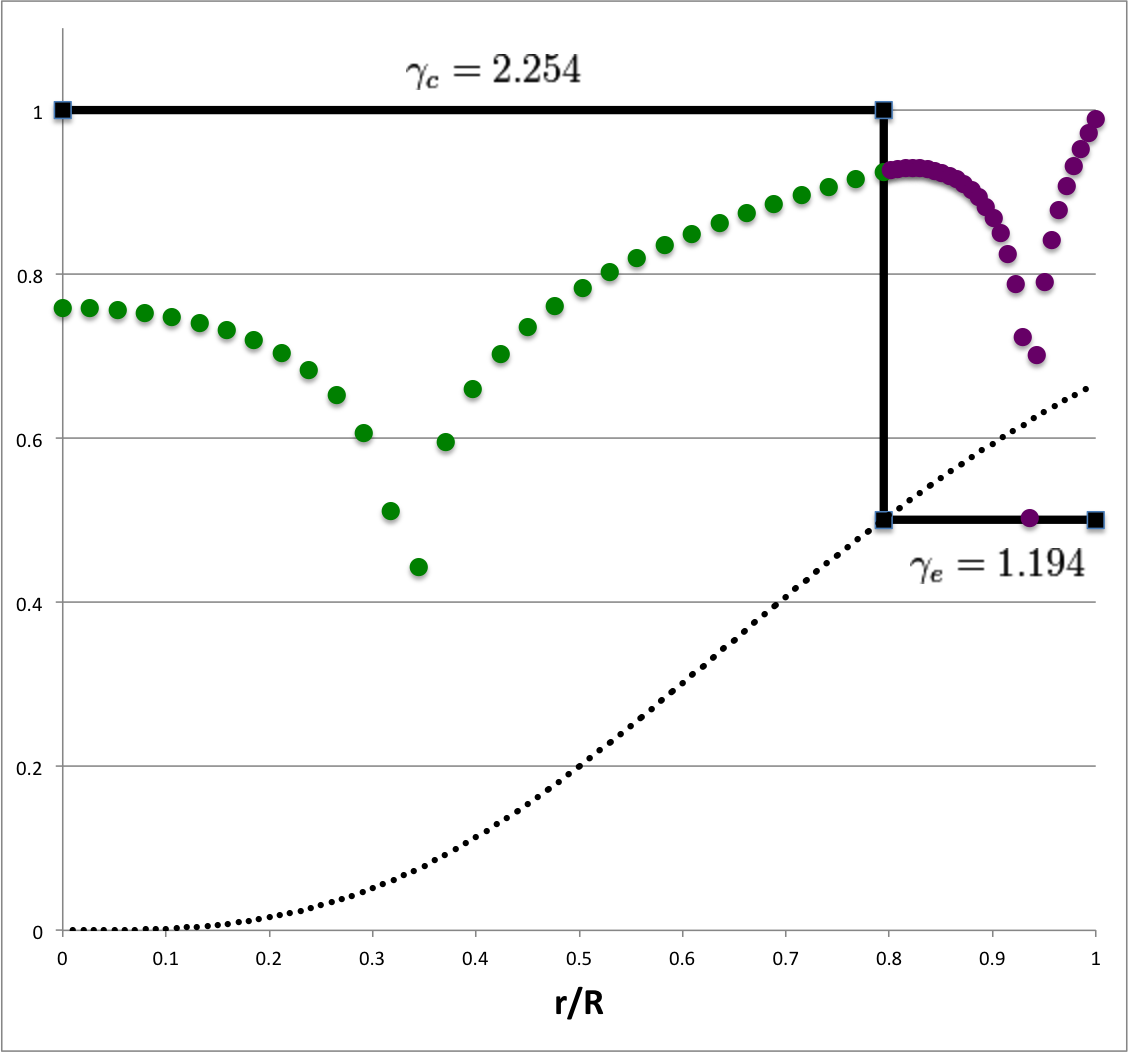
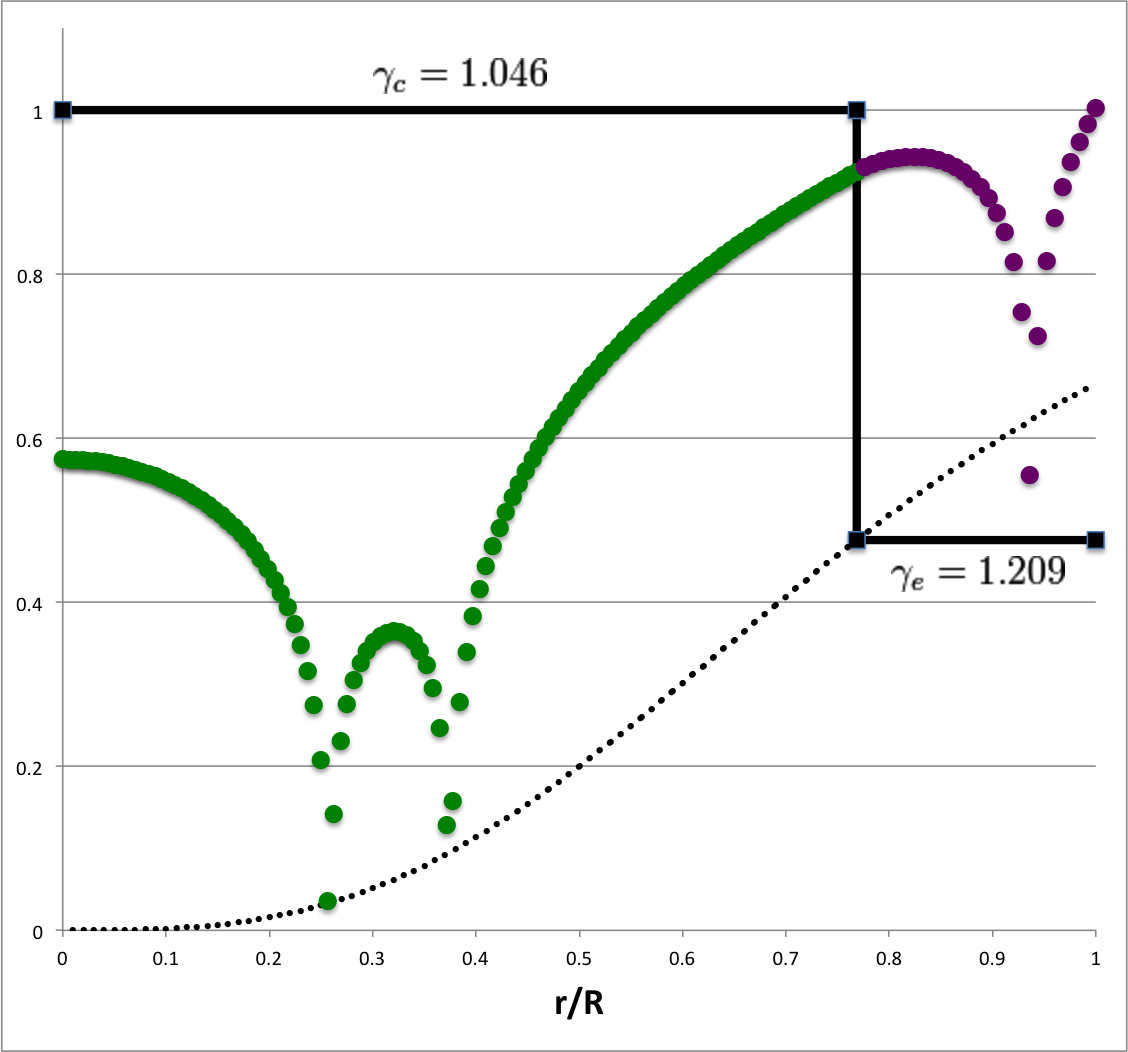
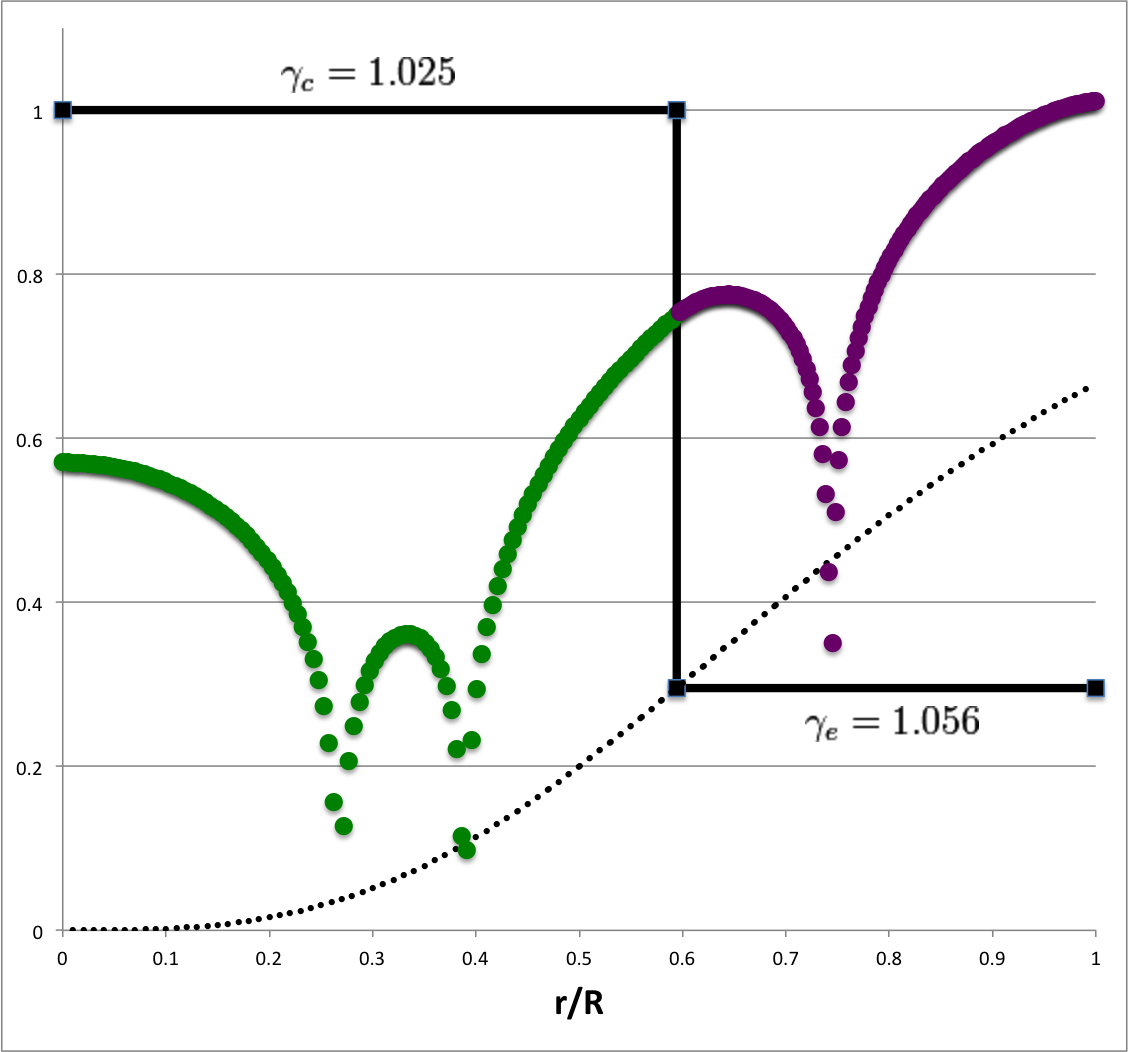
<div align="center" id="Figure1"> | |||
<table border="1" cellpadding="8"> | |||
<tr><th align="center" colspan="4">Figure 1</th></tr> | |||
<tr> | <tr> | ||
<td align=" | <td align="center" colspan="3"><math>~\alpha_e = -0.35 \, ;~~~c_0 = \sqrt{1+\alpha_e} - 1</math></td> | ||
<math>~0</math> | <td align="center" rowspan="18"> | ||
<td align="center"> | <table border="0" width="100%"> | ||
< | <tr><td align="center"> | ||
[[File:Quartic21Solution02Corrected.png|500px|quartic solution]] | |||
</td></tr> | |||
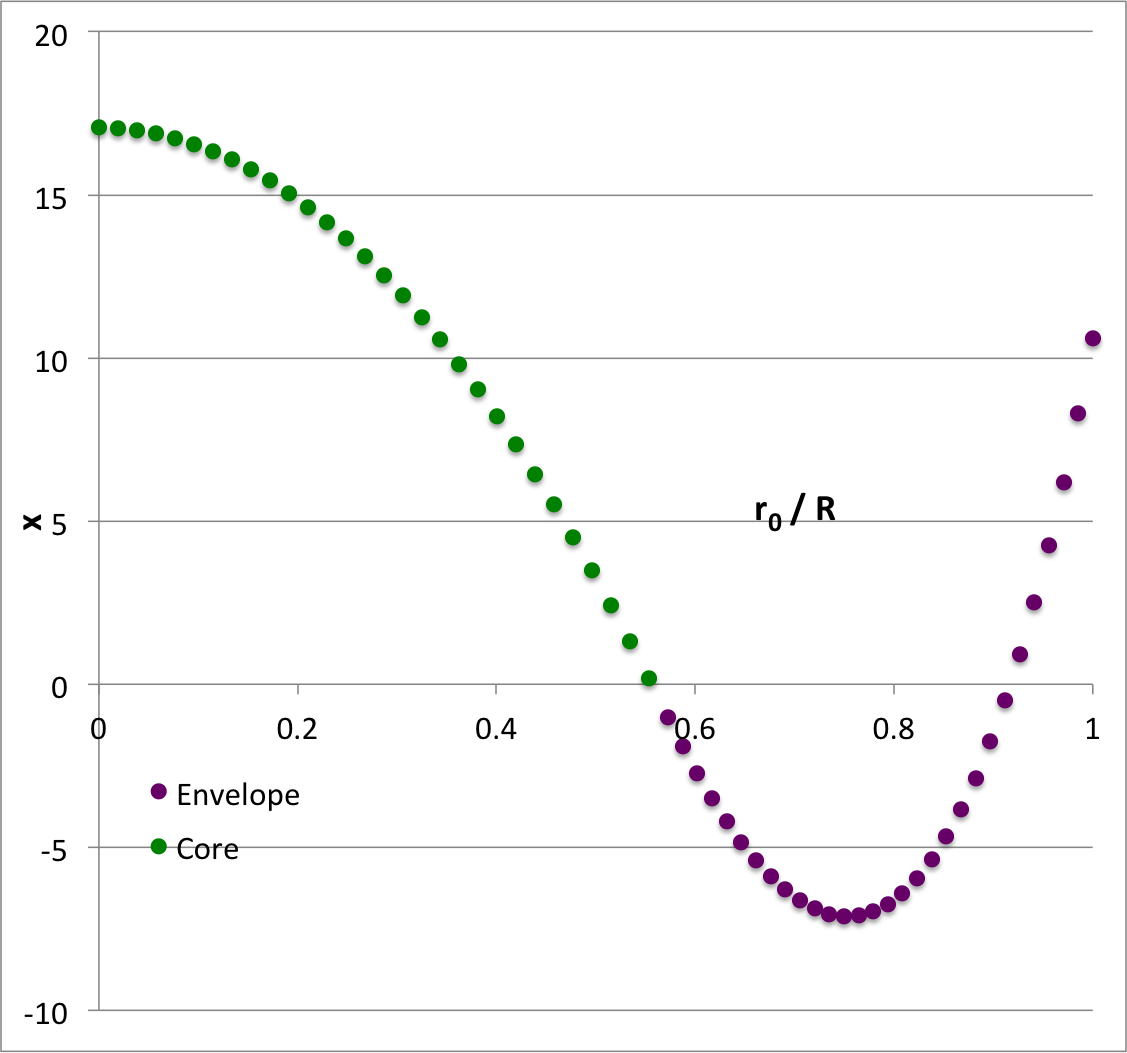
<math>~ | <tr><td align="left">''Directly Above:'' Plot shows for ''which'' equilibrium bipolytropic configurations with <math>~(n_c, n_e) = (0,0)</math> we are able to construct analytically prescribed eigenvectors for the radial oscillation mode, <math>~(\ell, j) = (2,1)</math>. The top (blue), middle (green), and bottom (orange) curves show how <math>~q</math>, <math>~\nu</math>, and <math>~\rho_e/\rho_c</math> vary with the specified value of the envelope's adiabatic exponent over the full, physically reasonable range of the parameter, <math>~-1 \le \alpha_e \le 3</math>. For the upper portion of each curve (dark blue, dark green, dark orange), the parameter, <math>~c_0</math>, is taken to be the "plus" root of its defining quadratic equation; the "minus" root defines <math>~c_0</math> along the lower portion of each curve (light blue, light green, light orange). | ||
\ | |||
''Upper-left Quadrant:'' An <math>~x(r_0/R)</math> plot showing the radial structure of the analytically prescribed eigenfunction for <math>~\alpha_e = -0.35</math> and <math>~c_0</math> (plus); its underlying, equilibrium model characteristics are identified by the black circular marker in the above plot. | |||
</math> | |||
</ | |||
</ | |||
</ | |||
''Lower-left Quadrant:'' The analytcially prescribed eigenfunction, <math>~x(r_0/R)</math>, for <math>~\alpha_e = -0.9</math> and <math>~c_0</math> (minus); its underlying, equilibrium model characteristics are identified by the yellow circular marker in the above plot. | |||
< | <sup>†</sup>Note that, as displayed here, the sign has been flipped on both <math>~x(r_0/R)</math> eigenfunctions so that, in practice, the amplitude at the interface is ''negative'' one, rather than positive one. Plotted in this way, we immediately recognize that both eigenfunctions are ''qualitatively'' similar to the <math>~j = 2</math> radial oscillation eigenfunction that [[User:Tohline/SSC/UniformDensity#Properties_of_Eigenfunction_Solutions|was derived by Sterne (1937) in the context of isolated, homogeneous spheres]]. | ||
<table | </td></tr> | ||
</table> | |||
</td> | |||
</tr> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"><math>~c_0</math> (plus):</td> | ||
<math>~ | <td align="center"><math>~-0.1937742</math></td> | ||
<td align="center" rowspan="8"> | |||
<td align="center"> | [[File:EigenfunctionP1Corrected.png|270px|quartic solution]] | ||
<math>~ | |||
<td align=" | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"><math>~\gamma_e</math>:</td> | ||
<math>~\ | <td align="center"><math>~1.1940299</math></td> | ||
</tr> | |||
<td align="center"> | <tr> | ||
<math>~ | <td align="right"><math>~n_e</math>:</td> | ||
<td align="center"><math>~5.1538462</math></td> | |||
<td align=" | |||
<math>~ | |||
</math> | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"><math>~q</math>:</td> | ||
<td align="center"><math>~0.6840119</math></td> | |||
<math>~ | |||
<td align=" | |||
<math>~ | |||
</math> | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"><math>~\nu</math>:</td> | ||
<math>~ \ | <td align="center"><math>~0.5466868</math></td> | ||
</td> | </tr> | ||
<td align="center"> | <tr> | ||
<math>~ | <td align="right"><math>~\rho_e/\rho_c</math>:</td> | ||
</td> | <td align="center"><math>~0.3902664</math></td> | ||
<td align=" | </tr> | ||
<math>~\ | <tr> | ||
<td align="right"><math>~\alpha_c</math>:</td> | |||
<td align="center"><math>~+0.8326585</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~\gamma_c</math>:</td> | |||
<td align="center"><math>~+1.845579</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center" colspan="3"><math>~\alpha_e = -0.9 \, ;~~~c_0 = -\sqrt{1+\alpha_e} - 1</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~c_0</math> (minus):</td> | |||
<td align="center"><math>~- 1.3162278</math></td> | |||
<td align="center" rowspan="8"> | |||
[[File:EigenfunctionM1Corrected.png|270px|quartic solution]] | |||
</td> | </td> | ||
</tr> | |||
<tr> | |||
<td align="right"><math>~\gamma_e</math>:</td> | |||
<td align="center"><math>~1.0256410</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~n_e</math>:</td> | |||
<td align="center"><math>~39</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~q</math>:</td> | |||
<td align="center"><math>~0.5728050</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~\nu</math>:</td> | |||
<td align="center"><math>~0.4586270</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~\rho_e/\rho_c</math>:</td> | |||
<td align="center"><math>~0.2731929</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~\alpha_c</math>:</td> | |||
<td align="center"><math>~-0.9595214</math></td> | |||
</tr> | |||
<tr> | |||
<td align="right"><math>~\gamma_c</math>:</td> | |||
<td align="center"><math>~+1.0102231</math></td> | |||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
<span id="STEP5"><font color="red"><b>STEP 5:</b></font></span> Finally, for each choice of <math>~\gamma_e</math> — or, alternatively, <math>~\alpha_e</math> — the physically relevant value of the core's adiabatic exponent is set by demanding that the ''dimensional'' eigenfrequencies of the envelope and core precisely match. That is, we demand that, | |||
<table border="1" cellpadding="8" align="right"> | |||
<tr><th align="center" colspan="1">Figure 2</th></tr> | |||
<tr> | <tr> | ||
<td align="center"> | <td align="center"> | ||
[[File:AlphaVsAlpha21BothCorrected.png|350px|quartic solution]] | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
<div align="center"> | |||
<math>~\omega^2_\mathrm{env} = \omega^2_\mathrm{core} \, .</math> | |||
</div> | |||
From [[#Eigenvector|above]], we know that, for the core, | |||
<div align="center"> | |||
<math>~3\omega^2_\mathrm{core}\biggr|_\mathrm{j=1} = 2\pi \gamma_c G \rho_c [ 20 - 8/\gamma_c] \, ;</math> | |||
</div> | |||
whereas, for the envelope, | |||
<div align="center"> | |||
<math>~3\omega^2_\mathrm{env}\biggr|_\mathrm{\ell=2} = 2\pi \gamma_e G \rho_e [ 3(\alpha_e + 5c_0 + 22)] \, .</math> | |||
</div> | </div> | ||
By demanding that these frequencies be identical, we conclude that, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 924: | Line 1,119: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~ \gamma_c </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 930: | Line 1,125: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{1}{20} \biggl[ 8 + 3\gamma_e \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(\alpha_e + 5c_0 + 22 \biggr)\biggr] \, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<!-- | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ \ | <math>~ \Rightarrow ~~~ \gamma_c </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 942: | Line 1,137: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~3 \, | <math>~\frac{1}{20}\biggl\{ 8 + \biggl(\frac{12}{3-\alpha_e}\biggr) \biggl[\alpha_e + 5(\sqrt{1+\alpha_e}-1) + 22 \biggr]\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr\}\, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
--> | |||
</table> | </table> | ||
</div> | </div> | ||
this boundary condition | Figure 2 shows how the required value of <math>~\alpha_c</math> varies with the choice of <math>~\alpha_e</math>; here, both axes have been flipped in order to run from incompressible <math>~(\alpha = +3)</math> at the left/bottom, to isothermal <math>~(\alpha = -1)</math> at the right/top. For the lower portion of the curve (red circular markers), the parameter, <math>~c_0</math>, is taken to be the "plus" root of its defining quadratic equation; the "minus" root defines <math>~c_0</math> along the upper portion of the curve (purple circular markers). The diagonal dashed-black line identifies where <math>~\alpha_c = \alpha_e</math>; in models below and to the right of this line, the envelope is more compressible than is the core, whereas in models above and to the left of this line, the core is more compressible than the envelope. | ||
<!-- OMIT Evidently there is one model for which the <math>~(\ell,j) = (2,1)</math> eigenvector is analytically specifiable in which the envelope and core are equally compressible; it is the model with <math>~\gamma_c = \gamma_e \approx 1.13</math> that is identified by where the <math>~c_0</math> (minus) segment of the curve intersects the diagonal black-dashed line. | |||
--> | |||
The eigenfrequency that corresponds to the ''specific'' eigenfunction that is displayed in upper-left quadrant of Figure 1 is identified by the black circular marker in Figure 2; as is indicated by the row of numbers on the left in Figure 1, this model has, | |||
<div align="center"> | |||
<math>~\gamma_c = 1.845579 </math> | |||
<math>~\Rightarrow </math> | |||
<math>~\alpha_c = +0.8326535 \, . </math> | |||
</div> | |||
The yellow circular marker in Figure 2 identifies the model whose analytically prescribed, <math>~(\ell,j) = (2,1)</math> eigenfunction is displayed in the lower-left quadrant of Figure 1; it has, | |||
<div align="center"> | |||
<math>~\gamma_c = 1.0102231 </math> | |||
<math>~\Rightarrow </math> | |||
<math>~\alpha_c = -0.9595214 \, . </math> | |||
</div> | |||
==Examining Alignment with Surface Boundary Condition== | |||
===Expectation=== | |||
As we have reviewed in [[User:Tohline/SSC/Stability/BiPolytrope0_0Details#Boundary_Condition|an accompanying discussion]], one astrophysically reasonable surface boundary condition provides a mathematical relationship between the logarithmic derivative of the eigenfunction with respect to the radius, in terms of the eigenfrequency as follows: | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 954: | Line 1,173: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{d\ln x}{d\ln\xi}</math> | <math>~ \frac{d\ln x}{d\ln \xi}\biggr|_{\xi = 1/q}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 960: | Line 1,179: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{3\omega^2 }{4\pi G\rho_c \gamma_e} \biggl( \frac{\nu}{q^3}\biggr) - \biggl( 3 - \frac{4}{\gamma_e}\biggr) </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~3 | <math>~\frac{3\omega^2 }{4\pi G\rho_c \gamma_e} \biggl( \frac{1+2q^3}{3q^3}\biggr) - \alpha_e </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 989: | Line 1,197: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{3\omega^2 }{2\pi \ | <math>~\frac{1}{3} \biggl[\frac{3\omega^2 }{2\pi G\rho_c \gamma_e} \biggr] \biggl( \frac{\rho_c}{\rho_e}\biggr) - \alpha_e </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,013: | Line 1,215: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{1}{3} \biggl[\mathfrak{F}_\mathrm{env} + 2\alpha_e \biggr] - \alpha_e </math> | ||
\frac{1}{ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,035: | Line 1,227: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{1}{3} \biggl[\mathfrak{F}_\mathrm{env} - \alpha_e \biggr] \, . </math> | ||
\frac{ | |||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
Now, according to our [[#Envelope_Segment|above-described envelope segment of the eigenfunction]], we established the analytic prescription, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\mathfrak{F}_\mathrm{env}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~(c_0 + 3\ell)(c_0 + 3\ell+5) \, ,</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
in which case the desired surface boundary condition is, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 1,069: | Line 1,256: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~ 3 \cdot \frac{d\ln x}{d\ln \xi}\biggr|_{\xi = 1/q}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,075: | Line 1,262: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ (c_0 + 3\ell)(c_0 + 3\ell+5) - \alpha_e </math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,090: | Line 1,274: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ [c_0^2 + c_0(6\ell + 5 ) + 3\ell(3\ell+5)] - (c_0^2 + 2c_0)</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,105: | Line 1,286: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ 3[ c_0(2\ell + 1 ) + \ell(3\ell+5)]</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | |||
</table> | |||
</div> | |||
That is, we expect to find the following, | |||
<div align="center" id="DesiredBoundaryCondition"> | |||
<table border="1" align="center"><tr><td align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<th align="center" colspan="3"> | |||
Desired Boundary Condition | |||
</th> | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{ | <math>~\frac{d\ln x}{d\ln \xi}\biggr|_{\xi = 1/q}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,119: | Line 1,311: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ c_0(2\ell + 1 ) + \ell(3\ell+5) \, .</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</td></tr></table> | |||
</div> | |||
===Analytic2=== | |||
Continuing, from above, a discussion specifically of the case, <math>~\ell = 2</math>, the analytically specified envelope eigenfunction is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~x_{\ell=2} |_\mathrm{env}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,135: | Line 1,333: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ | ||
\xi^{c_0}\biggl[ \frac{ 1 + q^3 A_{\ell=2} \xi^{3} + q^6 A_{\ell=2}B_{\ell=2}\xi^{6} }{ 1 + q^3 A_{\ell=2} + q^6 A_{\ell=2}B_{\ell=2}}\biggr] \, , | |||
\ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
where, the values of the newly introduced coefficients, | |||
<div align="center"> | |||
<table border="0" cellpadding="3" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~A_{\ell=2}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~\biggl[ \frac{c_0(c_0+5) - (c_0 + 6)(c_0 + 11)}{(c_0 + 3)(c_0+5) - \alpha_e}\biggr] = \frac{-2(2c_0+11)}{(2c_0+5)} \, ,</math> | ||
</td> | |||
</math> | </tr> | ||
<tr> | |||
<td align="right"> | |||
<math>~B_{\ell=2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{(c_0+3)(c_0+8) - (c_0 + 6)(c_0 + 11)}{(c_0 + 6)(c_0+8) - \alpha_e}\biggr] = \frac{-(c_0+7)}{2(c_0+4)} \, ,</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
in which case, | |||
<div align="center"> | <div align="center"> | ||
| Line 1,164: | Line 1,375: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{d\ln x}{d\ln \xi} = \frac{\xi}{x} \cdot \frac{dx}{d\xi} \biggl|_\mathrm{env}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,170: | Line 1,381: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{\xi}{x} \biggl\{ | ||
\frac{ | c_0\xi^{c_0-1}\biggl[ \frac{ 1 + q^3 A \xi^{3} + q^6 AB\xi^{6} }{ 1 + q^3 A + q^6 AB}\biggr] | ||
\ | + \xi^{c_0}\biggl[ \frac{ 3q^3 A \xi^{2} + 6q^6 AB\xi^{5} }{ 1 + q^3 A + q^6 AB}\biggr] | ||
\biggr\} | |||
\ | |||
\biggl[ | |||
\biggr\} | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,191: | Line 1,398: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl | c_0 | ||
+ \biggl[ \frac{ 3q^3 A \xi^{3} + 6q^6 AB\xi^{6} }{ 1 + q^3 A\xi^3 + q^6 AB\xi^6}\biggr] \, . | |||
+ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
Hence, at the surface <math>~(\xi = 1/q)</math>, we find, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{d\ln x}{d\ln \xi} \biggl|_{\xi=1/q}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,210: | Line 1,418: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl[ | c_0 | ||
+\biggl[ \frac{ 3 A + 6 AB }{ 1 + A + AB}\biggr] | |||
+ | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,228: | Line 1,433: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl[ | c_0 | ||
+\biggl[ \frac{ -12(2c_0+11)(c_0+4) + 12(2c_0+11)(c_0+7) }{ 2(2c_0 + 5)(c_0+4) - 4(2c_0+11)(c_0+4) + 2(2c_0+11) (c_0+7)}\biggr] | |||
- | |||
+ | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,246: | Line 1,448: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
c_0 | |||
+6\biggl[ \frac{ (2c_0^2 + 25c_0 + 77) -(2c_0^2 + 19c_0 +44) }{ (2c_0^2 + 13c_0 + 20) - 2(2c_0^2 + 19c_0 + 44) + (2c_0^2 + 25c_0 + 77)}\biggr] | |||
- 2( | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,264: | Line 1,463: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
c_0 | |||
+6 \biggl[ \frac{ 6c_0 +33 }{ 9}\biggr] | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,283: | Line 1,478: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
5c_0 + 22 \, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,296: | Line 1,486: | ||
</div> | </div> | ||
=== | It is gratifying — although, somewhat surprising (to me!) — to find that this precisely matches the [[#DesiredBoundaryCondition|above-defined, desired boundary condition]] for the case of <math>~\ell = 2</math>. | ||
===Duh!=== | |||
[[File:CommentButton02.png|right|100px|Comment by J. E. Tohline on 4 February 2017: This numerical determination of surface boundary conditions was carried out inside spreadsheet "FDflex22" of Excel file ''analyticeigenvectorcorrected.xlsx''.]]After also checking conformance with the expected boundary condition in [[User:Tohline/Appendix/Ramblings/Additional_Analytically_Specified_Eigenvectors_for_Zero-Zero_Bipolytropes#Check_Surface_Boundary_Condition|the case of analytic eigenfunctions having <math>~\ell = 3</math>]] and, separately (not shown), for numerically generated eigenfunctions having a wide range of oscillation frequencies, it dawned on us that the [[#DesiredBoundaryCondition|"desired" surface boundary condition]] may actually be a natural outcome of the envelope's LAWE. | |||
By constraining our discussion to models for which <math>~g^2 = \mathcal{B}</math> and <math>~\mathcal{D} = q^3</math>, the [[#The_Envelope.27s_LAWE|envelope's LAWE]] is, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 1,304: | Line 1,499: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,310: | Line 1,505: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{d\ | <math>~ | ||
\biggl[ 1 - q^3 \xi^3\biggr] \frac{d^2x}{d\xi^2} | |||
+ \biggl\{ 3 - 6q^3 \xi^3 \biggr\} | |||
\frac{1}{\xi} \cdot \frac{dx}{d\xi} | |||
+ \biggl[ | |||
q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e | |||
\biggr]\frac{x}{\xi^2} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
At the surface <math>~(\xi = 1/q)</math>, the coefficient of the second derivative term goes to zero, in which case the LAWE reduces in form to, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 1,321: | Line 1,524: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | |||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~=</math> | ||
| Line 1,328: | Line 1,531: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{\xi | -\frac{3}{\xi} \cdot \frac{dx}{d\xi} | ||
+ | + \biggl[ | ||
\mathfrak{F}_\mathrm{env} -\alpha_e | |||
\biggr]\frac{x}{\xi^2} | |||
</math> | </math> | ||
</td> | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ 3\cdot \frac{d\ln x}{d\ln \xi}\biggr|_\mathrm{surface} </math> | |||
</td> | |||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~=</math> | ||
| Line 1,343: | Line 1,548: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\ | \mathfrak{F}_\mathrm{env} -\alpha_e \, . | ||
</math> | </math> | ||
</td> | |||
</tr> | </tr> | ||
</table> | |||
</div> | |||
And this is ''precisely'' the condition that derives from the astrophysically reasonable boundary condition that we have [[User:Tohline/SSC/Stability/BiPolytrope0_0Details#Boundary_Condition|discussed separately]] and that has been [[#Expectation|reviewed, above]]. | |||
===Broader Analysis=== | |||
Let's, then, examine the behavior of the envelope's LAWE at the surface in the most general case — that is, when ''not'' constrained to <math>~g^2 = \mathcal{B}</math>. First, we note that, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~g^2 - \mathcal{B}</math> | ||
</math> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
\frac{ | \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \biggl( 1-q \biggr) + | ||
\frac{\rho_e}{\rho_c} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] -2\biggl(\frac{\rho_e}{\rho_c}\biggr) + 3\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 | |||
</math> | </math> | ||
</td> | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl\{ 2 \biggl[1 - \biggl(\frac{\rho_e}{\rho_c} \biggr) -q + q \biggl(\frac{\rho_e}{\rho_c} \biggr)\biggr] + | |||
\biggl( \frac{\rho_e}{\rho_c} \biggr)\frac{1}{q^2} -2 + 2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr\} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,404: | Line 1,601: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
\biggl | \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ - 2q + 2q \biggl(\frac{\rho_e}{\rho_c} \biggr) + | ||
\biggl( \frac{\rho_e}{\rho_c} \biggr)\frac{1}{q^2} \biggr] | |||
+\biggl | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,422: | Line 1,616: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
\biggl | \frac{1}{q^2} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] \, . | ||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
Hence, at the surface quite generally, the coefficient of the second derivative is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{1}{\mathcal{A}}\biggl[\mathcal{A} + (g^2 - \mathcal{B})\xi - \mathcal{A}\mathcal{D} \xi^3 \biggr]_{\xi=1/q}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,438: | Line 1,636: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ \biggl | <math>~ | ||
+ \biggl | \frac{1}{\mathcal{A}}\biggl\{ | ||
+ \biggl | 2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(1-\frac{\rho_e}{\rho_c}\biggr) | ||
+ \frac{1}{q^3} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] | |||
- \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 | |||
\biggr\} | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,453: | Line 1,654: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{1}{\mathcal{A}} \biggl(\frac{\rho_e}{\rho_c}\biggr)\biggl\{ | |||
+ \biggl[ | - 2\biggl(\frac{\rho_e}{\rho_c} -1\biggr) | ||
+ \frac{1}{q^3} \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] | |||
- \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) | |||
\biggr\} | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
0 \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
And, at the surface quite generally, the coefficient of the first derivative is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\frac{1}{\mathcal{A}}\biggl[3\mathcal{A} + 4(g^2 - \mathcal{B})\xi - 6\mathcal{A}\mathcal{D} \xi^3 \biggr]_{\xi=1/q}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~q | <math>~ | ||
\frac{1}{\mathcal{A}}\biggl\{ | |||
6\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(1-\frac{\rho_e}{\rho_c}\biggr) | |||
+ \frac{4}{q^3} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] | |||
- \frac{6}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 | |||
\biggr\} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,497: | Line 1,704: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{2}{3}\ | <math>~ | ||
\frac{2}{\mathcal{A}} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl\{ | |||
-3\biggl(\frac{\rho_e}{\rho_c} - 1\biggr) | |||
+ \frac{2}{q^3} \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] | |||
- \frac{3}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) | |||
\biggr\} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,527: | Line 1,729: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \frac{2}{\mathcal{A}} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ | ||
\biggl(\frac{\rho_e}{\rho_c} - 1\biggr) | |||
- \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) | |||
\biggr] \, . | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
Hence, at the surface quite generally, the envelope's LAWE becomes, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | |||
- \biggl[ 3\mathcal{A} + 4(g^2-\mathcal{B}) \xi - 6\mathcal{A} \mathcal{D} \xi^3 \biggr]_{\xi=1/q} \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\mathcal{A}\biggl[ | |||
\mathfrak{F} | \mathcal{D} \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]_{\xi=1/q} | ||
\ | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,553: | Line 1,762: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~\frac{\ | <math>~\Rightarrow~~~ | ||
- 2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ | |||
\biggl(\frac{\rho_e}{\rho_c} - 1\biggr) | |||
- \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) | |||
\biggr] \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,560: | Line 1,774: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \biggl(\frac{\rho_e}{\rho_c}\biggr)^2 \mathfrak{F}_\mathrm{env} \cdot \frac{1}{q^3} - 2\alpha_e \biggl(\frac{\rho_e}{\rho_c}\biggr)\biggl(1- \frac{\rho_e}{\rho_c}\biggr) | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,569: | Line 1,781: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow~~~ | |||
\biggl[2\biggl(\frac{\rho_e}{\rho_c}\biggr) + | |||
2q^3\biggl(1 - \frac{\rho_e}{\rho_c} \biggr) | |||
\biggr] \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl(\frac{\rho_e}{\rho_c}\biggr) \mathfrak{F}_\mathrm{env} - 2q^3 \alpha_e \biggl(1- \frac{\rho_e}{\rho_c}\biggr) | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{ | <math>~\Rightarrow~~~ | ||
\frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,596: | Line 1,807: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~\biggl[2 + 2q^3\biggl(1 - \frac{\rho_e}{\rho_c} \biggr)\biggl(\frac{\rho_e}{\rho_c}\biggr)^{-1} \biggr] ^{-1} | ||
\biggl[ \mathfrak{F}_\mathrm{env} - 2q^3 \alpha_e \biggl(1- \frac{\rho_e}{\rho_c}\biggr)\biggl(\frac{\rho_e}{\rho_c}\biggr)^{-1} \biggr] | |||
\biggl(\frac{\rho_e}{\rho_c}\biggr) | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,613: | Line 1,821: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~ | ||
\frac{\mathfrak{F}_\mathrm{env} - \Kappa \alpha_e}{2+\Kappa} \, , | |||
+ \ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
where, | |||
<div align="center"> | |||
<math>~\Kappa \equiv 2q^3\biggl(1 - \frac{\rho_e}{\rho_c} \biggr)\biggl(\frac{\rho_e}{\rho_c}\biggr)^{-1} \, .</math> | |||
</div> | |||
Notice that in the special case for which we have been able to identify analytically specifiable eigenvectors, namely, when | |||
<div align="center"> | |||
<math>~g^2 = \mathcal{B} </math> <math>~\Rightarrow</math> <math>~\Kappa = 1 \, ,</math> | |||
</div> | |||
this surface boundary condition simplifies to the ''expected'' expression, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | |||
\frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} | |||
<math>~ | |||
</math> | </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,650: | Line 1,853: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math>~\frac{1}{3} | ||
\biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \biggr] \, . | |||
- \ | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,661: | Line 1,860: | ||
</table> | </table> | ||
</div> | </div> | ||
Under what condition — other than when <math>~g^2=\mathcal{B}</math> — does the general expression generate the ''expected'' expression? We need, | |||
<div align="center"> | <div align="center"> | ||
| Line 1,668: | Line 1,868: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{1}{3} | ||
\biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \biggr] | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,674: | Line 1,876: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\biggl[2 + \Kappa \biggr] ^{-1} | ||
\biggl[ | \biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \Kappa \biggr] | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,686: | Line 1,884: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ (2 + \Kappa ) | |||
\biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \biggr] | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
3\biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \Kappa \biggr] | |||
\ | |||
\biggr] | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,702: | Line 1,900: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ | |||
(2 + \Kappa ) \mathfrak{F}_\mathrm{env} - (2 + \Kappa ) \alpha_e | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,709: | Line 1,909: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
3\mathfrak{F}_\mathrm{env} - 3\Kappa \alpha_e | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,718: | Line 1,916: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ | |||
(\Kappa -1) \mathfrak{F}_\mathrm{env} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
-2(\Kappa-1 ) \alpha_e | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\Rightarrow ~~~ | ||
\mathfrak{F}_\mathrm{env} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~2\ | <math>~ | ||
-2\alpha_e \, . | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
But, given that, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\mathfrak{F}_\mathrm{env}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,758: | Line 1,960: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} - 2\alpha_e | ||
\, | \, , | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,766: | Line 1,968: | ||
</div> | </div> | ||
we see that the ''expected'' boundary condition will result only for <math>~\omega_\mathrm{env}^2 = 0</math>, that is, only for, <math>~\sigma_c^2 = 0</math>. This is what we have been noticing as we have played with numerically generated eigenvectors: When integrating from the center of the zero-zero bipolytrope, to its surface, the naturally resulting (first) derivative of the eigenfunction at the surface of the configuration matches the ''expected'' surface boundary condition … | |||
* for all values of <math>\sigma_c^2</math>, when <math>~g^2= \mathcal{B}</math>, that is, when <math>~\Kappa=1</math>; | |||
* only for <math>~\sigma_c^2 = 0</math> in all other configurations, that is, for all <math>~\Kappa \ne 1</math>. | |||
What do we make of this? | |||
==Five Mode Summary== | |||
<div align="center"> | <div align="center"> | ||
<table border=" | <table border="1" cellpadding="5"> | ||
<tr> | |||
<td align="center" bgcolor="white"><math>~(\ell, j) = (2,1)</math></td> | |||
<td align="center" bgcolor="white"><math>~(\ell, j) = (2,2)</math></td> | |||
<td align="center" bgcolor="white"> </td> | |||
</tr> | |||
<tr> | |||
<td align="center">[[File:Annotate21.png|300px|Log(amplitude) plot for (ell,j) = (2,1)]]</td> | |||
<td align="center">[[File:Annotate22.png|300px|Log(amplitude) plot for (ell,j) = (2,2)]]</td> | |||
<td align="center"> </td> | |||
</tr> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <div align="center"><math>~\frac{3\omega^2}{2\pi G\rho_c} = 37.08874</math></div> | ||
<font size="-2">[[User:Tohline/Appendix/Ramblings/Additional_Analytically_Specified_Eigenvectors_for_Zero-Zero_Bipolytropes#Illustration21|more details …]]</font> | |||
</td> | </td> | ||
<td align=" | <td align="right"> | ||
< | <div align="center"><math>~\frac{3\omega^2}{2\pi G\rho_c} = 35.95210</math></div> | ||
<font size="-2">[[User:Tohline/Appendix/Ramblings/Additional_Analytically_Specified_Eigenvectors_for_Zero-Zero_Bipolytropes#Illustration22|more details …]]</font> | |||
<math>~ | |||
\ | |||
</ | |||
</td> | </td> | ||
<td align="center"> </td> | |||
</tr> | |||
<tr> | |||
<td align="center" bgcolor="lightblue" colspan="3"> </td> | |||
</tr> | |||
<tr> | |||
<td align="center" bgcolor="white"><math>~(\ell, j) = (3,1)</math></td> | |||
<td align="center" bgcolor="white"><math>~(\ell, j) = (3,2)</math></td> | |||
<td align="center" bgcolor="white"><math>~(\ell, j) = (3,3)</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">[[File:Annotate31.png|300px|Log(amplitude) plot for (ell,j) = (3,1)]]</td> | |||
<td align="center">[[File:Annotate32.png|300px|Log(amplitude) plot for (ell,j) = (3,2)]]</td> | |||
<td align="center">[[File:Annotate33.png|300px|Log(amplitude) plot for (ell,j) = (3,3)]]</td> | |||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
& | <div align="center"><math>~\frac{3\omega^2}{2\pi G\rho_c} = 12.452545</math></div> | ||
<font size="-2">[[User:Tohline/Appendix/Ramblings/Additional_Analytically_Specified_Eigenvectors_for_Zero-Zero_Bipolytropes#Illustration31|more details …]]</font> | |||
</td> | |||
<td align="right"> | |||
<div align="center"><math>~\frac{3\omega^2}{2\pi G\rho_c} = 35.05461</math></div> | |||
<font size="-2">[[User:Tohline/Appendix/Ramblings/Additional_Analytically_Specified_Eigenvectors_for_Zero-Zero_Bipolytropes#Illustration32|more details …]]</font> | |||
</td> | </td> | ||
<td align=" | <td align="right"> | ||
<div align="center"><math>~\frac{3\omega^2}{2\pi G\rho_c} = 87.41594</math></div> | |||
<font size="-2">[[User:Tohline/Appendix/Ramblings/Additional_Analytically_Specified_Eigenvectors_for_Zero-Zero_Bipolytropes#Illustration33|more details …]]</font> | |||
<math>~ | |||
</ | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
<div align="center"> | |||
<table border="1" align="center" cellpadding="5"> | |||
<tr> | |||
<th align="center">Model</th> | |||
<th align="center"><math>~\ell</math></th> | |||
<th align="center"><math>~j</math></th> | |||
<th align="center"><math>~q</math></th> | |||
<th align="center"><math>~\gamma_e</math></th> | |||
<th align="center"><math>~\gamma_c</math></th> | |||
<th align="center"><math>~\nu</math></th> | |||
<th align="center"><math>~\frac{\rho_e}{\rho_c}</math></th> | |||
<th align="center"><math>~\alpha_e</math></th> | |||
<th align="center"><math>~\alpha_c</math></th> | |||
<th align="center"><math>~n_e</math></th> | |||
<th align="center"><math>~n_c</math></th> | |||
<th align="center"><math>~g^2</math></th> | |||
<th align="center"><math>~f</math></th> | |||
<th align="center"><math>~\sigma_\mathfrak{G}^2</math></th> | |||
<th align="center">Analytic<br /><math>~\sigma_c^2</math></th> | |||
</tr> | |||
<tr> | |||
<td align="center">Analytic21</td> | |||
<td align="center">2</td> | |||
<td align="center">1</td> | |||
<td align="center">0.684</td> | |||
<td align="center">1.194</td> | |||
<td align="center">1.846</td> | |||
<td align="center">0.547</td> | |||
<td align="center">0.390</td> | |||
<td align="center">-0.35</td> | |||
<td align="center">+0.833</td> | |||
<td align="center">5.15</td> | |||
<td align="center">1.18</td> | |||
<td align="center">1.324</td> | |||
<td align="center">2.542</td> | |||
<td align="center">+0.761</td> | |||
<td align="center" bgcolor="pink">28.9116</td> | |||
</tr> | |||
<tr> | |||
<td align="center">Analytic22</td> | |||
<td align="center">2</td> | |||
<td align="center">2</td> | |||
<td align="center">0.887</td> | |||
<td align="center">1.799</td> | |||
<td align="center">1.022</td> | |||
<td align="center">0.799</td> | |||
<td align="center">0.583</td> | |||
<td align="center">+0.776</td> | |||
<td align="center">-0.914</td> | |||
<td align="center">1.25</td> | |||
<td align="center">46</td> | |||
<td align="center">1.146</td> | |||
<td align="center">1.444</td> | |||
<td align="center">-0.878</td> | |||
<td align="center" bgcolor="pink">34.9155</td> | |||
</tr> | |||
<tr> | |||
<td align="center">Analytic31</td> | |||
<td align="center">3</td> | |||
<td align="center">1</td> | |||
<td align="center">0.406</td> | |||
<td align="center">1.180</td> | |||
<td align="center">1.009</td> | |||
<td align="center">0.378</td> | |||
<td align="center">0.118</td> | |||
<td align="center">-0.390</td> | |||
<td align="center">-0.964</td> | |||
<td align="center">5.56</td> | |||
<td align="center">111</td> | |||
<td align="center">1.194</td> | |||
<td align="center">3.568</td> | |||
<td align="center">-0.180</td> | |||
<td align="center" bgcolor="pink">12.1770</td> | |||
</tr> | |||
<tr> | <tr> | ||
<td align=" | <td align="center">Analytic32</td> | ||
<td align="center">3</td> | |||
</td> | <td align="center">2</td> | ||
<td align="center"> | <td align="center">0.812</td> | ||
< | <td align="center">2.327</td> | ||
</td> | <td align="center">4.216</td> | ||
<td align=" | <td align="center">0.690</td> | ||
< | <td align="center">0.517</td> | ||
+ | <td align="center">+1.281</td> | ||
<td align="center">+2.051</td> | |||
<td align="center">0.754</td> | |||
</ | <td align="center">0.311</td> | ||
</td> | <td align="center">1.232</td> | ||
<td align="center">1.813</td> | |||
<td align="center">+9.654</td> | |||
<td align="center" bgcolor="pink">169.0733</td> | |||
</tr> | </tr> | ||
</table> | |||
</div> | |||
<div align="center"> | |||
<table border="1" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align=" | <td align="center" colspan="6"><math>~\sigma_c^2</math><br /> | ||
Determined from Numerical Integration by Enforcing Boundary Condition (B.C.)<br /> | |||
<math>\frac{d \ln x_\mathrm{env}}{d\ln \xi}\biggr|_\mathrm{surface} = c_0(2\ell+1) + \ell(3\ell+5)</math> | |||
<math>~ | |||
<math> | |||
</math> | |||
</td> | </td> | ||
</tr> | |||
<tr> | |||
<th align="center">Model</th> | |||
<td align="center"><math>~\ell=0</math><br /> <br />'''B.C.:''' <math>~c_0</math></td> | |||
<td align="center"><math>~\ell=1</math><br /> <br />'''B.C.:''' <math>~(3c_0+8)</math></td> | |||
<td align="center"><math>~\ell=2</math><br /> <br />'''B.C.:''' <math>~(5c_0+22)</math></td> | |||
<td align="center"><math>~\ell=3</math><br /> <br />'''B.C.:''' <math>~7(c_0+6)</math></td> | |||
<td align="center"><math>~\ell=4</math><br /> <br />'''B.C.:''' <math>~(9c_0+68)</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">Analytic21</td> | |||
<td align="center">-0.76017962</td> | |||
<td align="center">+9.881793</td> | |||
<td align="center" bgcolor="pink">+28.9116</td> | |||
<td align="center">+56.32919</td> | |||
<td align="center">-</td> | |||
</tr> | |||
<tr> | |||
<td align="center">Analytic22</td> | |||
<td align="center">-4.890477</td> | |||
<td align="center">+5.5864115</td> | |||
<td align="center" bgcolor="pink">+34.91550</td> | |||
<td align="center">+83.09678</td> | |||
<td align="center">-</td> | |||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | </div> | ||
==Broad Application== | |||
Here's one key lesson that can be drawn from our analytically specified oscillation modes. As has been [[#Core_Segment|documented above]], the quantum number, <math>~j</math>, associated with the eigenvector of the core is related to the oscillation frequency, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 1,841: | Line 2,170: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\sigma_c^2</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~\ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{3\omega^2}{2\pi G \rho_c} \, ,</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
via the expression, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\frac{\sigma_c^2}{\gamma_c} - 2\alpha_c</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ 2j(2j+5) </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,871: | Line 2,199: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\Rightarrow~~~\sigma_c^2</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{8[\alpha_c + j(2j+5)]}{3-\alpha_c} \, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
Also, as [[#Envelope_Segment|documented above]], the quantum number, <math>~\ell</math>, associated with the eigenvector of the envelope is related to the oscillation frequency via the expression, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{\sigma_c^2}{\gamma_e(\rho_e/\rho_c)} - 2\alpha_e</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~(c_0 + 3\ell)(c_0 + 3\ell + 5) </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~\sigma_c^2</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~\gamma_e \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[2\alpha_e + (c_0 + 3\ell)(c_0 + 3\ell + 5) \biggr]</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,915: | Line 2,246: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{ | <math>~\biggl(\frac{\rho_e}{\rho_c}\biggr) \frac{4[2c_0^2 + 4c_0 + c_0^2 + c_0(6\ell+5) + 3\ell(3\ell+5) ]}{3-\alpha_e}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,933: | Line 2,258: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{ | <math>~\biggl(\frac{\rho_e}{\rho_c}\biggr) \frac{12[c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) ]}{3-\alpha_e} \, ,</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
[[File:CommentButton02.png|right|100px|Comment by J. E. Tohline on 4 February 2017: This expression for the density ratio is a necessary but not sufficient relationship. According to each analytic solution, once the pair of quantum numbers <math>~(\ell,j)</math> has been specified, the two adiabatic indexes cannot be specified independently of one another.]]where, as a reminder, <math>~c_0 = [-1 \pm \sqrt{1+\alpha_e}]</math>. Eliminating <math>~\sigma_c^2</math> between these two relations gives, | |||
<div align="center" id="OtherSigmas"> | |||
<table border="1" cellpadding="8" align="center"><tr><td align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{\rho_e}{\rho_c}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,945: | Line 2,278: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{\ | <math>~\frac{2(3-\alpha_e) [\alpha_c + j(2j+5)]}{3(3-\alpha_c) [c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) ]} </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,957: | Line 2,290: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~c_0 \, . | <math>~\frac{(3-\alpha_e) \sigma_c^2}{12 [c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) ]} \, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</td></tr></table> | |||
</div> | </div> | ||
===Case A=== | |||
Consider, first, the astrophysical system in which <math>~(n_c, n_e) = (5, 1)</math>. Given that, in general, <math>~\alpha = (3-n)/(1+n)</math>, we therefore will be considering the system in which, <math>~(\alpha_c, \alpha_e) = (-\tfrac{1}{3}, 1) </math>. For this system, we should be able to analytically specify eigenvectors having the properties specified in the following table. | |||
<table border="1" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align=" | <td colspan="4" align="center"> | ||
< | Analytically Specifiable Eigenvectors for which<br /> | ||
<math>~(n_c, n_e) = (5, 1)</math> <math>~\Rightarrow</math> <math>~(\alpha_c, \alpha_e) = (-\tfrac{1}{3}, 1)</math> | |||
<math>~=</math> | |||
<math>~ | |||
( | |||
( | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align=" | <td colspan="2"> </td> | ||
<math>~ | <td colspan="2" align="center"><math>~\frac{\rho_e}{\rho_c}</math></td> | ||
</td> | </tr> | ||
<td align="center"> | <tr> | ||
<math>~=</math> | <td align="center"><math>~\ell</math></td> | ||
<td align="center"><math>~c_0</math></td> | |||
<td colspan="1" align="center"> | |||
<math>~j=1</math> <br /><math>~(\sigma_c^2 = 16)</math> | |||
</td> | </td> | ||
<td align=" | <td colspan="1" align="center"> | ||
<math>~ | <math>~j=2</math> <br /><math>~(\sigma_c^2 = 42.4)</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align=" | <td align="center" rowspan="2">1</td> | ||
<td align="center"><math>~-1 + \sqrt{2}</math></td> | |||
</td> | <td align="center">0.26034953</td> | ||
<td align="center"> | <td align="center">n/a <math>~(q > 1)</math></td> | ||
<math>~=</math> | </tr> | ||
</td> | <tr> | ||
<td align=" | <td align="center"><math>~-1 - \sqrt{2}</math></td> | ||
<math>~ | <td align="center">1.52 (n/a)</td> | ||
</td> | <td align="center">(n/a)</td> | ||
</tr> | |||
<tr> | |||
<td align="center" rowspan="2">2</td> | |||
<td align="center"><math>~-1 + \sqrt{2}</math></td> | |||
<td align="center">0.10636430</td> | |||
<td align="center">0.28186540</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~-1 - \sqrt{2}</math></td> | |||
<td align="center">0.24400066</td> | |||
<td align="center">0.64660175</td> | |||
</tr> | |||
<tr> | |||
<td align="center" rowspan="2">3</td> | |||
<td align="center"><math>~-1 + \sqrt{2}</math></td> | |||
<td align="center">0.05809795</td> | |||
<td align="center">0.15395957</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~-1 - \sqrt{2}</math></td> | |||
<td align="center">0.10216916</td> | |||
<td align="center">0.27074827</td> | |||
</tr> | </tr> | ||
</table> | </table> | ||
< | |||
=Related Discussions= | |||
* [[User:Tohline/Appendix/Ramblings/Additional_Analytically_Specified_Eigenvectors_for_Zero-Zero_Bipolytropes#Searching_for_Additional_Eigenvectors_of_Zero-Zero_Bipolytropes|Searching for Additional, Analytically Specified Eigenvectors of Zero-Zero Bipolytropes]] | |||
* [[User:Tohline/Appendix/Ramblings/NumericallyDeterminedEigenvectors#Numerically_Determined_Eigenvectors_of_a_Zero-Zero_Bipolytrope|Numerically Determined Eigenvectors]] | |||
<!-- OLD STUFF ... | |||
<div align="center"> | <div align="center"> | ||
<math>~\ | <math>~(\ell,j) = (2, 1)</math> <math>~\biggl|</math> <math>~\gamma_e = 1.194</math> <math>~\biggl|</math> <math>~\gamma_c = 2.254</math> <math>~\biggl|</math> <math>~\frac{3\omega^2}{2\pi G\rho_c} = 37.08874</math><br /> | ||
<br /> | |||
<math>~(\ell,j) = (2, 2)</math> <math>~\biggl|</math> <math>~\gamma_e =1.209 </math> <math>~\biggl|</math> <math>~\gamma_c = 1.046</math> <math>~\biggl|</math> <math>~\frac{3\omega^2}{2\pi G\rho_c} = 35.95210</math><br /> | |||
<br /> | |||
<math>~(\ell,j) = (3, 1)</math> <math>~\biggl|</math> <math>~\gamma_e = 1.344</math> <math>~\biggl|</math> <math>~\gamma_c = 1.023</math> <math>~\biggl|</math> <math>~\frac{3\omega^2}{2\pi G\rho_c} = 12.452545</math><br /> | |||
<br /> | |||
<math>~(\ell,j) = (3, 2)</math> <math>~\biggl|</math> <math>~\gamma_e = 1.056</math> <math>~\biggl|</math> <math>~\gamma_c = 1.025</math> <math>~\biggl|</math> <math>~\frac{3\omega^2}{2\pi G\rho_c} = 35.05461</math><br /> | |||
<br /> | |||
<math>~(\ell,j) = (3, 3)</math> <math>~\biggl|</math> <math>~\gamma_e = 1.840</math> <math>~\biggl|</math> <math>~\gamma_c = 1.325</math> <math>~\biggl|</math> <math>~\frac{3\omega^2}{2\pi G\rho_c} = 87.41594</math><br /> | |||
<br /> | |||
</div> | </div> | ||
<div align="center"> | <div align="center"> | ||
< | <table border="1" align="center"><tr><td align="center"> | ||
[[File:DenRatioAmpsMontage01.png|900px|Analytic Eigenfunctions for zero-zero bipolytropes]] | |||
</td></tr></table> | |||
</div> | </div> | ||
--> | |||
{{LSU_HBook_footer}} | {{LSU_HBook_footer}} | ||
Latest revision as of 21:19, 10 February 2017
Radial Oscillations of a Zero-Zero Bipolytrope
This chapter includes the interface-matching condition specified by P. Ledoux & Th. Walraven (1958). It replaces an earlier overview chapter, which summarized models in which an incorrect interface matching condition was used.

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
In a separate chapter on astrophysical interesting equilibrium structures, we have derived analytical expressions that define the equilibrium properties of bipolytropic configurations having <math>~(n_c, n_e) = (0, 0)</math>, that is, bipolytropes in which both the core and the envelope are uniform in density, but the densities in the two regions are different from one another. Letting <math>~R</math> be the radius and <math>~M_\mathrm{tot}</math> be the total mass of the bipolytrope, these configurations are fully defined once any two of the following three key parameters have been specified: The envelope-to-core density ratio, <math>~\rho_e/\rho_c</math>; the radial location of the envelope/core interface, <math>~q \equiv r_i/R</math>; and, the fractional mass that is contained within the core, <math>~\nu \equiv M_\mathrm{core}/M_\mathrm{tot}</math>. These three parameters are related to one another via the expression,
|
<math>~\frac{\rho_e}{\rho_c}</math> |
<math>~=</math> |
<math>~\frac{q^3}{\nu} \biggl( \frac{1-\nu}{1-q^3} \biggr) \, .</math> |
Equilibrium configurations can be constructed that have a wide range of parameter values; specifically,
<math>~0 \le q \le 1 \, ;</math> <math>~0 \le \nu \le 1 \, ;</math> and, <math>~0 \le \frac{\rho_e}{\rho_c} \le 1 \, .</math>
(We recognize from buoyancy arguments that any configuration in which the envelope density is larger than the core density will be Rayleigh-Taylor unstable, so we restrict our astrophysical discussion to structures for which <math>~\rho_e < \rho_c</math>.)
By employing the linear stability analysis techniques described in an accompanying chapter, we should, in principle, be able to identify a wide range of eigenvectors — that is, radial eigenfunctions and accompanying eigenfrequencies — that are associated with adiabatic radial oscillation modes in any one of these equilibrium, bipolytropic configurations. Using numerical techniques, Murphy & Fiedler (1985), for example, have carried out such an analysis of bipolytropic structures having <math>~(n_c, n_e) = (1,5)</math>. A pair of linear adiabatic wave equations (LAWEs) must be solved — one tuned to accommodate the properties of the core and another tuned to accommodate the properties of the envelope — then the pair of eigenfunctions must be matched smoothly at the radial location of the interface; the identified core- and envelope-eigenfrequencies must simultaneously match.
After identifying the precise form of the LAWEs that apply to the case of <math>~(n_c, n_e) = (0,0)</math> bipolytropes, we discovered that, for a restricted range of key parameters, the pair of equations can both be solved analytically.
Two Separate LAWEs
In an accompanying discussion, we derived the so-called,
Adiabatic Wave (or Radial Pulsation) Equation
|
<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math> |
For both regions of the bipolytrope, we define the dimensionless (Lagrangian) radial coordinate,
<math>~\xi \equiv \frac{r_0}{r_i} \, .</math>
So, the interface is, by definition, located at <math>~\xi = 1</math>; and, the surface is necessarily at <math>~\xi = q^{-1}</math>. As the material in the bipolytrope's core (envelope) is compressed/de-compressed during a radial oscillation, we will assume that heating/cooling occurs in a manner prescribed by an adiabat of index <math>~\gamma_c ~(\gamma_e)</math>; in general, <math>~\gamma_e \ne \gamma_c</math>. For convenience, we will also adopt the frequently used shorthand "alpha" notation,
<math>~\alpha_c \equiv 3 - \frac{4}{\gamma_c} \, ,</math> and <math>~\alpha_e \equiv 3 - \frac{4}{\gamma_e} \, .</math>
The Core's LAWE
After adopting, for convenience, the function notation,
|
<math>~g^2</math> |
<math>~\equiv</math> |
<math> 1 + \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \biggl( 1-q \biggr) + \frac{\rho_e}{\rho_c} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] \, , </math> |
we have deduced that, for the core, the LAWE may be written in the form,
|
<math>~0</math> |
<math>~=</math> |
<math>~ (1 - \eta^2)\frac{d^2x}{d\eta^2} + ( 4 - 6\eta^2 ) \frac{1}{\eta} \cdot \frac{dx}{d\eta} + \mathfrak{F}_\mathrm{core} x \, . </math> |
where,
<math>~\eta \equiv \frac{\xi}{g} \, ,</math> and <math>~\mathfrak{F}_\mathrm{core} \equiv \frac{3\omega_\mathrm{core}^2}{2\pi G\gamma_c \rho_c} - 2\alpha_c\, .</math>
Not surprisingly, this is identical in form to the eigenvalue problem that was first presented — and solved analytically — by Sterne (1937) in connection with his examination of radial oscillations in isolated uniform-density spheres. As is demonstrated below, for the core of our zero-zero bipolytrope, we can in principle adopt any one of the polynomial eigenfunctions and corresponding eigenfrequencies derived by Sterne.
The Envelope's LAWE
Subsequently, we also have deduced that, for the envelope, the governing LAWE becomes,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \biggl[ 1 + \frac{(g^2-\mathcal{B}) \xi}{\mathcal{A}} - \mathcal{D} \xi^3\biggr] \frac{d^2x}{d\xi^2} + \biggl\{ 3 + \frac{4(g^2-\mathcal{B}) \xi}{\mathcal{A}} - 6\mathcal{D} \xi^3 \biggr\} \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ \mathcal{D} \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]\frac{x}{\xi^2} \, , </math> |
where,
|
<math>~\mathcal{A}</math> |
<math>~\equiv</math> |
<math>~2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \, ; </math> |
|
<math>~\mathcal{B}</math> |
<math>~\equiv</math> |
<math>~1 + 2\biggl(\frac{\rho_e}{\rho_c}\biggr) - 3\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 \, , </math> |
|
<math>~\mathcal{D}</math> |
<math>~\equiv</math> |
<math>~\frac{1}{\mathcal{A}}\biggl( \frac{\rho_e}{\rho_c}\biggr)^2 = \biggl[ \frac{\rho_e/\rho_c}{2(1-\rho_e/\rho_c)} \biggr] \, , </math> |
|
<math>~\mathfrak{F}_\mathrm{env}</math> |
<math>~\equiv</math> |
<math>~\frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} - 2\alpha_e \, . </math> |
We have been unable to demonstrate that this governing equation can be solved analytically for arbitrary pairs of the key model parameters, <math>~q</math> and <math>~\rho_e/\rho_c</math>. But, if we choose parameter value pairs that satisfy the constraint,
<math>~g^2 = \mathcal{B} </math> <math>~\Rightarrow</math> <math>~g^2 = \frac{1+8q^3}{(1+2q^3)^2} \, ,</math> and, <math>~q^3 = \mathcal{D} = \biggl[ \frac{\rho_e/\rho_c}{2(1-\rho_e/\rho_c)} \biggr] </math> <math>~\Rightarrow</math> <math>~\frac{\rho_e}{\rho_c} = \frac{2q^3}{1+2q^3} \, ,</math>
then the LAWE that is relevant to the envelope simplifies. Specifically, it takes the form,
|
<math>~0</math> |
<math>~=</math> |
<math>~ ( 1 - q^3 \xi^3 ) \frac{d^2x}{d\xi^2} + ( 3 - 6q^3 \xi^3 ) \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]\frac{x}{\xi^2} </math> |
|
|
<math>~=</math> |
<math>~\frac{x}{\xi^2}\biggl\{ ( 1 - q^3 \xi^3 ) \biggl[ \frac{d}{d\ln\xi} \biggl( \frac{d\ln x}{d\ln \xi} \biggr) - \biggl( 1 - \frac{d\ln x}{d\ln \xi} \biggr)\cdot \frac{d\ln x}{d\ln \xi}\biggr] + ( 3 - 6q^3 \xi^3 ) \frac{d\ln x}{d\ln \xi} + \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr] \biggr\} \, . </math> |
Shortly after deriving this last expression, we realized that one possible solution is a simple power-law eigenfunction of the form,
<math>~x=a_0 \xi^{c_0} \, ,</math>
where the (constant) exponent is one of the roots of the quadratic equation,
<math>~c_0^2 + 2c_0 - \alpha_e = 0 \, ,</math> <math>~\Rightarrow</math> <math>~c_0 = -1 \pm \sqrt{1+\alpha_e} \, .</math>
This power-law eigenfunction must be paired with the associated, dimensionless eigenfrequency parameter,
|
<math>~\mathfrak{F}_\mathrm{env}</math> |
<math>~=</math> |
<math>~c_0(c_0+5) = 3c_0 + \alpha_e</math> |
|
<math>~\Rightarrow ~~~ \frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} </math> |
<math>~=</math> |
<math>~ 3(c_0 + \alpha_e) = 3[\alpha_e -1 \pm \sqrt{1+\alpha_e}] \, .</math> |
Next, we noticed the strong similarities between the mathematical properties of this eigenvalue problem and the one that was studied by C. Prasad (1948, MNRAS, 108, 414-416) in connection with, what we now recognize to be, a closely related problem. Drawing heavily from Prasad's analysis, we discovered an infinite number of eigenfunctions (each, a truncated polynomial expression) and associated eigenfrequencies that satisfy this governing envelope LAWE. The eigenvectors associated with the lowest few modes are tabulated, below.
Eigenvector
Core Segment
| Table 1: Analytically Specifiable Core Eigenvectors | ||
|---|---|---|
|
Mode |
Core Eigenfunction |
Core Eigenfrequency <math>~\frac{3\omega_\mathrm{core}^2}{2\pi \gamma_c G \rho_c} = 2[\alpha_c + j(2j+5)]</math> |
|
<math>~j=0 </math> |
<math>~x_\mathrm{core} = a_0 </math> |
<math>~6-8/\gamma_c</math> |
|
<math>~j=1 </math> |
<math>~x_\mathrm{core} = a_0 \biggl[ 1 - \frac{7}{5}\biggr(\frac{\xi^2}{g^2}\biggr) \biggr]</math> |
<math>~20-8/\gamma_c</math> |
|
<math>~j=2 </math> |
<math>~x_\mathrm{core} = a_0 \biggl[ 1 - \frac{18}{5}\biggr(\frac{\xi^2}{g^2}\biggr) + \frac{99}{35}\biggr(\frac{\xi^2}{g^2}\biggr)^2 \biggr]</math> |
<math>~42-8/\gamma_c</math> |
Envelope Segment
| Table 2: Analytically Specifiable Envelope Eigenvectors | ||||||||
|---|---|---|---|---|---|---|---|---|
|
Mode |
Envelope Eigenfunction |
Envelope Eigenfrequency <math>~\frac{3\omega_\mathrm{env}^2}{2\pi \gamma_e G \rho_e} = 3[\alpha_e + c_0(2\ell+1) + \ell(3\ell+5)]</math> |
||||||
|
<math>~\ell=0 </math> |
<math>~x_\mathrm{env} = b_0 \xi^{c_0}</math> |
<math>~3[\alpha_e + c_0]</math> |
||||||
|
<math>~\ell=1 </math> |
<math>~x_\mathrm{env} = b_0 \xi^{c_0}\biggl\{1 + \biggl[ \frac{c_0(c_0+5)-(c_0+3)(c_0+8)}{(c_0+3)(c_0+5) - \alpha_e} \biggr](q\xi)^3 \biggr\}</math> |
<math>~3[\alpha_e + 3c_0 +8]</math> |
||||||
|
<math>~\ell=2 </math> |
|
<math>~3[\alpha_e + 5c_0 +22]</math> |
||||||
Piecing Together
Here we illustrate how the two segments of the eigenfunction can be successfully pieced together for the specific case of <math>~(\ell,j) = (2,1)</math>.
STEP 1: Choose a value of the adiabatic exponent for the envelope, <math>~\gamma_e</math>. Then, the values of both <math>~\alpha_e</math> and <math>~c_0</math> are known as well; actually, because it is the root of a quadratic equation, <math>~c_0</math> can, in general, take on one of a pair of values. We will elaborate on this further, below.
STEP 2: Acknowledging that the value of <math>~q</math> has yet to be determined, fix the value of the leading, overall scaling coefficient, <math>~b_0</math>, such that† <math>~x_\mathrm{env} = 1</math> at the interface, that is, at <math>~\xi = 1</math>. For the case of <math>~\ell=2</math>, this means that, throughout the envelope, the eigenfunction is,
|
<math>~x_{\ell=2} |_\mathrm{env}</math> |
<math>~=</math> |
<math>~ \xi^{c_0}\biggl[ \frac{ 1 + q^3 A_{2} \xi^{3} + q^6 A_{2}B_{2}\xi^{6} }{ 1 + q^3 A_{2} + q^6 A_{2}B_{2}}\biggr] \, , </math> |
where, the values of the newly introduced coefficients,
|
<math>~A_{2}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \frac{c_0(c_0+5) - (c_0 + 6)(c_0 + 11)}{(c_0 + 3)(c_0+5) - \alpha_e}\biggr] \, ,</math> |
|
<math>~B_{2}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \frac{(c_0+3)(c_0+8) - (c_0 + 6)(c_0 + 11)}{(c_0 + 6)(c_0+8) - \alpha_e}\biggr] \, ,</math> |
are also both known.
STEP 3: Recognizing that this segment of the eigenfunction will only satisfy the envelope's LAWE if we restrict our discussion to equilibrium models for which <math>~g^2 = \mathcal{B} = [(1+8q^3)/(1+2q^3)^{2}]</math>, we must insert this same restriction on <math>~g^2</math> into the core's eigenfunction. At the same time, we should fix the value of the leading, overall scaling coefficient, <math>~a_0</math>, such that† <math>~x_\mathrm{core} = 1</math> at the interface <math>~(\xi = 1)</math>. For the case of <math>~j=1</math>, this means that, throughout the core, the eigenfunction is,
|
<math>~x_{j=1} |_\mathrm{core}</math> |
<math>~=</math> |
<math>~ \frac{5(1+8q^3) - 7 (1+2q^3)^2 \xi^2}{5(1+8q^3)-7(1+2q^3)^2} \, .</math> |
STEP 4: Now we need to match the two eigenfunctions at the interface. Following the discussion in §§57 & 58 of P. Ledoux & Th. Walraven (1958), the proper treatment is to ensure that fractional perturbation in the gas pressure (see their equation 57.31),
|
<math>~\frac{\delta P}{P}</math> |
<math>~=</math> |
<math>~- \gamma x \biggl( 3 + \frac{d\ln x}{d\ln \xi} \biggr) \, ,</math> |
is continuous across the interface. That is to say, at the interface <math>~(\xi = 1)</math>, we need to enforce the relation,
|
<math>~0</math> |
<math>~=</math> |
<math>~\biggl[ \gamma_c x_\mathrm{core} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \gamma_e x_\mathrm{env} \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=1}</math> |
|
|
<math>~=</math> |
<math>~\gamma_e \biggl[ \frac{\gamma_c}{\gamma_e} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=1}</math> |
|
<math>~\Rightarrow~~~ \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr|_{\xi=1}</math> |
<math>~=</math> |
<math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl( \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr)_{\xi=1} \, .</math> |
In the context of this interface-matching constraint (see their equation 62.1), P. Ledoux & Th. Walraven (1958) state the following: In the static (i.e., unperturbed equilibrium) model … discontinuities in <math>~\rho</math> or in <math>~\gamma</math> might occur at some [radius]. In the first case — that is, a discontinuity only in density, while <math>~\gamma_e = \gamma_c</math> — the interface conditions imply the continuity of <math>~\tfrac{1}{x} \cdot \tfrac{dx}{d\xi}</math> at that [radius]. In the second case — that is, a discontinuity in the adiabatic exponent — the dynamical condition may be written as above. This implies a discontinuity of the first derivative at any discontinuity of <math>~\gamma</math>.
When evaluated at the interface, the logarithmic derivatives of our pair of parameterized eigenfunction expressions are, respectively,
|
<math>\frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr|_{\xi=1}</math> |
<math>~=</math> |
<math>~ c_0 + \frac{3A_{2}\Chi + 6A_{2}B_{2} \Chi^2}{1 + A_{2}\Chi + A_{2}B_{2}\Chi^2} \, ; </math> |
|
<math> \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr|_{\xi=1}</math> |
<math>~=</math> |
<math>~\frac{14(1+2\Chi)^2}{7(1+2\Chi)^2 - 5(1+8\Chi)} \, ,</math> |
where we have made the notation substitution, <math>~\Chi \equiv q^3</math>. Allowing for a step function in the adiabatic exponent at the interface, our interface-matching constraint is, therefore,
|
<math>~ \frac{\gamma_c}{\gamma_e} \biggl[ \frac{14(1+2\Chi)^2}{7(1+2\Chi)^2 - 5(1+8\Chi)} \biggr] </math> |
<math>~=</math> |
<math>~ c_0 + \frac{3A_{2}\Chi + 6A_{2}B_{2} \Chi^2}{1 + A_{2}\Chi + A_{2}B_{2}\Chi^2} - 3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) </math> |
|
|
<math>~=</math> |
<math>~ \frac{\mathfrak{g}_0 + (\mathfrak{g}_0+3)A_{2}\Chi + (\mathfrak{g}_0+6)A_{2}B_{2} \Chi^2}{1 + A_{2}\Chi + A_{2}B_{2}\Chi^2} \, , </math> |
where,
<math>~\mathfrak{g}_0 \equiv c_0 + 3\biggl(1-\frac{\gamma_c}{\gamma_e} \biggr) \, .</math>
This can be rewritten as,
|
<math>~ 0 </math> |
<math>~=</math> |
<math>~ [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] [7(1+2\Chi)^2 - 5(1+8\Chi)] - 14(\gamma_c/\gamma_e) (1+2\Chi)^2 [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] </math> |
|
|
<math>~=</math> |
<math>~ [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] [2 - 12\Chi + 28\Chi^2 ] - (14 + 56\Chi + 56 \Chi^2)(\gamma_c/\gamma_e) [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] </math> |
|
|
<math>~=</math> |
<math>~ 2[\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] -12\Chi [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] + 28\Chi^2 [\mathfrak{g}_0 + (\mathfrak{g}_0 + 3)A_{2}\Chi + (\mathfrak{g}_0 + 6)A_{2}B_{2} \Chi^2] </math> |
|
|
|
<math>~ - 14(\gamma_c/\gamma_e) [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] - 56(\gamma_c/\gamma_e)\Chi [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] - 56 (\gamma_c/\gamma_e)\Chi^2 [1 + A_{2}\Chi + A_{2}B_{2}\Chi^2] \, . </math> |
Or we have, equivalently,
|
<math>~a\Chi^4 + b\Chi^3 + c\Chi^2 +d\Chi +e </math> |
<math>~=</math> |
<math>~0 \, ,</math> |
where,
|
<math>~e</math> |
<math>~\equiv</math> |
<math>~ 2\mathfrak{g}_0 - 14(\gamma_c/\gamma_e) \, ,</math> |
|
<math>~d</math> |
<math>~\equiv</math> |
<math>~2(\mathfrak{g}_0+3)A_{2} - 12\mathfrak{g}_0 - 14(\gamma_c/\gamma_e)A_{2} - 56(\gamma_c/\gamma_e)</math> |
|
|
<math>~=</math> |
<math>~2[\mathfrak{g}_0 + 3 -7(\gamma_c/\gamma_e)]A_{2} - 4[14(\gamma_c/\gamma_e) + 3\mathfrak{g}_0] \, ,</math> |
|
<math>~c</math> |
<math>~\equiv</math> |
<math>~2(\mathfrak{g}_0+6)A_{2}B_{2} - 12(\mathfrak{g}_0+3)A_{2} + 28\mathfrak{g}_0 - 14(\gamma_c/\gamma_e)A_{2}B_{2} - 56(\gamma_c/\gamma_e)A_{2} - 56(\gamma_c/\gamma_e) </math> |
|
|
<math>~=</math> |
<math>~2[\mathfrak{g}_0 + 6 -7(\gamma_c/\gamma_e)] A_{2}B_{2} - 4[9 + 14(\gamma_c/\gamma_e) + 3\mathfrak{g}_0]A_{2} + 28[\mathfrak{g}_0 - 2(\gamma_c/\gamma_e)] \, ,</math> |
|
<math>~b</math> |
<math>~\equiv</math> |
<math>~-12(\mathfrak{g}_0+6)A_{2}B_{2} + 28(\mathfrak{g}_0+3)A_{2} - 56(\gamma_c/\gamma_e)A_{2}B_{2} - 56(\gamma_c/\gamma_e)A_{2} </math> |
|
|
<math>~=</math> |
<math>~- 4[3\mathfrak{g}_0+18+14(\gamma_c/\gamma_e)]A_{2}B_{2} + 28[\mathfrak{g}_0+3-2(\gamma_c/\gamma_e)]A_{2} \, ,</math> |
|
<math>~a</math> |
<math>~\equiv</math> |
<math>~28[\mathfrak{g}_0+6 - 2(\gamma_c/\gamma_e)]A_{2}B_{2} \, .</math> |
The physically relevant (real) root of this quartic equation in <math>~\Chi</math> — see our accompanying detailed presentation — gives us the specific value of the dimensionless interface location, <math>~q</math>, for which the values of the two eigenfunctions match at the interface, and for which the first derivatives of the two eigenfunctions are discontinuous by the properly prescribed amount at the interface.
Example Solutions
| Table 3: Example Analytic Model Parameters for <math>~(\ell,j) = (2,1)</math> NOTE: <math>\mathfrak{F}_\mathrm{core} = 14</math> | |||||
|---|---|---|---|---|---|
| Eigenfunction | <math>~\gamma_c ~(n_c)</math> | <math>~\gamma_e</math> | <math>~q</math> | <math>~\frac{\rho_e}{\rho_c}</math> | <math>~\sigma_c^2</math> |
| <math>~\frac{5}{3} ~~\biggl(\frac{3}{2} \biggr)</math> | 1.1340607 | 0.6684554 | 0.3739731 | 25.333333 | |
| <math>~\frac{4}{3} ~~\biggl( 3 \biggr)</math> | 1.0263212 | 0.6385711 | 0.3424445 | 18.666666 | |
| <math>~\frac{6}{5} ~~\biggl( 5 \biggr)</math> | 1.0028319 | 0.6187646 | 0.3214875 | 16.000000 | |
It appears as though the eigenvectors (eigenfunction and eigenfrequency) of other radial oscillation modes can be identified by holding all other parameters fixed but changing the value of the quantum number, <math>~\ell</math>, in the expression provided below. Picking the configuration identified as model C21 in Table 3, for example, in addition to the parameter values provided in the table we have,
|
<math>~\alpha_e = 3 - \frac{4}{\gamma_e} = -0.9887044</math> |
and, |
<math>~c_0 = \sqrt{1+\alpha_e} - 1 = -0.8937192 \, ,</math> |
so we expect the variation in (the square of) the eigenfrequency, <math>~\sigma_c</math>, with <math>~\ell</math> to be,
|
<math>~\sigma_c^2</math> |
<math>~=</math> |
<math>~12\biggl[\frac{c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) }{(3-\alpha_e) } \biggr]\frac{\rho_e}{\rho_c} </math> |
|
|
<math>~=</math> |
<math>~0.9671938[c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) ] </math> |
|
|
<math>~=</math> |
<math>~0.9671938[-1.8824236 +(3.2125616)\ell + 3\ell^2 ] \, .</math> |
| Table 4: Additional Hypothesized Oscillation Modes for Model C21 | |||||
|---|---|---|---|---|---|
| <math>~\ell = 0</math><math>~\sigma_c^2 = -1.821</math> | <math>~\ell = 1</math><math>~\sigma_c^2 = +4.188</math> | <math>~\ell = 2</math><math>~\sigma_c^2 = +16</math> | <math>~\ell = 3</math><math>~\sigma_c^2 = +33.615</math> | <math>~\ell = 4</math><math>~\sigma_c^2 = +57.033</math> | <math>~\ell = 5</math><math>~\sigma_c^2 = +86.255</math> |
Each of the six plots displayed in Table 4 (click on a panel in order to view a larger image) was generated numerically by integrating the LAWE for the core, outward from the center of the configuration to the core/envelope interface, then integrating the LAWE for the envelope, from the interface outward to the surface. At the interface: the value of the envelope eigenfunction is set to the value of the eigenfunction of the core; and the slope of the envelope's eigenfunction (highlighted graphically in each plot by the green, dashed line segment) was based on the slope of the core's eigenfunction (highlighted graphically by the orange, dashed line segment) but shifted in a discontinuous fashion according to the above "Step 4" discussion. Each of the graphically illustrated Table 4 eigenfunctions has been scaled in such a way that the central value is unity; note that the panel labeled <math>~(\ell=2; \sigma_c^2 = +16)</math> displays an eigenfunction that is identical to the analytically defined eigenfunction displayed as Model C21 in Table 3, but it has been rescaled — and by necessity inverted — to provide a central value of unity.
Material that appears after this point in our presentation is under development and therefore
may contain incorrect mathematical equations and/or physical misinterpretations.
| Go Home |
Old, Incorrect Solutions
As is shown by the plot displayed in the right-hand panel of Figure 1, we have found different values of <math>~q</math> for each choice (STEP 1) of <math>~\gamma_e</math> (or, equivalently, choice of <math>~\alpha_e</math>). In this plot we have purposely flipped the horizontal axis so that the extreme left <math>~(\alpha_e = +3)</math> represents an incompressible <math>~(n = 0)</math> envelope, while the extreme right represents an isothermal <math>~(\gamma_e = 1)</math> envelope.
| Figure 1 | |||||
|---|---|---|---|---|---|
| <math>~\alpha_e = -0.35 \, ;~~~c_0 = \sqrt{1+\alpha_e} - 1</math> |
|
||||
| <math>~c_0</math> (plus): | <math>~-0.1937742</math> | ||||
| <math>~\gamma_e</math>: | <math>~1.1940299</math> | ||||
| <math>~n_e</math>: | <math>~5.1538462</math> | ||||
| <math>~q</math>: | <math>~0.6840119</math> | ||||
| <math>~\nu</math>: | <math>~0.5466868</math> | ||||
| <math>~\rho_e/\rho_c</math>: | <math>~0.3902664</math> | ||||
| <math>~\alpha_c</math>: | <math>~+0.8326585</math> | ||||
| <math>~\gamma_c</math>: | <math>~+1.845579</math> | ||||
| <math>~\alpha_e = -0.9 \, ;~~~c_0 = -\sqrt{1+\alpha_e} - 1</math> | |||||
| <math>~c_0</math> (minus): | <math>~- 1.3162278</math> | ||||
| <math>~\gamma_e</math>: | <math>~1.0256410</math> | ||||
| <math>~n_e</math>: | <math>~39</math> | ||||
| <math>~q</math>: | <math>~0.5728050</math> | ||||
| <math>~\nu</math>: | <math>~0.4586270</math> | ||||
| <math>~\rho_e/\rho_c</math>: | <math>~0.2731929</math> | ||||
| <math>~\alpha_c</math>: | <math>~-0.9595214</math> | ||||
| <math>~\gamma_c</math>: | <math>~+1.0102231</math> | ||||
STEP 5: Finally, for each choice of <math>~\gamma_e</math> — or, alternatively, <math>~\alpha_e</math> — the physically relevant value of the core's adiabatic exponent is set by demanding that the dimensional eigenfrequencies of the envelope and core precisely match. That is, we demand that,
| Figure 2 |
|---|
<math>~\omega^2_\mathrm{env} = \omega^2_\mathrm{core} \, .</math>
From above, we know that, for the core,
<math>~3\omega^2_\mathrm{core}\biggr|_\mathrm{j=1} = 2\pi \gamma_c G \rho_c [ 20 - 8/\gamma_c] \, ;</math>
whereas, for the envelope,
<math>~3\omega^2_\mathrm{env}\biggr|_\mathrm{\ell=2} = 2\pi \gamma_e G \rho_e [ 3(\alpha_e + 5c_0 + 22)] \, .</math>
By demanding that these frequencies be identical, we conclude that,
|
<math>~ \gamma_c </math> |
<math>~=</math> |
<math>~\frac{1}{20} \biggl[ 8 + 3\gamma_e \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(\alpha_e + 5c_0 + 22 \biggr)\biggr] \, .</math> |
Figure 2 shows how the required value of <math>~\alpha_c</math> varies with the choice of <math>~\alpha_e</math>; here, both axes have been flipped in order to run from incompressible <math>~(\alpha = +3)</math> at the left/bottom, to isothermal <math>~(\alpha = -1)</math> at the right/top. For the lower portion of the curve (red circular markers), the parameter, <math>~c_0</math>, is taken to be the "plus" root of its defining quadratic equation; the "minus" root defines <math>~c_0</math> along the upper portion of the curve (purple circular markers). The diagonal dashed-black line identifies where <math>~\alpha_c = \alpha_e</math>; in models below and to the right of this line, the envelope is more compressible than is the core, whereas in models above and to the left of this line, the core is more compressible than the envelope.
The eigenfrequency that corresponds to the specific eigenfunction that is displayed in upper-left quadrant of Figure 1 is identified by the black circular marker in Figure 2; as is indicated by the row of numbers on the left in Figure 1, this model has,
<math>~\gamma_c = 1.845579 </math> <math>~\Rightarrow </math> <math>~\alpha_c = +0.8326535 \, . </math>
The yellow circular marker in Figure 2 identifies the model whose analytically prescribed, <math>~(\ell,j) = (2,1)</math> eigenfunction is displayed in the lower-left quadrant of Figure 1; it has,
<math>~\gamma_c = 1.0102231 </math> <math>~\Rightarrow </math> <math>~\alpha_c = -0.9595214 \, . </math>
Examining Alignment with Surface Boundary Condition
Expectation
As we have reviewed in an accompanying discussion, one astrophysically reasonable surface boundary condition provides a mathematical relationship between the logarithmic derivative of the eigenfunction with respect to the radius, in terms of the eigenfrequency as follows:
|
<math>~ \frac{d\ln x}{d\ln \xi}\biggr|_{\xi = 1/q}</math> |
<math>~=</math> |
<math>~\frac{3\omega^2 }{4\pi G\rho_c \gamma_e} \biggl( \frac{\nu}{q^3}\biggr) - \biggl( 3 - \frac{4}{\gamma_e}\biggr) </math> |
|
|
<math>~=</math> |
<math>~\frac{3\omega^2 }{4\pi G\rho_c \gamma_e} \biggl( \frac{1+2q^3}{3q^3}\biggr) - \alpha_e </math> |
|
|
<math>~=</math> |
<math>~\frac{1}{3} \biggl[\frac{3\omega^2 }{2\pi G\rho_c \gamma_e} \biggr] \biggl( \frac{\rho_c}{\rho_e}\biggr) - \alpha_e </math> |
|
|
<math>~=</math> |
<math>~\frac{1}{3} \biggl[\mathfrak{F}_\mathrm{env} + 2\alpha_e \biggr] - \alpha_e </math> |
|
|
<math>~=</math> |
<math>~\frac{1}{3} \biggl[\mathfrak{F}_\mathrm{env} - \alpha_e \biggr] \, . </math> |
Now, according to our above-described envelope segment of the eigenfunction, we established the analytic prescription,
|
<math>~\mathfrak{F}_\mathrm{env}</math> |
<math>~=</math> |
<math>~(c_0 + 3\ell)(c_0 + 3\ell+5) \, ,</math> |
in which case the desired surface boundary condition is,
|
<math>~ 3 \cdot \frac{d\ln x}{d\ln \xi}\biggr|_{\xi = 1/q}</math> |
<math>~=</math> |
<math>~ (c_0 + 3\ell)(c_0 + 3\ell+5) - \alpha_e </math> |
|
|
<math>~=</math> |
<math>~ [c_0^2 + c_0(6\ell + 5 ) + 3\ell(3\ell+5)] - (c_0^2 + 2c_0)</math> |
|
|
<math>~=</math> |
<math>~ 3[ c_0(2\ell + 1 ) + \ell(3\ell+5)]</math> |
That is, we expect to find the following,
| ||||||
Analytic2
Continuing, from above, a discussion specifically of the case, <math>~\ell = 2</math>, the analytically specified envelope eigenfunction is,
|
<math>~x_{\ell=2} |_\mathrm{env}</math> |
<math>~=</math> |
<math>~ \xi^{c_0}\biggl[ \frac{ 1 + q^3 A_{\ell=2} \xi^{3} + q^6 A_{\ell=2}B_{\ell=2}\xi^{6} }{ 1 + q^3 A_{\ell=2} + q^6 A_{\ell=2}B_{\ell=2}}\biggr] \, , </math> |
where, the values of the newly introduced coefficients,
|
<math>~A_{\ell=2}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \frac{c_0(c_0+5) - (c_0 + 6)(c_0 + 11)}{(c_0 + 3)(c_0+5) - \alpha_e}\biggr] = \frac{-2(2c_0+11)}{(2c_0+5)} \, ,</math> |
|
<math>~B_{\ell=2}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \frac{(c_0+3)(c_0+8) - (c_0 + 6)(c_0 + 11)}{(c_0 + 6)(c_0+8) - \alpha_e}\biggr] = \frac{-(c_0+7)}{2(c_0+4)} \, ,</math> |
in which case,
|
<math>~\frac{d\ln x}{d\ln \xi} = \frac{\xi}{x} \cdot \frac{dx}{d\xi} \biggl|_\mathrm{env}</math> |
<math>~=</math> |
<math>~\frac{\xi}{x} \biggl\{ c_0\xi^{c_0-1}\biggl[ \frac{ 1 + q^3 A \xi^{3} + q^6 AB\xi^{6} }{ 1 + q^3 A + q^6 AB}\biggr] + \xi^{c_0}\biggl[ \frac{ 3q^3 A \xi^{2} + 6q^6 AB\xi^{5} }{ 1 + q^3 A + q^6 AB}\biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ c_0 + \biggl[ \frac{ 3q^3 A \xi^{3} + 6q^6 AB\xi^{6} }{ 1 + q^3 A\xi^3 + q^6 AB\xi^6}\biggr] \, . </math> |
Hence, at the surface <math>~(\xi = 1/q)</math>, we find,
|
<math>~\frac{d\ln x}{d\ln \xi} \biggl|_{\xi=1/q}</math> |
<math>~=</math> |
<math>~ c_0 +\biggl[ \frac{ 3 A + 6 AB }{ 1 + A + AB}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ c_0 +\biggl[ \frac{ -12(2c_0+11)(c_0+4) + 12(2c_0+11)(c_0+7) }{ 2(2c_0 + 5)(c_0+4) - 4(2c_0+11)(c_0+4) + 2(2c_0+11) (c_0+7)}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ c_0 +6\biggl[ \frac{ (2c_0^2 + 25c_0 + 77) -(2c_0^2 + 19c_0 +44) }{ (2c_0^2 + 13c_0 + 20) - 2(2c_0^2 + 19c_0 + 44) + (2c_0^2 + 25c_0 + 77)}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ c_0 +6 \biggl[ \frac{ 6c_0 +33 }{ 9}\biggr] </math> |
|
|
<math>~=</math> |
<math>~ 5c_0 + 22 \, . </math> |
It is gratifying — although, somewhat surprising (to me!) — to find that this precisely matches the above-defined, desired boundary condition for the case of <math>~\ell = 2</math>.
Duh!
After also checking conformance with the expected boundary condition in the case of analytic eigenfunctions having <math>~\ell = 3</math> and, separately (not shown), for numerically generated eigenfunctions having a wide range of oscillation frequencies, it dawned on us that the "desired" surface boundary condition may actually be a natural outcome of the envelope's LAWE.
By constraining our discussion to models for which <math>~g^2 = \mathcal{B}</math> and <math>~\mathcal{D} = q^3</math>, the envelope's LAWE is,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \biggl[ 1 - q^3 \xi^3\biggr] \frac{d^2x}{d\xi^2} + \biggl\{ 3 - 6q^3 \xi^3 \biggr\} \frac{1}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ q^3 \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]\frac{x}{\xi^2} \, . </math> |
At the surface <math>~(\xi = 1/q)</math>, the coefficient of the second derivative term goes to zero, in which case the LAWE reduces in form to,
|
<math>~0</math> |
<math>~=</math> |
<math>~ -\frac{3}{\xi} \cdot \frac{dx}{d\xi} + \biggl[ \mathfrak{F}_\mathrm{env} -\alpha_e \biggr]\frac{x}{\xi^2} </math> |
|
<math>~\Rightarrow ~~~ 3\cdot \frac{d\ln x}{d\ln \xi}\biggr|_\mathrm{surface} </math> |
<math>~=</math> |
<math>~ \mathfrak{F}_\mathrm{env} -\alpha_e \, . </math> |
And this is precisely the condition that derives from the astrophysically reasonable boundary condition that we have discussed separately and that has been reviewed, above.
Broader Analysis
Let's, then, examine the behavior of the envelope's LAWE at the surface in the most general case — that is, when not constrained to <math>~g^2 = \mathcal{B}</math>. First, we note that,
|
<math>~g^2 - \mathcal{B}</math> |
<math>~=</math> |
<math> \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \biggl( 1-q \biggr) + \frac{\rho_e}{\rho_c} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] -2\biggl(\frac{\rho_e}{\rho_c}\biggr) + 3\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 </math> |
|
|
<math>~=</math> |
<math> \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl\{ 2 \biggl[1 - \biggl(\frac{\rho_e}{\rho_c} \biggr) -q + q \biggl(\frac{\rho_e}{\rho_c} \biggr)\biggr] + \biggl( \frac{\rho_e}{\rho_c} \biggr)\frac{1}{q^2} -2 + 2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr\} </math> |
|
|
<math>~=</math> |
<math> \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ - 2q + 2q \biggl(\frac{\rho_e}{\rho_c} \biggr) + \biggl( \frac{\rho_e}{\rho_c} \biggr)\frac{1}{q^2} \biggr] </math> |
|
|
<math>~=</math> |
<math> \frac{1}{q^2} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] \, . </math> |
Hence, at the surface quite generally, the coefficient of the second derivative is,
|
<math>~\frac{1}{\mathcal{A}}\biggl[\mathcal{A} + (g^2 - \mathcal{B})\xi - \mathcal{A}\mathcal{D} \xi^3 \biggr]_{\xi=1/q}</math> |
<math>~=</math> |
<math>~ \frac{1}{\mathcal{A}}\biggl\{ 2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(1-\frac{\rho_e}{\rho_c}\biggr) + \frac{1}{q^3} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] - \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{\mathcal{A}} \biggl(\frac{\rho_e}{\rho_c}\biggr)\biggl\{ - 2\biggl(\frac{\rho_e}{\rho_c} -1\biggr) + \frac{1}{q^3} \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] - \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ 0 \, . </math> |
And, at the surface quite generally, the coefficient of the first derivative is,
|
<math>~\frac{1}{\mathcal{A}}\biggl[3\mathcal{A} + 4(g^2 - \mathcal{B})\xi - 6\mathcal{A}\mathcal{D} \xi^3 \biggr]_{\xi=1/q}</math> |
<math>~=</math> |
<math>~ \frac{1}{\mathcal{A}}\biggl\{ 6\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl(1-\frac{\rho_e}{\rho_c}\biggr) + \frac{4}{q^3} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] - \frac{6}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr)^2 \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{2}{\mathcal{A}} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl\{ -3\biggl(\frac{\rho_e}{\rho_c} - 1\biggr) + \frac{2}{q^3} \biggl[ \biggl( \frac{\rho_e}{\rho_c} \biggr) + 2q^3 \biggl(\frac{\rho_e}{\rho_c} - 1 \biggr) \biggr] - \frac{3}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{2}{\mathcal{A}} \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl(\frac{\rho_e}{\rho_c} - 1\biggr) - \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr] \, . </math> |
Hence, at the surface quite generally, the envelope's LAWE becomes,
|
<math>~ - \biggl[ 3\mathcal{A} + 4(g^2-\mathcal{B}) \xi - 6\mathcal{A} \mathcal{D} \xi^3 \biggr]_{\xi=1/q} \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} </math> |
<math>~=</math> |
<math>~ \mathcal{A}\biggl[ \mathcal{D} \mathfrak{F}_\mathrm{env} \xi^3 -\alpha_e \biggr]_{\xi=1/q} </math> |
|
<math>~\Rightarrow~~~ - 2\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[ \biggl(\frac{\rho_e}{\rho_c} - 1\biggr) - \frac{1}{q^3}\biggl(\frac{\rho_e}{\rho_c}\biggr) \biggr] \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} </math> |
<math>~=</math> |
<math>~ \biggl(\frac{\rho_e}{\rho_c}\biggr)^2 \mathfrak{F}_\mathrm{env} \cdot \frac{1}{q^3} - 2\alpha_e \biggl(\frac{\rho_e}{\rho_c}\biggr)\biggl(1- \frac{\rho_e}{\rho_c}\biggr) </math> |
|
<math>~\Rightarrow~~~ \biggl[2\biggl(\frac{\rho_e}{\rho_c}\biggr) + 2q^3\biggl(1 - \frac{\rho_e}{\rho_c} \biggr) \biggr] \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} </math> |
<math>~=</math> |
<math>~ \biggl(\frac{\rho_e}{\rho_c}\biggr) \mathfrak{F}_\mathrm{env} - 2q^3 \alpha_e \biggl(1- \frac{\rho_e}{\rho_c}\biggr) </math> |
|
<math>~\Rightarrow~~~ \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} </math> |
<math>~=</math> |
<math>~\biggl[2 + 2q^3\biggl(1 - \frac{\rho_e}{\rho_c} \biggr)\biggl(\frac{\rho_e}{\rho_c}\biggr)^{-1} \biggr] ^{-1} \biggl[ \mathfrak{F}_\mathrm{env} - 2q^3 \alpha_e \biggl(1- \frac{\rho_e}{\rho_c}\biggr)\biggl(\frac{\rho_e}{\rho_c}\biggr)^{-1} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{\mathfrak{F}_\mathrm{env} - \Kappa \alpha_e}{2+\Kappa} \, , </math> |
where,
<math>~\Kappa \equiv 2q^3\biggl(1 - \frac{\rho_e}{\rho_c} \biggr)\biggl(\frac{\rho_e}{\rho_c}\biggr)^{-1} \, .</math>
Notice that in the special case for which we have been able to identify analytically specifiable eigenvectors, namely, when
<math>~g^2 = \mathcal{B} </math> <math>~\Rightarrow</math> <math>~\Kappa = 1 \, ,</math>
this surface boundary condition simplifies to the expected expression,
|
<math>~ \frac{d\ln x}{d\ln\xi} \biggr|_\mathrm{surface} </math> |
<math>~=</math> |
<math>~\frac{1}{3} \biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \biggr] \, . </math> |
Under what condition — other than when <math>~g^2=\mathcal{B}</math> — does the general expression generate the expected expression? We need,
|
<math>~\frac{1}{3} \biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \biggr] </math> |
<math>~=</math> |
<math>~\biggl[2 + \Kappa \biggr] ^{-1} \biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \Kappa \biggr] </math> |
|
<math>~\Rightarrow ~~~ (2 + \Kappa ) \biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \biggr] </math> |
<math>~=</math> |
<math>~ 3\biggl[ \mathfrak{F}_\mathrm{env} - \alpha_e \Kappa \biggr] </math> |
|
<math>~\Rightarrow ~~~ (2 + \Kappa ) \mathfrak{F}_\mathrm{env} - (2 + \Kappa ) \alpha_e </math> |
<math>~=</math> |
<math>~ 3\mathfrak{F}_\mathrm{env} - 3\Kappa \alpha_e </math> |
|
<math>~\Rightarrow ~~~ (\Kappa -1) \mathfrak{F}_\mathrm{env} </math> |
<math>~=</math> |
<math>~ -2(\Kappa-1 ) \alpha_e </math> |
|
<math>~\Rightarrow ~~~ \mathfrak{F}_\mathrm{env} </math> |
<math>~=</math> |
<math>~ -2\alpha_e \, . </math> |
But, given that,
|
<math>~\mathfrak{F}_\mathrm{env}</math> |
<math>~\equiv</math> |
<math>~\frac{3\omega^2_\mathrm{env}}{2\pi G \gamma_e \rho_e} - 2\alpha_e \, , </math> |
we see that the expected boundary condition will result only for <math>~\omega_\mathrm{env}^2 = 0</math>, that is, only for, <math>~\sigma_c^2 = 0</math>. This is what we have been noticing as we have played with numerically generated eigenvectors: When integrating from the center of the zero-zero bipolytrope, to its surface, the naturally resulting (first) derivative of the eigenfunction at the surface of the configuration matches the expected surface boundary condition …
- for all values of <math>\sigma_c^2</math>, when <math>~g^2= \mathcal{B}</math>, that is, when <math>~\Kappa=1</math>;
- only for <math>~\sigma_c^2 = 0</math> in all other configurations, that is, for all <math>~\Kappa \ne 1</math>.
What do we make of this?
Five Mode Summary
| Model | <math>~\ell</math> | <math>~j</math> | <math>~q</math> | <math>~\gamma_e</math> | <math>~\gamma_c</math> | <math>~\nu</math> | <math>~\frac{\rho_e}{\rho_c}</math> | <math>~\alpha_e</math> | <math>~\alpha_c</math> | <math>~n_e</math> | <math>~n_c</math> | <math>~g^2</math> | <math>~f</math> | <math>~\sigma_\mathfrak{G}^2</math> | Analytic <math>~\sigma_c^2</math> |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Analytic21 | 2 | 1 | 0.684 | 1.194 | 1.846 | 0.547 | 0.390 | -0.35 | +0.833 | 5.15 | 1.18 | 1.324 | 2.542 | +0.761 | 28.9116 |
| Analytic22 | 2 | 2 | 0.887 | 1.799 | 1.022 | 0.799 | 0.583 | +0.776 | -0.914 | 1.25 | 46 | 1.146 | 1.444 | -0.878 | 34.9155 |
| Analytic31 | 3 | 1 | 0.406 | 1.180 | 1.009 | 0.378 | 0.118 | -0.390 | -0.964 | 5.56 | 111 | 1.194 | 3.568 | -0.180 | 12.1770 |
| Analytic32 | 3 | 2 | 0.812 | 2.327 | 4.216 | 0.690 | 0.517 | +1.281 | +2.051 | 0.754 | 0.311 | 1.232 | 1.813 | +9.654 | 169.0733 |
| <math>~\sigma_c^2</math> Determined from Numerical Integration by Enforcing Boundary Condition (B.C.) |
|||||
| Model | <math>~\ell=0</math> B.C.: <math>~c_0</math> |
<math>~\ell=1</math> B.C.: <math>~(3c_0+8)</math> |
<math>~\ell=2</math> B.C.: <math>~(5c_0+22)</math> |
<math>~\ell=3</math> B.C.: <math>~7(c_0+6)</math> |
<math>~\ell=4</math> B.C.: <math>~(9c_0+68)</math> |
|---|---|---|---|---|---|
| Analytic21 | -0.76017962 | +9.881793 | +28.9116 | +56.32919 | - |
| Analytic22 | -4.890477 | +5.5864115 | +34.91550 | +83.09678 | - |
Broad Application
Here's one key lesson that can be drawn from our analytically specified oscillation modes. As has been documented above, the quantum number, <math>~j</math>, associated with the eigenvector of the core is related to the oscillation frequency,
|
<math>~\sigma_c^2</math> |
<math>~\equiv</math> |
<math>~\frac{3\omega^2}{2\pi G \rho_c} \, ,</math> |
via the expression,
|
<math>~\frac{\sigma_c^2}{\gamma_c} - 2\alpha_c</math> |
<math>~=</math> |
<math>~ 2j(2j+5) </math> |
|
<math>~\Rightarrow~~~\sigma_c^2</math> |
<math>~=</math> |
<math>~\frac{8[\alpha_c + j(2j+5)]}{3-\alpha_c} \, .</math> |
Also, as documented above, the quantum number, <math>~\ell</math>, associated with the eigenvector of the envelope is related to the oscillation frequency via the expression,
|
<math>~\frac{\sigma_c^2}{\gamma_e(\rho_e/\rho_c)} - 2\alpha_e</math> |
<math>~=</math> |
<math>~(c_0 + 3\ell)(c_0 + 3\ell + 5) </math> |
|
<math>~\Rightarrow~~~\sigma_c^2</math> |
<math>~=</math> |
<math>~\gamma_e \biggl(\frac{\rho_e}{\rho_c}\biggr) \biggl[2\alpha_e + (c_0 + 3\ell)(c_0 + 3\ell + 5) \biggr]</math> |
|
|
<math>~=</math> |
<math>~\biggl(\frac{\rho_e}{\rho_c}\biggr) \frac{4[2c_0^2 + 4c_0 + c_0^2 + c_0(6\ell+5) + 3\ell(3\ell+5) ]}{3-\alpha_e}</math> |
|
|
<math>~=</math> |
<math>~\biggl(\frac{\rho_e}{\rho_c}\biggr) \frac{12[c_0^2 + c_0(2\ell+3) + \ell(3\ell+5) ]}{3-\alpha_e} \, ,</math> |
where, as a reminder, <math>~c_0 = [-1 \pm \sqrt{1+\alpha_e}]</math>. Eliminating <math>~\sigma_c^2</math> between these two relations gives,
|
Case A
Consider, first, the astrophysical system in which <math>~(n_c, n_e) = (5, 1)</math>. Given that, in general, <math>~\alpha = (3-n)/(1+n)</math>, we therefore will be considering the system in which, <math>~(\alpha_c, \alpha_e) = (-\tfrac{1}{3}, 1) </math>. For this system, we should be able to analytically specify eigenvectors having the properties specified in the following table.
|
Analytically Specifiable Eigenvectors for which |
|||
| <math>~\frac{\rho_e}{\rho_c}</math> | |||
| <math>~\ell</math> | <math>~c_0</math> |
<math>~j=1</math> |
<math>~j=2</math> |
| 1 | <math>~-1 + \sqrt{2}</math> | 0.26034953 | n/a <math>~(q > 1)</math> |
| <math>~-1 - \sqrt{2}</math> | 1.52 (n/a) | (n/a) | |
| 2 | <math>~-1 + \sqrt{2}</math> | 0.10636430 | 0.28186540 |
| <math>~-1 - \sqrt{2}</math> | 0.24400066 | 0.64660175 | |
| 3 | <math>~-1 + \sqrt{2}</math> | 0.05809795 | 0.15395957 |
| <math>~-1 - \sqrt{2}</math> | 0.10216916 | 0.27074827 | |
Related Discussions
- Searching for Additional, Analytically Specified Eigenvectors of Zero-Zero Bipolytropes
- Numerically Determined Eigenvectors

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |