Difference between revisions of "User:Tohline/SSC/FreeEnergy/PowerPoint"
(→Case M: Finished (re)deriving x) |
(→Case M Equilibrium Conditions: Add link back to discussion of Lane-Emden equation) |
||
| (43 intermediate revisions by the same user not shown) | |||
| Line 235: | Line 235: | ||
=====Virial Equilibrium===== | =====Virial Equilibrium===== | ||
<table align="right" border="0" cellpadding="5" width="360px"> | |||
<tr><th align="center"><font size="+1">Figure 1</font></th></tr> | |||
<tr><td align="center"> | |||
[[File:PvsR_simplest.png|350px|Simplest Case M sequence]] | |||
</td></tr></table> | |||
So the statement of virial equilibrium becomes, | So the statement of virial equilibrium becomes, | ||
<div align="center"> | <div align="center"> | ||
| Line 293: | Line 298: | ||
</table> | </table> | ||
</div> | </div> | ||
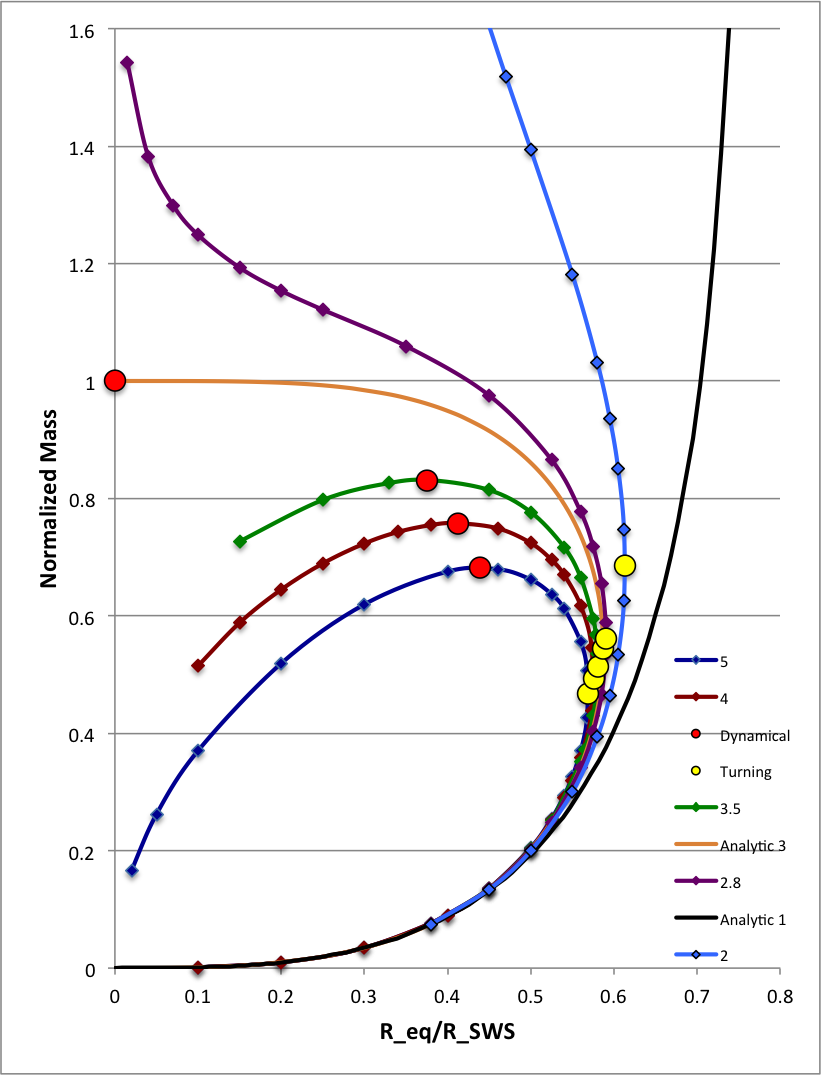
The light-blue dots in Figure 1 trace the equilibrium sequence that is defined by this virial equilibrium function in the case of <math>~n = 5</math>. | |||
=====Dynamical Instability===== | =====Dynamical Instability===== | ||
Along the "Case M" equilibrium sequence, the transition from stable to unstable configurations occurs at, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 361: | Line 368: | ||
</table> | </table> | ||
</div> | </div> | ||
The location of this critical configuration along the <math>~n=5</math> equilibrium sequence is marked by the red circular dot in Figure 1. | |||
=====Turning Point===== | =====Turning Point===== | ||
| Line 392: | Line 401: | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{3}{ 5\pi x^{5} } | <math>~\frac{3}{ 5\pi x^{5} } | ||
+ \frac{3}{4\pi}\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggl[ \frac{(n-3)}{n } - 4\biggr] x^{(3 | + \frac{3}{4\pi}\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggl[ \frac{(n-3)}{n } - 4\biggr] \frac{x^{(n-3)/n } }{x^5} | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 406: | Line 415: | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{3}{ 5\pi x^{5} } | <math>~\frac{3}{ 5\pi x^{5} } | ||
- 3\biggl(\frac{3}{4\pi} \biggr)^{(n+1)/n} \biggl[ \frac{n+1}{n } \biggr] x^{(3 | - 3\biggl(\frac{3}{4\pi} \biggr)^{(n+1)/n} \biggl[ \frac{n+1}{n } \biggr] \frac{x^{(n-3)/n } }{x^5} \, . | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 419: | Line 428: | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~ | ||
[ x_\mathrm{eq}^{(3 | [ x_\mathrm{eq}^{(n-3)/n } ]_\mathrm{turn} | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 443: | Line 452: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl[ \frac{4n}{ 15(n+1)}\biggl(\frac{4\pi}{3} \biggr)^{1/n} \biggr]^{n/(3-n)} | \biggl[ \frac{4n}{ 15(n+1)}\biggl(\frac{4\pi}{3} \biggr)^{1/n} \biggr]^{n/(n-3)} \, . | ||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
And, returning to the virial equilibrium expression, we find that, associated with this equilibrium radius, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \frac{P_\mathrm{max}}{P_\mathrm{norm}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{3}{2^2\cdot 5\pi x^{4}_\mathrm{turn} }\biggl[ 5\biggl(\frac{3}{4\pi} \biggr)^{1/n} x^{(n-3)/n }_\mathrm{turn} | |||
- 1\biggr] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \Rightarrow ~~~2^2\cdot 5\pi x^{4}_\mathrm{turn} \biggl(\frac{P_\mathrm{max}}{P_\mathrm{norm}}\biggr)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~15\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggl[ \frac{4n}{ 15(n+1)}\biggl(\frac{4\pi}{3} \biggr)^{1/n} \biggr] - 3 </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl(\frac{n-3}{ n+1} \biggr) </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \Rightarrow ~~~\frac{P_\mathrm{max}}{P_\mathrm{norm}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{20\pi}\biggl(\frac{n-3}{ n+1} \biggr) \biggl[ \frac{ 15(n+1)}{4n}\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggr]^{4n/(n-3)} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Notice that, under the assumption that all three structural filling-factors are unity, <math>~[x_\mathrm{eq}]_\mathrm{turn} = [x_\mathrm{eq}]_\mathrm{crit}</math>, that is, the location of the turning point coincides precisely with the point along the equilibrium sequence where the transition from stable to unstable equilibrium configurations occurs (marked by the red circular dot in Figure 1). | |||
=====Case M Summary===== | |||
<div align="center"> | |||
<table border="1" align="center" cellpadding="8"> | |||
<tr><th align="center" colspan="2"> | |||
<font size="+1">Case M</font> | |||
</th></tr> | |||
<tr><td align="center" colspan="2"> | |||
Order-of-Magnitude Analysis: Assume <math>~{\tilde\mathfrak{f}}_M = {\tilde\mathfrak{f}}_W = {\tilde\mathfrak{f}}_A = 1</math> | |||
</td></tr> | |||
<tr> | |||
<td align="center"> | |||
Virial Equilibrium: | |||
</td> | |||
<td align="center"> | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \frac{P_e}{P_\mathrm{norm}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{3}{2^2\cdot 5\pi }\biggl(\frac{R_\mathrm{eq}}{R_\mathrm{norm}}\biggr)^{-4} | |||
\biggl[ 5\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggl(\frac{R_\mathrm{eq}}{R_\mathrm{norm}}\biggr)^{(n-3)/n }_\mathrm{eq} | |||
- 1\biggr] </math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<p>Dynamical Instability:</p> | |||
<math>~(n>3)</math> | |||
</td> | |||
<td align="center"> | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr|_\mathrm{crit}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ | |||
\frac{4n}{3\cdot 5(n+1)} \biggl(\frac{4\pi}{3} \biggr)^{1/n}\biggr]^{n/(n-3)} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<p>Turning Point <math>~(P_\mathrm{max} )</math>:</p> | |||
<math>~(n>3)</math> | |||
</td> | |||
<td align="center"> | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{P_\mathrm{max}}{P_\mathrm{norm}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{20\pi}\biggl(\frac{n-3}{ n+1} \biggr) \biggl[ \frac{ 15(n+1)}{4n}\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggr]^{4n/(n-3)} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr|_\mathrm{turn} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{4n}{ 3\cdot 5(n+1)}\biggl(\frac{4\pi}{3} \biggr)^{1/n} \biggr]^{n/(n-3)} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
====Case P==== | |||
Set <math>~K</math> and <math>~P_e</math> constant and examine how the free-energy behaves as a function of the coordinates, <math>~(R,M_\mathrm{tot})</math>. In this case (see, for example, [[User:Tohline/SSC/FreeEnergy/PolytropesEmbedded#Case_P|here]]), | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~a</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{3}{5} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~b</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~n\biggl(\frac{4\pi}{3} \biggr)^{-1/n} \frac{\tilde{\mathfrak{f}}_A}{\tilde{\mathfrak{f}}_M^{(n+1)/n}} | |||
\biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} | |||
\biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{3(n-1)/[n(n-3)]} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~c</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{4\pi}{3} \biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{(5-n)/(n-3)} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~R_0 = R_\mathrm{SWS} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl( \frac{n+1}{n} \biggr)^{1/2} G^{-1/2} K_n^{n/(n+1)} P_\mathrm{e}^{(1-n)/[2(n+1)]} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~M_\mathrm{SWS} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{n+1}{n} \biggr)^{3/2} G^{-3/2} K_n^{2n/(n+1)} P_\mathrm{e}^{(3-n)/[2(n+1)]} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
where the structural form factors for pressure-truncated polytropes are precisely defined [[User:Tohline/SSC/Virial/FormFactors#PTtable|here]]. If we set all three structural form-factors to unity, we have, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{a}{3c}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{b}{nc}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)\biggr]^{(n+1)/n} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
=====Virial Equilibrium===== | |||
So, the statement of virial equilibrium becomes, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)\biggr]^{(n+1)/n} x^{(n-3)/n }_\mathrm{eq} | |||
- \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} - x^{4}_\mathrm{eq} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 0 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<div align="center"> | |||
<math>~\xi_1</math> | |||
</div> | |||
<div align="center"> | |||
<table border="1" cellpadding="8" align="center"> | |||
<tr> | |||
<td align="center" colspan="4"> | |||
Known Analytic Lane-Emden Functions | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~n</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\theta_n(\xi)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~-\theta^'_n</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\xi_1</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~1-\frac{\xi^2}{6}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\frac{\xi}{3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\sqrt{6}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~1</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\frac{\sin\xi}{\xi}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\frac{\sin\xi}{\xi^2} - \frac{\cos\xi}{\xi}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\pi</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<math>~5</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\biggl[ 1 + \frac{\xi^2}{3} \biggr]^{-1/ 2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\frac{\xi}{3}\biggl[ 1 + \frac{\xi^2}{3} \biggr]^{-3/ 2} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~\infty</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
=====Dynamical Instability===== | |||
Along the "Case P" equilibrium sequence, the transition from stable to unstable configurations occurs at, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~[x_\mathrm{eq}]^4_\mathrm{crit} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(n-3)}{3(n+1)} \biggl[ \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(n-3)}{20\pi n} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} | |||
= \frac{2^2\pi (n-3)}{3^2\cdot 5 n} \biggl[\frac{3}{4\pi}\biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)\biggr]^{2} | |||
\, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
which, in combination with the "Case P" virial equilibrium expression gives, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ 0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl\{ \biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{1/2} x_\mathrm{crit}^2 \biggr\}^{(n+1)/n} x^{(n-3)/n }_\mathrm{crit} | |||
- \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl\{ \biggl[\frac{20\pi n}{(n-3)}\biggr] x^4_\mathrm{crit} \biggr\} - x^{4}_\mathrm{crit} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{(n+1)/(2n)} x^{(3n-1)/n }_\mathrm{crit} | |||
- x^4_\mathrm{crit} \biggl\{ \biggl[\frac{3(n+1)}{(n-3)}\biggr] +1 \biggr\} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{(n+1)/(2n)} x^{(3n-1)/n }_\mathrm{crit} | |||
- \biggl[\frac{4n}{(n-3)}\biggr] x^4_\mathrm{crit} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ x^{(n+1)/n}_\mathrm{crit} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[\frac{(n-3)}{4n}\biggr] \biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{(n+1)/(2n)} | |||
</math> | |||
</td> | |||
</tr> | |||
<!-- | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ [x_\mathrm{eq}]_\mathrm{crit} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~3\cdot 2^{-(3n+1)/(n+1)} \biggl( \frac{5}{\pi} \biggr)^{1/2} \biggl[\frac{(n-3)}{n}\biggr]^{(n-1)/[2(n+1)]} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
--> | |||
</table> | |||
</div> | |||
=====Turning Points===== | |||
Let's simplify the notation, defining, | |||
<div align="center"> | |||
<math>~m \equiv \frac{3}{4\pi}\biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr) \, .</math> | |||
</div> | |||
The statement of virial equilibrium becomes, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~m^{(n+1)/n} x^{(n-3)/n } | |||
- c_0m^2 - x^{4} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 0 \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
where, | |||
<div align="center"> | |||
<math>~c_0 \equiv \biggl[ \frac{4\pi(n+1)}{15n} \biggr] \, .</math> | |||
</div> | |||
Differentiating gives, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ 0 </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl(\frac{n+1}{n}\biggr)m^{1/n} x^{(n-3)/n } dm | |||
+ \biggl(\frac{n-3}{n}\biggr)m^{(n+1)/n} x^{-3/n } dx | |||
- 2c_0m dm - 4x^{3} dx | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ (n+1)m^{1/n} x^{(n-3)/n } - 2c_0 n m \biggr] dm | |||
+ \biggl[ (n-3) m^{(n+1)/n} x^{-3/n } - 4nx^{3} \biggr] dx | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~\frac{dm}{dx}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{4nx^{3} - (n-3) m^{(n+1)/n} x^{-3/n } }{(n+1)m^{1/n} x^{(n-3)/n } - 2c_0 n m} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
---- | |||
One turning point occurs where the numerator is zero, that is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~4nx^{3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(n-3) m^{(n+1)/n} x^{-3/n } </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ 4nx^{3(n+1)/n}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(n-3) m^{(n+1)/n} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ \frac{m}{x^3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[\frac{4n}{(n-3)}\biggr]^{n/(n+1)} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Plugging this into the virial equilibrium expression gives, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ 0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl\{ \biggl[\frac{4n}{(n-3)}\biggr]^{n/(n+1)} x^3 \biggr\}^{(n+1)/n} x^{(n-3)/n } | |||
- c_0\biggl\{ \biggl[\frac{4n}{(n-3)}\biggr]^{n/(n+1)} x^3 \biggr\}^2 - x^{4} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[\frac{4n}{(n-3)}\biggr] x^4 | |||
- c_0 \biggl[\frac{4n}{(n-3)}\biggr]^{2n/(n+1)} x^6 - x^{4} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ c_0 \biggl[\frac{4n}{(n-3)}\biggr]^{2n/(n+1)} x^2 </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[\frac{4n}{(n-3)}\biggr] - 1 </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ x^2 </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{15n}{4\pi(n+1)} \biggr]\biggl[\frac{3(n+1)}{(n-3)}\biggr] \biggl[\frac{(n-3)}{4n}\biggr]^{2n/(n+1)} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{3^2\cdot 5 n}{4\pi(n-3)} \biggr]\biggl[\frac{(n-3)}{4n}\biggr]^{2n/(n+1)} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
The associated mass is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>\biggl( \frac{M_\mathrm{max}}{M_\mathrm{SWS}}\biggr) = \biggl(\frac{4\pi}{3} \biggr)m</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{4\pi}{3} \biggl[\frac{n-3}{4n}\biggr]^{-n/(n+1)} | |||
\biggl[ \frac{3^2\cdot 5 n}{4\pi(n-3)} \biggr]^{3/2}\biggl[\frac{(n-3)}{4n}\biggr]^{3n/(n+1)} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{4\pi}{3} \biggl[\frac{n-3}{4n}\biggr]^{2n/(n+1)} | |||
\biggl[ \frac{3^2\cdot 5 n}{4\pi(n-3)} \biggr]^{3/2} | |||
\, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Notice that, for <math>~n=3</math>, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>\biggl( \frac{M_\mathrm{max}}{M_\mathrm{SWS}}\biggr)_{n=3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[\frac{3^4\cdot 5^3 }{2^{8} \pi} \biggr]^{1/2} | |||
\, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
---- | |||
Another turning point occurs where the denominator is zero, that is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~(n+1)m^{1/n} x^{(n-3)/n } </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~2c_0 n m</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ (n+1)x^{(n-3)/n } </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~2c_0 n m^{(n-1)/n}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ \frac{x^{n-3}}{ m^{n-1}} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{2c_0 n}{(n+1)} \biggr]^n</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{8\pi}{15} \biggr]^n</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ m</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ x^{(n-3)/(n-1)} \biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)}\biggr] \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Plugging this into the virial equilibrium expression gives, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ x^{4} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ x^{(n-3)/(n-1)} \biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)}\biggr]^{(n+1)/n} x^{(n-3)/n } | |||
- c_0\biggl[ x^{(n-3)/(n-1)} \biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)}\biggr]^2 </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{(n-3)(n+1)/[n(n-1)]} \cdot x^{(n-3)/n } | |||
- c_0 \biggl( \frac{15}{8\pi} \biggr)^{2n/(n-1)} x^{2(n-3)/(n-1)} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{2(n-3)/(n-1)} | |||
- \biggl[ \frac{(n+1)}{2n} \biggr]\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{2(n-3)/(n-1)} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{2(n-3)/(n-1)} \biggl[1 | |||
- \frac{(n+1)}{2n} \biggr] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ x^{2(n+1)/(n-1)}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \frac{(n-1)}{2n} \biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ \frac{R_\mathrm{max}}{R_\mathrm{SWS}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \biggl[\frac{(n-1)}{2n} \biggr]^{(n-1)/[2(n+1)]} \biggl( \frac{15}{8\pi} \biggr)^{1/2} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
And the associated mass is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr|_\mathrm{turn}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{4\pi}{3}\biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)} | |||
\biggl\{ \biggl[\frac{(n-1)}{2n} \biggr]^{(n-1)/[2(n+1)]} \biggl( \frac{15}{8\pi} \biggr)^{1/2} \biggr\}^{(n-3)/(n-1)} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{4\pi}{3}\biggl( \frac{15}{8\pi} \biggr)^{3/2} \biggl[\frac{(n-1)}{2n} \biggr]^{(n-3)/[2(n+1)]} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
=====Case P Summary===== | |||
<div align="center"> | |||
<table border="1" align="center" cellpadding="8"> | |||
<tr><th align="center" colspan="2"> | |||
<font size="+1">Case P</font> | |||
</th></tr> | |||
<tr><td align="center" colspan="2"> | |||
Order-of-Magnitude Analysis: Assume <math>~{\tilde\mathfrak{f}}_M = {\tilde\mathfrak{f}}_W = {\tilde\mathfrak{f}}_A = 1</math> | |||
</td></tr> | |||
<tr> | |||
<td align="center"> | |||
Virial Equilibrium: | |||
</td> | |||
<td align="center"> | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)\biggr]^{(n+1)/n} | |||
\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{SWS}} \biggr)^{(n-3)/n } | |||
- \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} | |||
- \biggl( \frac{R_\mathrm{eq}}{R_\mathrm{SWS}} \biggr)^{4} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 0 </math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<p>Dynamical Instability:</p> | |||
<math>~(n > 3)</math> | |||
</td> | |||
<td align="center"> | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{R_\mathrm{eq}}{R_\mathrm{SWS}} \biggr|_\mathrm{crit}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[\frac{(n-3)}{4n}\biggr]^{n/(n+1)} \biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{1/2} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<p>Turning Point <math>~(M_\mathrm{max} )</math>:</p> | |||
<math>~(n>3)</math> | |||
</td> | |||
<td align="center"> | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>\frac{M_\mathrm{max}}{M_\mathrm{SWS}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{4\pi}{3} \biggl[\frac{n-3}{4n}\biggr]^{2n/(n+1)} | |||
\biggl[ \frac{3^2\cdot 5 n}{4\pi(n-3)} \biggr]^{3/2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\frac{R_\mathrm{eq}}{R_\mathrm{SWS}} \biggr|_\mathrm{turn} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[\frac{(n-3)}{4n}\biggr]^{n/(n+1)} \biggl[ \frac{3^2\cdot 5 n}{2^2\pi(n-3)} \biggr]^{1/2} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<p>Turning Point <math>~(R_\mathrm{max} )</math>:</p> | |||
<math>~(n>1)</math> | |||
</td> | |||
<td align="center"> | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \frac{R_\mathrm{max}}{R_\mathrm{SWS}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \biggl[\frac{(n-1)}{2n} \biggr]^{(n-1)/[2(n+1)]} \biggl( \frac{15}{8\pi} \biggr)^{1/2} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr|_\mathrm{turn}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{4\pi}{3}\biggl( \frac{15}{8\pi} \biggr)^{3/2} \biggl[\frac{(n-1)}{2n} \biggr]^{(n-3)/[2(n+1)]} </math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<div align="left"> | |||
<table border="2" cellpadding="5" align="center"> | |||
<tr><th align="center" colspan="2"><font size="+1">Figure 2</font></th></tr> | |||
<tr> | |||
<td align="center">[[File:Stahler1983TitlePage0.png|350px|center|Stahler (1983) Title Page]]</td> | |||
<td align="center" rowspan="2"> | |||
[[File:MimicStahlerPlot3.png|center|350px|Case P equilibrium sequences with key configurations highlighted]] | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
[[File:Stahler_MRdiagram1.png|350px|center|Stahler (1983) Figure 17 (edited)]] | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
The right-hand panel of Figure 2 presents substantial segments of ''Case P'' virial equilibrium sequences for a range of polytropic indexes (n = 1, 2, 2.8, 3, 3.5, 4, 5). For each sequence, the location of the <math>~R_\mathrm{max}</math> and <math>~M_\mathrm{max}</math> turning points — if they exist — are denoted by a yellow or red circular dot, respectively. The point along each <math>~(n \geq 3)</math> sequence at which the transition from dynamically stable to dynamically unstable structures occurs coincides with the location of <math>~M_\mathrm{max}</math> (''i.e.,'' with the red circular dot). | |||
For display purposes, all normalized masses <math>~(M_\mathrm{tot}/M_\mathrm{SWS})</math> have been further normalized to the maximum mass on the n = 3 sequence. | |||
</div> | |||
====Detailed Force-Balance Models==== | |||
=====Structural Form Factors===== | |||
The following table of structural form factors has been drawn from [[User:Tohline/SSC/Virial/FormFactors#PTtable|here]], | |||
<div align="center" id="PTtable"> | |||
<table border="1" align="center" cellpadding="5"> | |||
<tr> | |||
<th align="center" colspan="1"> | |||
Structural Form Factors for <font color="red">Isolated</font> Polytropes | |||
</th> | |||
<th align="center" colspan="1"> | |||
Structural Form Factors for <font color="red">Pressure-Truncated</font> Polytropes | |||
</th> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{f}_M</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \biggl[ - \frac{3\theta^'}{\xi} \biggr]_{\xi_1} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>\mathfrak{f}_W </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{3^2\cdot 5}{5-n} \biggl[ \frac{\theta^'}{\xi} \biggr]^2_{\xi_1} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>\mathfrak{f}_A </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math> | |||
\frac{3(n+1) }{(5-n)} ~\biggl[ \theta^' \biggr]^2_{\xi_1} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td> | |||
<td align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\tilde\mathfrak{f}_M</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \biggl( - \frac{3\tilde\theta^'}{\tilde\xi} \biggr) </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>\tilde\mathfrak{f}_W</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>\frac{3\cdot 5}{(5-n)\tilde\xi^2} | |||
\biggl[\tilde\theta^{n+1} + 3 (\tilde\theta^')^2 - \tilde\mathfrak{f}_M \tilde\theta \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\tilde\mathfrak{f}_A | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{(5-n)} \biggl\{ 6\tilde\theta^{n+1} + (n+1) | |||
\biggl[3 (\tilde\theta^')^2 - \tilde\mathfrak{f}_M \tilde\theta \biggr] \biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
and [[User:Tohline/SSC/Virial/FormFactors#Summary_.28n.3D5.29|here]], | |||
<div align="center"> | |||
<table border="1" align="center" cellpadding="10"> | |||
<tr><th align="center"> | |||
Structural Form Factors for <font color="red">Pressure-Truncated</font> n = 5 Polytropes | |||
</th></tr> | |||
<tr><td align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\tilde\mathfrak{f}_M</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
( 1 + \ell^2 )^{-3/2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\tilde\mathfrak{f}_W</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{5}{2^4} \cdot \ell^{-5} | |||
\biggl[ \ell \biggl( \ell^4 - \frac{8}{3}\ell^2 - 1 \biggr)(1 + \ell^2)^{-3} + \tan^{-1}(\ell ) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\tilde\mathfrak{f}_A</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{3}{2^3} \ell^{-3} [ \tan^{-1}(\ell ) + \ell (\ell^2-1) (1+\ell^2)^{-2} ] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="left" colspan="3"> | |||
where, <math>~\ell \equiv \frac{\tilde\xi}{\sqrt{3}}</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td></tr> | |||
</table> | |||
</div> | |||
=====Case M Equilibrium Conditions===== | |||
Employing the [[User:Tohline/SphericallySymmetricConfigurations/Virial#Choices_Made_by_Other_Researchers|renormalization factors]], | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{R_\mathrm{Horedt}}{R_\mathrm{norm}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{4\pi}{(n+1)^n}\biggr]^{1/(n-3)} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{P_\mathrm{Horedt}}{P_\mathrm{norm}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{(n+1)^3}{4\pi}\biggr]^{(n+1)/(n-3)} \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
we find from detailed force-balance analyses that the [[User:Tohline/SSC/Structure/PolytropesEmbedded#General_Properties|equilibrium radius and corresponding external pressure]] for "Case M" configurations are, | |||
<div align="center"> | |||
<table border="0" cellpadding="3"> | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
~\frac{R_\mathrm{eq}}{R_\mathrm{norm}} = \frac{R_\mathrm{eq}}{R_\mathrm{Horedt}} \cdot \frac{R_\mathrm{Horedt}}{R_\mathrm{norm}} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{4\pi}{(n+1)^n}\biggr]^{1/(n-3)} | |||
\tilde\xi ( -\tilde\xi^2 \tilde\theta' )^{(1-n)/(n-3)} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
which matches the expression derived in an [[User:Tohline/SSC/Structure/Polytropes#Lane-Emden_Equation|ASIDE box found with our introduction of the Lane-Emden equation]], and | |||
<div align="center"> | |||
<table border="0" cellpadding="3"> | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
~\frac{P_\mathrm{e}}{P_\mathrm{norm}} = \frac{P_\mathrm{e}}{P_\mathrm{Horedt}} \cdot \frac{P_\mathrm{Horedt}}{P_\mathrm{norm}} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{(n+1)^3}{4\pi}\biggr]^{(n+1)/(n-3)} | |||
\tilde\theta_n^{n+1}( -\tilde\xi^2 \tilde\theta' )^{2(n+1)/(n-3)} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
<!-- Next two equations are in a form suitable for the powerpoint presentation | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
~\frac{R_\mathrm{eq}}{R_\mathrm{norm}} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{4\pi}{(n+1)^n}\biggr]^{1/(n-3)} | |||
\tilde\xi ( -\tilde\xi^2 \tilde\theta' )^{(1-n)/(n-3)} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
~\frac{P_\mathrm{e}}{P_\mathrm{norm}} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{(n+1)^3}{4\pi}\biggr]^{(n+1)/(n-3)} | |||
\tilde\theta_n^{n+1}( -\tilde\xi^2 \tilde\theta' )^{2(n+1)/(n-3)} | |||
</math> | |||
</td> | |||
</tr> | |||
--> | |||
</table> | |||
</div> | |||
There are two turning points: One associated with a maximum in <math>~P_e</math> and one associated with a maximum in <math>~R_\mathrm{eq}</math>. According to [[User:Tohline/SSC/Structure/PolytropesEmbedded#Turning_Points|Kimura's discussion]], the first of these occurs in the configuration for which, | |||
<div align="center"> | |||
<table border="0" align="center" cellpadding="5"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\tilde\theta^{n+1}}{(\tilde\theta^')^2} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(n-3)}{2} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
… | |||
</td> | |||
<td align="left"> | |||
For n = 5, this occurs when <math>~\tilde\xi = 3</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
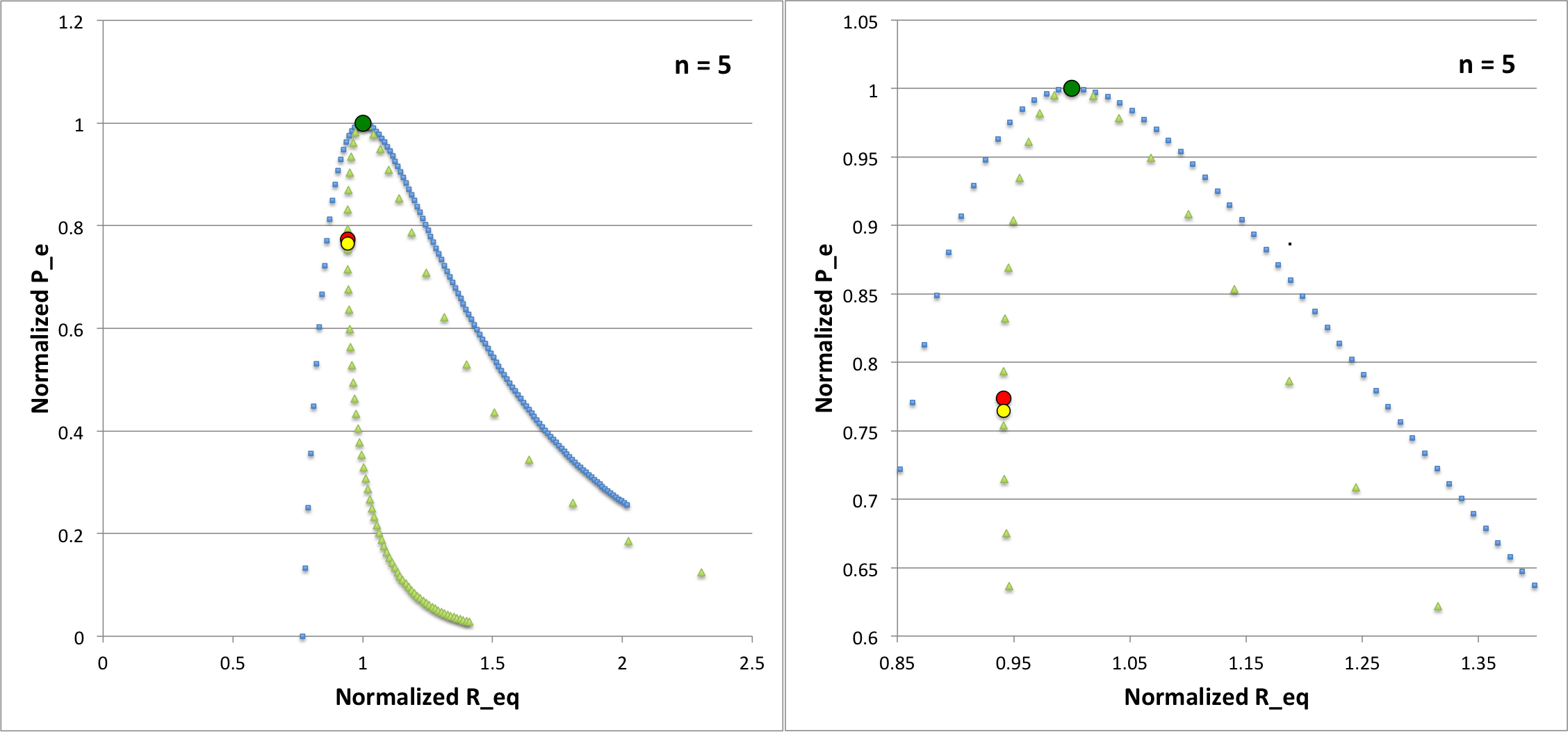
This point along the equilibrium sequence is identified by the dark green circular dot in Figure 3, below. The second occurs in the configuration for which, | |||
<div align="center"> | |||
<table border="0" align="center" cellpadding="5"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\tilde\xi \tilde\theta^{n}}{(-\tilde\theta^')} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(n-3)}{(n-1)} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
… | |||
</td> | |||
<td align="left"> | |||
For n = 5, this occurs when <math>~\tilde\xi = \sqrt{15} \approx 3.87298</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
This point along the equilibrium sequence is identified by the yellow circular dot in Figure 3, below. In addition, we have identified the point of dynamical instability. | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{(n-3)}{3^2(n+1)} \cdot \frac{a}{c} = \frac{1}{3^3} \cdot \frac{3}{2^2\pi} \biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)^{-1} \frac{3}{5} \cdot \frac{\tilde\mathfrak{f}_W}{\tilde\mathfrak{f}_M^2}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{1}{2^2\cdot 3 \cdot 5\pi} \biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)^{-1} \frac{5}{2^4} | |||
(1+\ell^2)^3 \cdot \ell^{-5} \biggl[ \ell \biggl( \ell^4 - \frac{8}{3} \ell^2 -1\biggr) (1+\ell^2)^{-3} + \tan^{-1}\ell \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ \biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{1}{2^6\cdot 3 \cdot \pi \ell^5} | |||
\biggl[ \ell \biggl( \ell^4 - \frac{8}{3} \ell^2 -1\biggr) + (1+\ell^2)^3 \tan^{-1}\ell \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
But the equilibrium condition for n = 5 configurations is, | |||
<div align="center"> | |||
<table border="0" cellpadding="3"> | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
~\biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4 | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{(n+1)^3}{4\pi}\biggr]^{(n+1)/(n-3)} | |||
\tilde\theta_n^{n+1}( -\tilde\xi^2 \tilde\theta' )^{2(n+1)/(n-3)} | |||
\biggl\{ \biggl[ \frac{4\pi}{(n+1)^n}\biggr]^{1/(n-3)} | |||
\tilde\xi ( -\tilde\xi^2 \tilde\theta' )^{(1-n)/(n-3)} \biggr\}^4 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{2 \cdot 3^3}{\pi}\biggr]^{3} | |||
\tilde\theta_n^{6}\tilde\xi^{12}( - \tilde\theta' )^{6} | |||
\biggl\{ \frac{1}{6}\biggl[ \frac{\pi}{2\cdot 3^3}\biggr]^{1/2} | |||
\tilde\xi^{-3} ( - \tilde\theta' )^{-2} \biggr\}^4 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{2^4\cdot 3^4} | |||
\biggl[ \frac{2 \cdot 3^3}{\pi}\biggr] | |||
\tilde\theta_n^{6} ( - \tilde\theta' )^{-2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{2^3\cdot 3\pi} | |||
(1+\ell^2)^{-3} \biggl[\frac{\ell}{3^{1/ 2}} (1+\ell^2)^{-3/2} \biggr]^{-2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{2^3\pi} \cdot \ell^{-2} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Putting the two expressions together gives, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{1}{2^3\pi} \cdot \ell^{-2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{1}{2^6\cdot 3 \cdot \pi \ell^5} | |||
\biggl[ \ell \biggl( \ell^4 - \frac{8}{3} \ell^2 -1\biggr) + (1+\ell^2)^3 \tan^{-1}\ell \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~2^3\cdot 3 \cdot \ell^{3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\ell^5 - \frac{8}{3}\ell^3 - \ell + (1+\ell^2)^3 \tan^{-1}\ell | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~\biggl[\frac{(1+\ell^2)^3}{\ell}\biggr] \tan^{-1}\ell </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
1 + \biggl[ \frac{2^4\cdot 5}{3} \biggr] \ell^{2} - \ell^4 | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<!-- Next equation in format suitable for powerpoint presentation | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[\frac{(1+\ell^2)^3}{\ell}\biggr] \tan^{-1}\ell </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
1 + \biggl[ \frac{2^4\cdot 5}{3} \biggr] \ell^{2} - \ell^4 | |||
</math> | |||
</td> | |||
<td align="center"> | |||
… | |||
</td> | |||
<td align="left"> | |||
For n = 5, this occurs when <math>~\tilde\xi \approx 3.85065</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
--> | |||
This agrees with the [[User:Tohline/SSC/FreeEnergy/PolytropesEmbedded#Case_M|expression derived in a separate <font color="red">ASIDE</font>]]; as was pointed out in that context, the root of this equation is: <math>~\ell \approx 2.223175</math>, that is, <math>~\tilde\xi \approx 3.85065</math>. This point along the equilibrium sequence is identified by the red circular dot in Figure 3, below. It is almost — but definitely not — coincident with the configuration along the sequence (marked by the yellow circular dot) that is associated with the minimum-radius turning point. | |||
<div align="center" id="Figure3"> | |||
<table border="0" align="center"><tr><th align="center"> | |||
Figure 3: Case M Equilibrium Sequence | |||
</th></tr> | |||
<tr><td align="center"> | |||
[[File:CaseMcompareTogether.png|700px|center|Case M pressure-truncated n=5 polytrope]] | |||
</td></tr> | |||
</table> | |||
</div> | |||
Now for a movie! | |||
<div align="center"> | |||
<table border="1" align="center"> | |||
<tr> | |||
<td align="center"> | |||
[[File:CaseMfreeEnergy50.gif|750px|Case M movie of free-energy structures]] | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
=====Case P Equilibrium Conditions===== | |||
The [[User:Tohline/SSC/Structure/PolytropesEmbedded#Stahler.27s_Presentation|equilibrium radius and corresponding configuration mass]] from a "Case P" analysis are, | |||
<div align="center"> | |||
<table border="0" cellpadding="3"> | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
~\frac{R_\mathrm{eq}}{R_\mathrm{SWS} } | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math> | |||
\biggl( \frac{n}{4\pi} \biggr)^{1/2} \tilde\xi {\tilde\theta}_n^{(n-1)/2} \, , | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
~\frac{M_\mathrm{tot}}{M_\mathrm{SWS} } | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=~</math> | |||
</td> | |||
<td align="left"> | |||
<math> | |||
\biggl( \frac{n^3}{4\pi} \biggr)^{1/2} {\tilde\theta}_n^{(n-3)/2} (-\tilde\xi^2 \tilde\theta^') \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
According to our [[User:Tohline/SSC/Structure/PolytropesEmbedded#Other_Limits|review of, especially, Kimura's work]], the turning point associated with <math>~M_\mathrm{max}</math> occurs where, | |||
<div align="center"> | |||
<table border="0" align="center" cellpadding="5"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\tilde\theta^{n+1}}{(\tilde\theta^')^2} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{(n-3)}{2} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
… | |||
</td> | |||
<td align="left"> | |||
For n = 5, this occurs when <math>~\tilde\xi = 3 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
And a turning point associated with <math>~R_\mathrm{max}</math> occurs where, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\tilde\xi (-\tilde\theta^')}{\tilde\theta}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{2}{(n-1)} \, .</math> | |||
</td> | |||
</tr> | |||
<!-- Next expression for PowerPoint presentation | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{\tilde\xi (-\tilde\theta^')}{\tilde\theta}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{2}{(n-1)} </math> | |||
</td> | |||
<td align="center"> | |||
… | |||
</td> | |||
<td align="left"> | |||
For n = 5, this occurs when <math>~\tilde\xi = \sqrt{3} </math> | |||
</td> | |||
</tr> | |||
--> | |||
</table> | |||
</div> | |||
For <math>~n=5</math> configurations, this means, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{1}{2} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\tilde\xi \biggl( 1 + \frac{\tilde\xi^2}{3}\biggr)^{1/ 2} \frac{\tilde\xi}{3} \biggl( 1 + \frac{\tilde\xi^2}{3}\biggr)^{-3/ 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{\ell^2}{(1+\ell^2)} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ (1+\ell^2)</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
2\ell^2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ \ell^2</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
1 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ \tilde\xi</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\sqrt{3} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
===Five-One Bipolytropes=== | |||
====Basic Properties==== | |||
For bipolytropes, in general, let <math>~n =n_c</math> and <math>~j = n_e</math>. The statement of virial equilibrium is, then, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{ b}{n_c c}\cdot x^{(n_c-3)/n_c }_\mathrm{eq} - \frac{a}{3c} + \frac{1}{n_e}\cdot x^{(n_e-3)/n_e}_\mathrm{eq} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 0 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
And the critical equilibrium configuration has, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~[x_\mathrm{eq}^{(n_e-3)/n_e}]_\mathrm{crit} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{a}{3^2c}\biggl[ \frac{n_e^2(n_c-3)}{n_c-n_e} \biggr] | |||
\, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Here we choose to set <math>~n_c = 5</math> and <math>~n_e = 1</math>. Hence, these two conditions become, respectively, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{ b}{5 c}\cdot x^{2/5 }_\mathrm{eq} + x^{-2}_\mathrm{eq} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \frac{a}{3c} \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
and, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~[x_\mathrm{eq}]_\mathrm{crit} </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{2\cdot 3^2c}{a} \biggr]^{1/2} | |||
\, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<!-- EQUATIONS USEFUL in POWERPOINT PRESENTATION | |||
<div align="center"> | |||
<math>~\theta_5(\xi) ~~~~\theta_1(\eta)</math> | |||
<math>~\mu_e/\mu_c</math> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\ell_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{\xi_i}{\sqrt{3}}</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
--> | |||
Comparing the general free-energy expression at the beginning of this chapter with the free-energy expression provided via our [[User:Tohline/SSC/FreeEnergy/PolytropesEmbedded#BiPolytropeFreeEnergy|accompanying summary discussion of five-one bipolytropes]], we find that, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~a</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(3\mathfrak{L}_i +12\mathfrak{K}_i) x_\mathrm{eq} | |||
\biggl[ \biggl( \frac{3^6}{2^5\pi} \biggr)^{1/2} \frac{\ell_i^3}{(1+\ell_i^2)^3} \biggr] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~b</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(5 \mathfrak{L}_i) x_\mathrm{eq}^{3/5} \biggl[ \biggl( \frac{3^6}{2^5\pi} \biggr)^{1/2} \frac{\ell_i^3}{(1+\ell_i^2)^3} \biggr] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~c</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~(4\mathfrak{K}_i) x_\mathrm{eq}^{3} \biggl[ \biggl( \frac{3^6}{2^5\pi} \biggr)^{1/2} \frac{\ell_i^3}{(1+\ell_i^2)^3} \biggr] </math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
where, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{L}_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{(\ell_i^4-1)}{\ell_i^2} + \frac{(1+\ell_i^2)^3}{\ell_i^3} \cdot \tan^{-1}\ell_i \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{K}_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{\Lambda_i}{\eta_i} + \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Lambda_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{\eta_i} - \ell_i \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\eta_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~3 \biggl( \frac{\mu_e}{\mu_c} \biggr) \biggl[\frac{\ell_i }{(1+\ell_i^2)}\biggr] \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
and, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~x_\mathrm{eq} = \frac{R_\mathrm{eq}}{R_\mathrm{norm}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{(1+\ell_i^2)^3}{3^3\ell_i^3} \biggl[ \frac{1 }{(1+\Lambda_i^2)} \biggr] | |||
\biggl\{ 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \biggr\}^{-1}</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<!-- MORE EXPRESSIONS FOR POWERPOINT PRESENTATION | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~x_\mathrm{eq}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{(1+\ell_i^2)^3}{3^3\ell_i^3} \biggl[ \frac{1 }{(1+\Lambda_i^2)} \biggr] | |||
\biggl\{ 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \biggr\}^{-1}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Lambda_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{\eta_i} - \ell_i </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\eta_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~3 \biggl( \frac{\mu_e}{\mu_c} \biggr) \biggl[\frac{\ell_i }{(1+\ell_i^2)}\biggr] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~R_0 = R_\mathrm{norm}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl( \frac{G}{K_c} \biggr)^{5/2} M_\mathrm{tot}^2 | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 449: | Line 2,574: | ||
</table> | </table> | ||
</div> | </div> | ||
--> | |||
In rewriting this last expression, we have made use of the two relations, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~q \equiv \frac{r_i}{R}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl\{ 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \biggr\}^{-1} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{\ell_i q}{(1+\Lambda_i^2)^{1/2}} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
Consistent with our [[User:Tohline/SSC/BipolytropeGeneralization#Free_Energy_and_Its_Derivatives|generic discussion of the stability of bipolytropes]] and the ''specific'' discussion of [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Stability_Condition|the stability of bipolytropes having]] <math>~(n_c, n_e) = (5, 1)</math>, it can straightforwardly be shown that <math>~\partial \mathfrak{G}/\partial \chi = 0</math> is satisfied by setting <math>~\Chi = 1</math>; that is, the equilibrium condition is, | |||
Furthermore, the equilibrium configuration is unstable whenever <math>~\partial^2 \mathfrak{G}/\partial \chi^2 < 0</math>, that is, the transition from stable to unstable configurations whenever, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{ \mathfrak{L}_i}{\mathfrak{K}_i}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~20 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
[[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Stability_Condition|Table 1 of an accompanying chapter]] — and the red-dashed curve in the figure adjacent to that table — identifies some key properties of the model that marks the transition from stable to unstable configurations along equilibrium sequences that have various values of the mean-molecular weight ratio, <math>~\mu_e/\mu_c</math>. | |||
====Coincidence Between Points of Secular and Dynamical Instability==== | |||
From the [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Model_Sequences|accompanying graphical display of equilibrium sequences]], it seems that a <math>~\nu_\mathrm{max}</math> turning point will only exist in five-one bipolytropes for <math>~\mu_e/\mu_c</math> less than some value — call it, <math>~(\mu_e/\mu_c)_\mathrm{begin}</math> — which is less than but approximately equal to <math>~\tfrac{1}{3}</math>. As we move along any sequence for which <math>~\mu_e/\mu_c < (\mu_e/\mu_c)_\mathrm{begin}</math>, in the direction of increasing <math>~\ell_i</math>, it is fair to ask whether the system becomes dynamically unstable (at <math>~[x_\mathrm{eq}]_\mathrm{crit}</math>) before or after it encounters the point of secular instability marked by <math>~\nu_\mathrm{max}</math>. | |||
{{LSU_HBook_footer}} | {{LSU_HBook_footer}} | ||
Latest revision as of 15:42, 7 September 2016
Supporting Derivations for Free-Energy PowerPoint Presentation
The derivations presented here are an extension of our accompanying free-energy synopsis. These additional details proved to be helpful while developing an overarching PowerPoint presentation.

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
General Free-Energy Expression
We're considering a free-energy function of the following form:
|
<math>~\mathfrak{G}^*_\mathrm{type}</math> |
<math>~=</math> |
<math>~-ax^{-1} + b x^{-3/n} + c x^{-3/j} + \mathfrak{G}_0 \, ,</math> |
where,
<math>~x \equiv \frac{R}{R_0} \, .</math>
As we have shown, setting,
|
<math>~\frac{\partial \mathfrak{G}^*_\mathrm{type}}{\partial x}</math> |
<math>~=</math> |
<math>~0 \, ,</math> |
generates a mathematical statement of virial equilibrium, namely,
|
<math>~\frac{ b}{nc}\cdot x^{(n-3)/n }_\mathrm{eq} - \frac{a}{3c} + \frac{1}{j}\cdot x^{(j-3)/j}_\mathrm{eq} </math> |
<math>~=</math> |
<math>~ 0 \, .</math> |
And equilibrium configurations for which the second (as well as first) derivative of the free energy is zero are found at "critical" radii given by the expression,
|
<math>~[x_\mathrm{eq}^{(j-3)/j}]_\mathrm{crit} </math> |
<math>~=</math> |
<math>~ \frac{a}{3^2c}\biggl[ \frac{j^2(n-3)}{n-j} \biggr] \, . </math> |
Pressure-Truncated Polytropes
For pressure-truncated polytropes, set <math>~j=-1</math> and let <math>~n</math> be the chosen polytropic index. In this case, the statement of virial equilibrium is,
|
<math>~\frac{ b}{nc}\cdot x^{(n-3)/n }_\mathrm{eq} - \frac{a}{3c} - x^{4}_\mathrm{eq} </math> |
<math>~=</math> |
<math>~ 0 \, ;</math> |
and the critical equilibrium configuration has,
|
<math>~[x_\mathrm{eq}]_\mathrm{crit} </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{a(n-3)}{3^2c (n+1)}\biggr]^{1/4} \, . </math> |
Case M
Set <math>~K</math> and <math>~M_\mathrm{tot}</math> constant and examine how the free-energy behaves as a function of the coordinates, <math>~(R,P_e)</math>. In this case (see, for example, here),
|
<math>~a</math> |
<math>~\equiv</math> |
<math>~\frac{3}{5} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2}\, , </math> |
|
<math>~b</math> |
<math>~\equiv</math> |
<math>~n\biggl(\frac{4\pi}{3} \biggr)^{-1/n} \frac{\tilde{\mathfrak{f}}_A}{\tilde{\mathfrak{f}}_M^{(n+1)/n}} \, , </math> |
|
<math>~c</math> |
<math>~\equiv</math> |
<math>~\frac{4\pi}{3}\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr) \, , </math> |
where the structural form factors for pressure-truncated polytropes are precisely defined here. And (see, for example, here),
|
<math>~R_0 = R_\mathrm{norm}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \biggl( \frac{G}{K} \biggr)^n M_\mathrm{tot}^{n-1} \biggr]^{1/(n-3)} \, ,</math> |
|
<math>~P_\mathrm{norm}</math> |
<math>~\equiv</math> |
<math>~\biggl[ \frac{K^{4n}}{G^{3(n+1)} M_\mathrm{tot}^{2(n+1)}} \biggr]^{1/(n-3)} \, .</math> |
If we set all three structural form-factors to unity, we have,
|
<math>~\frac{a}{3c}</math> |
<math>~=</math> |
<math>~\frac{3}{2^2\cdot 5\pi}\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)^{-1} \, ,</math> |
|
<math>~\frac{b}{nc}</math> |
<math>~=</math> |
<math>~\biggl(\frac{3}{4\pi} \biggr)^{(n+1)/n} \biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)^{-1} \, .</math> |
Virial Equilibrium
| Figure 1 |
|---|
So the statement of virial equilibrium becomes,
|
<math>~ x^{4}_\mathrm{eq} </math> |
<math>~=</math> |
<math>~\biggl[ \biggl(\frac{3}{4\pi} \biggr)^{(n+1)/n} x^{(n-3)/n }_\mathrm{eq} - \frac{3}{2^2\cdot 5\pi}\biggr]\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)^{-1} </math> |
|
|
<math>~=</math> |
<math>~\frac{3}{2^2\cdot 5\pi}\biggl[ 5\biggl(\frac{3}{4\pi} \biggr)^{1/n} x^{(n-3)/n }_\mathrm{eq} - 1\biggr]\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)^{-1} </math> |
|
<math>~ \Rightarrow ~~~ \frac{P_e}{P_\mathrm{norm}}</math> |
<math>~=</math> |
<math>~\frac{3}{2^2\cdot 5\pi x^{4}_\mathrm{eq} }\biggl[ 5\biggl(\frac{3}{4\pi} \biggr)^{1/n} x^{(n-3)/n }_\mathrm{eq} - 1\biggr] \, . </math> |
The light-blue dots in Figure 1 trace the equilibrium sequence that is defined by this virial equilibrium function in the case of <math>~n = 5</math>.
Dynamical Instability
Along the "Case M" equilibrium sequence, the transition from stable to unstable configurations occurs at,
|
<math>~[x_\mathrm{eq}]^4_\mathrm{crit} </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{(n-3)}{3(n+1)}\biggr] \frac{3}{2^2\cdot 5\pi}\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)^{-1} </math> |
|
<math>~\Rightarrow ~~~ \frac{2^2\cdot 5\pi}{3}\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)[x_\mathrm{eq}]^4_\mathrm{crit} </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{(n-3)}{3(n+1)}\biggr] </math> |
which, in combination with the virial equilibrium condition gives,
|
<math>~5\biggl(\frac{3}{4\pi} \biggr)^{1/n} [x_\mathrm{eq}]^{(n-3)/n }_\mathrm{crit} -1</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{(n-3)}{3(n+1)}\biggr] </math> |
|
<math>~\Rightarrow~~~ [x_\mathrm{eq}]_\mathrm{crit} </math> |
<math>~=</math> |
<math>~\biggl[ \frac{4n}{3\cdot 5(n+1)} \biggl(\frac{4\pi}{3} \biggr)^{1/n}\biggr]^{n/(n-3)} \, . </math> |
The location of this critical configuration along the <math>~n=5</math> equilibrium sequence is marked by the red circular dot in Figure 1.
Turning Point
Let's examine the curvature of the equilibrium sequence.
|
<math>~ \frac{d}{dx}\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)</math> |
<math>~=</math> |
<math>~ - \frac{3}{ 5\pi x^{5} }\biggl[ 5\biggl(\frac{3}{4\pi} \biggr)^{1/n} x^{(n-3)/n } - 1\biggr] + \frac{3(n-3)}{2^2n \pi x^{4} }\biggl(\frac{3}{4\pi} \biggr)^{1/n} x^{-3/n } </math> |
|
|
<math>~=</math> |
<math>~\frac{3}{ 5\pi x^{5} } + \frac{3}{4\pi}\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggl[ \frac{(n-3)}{n } - 4\biggr] \frac{x^{(n-3)/n } }{x^5} </math> |
|
|
<math>~=</math> |
<math>~\frac{3}{ 5\pi x^{5} } - 3\biggl(\frac{3}{4\pi} \biggr)^{(n+1)/n} \biggl[ \frac{n+1}{n } \biggr] \frac{x^{(n-3)/n } }{x^5} \, . </math> |
Setting this derivative to zero let's us identify the location of the turning point that identifies <math>~P_\mathrm{max}.</math>
|
<math>~ [ x_\mathrm{eq}^{(n-3)/n } ]_\mathrm{turn} </math> |
<math>~=</math> |
<math>~ \frac{1}{ 5\pi }\biggl[ \frac{n }{n+1} \biggr] \biggl(\frac{4\pi}{3} \biggr)^{(n+1)/n} </math> |
|
<math>~\Rightarrow~~~ [ x_\mathrm{eq} ]_\mathrm{turn} </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{4n}{ 15(n+1)}\biggl(\frac{4\pi}{3} \biggr)^{1/n} \biggr]^{n/(n-3)} \, . </math> |
And, returning to the virial equilibrium expression, we find that, associated with this equilibrium radius,
|
<math>~ \frac{P_\mathrm{max}}{P_\mathrm{norm}}</math> |
<math>~=</math> |
<math>~\frac{3}{2^2\cdot 5\pi x^{4}_\mathrm{turn} }\biggl[ 5\biggl(\frac{3}{4\pi} \biggr)^{1/n} x^{(n-3)/n }_\mathrm{turn} - 1\biggr] </math> |
|
<math>~ \Rightarrow ~~~2^2\cdot 5\pi x^{4}_\mathrm{turn} \biggl(\frac{P_\mathrm{max}}{P_\mathrm{norm}}\biggr)</math> |
<math>~=</math> |
<math>~15\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggl[ \frac{4n}{ 15(n+1)}\biggl(\frac{4\pi}{3} \biggr)^{1/n} \biggr] - 3 </math> |
|
|
<math>~=</math> |
<math>~\biggl(\frac{n-3}{ n+1} \biggr) </math> |
|
<math>~ \Rightarrow ~~~\frac{P_\mathrm{max}}{P_\mathrm{norm}}</math> |
<math>~=</math> |
<math>~\frac{1}{20\pi}\biggl(\frac{n-3}{ n+1} \biggr) \biggl[ \frac{ 15(n+1)}{4n}\biggl(\frac{3}{4\pi} \biggr)^{1/n} \biggr]^{4n/(n-3)} \, .</math> |
Notice that, under the assumption that all three structural filling-factors are unity, <math>~[x_\mathrm{eq}]_\mathrm{turn} = [x_\mathrm{eq}]_\mathrm{crit}</math>, that is, the location of the turning point coincides precisely with the point along the equilibrium sequence where the transition from stable to unstable equilibrium configurations occurs (marked by the red circular dot in Figure 1).
Case M Summary
|
Case M | |||||||
|---|---|---|---|---|---|---|---|
|
Order-of-Magnitude Analysis: Assume <math>~{\tilde\mathfrak{f}}_M = {\tilde\mathfrak{f}}_W = {\tilde\mathfrak{f}}_A = 1</math> | |||||||
|
Virial Equilibrium: |
|
||||||
|
Dynamical Instability: <math>~(n>3)</math> |
|
||||||
|
Turning Point <math>~(P_\mathrm{max} )</math>: <math>~(n>3)</math> |
|
||||||
Case P
Set <math>~K</math> and <math>~P_e</math> constant and examine how the free-energy behaves as a function of the coordinates, <math>~(R,M_\mathrm{tot})</math>. In this case (see, for example, here),
|
<math>~a</math> |
<math>~=</math> |
<math>~\frac{3}{5} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \, , </math> |
|
<math>~b</math> |
<math>~=</math> |
<math>~n\biggl(\frac{4\pi}{3} \biggr)^{-1/n} \frac{\tilde{\mathfrak{f}}_A}{\tilde{\mathfrak{f}}_M^{(n+1)/n}} \biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{3(n-1)/[n(n-3)]} \, , </math> |
|
<math>~c</math> |
<math>~=</math> |
<math>~\frac{4\pi}{3} \biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{(5-n)/(n-3)} \, , </math> |
|
<math>~R_0 = R_\mathrm{SWS} </math> |
<math>~\equiv</math> |
<math>~\biggl( \frac{n+1}{n} \biggr)^{1/2} G^{-1/2} K_n^{n/(n+1)} P_\mathrm{e}^{(1-n)/[2(n+1)]} \, ,</math> |
|
<math>~M_\mathrm{SWS} </math> |
<math>~\equiv</math> |
<math>~ \biggl( \frac{n+1}{n} \biggr)^{3/2} G^{-3/2} K_n^{2n/(n+1)} P_\mathrm{e}^{(3-n)/[2(n+1)]} \, .</math> |
where the structural form factors for pressure-truncated polytropes are precisely defined here. If we set all three structural form-factors to unity, we have,
|
<math>~\frac{a}{3c}</math> |
<math>~=</math> |
<math>~ \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} </math> |
|
<math>~\frac{b}{nc}</math> |
<math>~=</math> |
<math>~ \biggl[ \biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)\biggr]^{(n+1)/n} </math> |
Virial Equilibrium
So, the statement of virial equilibrium becomes,
|
<math>~\biggl[ \biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)\biggr]^{(n+1)/n} x^{(n-3)/n }_\mathrm{eq} - \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} - x^{4}_\mathrm{eq} </math> |
<math>~=</math> |
<math>~ 0 \, .</math> |
<math>~\xi_1</math>
|
Known Analytic Lane-Emden Functions |
|||
|
<math>~n</math> |
<math>~\theta_n(\xi)</math> |
<math>~-\theta^'_n</math> |
<math>~\xi_1</math> |
|
<math>~0</math> |
<math>~1-\frac{\xi^2}{6}</math> |
<math>~\frac{\xi}{3}</math> |
<math>~\sqrt{6}</math> |
|
<math>~1</math> |
<math>~\frac{\sin\xi}{\xi}</math> |
<math>~\frac{\sin\xi}{\xi^2} - \frac{\cos\xi}{\xi}</math> |
<math>~\pi</math> |
|
<math>~5</math> |
<math>~\biggl[ 1 + \frac{\xi^2}{3} \biggr]^{-1/ 2}</math> |
<math>~\frac{\xi}{3}\biggl[ 1 + \frac{\xi^2}{3} \biggr]^{-3/ 2} </math> |
<math>~\infty</math> |
Dynamical Instability
Along the "Case P" equilibrium sequence, the transition from stable to unstable configurations occurs at,
|
<math>~[x_\mathrm{eq}]^4_\mathrm{crit} </math> |
<math>~=</math> |
<math>~ \frac{(n-3)}{3(n+1)} \biggl[ \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{(n-3)}{20\pi n} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} = \frac{2^2\pi (n-3)}{3^2\cdot 5 n} \biggl[\frac{3}{4\pi}\biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)\biggr]^{2} \, , </math> |
which, in combination with the "Case P" virial equilibrium expression gives,
|
<math>~ 0</math> |
<math>~=</math> |
<math>~\biggl\{ \biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{1/2} x_\mathrm{crit}^2 \biggr\}^{(n+1)/n} x^{(n-3)/n }_\mathrm{crit} - \frac{3}{20\pi} \biggl( \frac{n+1}{n} \biggr) \biggl\{ \biggl[\frac{20\pi n}{(n-3)}\biggr] x^4_\mathrm{crit} \biggr\} - x^{4}_\mathrm{crit} </math> |
|
|
<math>~=</math> |
<math>~\biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{(n+1)/(2n)} x^{(3n-1)/n }_\mathrm{crit} - x^4_\mathrm{crit} \biggl\{ \biggl[\frac{3(n+1)}{(n-3)}\biggr] +1 \biggr\} </math> |
|
|
<math>~=</math> |
<math>~\biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{(n+1)/(2n)} x^{(3n-1)/n }_\mathrm{crit} - \biggl[\frac{4n}{(n-3)}\biggr] x^4_\mathrm{crit} </math> |
|
<math>~\Rightarrow~~~ x^{(n+1)/n}_\mathrm{crit} </math> |
<math>~=</math> |
<math>~\biggl[\frac{(n-3)}{4n}\biggr] \biggl[ \frac{3^2\cdot 5 n}{2^2\pi (n-3)} \biggr]^{(n+1)/(2n)} </math> |
Turning Points
Let's simplify the notation, defining,
<math>~m \equiv \frac{3}{4\pi}\biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr) \, .</math>
The statement of virial equilibrium becomes,
|
<math>~m^{(n+1)/n} x^{(n-3)/n } - c_0m^2 - x^{4} </math> |
<math>~=</math> |
<math>~ 0 \, ,</math> |
where,
<math>~c_0 \equiv \biggl[ \frac{4\pi(n+1)}{15n} \biggr] \, .</math>
Differentiating gives,
|
<math>~ 0 </math> |
<math>~=</math> |
<math>~\biggl(\frac{n+1}{n}\biggr)m^{1/n} x^{(n-3)/n } dm + \biggl(\frac{n-3}{n}\biggr)m^{(n+1)/n} x^{-3/n } dx - 2c_0m dm - 4x^{3} dx </math> |
|
|
<math>~=</math> |
<math>~\biggl[ (n+1)m^{1/n} x^{(n-3)/n } - 2c_0 n m \biggr] dm + \biggl[ (n-3) m^{(n+1)/n} x^{-3/n } - 4nx^{3} \biggr] dx </math> |
|
<math>~\Rightarrow ~~~\frac{dm}{dx}</math> |
<math>~=</math> |
<math>~ \frac{4nx^{3} - (n-3) m^{(n+1)/n} x^{-3/n } }{(n+1)m^{1/n} x^{(n-3)/n } - 2c_0 n m} \, . </math> |
One turning point occurs where the numerator is zero, that is,
|
<math>~4nx^{3}</math> |
<math>~=</math> |
<math>~(n-3) m^{(n+1)/n} x^{-3/n } </math> |
|
<math>~\Rightarrow ~~~ 4nx^{3(n+1)/n}</math> |
<math>~=</math> |
<math>~(n-3) m^{(n+1)/n} </math> |
|
<math>~\Rightarrow ~~~ \frac{m}{x^3}</math> |
<math>~=</math> |
<math>~\biggl[\frac{4n}{(n-3)}\biggr]^{n/(n+1)} \, .</math> |
Plugging this into the virial equilibrium expression gives,
|
<math>~ 0</math> |
<math>~=</math> |
<math>~\biggl\{ \biggl[\frac{4n}{(n-3)}\biggr]^{n/(n+1)} x^3 \biggr\}^{(n+1)/n} x^{(n-3)/n } - c_0\biggl\{ \biggl[\frac{4n}{(n-3)}\biggr]^{n/(n+1)} x^3 \biggr\}^2 - x^{4} </math> |
|
|
<math>~=</math> |
<math>~\biggl[\frac{4n}{(n-3)}\biggr] x^4 - c_0 \biggl[\frac{4n}{(n-3)}\biggr]^{2n/(n+1)} x^6 - x^{4} </math> |
|
<math>~\Rightarrow~~~ c_0 \biggl[\frac{4n}{(n-3)}\biggr]^{2n/(n+1)} x^2 </math> |
<math>~=</math> |
<math>~\biggl[\frac{4n}{(n-3)}\biggr] - 1 </math> |
|
<math>~\Rightarrow~~~ x^2 </math> |
<math>~=</math> |
<math>~\biggl[ \frac{15n}{4\pi(n+1)} \biggr]\biggl[\frac{3(n+1)}{(n-3)}\biggr] \biggl[\frac{(n-3)}{4n}\biggr]^{2n/(n+1)} </math> |
|
|
<math>~=</math> |
<math>~\biggl[ \frac{3^2\cdot 5 n}{4\pi(n-3)} \biggr]\biggl[\frac{(n-3)}{4n}\biggr]^{2n/(n+1)} \, .</math> |
The associated mass is,
|
<math>\biggl( \frac{M_\mathrm{max}}{M_\mathrm{SWS}}\biggr) = \biggl(\frac{4\pi}{3} \biggr)m</math> |
<math>~=</math> |
<math>~ \frac{4\pi}{3} \biggl[\frac{n-3}{4n}\biggr]^{-n/(n+1)} \biggl[ \frac{3^2\cdot 5 n}{4\pi(n-3)} \biggr]^{3/2}\biggl[\frac{(n-3)}{4n}\biggr]^{3n/(n+1)} </math> |
|
|
<math>~=</math> |
<math>~ \frac{4\pi}{3} \biggl[\frac{n-3}{4n}\biggr]^{2n/(n+1)} \biggl[ \frac{3^2\cdot 5 n}{4\pi(n-3)} \biggr]^{3/2} \, .</math> |
Notice that, for <math>~n=3</math>,
|
<math>\biggl( \frac{M_\mathrm{max}}{M_\mathrm{SWS}}\biggr)_{n=3}</math> |
<math>~=</math> |
<math>~ \biggl[\frac{3^4\cdot 5^3 }{2^{8} \pi} \biggr]^{1/2} \, .</math> |
Another turning point occurs where the denominator is zero, that is,
|
<math>~(n+1)m^{1/n} x^{(n-3)/n } </math> |
<math>~=</math> |
<math>~2c_0 n m</math> |
|
<math>~\Rightarrow ~~~ (n+1)x^{(n-3)/n } </math> |
<math>~=</math> |
<math>~2c_0 n m^{(n-1)/n}</math> |
|
<math>~\Rightarrow ~~~ \frac{x^{n-3}}{ m^{n-1}} </math> |
<math>~=</math> |
<math>~\biggl[ \frac{2c_0 n}{(n+1)} \biggr]^n</math> |
|
|
<math>~=</math> |
<math>~\biggl[ \frac{8\pi}{15} \biggr]^n</math> |
|
<math>~\Rightarrow~~~ m</math> |
<math>~=</math> |
<math>~\biggl[ x^{(n-3)/(n-1)} \biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)}\biggr] \, .</math> |
Plugging this into the virial equilibrium expression gives,
|
<math>~ x^{4} </math> |
<math>~=</math> |
<math>~\biggl[ x^{(n-3)/(n-1)} \biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)}\biggr]^{(n+1)/n} x^{(n-3)/n } - c_0\biggl[ x^{(n-3)/(n-1)} \biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)}\biggr]^2 </math> |
|
|
<math>~=</math> |
<math>~\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{(n-3)(n+1)/[n(n-1)]} \cdot x^{(n-3)/n } - c_0 \biggl( \frac{15}{8\pi} \biggr)^{2n/(n-1)} x^{2(n-3)/(n-1)} </math> |
|
|
<math>~=</math> |
<math>~\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{2(n-3)/(n-1)} - \biggl[ \frac{(n+1)}{2n} \biggr]\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{2(n-3)/(n-1)} </math> |
|
|
<math>~=</math> |
<math>~\biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} x^{2(n-3)/(n-1)} \biggl[1 - \frac{(n+1)}{2n} \biggr] </math> |
|
<math>~\Rightarrow~~~ x^{2(n+1)/(n-1)}</math> |
<math>~=</math> |
<math>~ \frac{(n-1)}{2n} \biggl( \frac{15}{8\pi} \biggr)^{(n+1)/(n-1)} </math> |
|
<math>~\Rightarrow~~~ \frac{R_\mathrm{max}}{R_\mathrm{SWS}}</math> |
<math>~=</math> |
<math>~ \biggl[\frac{(n-1)}{2n} \biggr]^{(n-1)/[2(n+1)]} \biggl( \frac{15}{8\pi} \biggr)^{1/2} \, .</math> |
And the associated mass is,
|
<math>~\frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr|_\mathrm{turn}</math> |
<math>~=</math> |
<math>~\frac{4\pi}{3}\biggl( \frac{15}{8\pi} \biggr)^{n/(n-1)} \biggl\{ \biggl[\frac{(n-1)}{2n} \biggr]^{(n-1)/[2(n+1)]} \biggl( \frac{15}{8\pi} \biggr)^{1/2} \biggr\}^{(n-3)/(n-1)} </math> |
|
|
<math>~=</math> |
<math>~\frac{4\pi}{3}\biggl( \frac{15}{8\pi} \biggr)^{3/2} \biggl[\frac{(n-1)}{2n} \biggr]^{(n-3)/[2(n+1)]} \, .</math> |
Case P Summary
|
Case P | |||||||
|---|---|---|---|---|---|---|---|
|
Order-of-Magnitude Analysis: Assume <math>~{\tilde\mathfrak{f}}_M = {\tilde\mathfrak{f}}_W = {\tilde\mathfrak{f}}_A = 1</math> | |||||||
|
Virial Equilibrium: |
|
||||||
|
Dynamical Instability: <math>~(n > 3)</math> |
|
||||||
|
Turning Point <math>~(M_\mathrm{max} )</math>: <math>~(n>3)</math> |
|
||||||
|
Turning Point <math>~(R_\mathrm{max} )</math>: <math>~(n>1)</math> |
|
||||||
The right-hand panel of Figure 2 presents substantial segments of Case P virial equilibrium sequences for a range of polytropic indexes (n = 1, 2, 2.8, 3, 3.5, 4, 5). For each sequence, the location of the <math>~R_\mathrm{max}</math> and <math>~M_\mathrm{max}</math> turning points — if they exist — are denoted by a yellow or red circular dot, respectively. The point along each <math>~(n \geq 3)</math> sequence at which the transition from dynamically stable to dynamically unstable structures occurs coincides with the location of <math>~M_\mathrm{max}</math> (i.e., with the red circular dot).
For display purposes, all normalized masses <math>~(M_\mathrm{tot}/M_\mathrm{SWS})</math> have been further normalized to the maximum mass on the n = 3 sequence.
Detailed Force-Balance Models
Structural Form Factors
The following table of structural form factors has been drawn from here,
|
Structural Form Factors for Isolated Polytropes |
Structural Form Factors for Pressure-Truncated Polytropes |
||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
|
and here,
|
Structural Form Factors for Pressure-Truncated n = 5 Polytropes | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ||||||||||||
Case M Equilibrium Conditions
Employing the renormalization factors,
|
<math>~\frac{R_\mathrm{Horedt}}{R_\mathrm{norm}}</math> |
<math>~=</math> |
<math>~\biggl[ \frac{4\pi}{(n+1)^n}\biggr]^{1/(n-3)} \, ,</math> |
|
<math>~\frac{P_\mathrm{Horedt}}{P_\mathrm{norm}}</math> |
<math>~=</math> |
<math>~\biggl[ \frac{(n+1)^3}{4\pi}\biggr]^{(n+1)/(n-3)} \, ,</math> |
we find from detailed force-balance analyses that the equilibrium radius and corresponding external pressure for "Case M" configurations are,
|
<math> ~\frac{R_\mathrm{eq}}{R_\mathrm{norm}} = \frac{R_\mathrm{eq}}{R_\mathrm{Horedt}} \cdot \frac{R_\mathrm{Horedt}}{R_\mathrm{norm}} </math> |
<math>~=~</math> |
<math>~\biggl[ \frac{4\pi}{(n+1)^n}\biggr]^{1/(n-3)} \tilde\xi ( -\tilde\xi^2 \tilde\theta' )^{(1-n)/(n-3)} \, , </math> |
which matches the expression derived in an ASIDE box found with our introduction of the Lane-Emden equation, and
|
<math> ~\frac{P_\mathrm{e}}{P_\mathrm{norm}} = \frac{P_\mathrm{e}}{P_\mathrm{Horedt}} \cdot \frac{P_\mathrm{Horedt}}{P_\mathrm{norm}} </math> |
<math>~=~</math> |
<math>~\biggl[ \frac{(n+1)^3}{4\pi}\biggr]^{(n+1)/(n-3)} \tilde\theta_n^{n+1}( -\tilde\xi^2 \tilde\theta' )^{2(n+1)/(n-3)} \, . </math> |
There are two turning points: One associated with a maximum in <math>~P_e</math> and one associated with a maximum in <math>~R_\mathrm{eq}</math>. According to Kimura's discussion, the first of these occurs in the configuration for which,
|
<math>~\frac{\tilde\theta^{n+1}}{(\tilde\theta^')^2} </math> |
<math>~=</math> |
<math>~ \frac{(n-3)}{2} </math> |
… |
For n = 5, this occurs when <math>~\tilde\xi = 3</math> |
This point along the equilibrium sequence is identified by the dark green circular dot in Figure 3, below. The second occurs in the configuration for which,
|
<math>~\frac{\tilde\xi \tilde\theta^{n}}{(-\tilde\theta^')} </math> |
<math>~=</math> |
<math>~ \frac{(n-3)}{(n-1)} </math> |
… |
For n = 5, this occurs when <math>~\tilde\xi = \sqrt{15} \approx 3.87298</math> |
This point along the equilibrium sequence is identified by the yellow circular dot in Figure 3, below. In addition, we have identified the point of dynamical instability.
|
<math>~\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4</math> |
<math>~=</math> |
<math>~\frac{(n-3)}{3^2(n+1)} \cdot \frac{a}{c} = \frac{1}{3^3} \cdot \frac{3}{2^2\pi} \biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)^{-1} \frac{3}{5} \cdot \frac{\tilde\mathfrak{f}_W}{\tilde\mathfrak{f}_M^2}</math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{2^2\cdot 3 \cdot 5\pi} \biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)^{-1} \frac{5}{2^4} (1+\ell^2)^3 \cdot \ell^{-5} \biggl[ \ell \biggl( \ell^4 - \frac{8}{3} \ell^2 -1\biggr) (1+\ell^2)^{-3} + \tan^{-1}\ell \biggr] </math> |
|
<math>~\Rightarrow ~~~ \biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4</math> |
<math>~=</math> |
<math>~ \frac{1}{2^6\cdot 3 \cdot \pi \ell^5} \biggl[ \ell \biggl( \ell^4 - \frac{8}{3} \ell^2 -1\biggr) + (1+\ell^2)^3 \tan^{-1}\ell \biggr] </math> |
But the equilibrium condition for n = 5 configurations is,
|
<math> ~\biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4 </math> |
<math>~=~</math> |
<math>~ \biggl[ \frac{(n+1)^3}{4\pi}\biggr]^{(n+1)/(n-3)} \tilde\theta_n^{n+1}( -\tilde\xi^2 \tilde\theta' )^{2(n+1)/(n-3)} \biggl\{ \biggl[ \frac{4\pi}{(n+1)^n}\biggr]^{1/(n-3)} \tilde\xi ( -\tilde\xi^2 \tilde\theta' )^{(1-n)/(n-3)} \biggr\}^4 </math> |
|
|
<math>~=~</math> |
<math>~ \biggl[ \frac{2 \cdot 3^3}{\pi}\biggr]^{3} \tilde\theta_n^{6}\tilde\xi^{12}( - \tilde\theta' )^{6} \biggl\{ \frac{1}{6}\biggl[ \frac{\pi}{2\cdot 3^3}\biggr]^{1/2} \tilde\xi^{-3} ( - \tilde\theta' )^{-2} \biggr\}^4 </math> |
|
|
<math>~=~</math> |
<math>~\frac{1}{2^4\cdot 3^4} \biggl[ \frac{2 \cdot 3^3}{\pi}\biggr] \tilde\theta_n^{6} ( - \tilde\theta' )^{-2} </math> |
|
|
<math>~=~</math> |
<math>~\frac{1}{2^3\cdot 3\pi} (1+\ell^2)^{-3} \biggl[\frac{\ell}{3^{1/ 2}} (1+\ell^2)^{-3/2} \biggr]^{-2} </math> |
|
|
<math>~=~</math> |
<math>~\frac{1}{2^3\pi} \cdot \ell^{-2} </math> |
Putting the two expressions together gives,
|
<math>~\frac{1}{2^3\pi} \cdot \ell^{-2}</math> |
<math>~=</math> |
<math>~ \frac{1}{2^6\cdot 3 \cdot \pi \ell^5} \biggl[ \ell \biggl( \ell^4 - \frac{8}{3} \ell^2 -1\biggr) + (1+\ell^2)^3 \tan^{-1}\ell \biggr] </math> |
|
<math>~\Rightarrow ~~~2^3\cdot 3 \cdot \ell^{3}</math> |
<math>~=</math> |
<math>~ \ell^5 - \frac{8}{3}\ell^3 - \ell + (1+\ell^2)^3 \tan^{-1}\ell </math> |
|
<math>~\Rightarrow ~~~\biggl[\frac{(1+\ell^2)^3}{\ell}\biggr] \tan^{-1}\ell </math> |
<math>~=</math> |
<math>~ 1 + \biggl[ \frac{2^4\cdot 5}{3} \biggr] \ell^{2} - \ell^4 </math> |
This agrees with the expression derived in a separate ASIDE; as was pointed out in that context, the root of this equation is: <math>~\ell \approx 2.223175</math>, that is, <math>~\tilde\xi \approx 3.85065</math>. This point along the equilibrium sequence is identified by the red circular dot in Figure 3, below. It is almost — but definitely not — coincident with the configuration along the sequence (marked by the yellow circular dot) that is associated with the minimum-radius turning point.
Now for a movie!
Case P Equilibrium Conditions
The equilibrium radius and corresponding configuration mass from a "Case P" analysis are,
|
<math> ~\frac{R_\mathrm{eq}}{R_\mathrm{SWS} } </math> |
<math>~=~</math> |
<math> \biggl( \frac{n}{4\pi} \biggr)^{1/2} \tilde\xi {\tilde\theta}_n^{(n-1)/2} \, , </math> |
|
<math> ~\frac{M_\mathrm{tot}}{M_\mathrm{SWS} } </math> |
<math>~=~</math> |
<math> \biggl( \frac{n^3}{4\pi} \biggr)^{1/2} {\tilde\theta}_n^{(n-3)/2} (-\tilde\xi^2 \tilde\theta^') \, . </math> |
According to our review of, especially, Kimura's work, the turning point associated with <math>~M_\mathrm{max}</math> occurs where,
|
<math>~\frac{\tilde\theta^{n+1}}{(\tilde\theta^')^2} </math> |
<math>~=</math> |
<math>~ \frac{(n-3)}{2} </math> |
… |
For n = 5, this occurs when <math>~\tilde\xi = 3 \, .</math> |
And a turning point associated with <math>~R_\mathrm{max}</math> occurs where,
|
<math>~\frac{\tilde\xi (-\tilde\theta^')}{\tilde\theta}</math> |
<math>~=</math> |
<math>~\frac{2}{(n-1)} \, .</math> |
For <math>~n=5</math> configurations, this means,
|
<math>~\frac{1}{2} </math> |
<math>~=</math> |
<math>~ \tilde\xi \biggl( 1 + \frac{\tilde\xi^2}{3}\biggr)^{1/ 2} \frac{\tilde\xi}{3} \biggl( 1 + \frac{\tilde\xi^2}{3}\biggr)^{-3/ 2} </math> |
|
|
<math>~=</math> |
<math>~ \frac{\ell^2}{(1+\ell^2)} </math> |
|
<math>~\Rightarrow ~~~ (1+\ell^2)</math> |
<math>~=</math> |
<math>~ 2\ell^2 </math> |
|
<math>~\Rightarrow ~~~ \ell^2</math> |
<math>~=</math> |
<math>~ 1 </math> |
|
<math>~\Rightarrow ~~~ \tilde\xi</math> |
<math>~=</math> |
<math>~ \sqrt{3} \, . </math> |
Five-One Bipolytropes
Basic Properties
For bipolytropes, in general, let <math>~n =n_c</math> and <math>~j = n_e</math>. The statement of virial equilibrium is, then,
|
<math>~\frac{ b}{n_c c}\cdot x^{(n_c-3)/n_c }_\mathrm{eq} - \frac{a}{3c} + \frac{1}{n_e}\cdot x^{(n_e-3)/n_e}_\mathrm{eq} </math> |
<math>~=</math> |
<math>~ 0 \, .</math> |
And the critical equilibrium configuration has,
|
<math>~[x_\mathrm{eq}^{(n_e-3)/n_e}]_\mathrm{crit} </math> |
<math>~=</math> |
<math>~ \frac{a}{3^2c}\biggl[ \frac{n_e^2(n_c-3)}{n_c-n_e} \biggr] \, . </math> |
Here we choose to set <math>~n_c = 5</math> and <math>~n_e = 1</math>. Hence, these two conditions become, respectively,
|
<math>~\frac{ b}{5 c}\cdot x^{2/5 }_\mathrm{eq} + x^{-2}_\mathrm{eq} </math> |
<math>~=</math> |
<math>~ \frac{a}{3c} \, ,</math> |
and,
|
<math>~[x_\mathrm{eq}]_\mathrm{crit} </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{2\cdot 3^2c}{a} \biggr]^{1/2} \, . </math> |
Comparing the general free-energy expression at the beginning of this chapter with the free-energy expression provided via our accompanying summary discussion of five-one bipolytropes, we find that,
|
<math>~a</math> |
<math>~=</math> |
<math>~(3\mathfrak{L}_i +12\mathfrak{K}_i) x_\mathrm{eq} \biggl[ \biggl( \frac{3^6}{2^5\pi} \biggr)^{1/2} \frac{\ell_i^3}{(1+\ell_i^2)^3} \biggr] </math> |
|
<math>~b</math> |
<math>~=</math> |
<math>~(5 \mathfrak{L}_i) x_\mathrm{eq}^{3/5} \biggl[ \biggl( \frac{3^6}{2^5\pi} \biggr)^{1/2} \frac{\ell_i^3}{(1+\ell_i^2)^3} \biggr] </math> |
|
<math>~c</math> |
<math>~=</math> |
<math>~(4\mathfrak{K}_i) x_\mathrm{eq}^{3} \biggl[ \biggl( \frac{3^6}{2^5\pi} \biggr)^{1/2} \frac{\ell_i^3}{(1+\ell_i^2)^3} \biggr] </math> |
where,
|
<math>~\mathfrak{L}_i</math> |
<math>~\equiv</math> |
<math>~\frac{(\ell_i^4-1)}{\ell_i^2} + \frac{(1+\ell_i^2)^3}{\ell_i^3} \cdot \tan^{-1}\ell_i \, ,</math> |
|
<math>~\mathfrak{K}_i</math> |
<math>~\equiv</math> |
<math>~\frac{\Lambda_i}{\eta_i} + \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] \, ,</math> |
|
<math>~\Lambda_i</math> |
<math>~\equiv</math> |
<math>~\frac{1}{\eta_i} - \ell_i \, ,</math> |
|
<math>~\eta_i</math> |
<math>~=</math> |
<math>~3 \biggl( \frac{\mu_e}{\mu_c} \biggr) \biggl[\frac{\ell_i }{(1+\ell_i^2)}\biggr] \, ,</math> |
and,
|
<math>~x_\mathrm{eq} = \frac{R_\mathrm{eq}}{R_\mathrm{norm}}</math> |
<math>~=</math> |
<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} </math> |
|
|
<math>~=</math> |
<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{(1+\ell_i^2)^3}{3^3\ell_i^3} \biggl[ \frac{1 }{(1+\Lambda_i^2)} \biggr] \biggl\{ 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \biggr\}^{-1}</math> |
In rewriting this last expression, we have made use of the two relations,
|
<math>~q \equiv \frac{r_i}{R}</math> |
<math>~=</math> |
<math>~\biggl\{ 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \biggr\}^{-1} \, ,</math> |
|
<math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}}</math> |
<math>~=</math> |
<math>~ \frac{\ell_i q}{(1+\Lambda_i^2)^{1/2}} \, . </math> |
Consistent with our generic discussion of the stability of bipolytropes and the specific discussion of the stability of bipolytropes having <math>~(n_c, n_e) = (5, 1)</math>, it can straightforwardly be shown that <math>~\partial \mathfrak{G}/\partial \chi = 0</math> is satisfied by setting <math>~\Chi = 1</math>; that is, the equilibrium condition is,
Furthermore, the equilibrium configuration is unstable whenever <math>~\partial^2 \mathfrak{G}/\partial \chi^2 < 0</math>, that is, the transition from stable to unstable configurations whenever,
|
<math>~\frac{ \mathfrak{L}_i}{\mathfrak{K}_i}</math> |
<math>~=</math> |
<math>~20 \, .</math> |
Table 1 of an accompanying chapter — and the red-dashed curve in the figure adjacent to that table — identifies some key properties of the model that marks the transition from stable to unstable configurations along equilibrium sequences that have various values of the mean-molecular weight ratio, <math>~\mu_e/\mu_c</math>.
Coincidence Between Points of Secular and Dynamical Instability
From the accompanying graphical display of equilibrium sequences, it seems that a <math>~\nu_\mathrm{max}</math> turning point will only exist in five-one bipolytropes for <math>~\mu_e/\mu_c</math> less than some value — call it, <math>~(\mu_e/\mu_c)_\mathrm{begin}</math> — which is less than but approximately equal to <math>~\tfrac{1}{3}</math>. As we move along any sequence for which <math>~\mu_e/\mu_c < (\mu_e/\mu_c)_\mathrm{begin}</math>, in the direction of increasing <math>~\ell_i</math>, it is fair to ask whether the system becomes dynamically unstable (at <math>~[x_\mathrm{eq}]_\mathrm{crit}</math>) before or after it encounters the point of secular instability marked by <math>~\nu_\mathrm{max}</math>.

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |