User:Tohline/SSC/Stability/n3PolytropeLAWE

Radial Oscillations of n = 3 Polytropic Spheres

Background

Our Formulation of the Problem

In an accompanying discussion, we derived the so-called,

Adiabatic Wave (or Radial Pulsation) Equation

<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math>

whose solution gives eigenfunctions that describe various radial modes of oscillation in spherically symmetric, self-gravitating fluid configurations. Because this widely used form of the radial pulsation equation is not dimensionless but, rather, has units of inverse length-squared, we have found it useful to also recast it in the following dimensionless form:

<math> \frac{d^2x}{d\chi_0^2} + \biggl[\frac{4}{\chi_0} - \biggl(\frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{P_0}{P_c}\biggr)^{-1} \biggl(\frac{g_0}{g_\mathrm{SSC}}\biggr) \biggr] \frac{dx}{d\chi_0} + \biggl(\frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{P_0}{P_c}\biggr)^{-1} \biggl(\frac{1}{\gamma_\mathrm{g}} \biggr)\biggl[\tau_\mathrm{SSC}^2 \omega^2 + (4 - 3\gamma_\mathrm{g})\biggl(\frac{g_0}{g_\mathrm{SSC}}\biggr) \frac{1}{\chi_0} \biggr] x = 0 , </math>

where,

<math>~g_\mathrm{SSC} \equiv \frac{P_c}{R\rho_c} \, ,</math>       and       <math>~\tau_\mathrm{SSC} \equiv \biggl[\frac{R^2 \rho_c}{P_c}\biggr]^{1/2} \, .</math>

In a separate discussion, we showed that specifically for isolated, polytropic configurations, this linear adiabatic wave equation (LAWE) can be rewritten as,

<math>~0 </math>	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[\frac{4 - (n+1)V(\xi)}{\xi} \biggr] \frac{dx}{d\xi} + \biggl[\frac{\omega^2}{\gamma_g \theta} \biggl(\frac{n+1 }{4\pi G \rho_c} \biggr) - \biggl(3-\frac{4}{\gamma_g}\biggr) \cdot \frac{(n+1)V(x)}{\xi^2} \biggr] x </math>
	<math>~=</math>	<math>~\frac{d^2x}{d\xi^2} + \biggl[\frac{4}{\xi} - \frac{(n+1)}{\theta} \biggl(- \frac{d\theta}{d\xi}\biggr)\biggr] \frac{dx}{d\xi} + \frac{(n+1)}{\theta}\biggl[\frac{\sigma_c^2}{6\gamma_g } - \frac{\alpha}{\xi} \biggl(- \frac{d\theta}{d\xi}\biggr)\biggr] x \, ,</math>

where we have adopted the dimensionless frequency notation,

<math>~\sigma_c^2</math>

<math>~\equiv</math>

<math>~\frac{3\omega^2}{2\pi G \rho_c} \, .</math>

In this chapter we carry out a numerical integration of this governing LAWE for <math>~n=3</math> polytropes. The results are presented below.

Schwarzschild (1941)

We can directly compare our results with Schwarzschild's (1941) published work on "Overtone Pulsations for the Standard [Stellar] Model." To begin with, it is straightforward to demonstrate that the last form of the LAWE, provided above, matches equation (2) from Schwarzschild (1941), if <math>~n</math> is set to 3; note as well that Schwarzschild's dimensionless oscillation frequency — defined in his equation (1) and which we will label, <math>~\omega_\mathrm{Sch}</math> — is related to our dimensionless frequency via the expression,

<math>~\sigma_c^2</math>

<math>~~\leftrightarrow~~</math>

<math>~\biggl( \frac{3\gamma_g}{2} \biggr) \omega_\mathrm{Sch}^2 \, .</math>

Paragraph extracted from M. Schwarzschild (1941) "Overtone Pulsations for the Standard Model" ApJ, vol. 94, pp. 245 - 252 © American Astronomical Society

3A. S. Eddington (1930), The Internal Constitution of the Stars, pp. 188 and 192.

Schwarzschild (1941) numerically integrated the LAWE for <math>~n=3</math> polytropic spheres to find eigenvectors (i.e., the spatially discrete eigenfunction and corresponding eigenfrequency) for five separate oscillation modes (the fundamental mode, plus the 1^st, 2^nd, 3^rd, and 4^th overtones) for models having four different adopted adiabatic indexes <math>~\gamma_g = \tfrac{4}{3}, \tfrac{10}{7}, \tfrac{20}{13}, \tfrac{5}{3})</math>. Our Table 1 catalogs the eigenfrequencies that Schwarzschild determined (see his Table 1) for these twenty different models/modes.

Table 1:   From Table 1 of M. Schwarzschild (1941)
Mode	<math>~\alpha = 0.0</math> <math>~(\gamma_g = 4/3)</math>	<math>~\alpha = 0.2</math> <math>~(\gamma_g = 10/7)</math>	<math>~\alpha = 0.4</math> <math>~(\gamma_g = 20/13)</math>		<math>~\alpha = 0.6</math> <math>~(\gamma_g = 5/3)</math>
	<math>~\omega_\mathrm{Sch}^2</math>	<math>~\omega_\mathrm{Sch}^2</math>	<math>~\omega_\mathrm{Sch}^2</math>	<math>~\mathfrak{F} = \biggl[\frac{3\omega_\mathrm{Sch}^2}{2} - 2\alpha \biggr]</math>	<math>~\omega_\mathrm{Sch}^2</math>
0	0.00000	0.05882	0.10391	-0.64414	0.13670
1	0.16643	0.19139	0.21998	-0.47003	0.25090
2	0.3392	0.3648	0.3920	-0.2120	0.4209
3	0.5600	0.5863	0.6136	+0.1204	0.6420
4	0.8283	0.8554	0.8832	+0.5248	0.9117

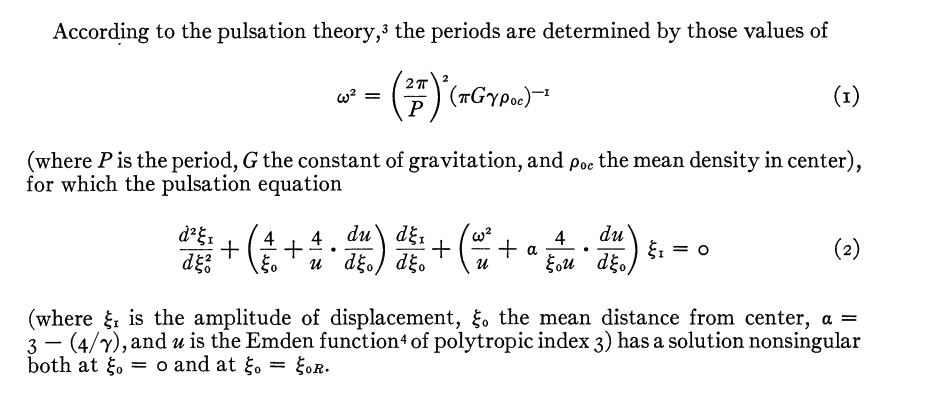
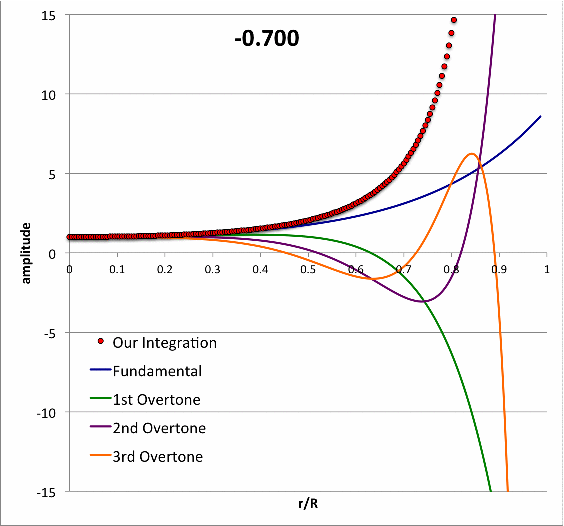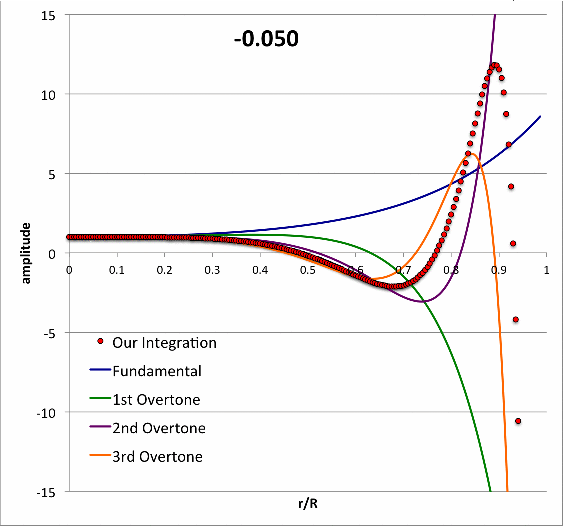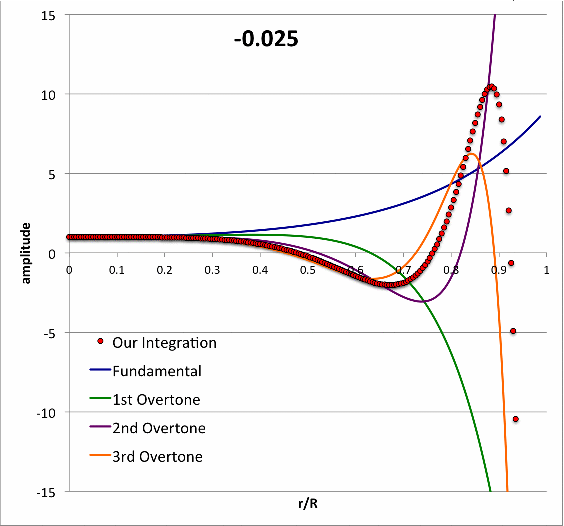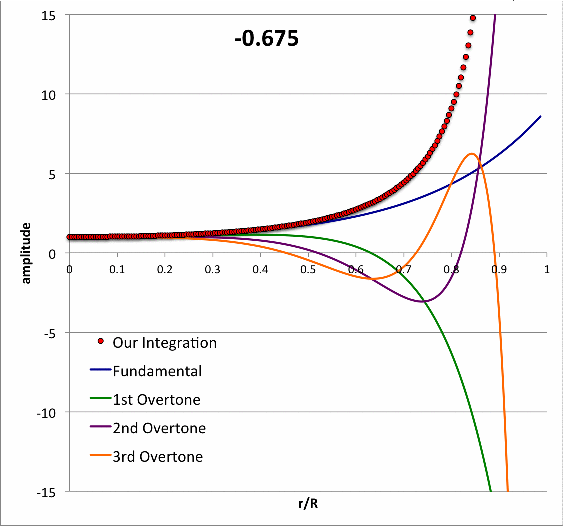
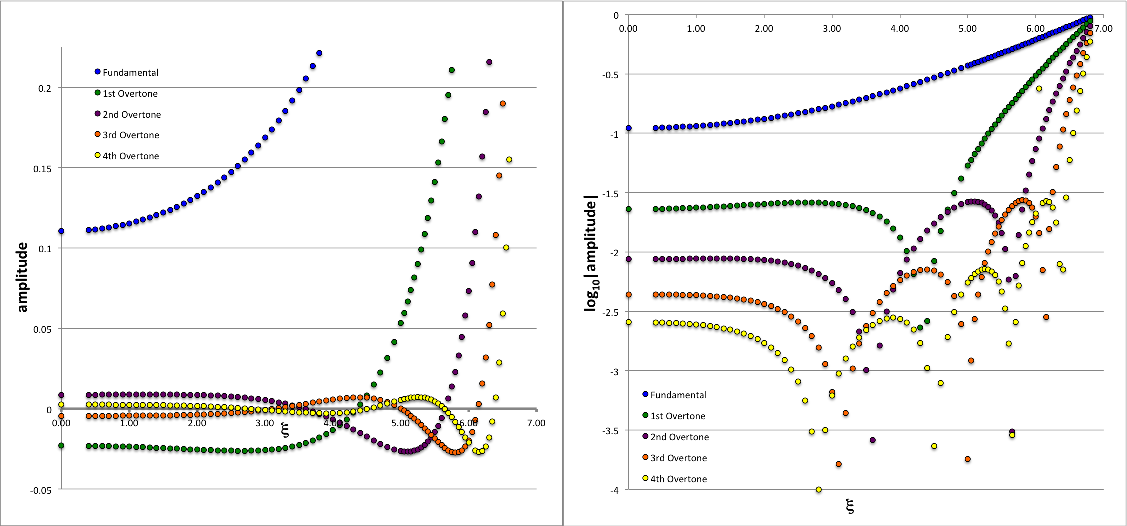
Schwarzschild (1941) also documented the radial structure of the eigenfunction that is associated with each of these twenty model/mode eigenfrequencies. Each column of his Table 4, except the first, presents numerical values of the amplitude of a specific model/mode at 84 discrete radial locations throughout the n = 3 polytrope; the first column of the table lists the corresponding radial coordinate, <math>~\xi</math>. Focusing on the model that he analyzed assuming <math>~\alpha = 0.4</math>, we have typed his five columns of data into an Excel spreadsheet and have used this data to generate the pair of plots displayed, below, in Figure 1. The left-hand panel displays the eigenfunction amplitude versus radius, <math>~x(\xi)</math>, for the fundamental mode as well as for the first four overtones; it essentially replicates Figure 1 from Schwarzschild (1941). The right-hand panel displays the same data, but as a semi-log plot; specifically, it displays <math>~y(\xi)</math>, where,

<math>~y \equiv \frac{1}{2} \log_{10}[x^2 + 10^{-8}] \, .</math>

Each sharp valley in this semi-log plot highlights the location of a node in the corresponding eigenfunction, that is, it identifies where <math>~x(\xi)</math> crosses through zero.

Figure 1:  Schwarzschild's Eigenfunctions for an n = 3 Polytrope with α = 0.4 (γ = 20/13)

Numerical Integration

Here we use the finite-difference algorithm described separately to integrate the discretized LAWE from the center of the polytropic configuration, outward to its surface, which in this case — see, for example, p. 77 of Horedt (2004) — is located at the polytropic-coordinate location,

<math>~\xi_\mathrm{max} = 6.89684862 \, .</math>

It is assumed, at the outset, that we have in hand an appropriately discretized description of the unperturbed, equilibrium properties of an <math>~n=3</math> polytrope; specifically, at each radial grid line, we have tabulated values of the radial coordinate, <math>~0 \le \xi_i \le \xi_\mathrm{max}</math>, the Lane-Emden function, <math>~\theta_i</math>, and its first radial derivative, <math>~\theta_i'</math>.

The algorithm is as follows (substitute <math>~n=3</math> everywhere):

• Establish an equally spaced radial-coordinate grid containing <math>~N</math> grid zones (and, accordingly, <math>~N+1</math> grid lines), in which case the grid-spacing parameter, <math>~\Delta_\xi \equiv \xi_\mathrm{max}/N</math>.
• Specify a value of the adiabatic exponent, <math>~\gamma</math>, which, in turn, determines the value of the parameter, <math>~\alpha \equiv (3-4/\gamma) \, .</math>
• Choose a value for the (square of the) dimensionless oscillation frequency, <math>~\sigma_c^2</math>, which we will accomplish by assigning a value to the parameter,

  <math>~\mathfrak{F} \equiv \frac{\sigma_c^2}{\gamma} - 2\alpha \, .</math>

• Set the eigenfunction to unity at the center <math>~(\xi_0 = 0)</math> of the configuration, that is, set <math>~x_0 = 1</math>.
• Determine the value of the eigenfunction at the first grid line away from the center — having coordinate location, <math>~\xi_1 = \Delta_\xi </math> — via the expression,

  <math>~ x_1 </math>

  <math>~=</math>

  <math>~ x_0 \biggl[ 1 - \frac{\Delta_\xi^2 (n+1) \mathfrak{F}}{12} \biggr] \, .</math>

• At all other grid lines, <math>~i=2,N</math>, determine the value of the eigenfunction, <math>~x_i</math>, via the expression,

  <math>~x_i \biggl[2\theta_{i-1} +\frac{4\Delta_\xi \theta_{i-1}}{\xi_{i-1}} - \Delta_\xi (n+1)(- \theta^')_{i-1}\biggr] </math>

  <math>~=</math>

  <math>~ x_{i-1}\biggl\{4\theta_{i-1} - \frac{\Delta_\xi^2(n+1)}{3}\biggl[ \mathfrak{F}+2\alpha - 2\alpha \biggl(- \frac{3\theta^'}{\xi}\biggr)_{i-1} \biggr] \biggr\} + x_{i-2} \biggl[\frac{4\Delta_\xi \theta_{i-1}}{\xi_{i-1}} - \Delta_\xi (n+1)(- \theta^')_{i-1} - 2\theta_{i-1}\biggr] \, .</math>

Now, in searching for an appropriate boundary condition at the surface of the configuration, it will be useful to tabulate, not only the value of the eigenfunction at the surface, <math>~x_N</math>, but also its logarithmic derivative. A finite-difference expression of this logarithmic derivative that is consistent with the above-described finite-difference algorithm, is,

<math>~\frac{d\ln x}{d\ln \xi} \biggr|_\mathrm{surface}</math>

<math>~\approx</math>

<math>~\frac{\xi_\mathrm{max}}{x_N} \biggl[ \frac{x_{N+1}-x_{N-1}}{2\Delta_\xi} \biggr] \, .</math>

Everything is known here, except for the quantity, <math>~x_{N+1}</math>, which can be evaluated using the last expression in our algorithm one more time to, in effect, evaluate the eigenfunction just outside the surface. That is, we obtain <math>~x_{N+1}</math> and, in turn, obtain a value for the logarithmic derivative at the surface, via the expression,

<math>~x_{N+1} \biggl[2\theta_{N} +\frac{4\Delta_\xi \theta_{N}}{\xi_\mathrm{max}} - \Delta_\xi (n+1)(- \theta^')_{N}\biggr] </math>

<math>~=</math>

<math>~ x_{N}\biggl\{4\theta_{N} - \frac{\Delta_\xi^2(n+1)}{3}\biggl[ \mathfrak{F}+2\alpha - 2\alpha \biggl(- \frac{3\theta^'}{\xi}\biggr)_{N} \biggr] \biggr\} + x_{N-1} \biggl[\frac{4\Delta_\xi \theta_{N}}{\xi_\mathrm{max}} - \Delta_\xi (n+1)(- \theta^')_{N} - 2\theta_{N}\biggr] \, .</math>

Related Discussions

• Radial Oscillations of Uniform-density sphere
• Radial Oscillations of Isolated Polytropes
  • Setup
  • n = 1:  Attempt at Formulating an Analytic Solution
  • n = 3:  Numerical Solution to compare with M. Schwarzschild (1941)
  • n = 5:  Attempt at Formulating an Analytic Solution