User:Tohline/SSC/Structure/PolytropesASIDE1

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
ASIDE: Whitworth's Scaling
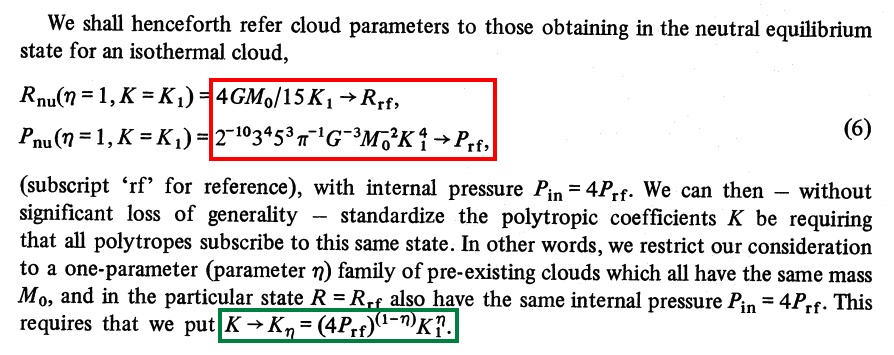
In his study of the "global gravitational stability [of] one-dimensional polytropes," Whitworth (1981, MNRAS, 195, 967) normalizes (or "references") various derived mathematical expressions for configuration radii, <math>R</math>, and for the pressure exerted by an external bounding medium, <math>P_\mathrm{ex}</math>, to quantities he refers to as, respectively, <math>R_\mathrm{rf}</math> and <math>P_\mathrm{rf}</math>. The paragraph from his paper in which these two reference quantities are defined is shown here:
In order to map Whitworth's terminology to ours, we note, first, that he uses <math>M_0</math> to represent the spherical configuration's total mass, which we refer to simply as <math>M</math>; and his parameter <math>\eta</math> is related to our <math>~n</math> via the relation,
<math>\eta = 1 + \frac{1}{n} \, .</math>
Hence, Whitworth writes the polytropic equation of state as,
<math>P = K_\eta \rho^\eta \, ,</math>
whereas, using our standard notation, this same key relation is written as,
<math>~P = K_\mathrm{n} \rho^{1+1/n}</math> ;
and his parameter <math>K_\eta</math> is identical to our <math>~K_\mathrm{n}</math>.
According to the second (bottom) expression identified by the red outlined box drawn above,
<math> P_\mathrm{rf} = \frac{3^4 5^3}{2^{10} \pi} \biggl( \frac{K_1^4}{G^3 M^2} \biggr) \, , </math>
and inverting the expression inside the green outlined box gives,
<math> K_1 = \biggl[ K_n (4 P_\mathrm{rf})^{\eta - 1} \biggr]^{1/\eta} \, . </math>
Hence,
<math> P_\mathrm{rf} = \frac{3^4 5^3}{2^{10} \pi} \biggl( \frac{1}{G^3 M^2} \biggr)\biggl[ K_n (4 P_\mathrm{rf})^{\eta - 1} \biggr]^{4/\eta} \, , </math>
or, gathering all factors of <math>P_\mathrm{rf}</math> to the left-hand side,
<math> P_\mathrm{rf}^{(4-3\eta)} = 2^{-2(4+\eta)} \biggl( \frac{3^4 5^3}{\pi} \biggr)^\eta \biggl[ \frac{K_n^4}{G^{3\eta} M^{2\eta}} \biggr] \, . </math>
Analogously, according to the first (top) expression identified inside the red outlined box,
<math> R_\mathrm{rf} = \frac{2^2 GM}{3\cdot 5 K_1} = 2^{2/\eta} \biggl( \frac{GM}{3\cdot 5}\biggr) K_n^{-1/\eta} P_\mathrm{rf}^{(1-\eta)/\eta} ~~~~\Rightarrow~~~~ R_\mathrm{rf}^\eta = \frac{2^{2}}{K_n} \biggl( \frac{GM}{3\cdot 5}\biggr)^\eta P_\mathrm{rf}^{(1-\eta)} \, . </math>
| Examples | |||
|
<math>~n</math> |
<math>\eta = 1+1/n</math> |
<math>P_\mathrm{rf}</math> |
<math>R_\mathrm{rf}</math> |
|
1 |
2 |
<math>\frac{2^{6}\pi}{3^4 5^3} \biggl[ \frac{G^{3} M^{2} }{K^2}\biggr]</math> |
<math>\biggl[ \frac{3^2 5}{2^4 \pi} \biggl( \frac{K}{G} \biggr) \biggr]^{1/2}</math> |
|
5 |
6/5 |
<math>\frac{3^{12} 5^{9}}{2^{26} \pi^3} \biggl[ \frac{K^{10}}{G^9 M^6} \biggr]</math> |
<math>\biggl[ \frac{2^{12} \pi}{3^6 5^5} \biggl( \frac{G^5 M^4}{K^5} \biggr) \biggr]^{1/2}</math> |
|
<math>\infty</math> |
1 |
<math> \frac{3^4 5^3}{2^{10}\pi} \biggl[ \frac{K^4}{G^{3} M^{2} }\biggr]</math> |
<math>\frac{2^2GM}{3\cdot 5 K}</math> |

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |